In mathematics

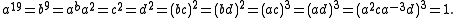
Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, the third Janko group J₃, also known as the Higman-Janko-McKay group, is a finite

Finite group

In mathematics and abstract algebra, a finite group is a group whose underlying set G has finitely many elements. During the twentieth century, mathematicians investigated certain aspects of the theory of finite groups in great depth, especially the local theory of finite groups, and the theory of...

 simple

Simple group

In mathematics, a simple group is a nontrivial group whose only normal subgroups are the trivial group and the group itself. A group that is not simple can be broken into two smaller groups, a normal subgroup and the quotient group, and the process can be repeated...

 sporadic group

Sporadic group

In the mathematical field of group theory, a sporadic group is one of the 26 exceptional groups in the classification of finite simple groups. A simple group is a group G that does not have any normal subgroups except for the subgroup consisting only of the identity element, and G itself...

 of order 50232960. Evidence for its existence was uncovered by , and it was shown to exist by . Janko predicted both J₃ and J₂ as simple groups having 2¹⁺⁴:A₅ as a centralizer of an involution.

J₃ has an outer automorphism group

Outer automorphism group

In mathematics, the outer automorphism group of a group Gis the quotient Aut / Inn, where Aut is the automorphism group of G and Inn is the subgroup consisting of inner automorphisms. The outer automorphism group is usually denoted Out...

 of order 2 and a Schur multiplier

Schur multiplier

In mathematical group theory, the Schur multiplier or Schur multiplicator is the second homology group H_2 of a group G.It was introduced by in his work on projective representations.-Examples and properties:...

 of order 3, and its triple cover has a unitary 9 dimensional representation

Modular representation theory

Modular representation theory is a branch of mathematics, and that part of representation theory that studies linear representations of finite group G over a field K of positive characteristic...

 over the field with 4 elements. constructed it via an underlying geometry. and it has a modular representation of dimension eighteen over the finite field of nine elements.

J₃ is one of the 6 sporadic simple groups called the pariahs

Pariah group

In mathematical group theory, the term pariah was introduced by to refer to the six sporadic simple groups that are not subquotients of the monster simple group.These groups are:*Three of the Janko groups: J1, J3, and J4.*The Lyons group...

, because showed that it is not found within the Monster group

Monster group

In the mathematical field of group theory, the Monster group M or F1 is a group of finite order:...

.

Presentations

In terms of generators a, b, c, and d its automorphism group J₃:2 can be presented as


A presentation for J₃ in terms of (different) generators a, b, c, d is

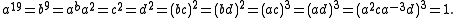
Maximal subgroups

showed that J₃ has 9 conjugacy classes of maximal subgroups:

• PSL(2,16):2, order 8160
• PSL(2,19), order 3420
• PSL(2,19), conjugate to preceding class in J₃:2
• 2⁴:(3 × A₅), order 2880
• PSL(2,17), order 2448
• (3 × A₆):2₂, order 2160 - normalizer of subgroup of order 3
• 3²⁺¹⁺²:8, order 1944 - normalizer of Sylow 3-subgroup
• 2¹⁺⁴:A₅, order 1920 - centralizer of involution
• 2²⁺⁴:(3 × S₃), order 1152

External links

• Atlas of Finite Group Representations: J₃ version 2
• Atlas of Finite Group Representations: J₃ version 3