Jordanus Nemorarius
Encyclopedia
Jordanus de Nemore was a thirteenth-century European mathematician
who wrote treatises on at least 6 different important mathematical subjects: the science of weights; “algorismi” treatises on practical arithmetic; pure arithmetic; algebra; geometry; and stereographic projection. Most of these treatises exist in several versions or reworkings from the Middle Ages. We know nothing about him personally, other than the approximate date of his work.
his name was often given as "Jordanus Nemorarius", an improper form. We know nothing of his nationality.
An entry in the nineteenth-century manuscript catalogue for the Sächsische Landesbibliothek in Dresden
suggested that Jordanus taught at the University of Toulouse
, but the text in question was not written by Jordanus and this possible association is without foundation. A fourteenth-century chronicle of the Order of Preachers by the Englishman Nicholas Trivet
(or Triveth, 1258–1328) suggested that the second master-general of the Dominican Order
, Jordanus of Saxony (d. 1237) wrote two mathematical texts with titles similar to two by Jordanus de Nemore, but this late suggestion is more likely a confusion on the part of Trivet, rather than any proof of identity. Jordanus of Saxony never uses the name “de Nemore” and is nowhere else credited with mathematical writings – in fact he had lectured in theology at the University of Paris
. Likewise the name of Jordanus of Saxony is never found with a mathematical text. This identity, popular among some in the nineteenth and twentieth centuries, has been for the most part abandoned.
It is assumed that Jordanus did work in the first part of the thirteenth century (or even in the late twelfth) since his works are contained in a booklist, the Biblionomia of Richard de Fournival
, compiled between 1246 and 1260.
) owes much of its importance to the work of Jordanus. In the Elementa super demonstrationem ponderum, he introduces the concept of “positional gravity” and the use of component forces. Pierre Duhem
(in his Origines de la statique, 1905) thought that Jordanus also introduces infinitesimal considerations into statics
in his discussion of "virtual" displacements (this being another interpretation of Duhem) of objects in equilibrium. He proves the law of the lever by means of the principle of work. The De ratione ponderis also proves the conditions of equilibrium of unequal weights on planes inclined at different angles – long before Galileo
.
The Elementa super demonstrationem ponderum seems to be the one work which can definitely be ascribed to Jordanus; and the first of the series. Jordanus took what Joseph Brown has called the "Logician’s Abstract of On the Karaston" (a skillful compression of the conclusions of Thābit ibn Qurra
’s Liber karastonis) and created a new treatise (7 axioms and 9 propositions) in order to establish a mathematical basis for the four propositions on the Roman
balance called the Liber de canonio. An early commentary on this (which also contains a necessary correction to Proposition 9) is the “Corpus Christi Commentary”.
The Liber de ponderibus fuses the seven axioms and nine propositions of the Elementa to the four propositions of the De canonio. There are at least two commentary traditions to the Liber de ponderibus which improve some of the demonstrations and better integrate the two sources.
The De ratione ponderis is a skillfully corrected and expanded version (45 propositions) of the Elementa. This is usually ascribed to Jordanus, but more likely it is the work of an unidentified mathematician because the citations by Jordanus of his other works are deleted.
Related to these treatises is an anonymous set of comments, each of which begins with the words “Aliud commentum” (and thus known as the “Aliud commentum” version). This commentary surpasses all others, especially the commentary on Proposition 1.
i treatises in this category, examined by Gustaf Eneström
early in the twentieth century, dealing with practical arithmetic
.
The Communis et consuetus (its opening words) appears to be the earliest form of the work, closely related to the much expanded Demonstratio de algorismo. Eneström believed that the Communis et consuetus was certainly by Jordanus.
The later Demonstratio de algorismo contains 21 definitions and 34 propositions. This is probably a later version of the Communis et consuetus, made either by Jordanus himself or by some other thirteenth-century mathematician.
The Tractatus minutiarum on fractions seems to be a second part of the Communis et consuetus – they are often found together in the manuscripts.
The Demonstratio de minutiius likewise is linked to the Demonstratio de algorismo, and contains and expands the propositions found in the Tractatus minutiarum – again a re-edition of the original text.
The Algorismus demonstratus is a spurious attribution although for a long time this item was ascribed to Jordanus. Up until Eneström began to sort out the various treatises, the Algorismus demonstratus – since it was the only one published (ed. Johannes Schöner
, Nuremberg, 1543) – was the heading under which all the treatises were grouped. Eneström thought it highly unlikely, however, that this version was the work of Jordanus since no manuscript ascribes it to him (if they give an author, it is generally a Magister Gernarus, or Gerhardus or Gernandus). The first part of this treatise (also known as the Algorismus de integris) contains definitions, axioms and 43 propositions. The second part (the Algorismus de minutiis) contains definitions and 42 propositions. Eneström shows that while different from the algorismi treatises of Jordanus, the Algorismus demonstratus is still closely related to them.
contains over 400 propositions divided into ten books. There are three versions or editions in manuscript form, the second one with different or expanded proofs than found in the first, and a number of propositions added at the end; the third version inserts the added propositions into their logical position in the text, and again changed some of the proofs. Jordanus’ aim was to write a complete summary of arithmetic, similar to what Euclid
had done for geometry
.
Jordanus collected and organized the whole field of arithmetic, based both on Euclid’s work and on that of Boethius
. Definitions, axioms and postulates lead to propositions with proofs which are somewhat sketchy at times, leaving the reader to complete the argument. Here also Jordanus uses letters to represent numbers, but numerical examples, of the type found in the De numeris datis, are not given.
, Barnabas Hughes, has found two sets of manuscripts for this text, one containing 95 propositions, the other, 113. As well some of the common propositions have different proofs. There are also 4 digests or revisions in manuscript form.
Jordanus’ De numeris datis was the first treatise in advanced algebra composed in Western Europe, building on elementary algebra provided in twelfth-century translations from Arabic sources. It anticipates by 350 years the introduction of algebraic analysis by François Viète
into Renaissance
mathematics
. Jordanus used a system similar to that of Viète (although couched on non-symbolic terms) of formulating the equation (setting out the problem in terms of what is known and of what is to be found), of transforming the initial given equation into a solution, and the introduction of specific numbers that fulfil the conditions set by the problem.
at its best. It contains propositions on such topics as the ratios of sides and angles of triangles; the division of straight lines, triangles, and quadrangles under different conditions; the ratio of arcs and plane segments in the same or in different circles; trisecting an angle; the area of triangles given the length of the sides; squaring the circle.
Again there are two versions of this text: the shorter and presumably first edition (the Liber philotegni Iordani de Nemore) and a longer version (Liber de triangulis Iordani) which divides the text into books, re-arranges and expands book 2, and adds propositions 4-12 to 4-28. This latter set of 17 propositions also circulated separately. While the longer version may not be by Jordanus, it was certainly complete by the end of the thirteenth century.
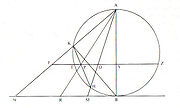This treatise of five propositions deals with various aspects of stereographic projection
(used in planispheric astrolabes). The first and historically the most important proposition proves for all cases that circles on the surface of a sphere when projected stereographically on a plane remain circles (or a circle of infinite radius, i.e., a straight line). While this property was known long before Jordanus, it had never been proved.
There are three versions of the treatise: the basic text, a second version with an introduction and a much expanded text, and a third, only slightly expanded. The introduction is sometimes found with version 1 and 3, but it was obviously written by someone else.
engraving), and the Pre-exercitamina (“a short introductory exercise”?) are dubiously ascribed to Jordanus. A number of other texts including a Liber de speculis and a Compositum astrolabii are spurious ascriptions.
1. Mechanics: The three main treatises and the “Aliud commentum” version (Latin and English) are published in The Medieval Science of Weights, ed. Ernest A. Moody and Marshall Clagett (Madison: University of Wisconsin Press, 1952). The commentaries are also found in Joseph E. Brown, “The ‘Scientia de ponderibus’ in the Later Middle Ages,” PhD. Dissertation, University of Wisconsin, 1967. The Liber de ponderibus and the “Aliud commentum” version were published by Petrus Apianus
(= Peter Bienewitz) in Nuremberg, 1533; and the De ratione ponderis was published by Nicolò Tartaglia
in Venice, 1565.
2. The Algorismi treatises: The articles by Gustaf Eneström, which contain the Latin text of the introductions, definitions and propositions, but only some of the proofs, were published in Biblioteca Mathematica, ser 3, vol. 7 (1906–07), 24-37; 8 (1907–08), 135-153; 13 (1912–13), 289-332; 14 (1913–14) 41-54 and 99-149.
3. Arithmetic (the De elementis arithmetice artis): Jacques Lefèvre d’Étaples (1455–1536) published a version (with his own demonstrations and comments) in Paris in 1496; this was reprinted Paris, 1514. The modern edition is: H. L. L. Busard, Jordanus de Nemore, De elementis arithmetice artis. A Medieval Treatise on Number Theory (Stuttgart: Franz Steiner Verlag, 1991), 2 parts.
4. Algebra (De numeris data): The text was published in the 19th century, but a critical edition now exists: Jordanus de Nemore, De numeris datis, ed. Barnabas B. Hughes (Berkeley: University of California Press, 1981).
5. Geometry: "De triangulis" was first published by M.Curtze in "Mittheilungen des Copernicusvereins für Wissenschaft und Kunst" Heft VI - Thorn, 1887. See in Kujawsko-Pomorska Digital Library: http://kpbc.umk.pl/dlibra/docmetadata?id=39881. More recently, the Liber philotegni Iordani and the Liber de triangulis Iordani have been critically edited and translated in: Marshall Clagett, Archimedes in the Middle Ages (Philadelphia: American Philosophical Society, 1984), 5: 196-293 and 346-477, which is much improved over Curtze's edition.
6. Stereographic projection: The text of version 3 of the Demonstratio de plana spera and the introduction were published in the sixteenth century – Basel, 1536 and Venice, 1558. All versions are edited and translated in: Ron B. Thomson, Jordanus de Nemore and the Mathematics of Astrolabes: De Plana Spera (Toronto: Pontifical Institute of Mediaeval Studies, 1978).
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
who wrote treatises on at least 6 different important mathematical subjects: the science of weights; “algorismi” treatises on practical arithmetic; pure arithmetic; algebra; geometry; and stereographic projection. Most of these treatises exist in several versions or reworkings from the Middle Ages. We know nothing about him personally, other than the approximate date of his work.
Life
No biographical details are known about Jordanus de Nemore. Cited in the early manuscripts simply as “Jordanus”, he was later given the sobriquet of “de Nemore” (“of the Forest,” “Forester”) which does not add any firm biographical information. In the RenaissanceRenaissance
The Renaissance was a cultural movement that spanned roughly the 14th to the 17th century, beginning in Italy in the Late Middle Ages and later spreading to the rest of Europe. The term is also used more loosely to refer to the historical era, but since the changes of the Renaissance were not...
his name was often given as "Jordanus Nemorarius", an improper form. We know nothing of his nationality.
An entry in the nineteenth-century manuscript catalogue for the Sächsische Landesbibliothek in Dresden
Dresden
Dresden is the capital city of the Free State of Saxony in Germany. It is situated in a valley on the River Elbe, near the Czech border. The Dresden conurbation is part of the Saxon Triangle metropolitan area....
suggested that Jordanus taught at the University of Toulouse
University of Toulouse
The Université de Toulouse is a consortium of French universities, grandes écoles and other institutions of higher education and research, named after one of the earliest universities established in Europe in 1229, and including the successor universities to that earlier university...
, but the text in question was not written by Jordanus and this possible association is without foundation. A fourteenth-century chronicle of the Order of Preachers by the Englishman Nicholas Trivet
Nicholas Trivet
Nicholas Trivet was an English Anglo-Norman chronicler.Trivet was born in Somerset and was the son of Sir Thomas Trevet , a judge who came of a Norfolk or Somerset family...
(or Triveth, 1258–1328) suggested that the second master-general of the Dominican Order
Dominican Order
The Order of Preachers , after the 15th century more commonly known as the Dominican Order or Dominicans, is a Catholic religious order founded by Saint Dominic and approved by Pope Honorius III on 22 December 1216 in France...
, Jordanus of Saxony (d. 1237) wrote two mathematical texts with titles similar to two by Jordanus de Nemore, but this late suggestion is more likely a confusion on the part of Trivet, rather than any proof of identity. Jordanus of Saxony never uses the name “de Nemore” and is nowhere else credited with mathematical writings – in fact he had lectured in theology at the University of Paris
University of Paris
The University of Paris was a university located in Paris, France and one of the earliest to be established in Europe. It was founded in the mid 12th century, and officially recognized as a university probably between 1160 and 1250...
. Likewise the name of Jordanus of Saxony is never found with a mathematical text. This identity, popular among some in the nineteenth and twentieth centuries, has been for the most part abandoned.
It is assumed that Jordanus did work in the first part of the thirteenth century (or even in the late twelfth) since his works are contained in a booklist, the Biblionomia of Richard de Fournival
Richard de Fournival
Richard de Fournival or Richart de Fornival was a medieval philosopher and trouvère perhaps best known for the Bestiaire d'amour .-Life:...
, compiled between 1246 and 1260.
Mechanics: scientia de ponderibus (the science of weights)
The medieval “science of weights” (i.e., mechanicsMechanics
Mechanics is the branch of physics concerned with the behavior of physical bodies when subjected to forces or displacements, and the subsequent effects of the bodies on their environment....
) owes much of its importance to the work of Jordanus. In the Elementa super demonstrationem ponderum, he introduces the concept of “positional gravity” and the use of component forces. Pierre Duhem
Pierre Duhem
Pierre Maurice Marie Duhem was a French physicist, mathematician and philosopher of science, best known for his writings on the indeterminacy of experimental criteria and on scientific development in the Middle Ages...
(in his Origines de la statique, 1905) thought that Jordanus also introduces infinitesimal considerations into statics
Statics
Statics is the branch of mechanics concerned with the analysis of loads on physical systems in static equilibrium, that is, in a state where the relative positions of subsystems do not vary over time, or where components and structures are at a constant velocity...
in his discussion of "virtual" displacements (this being another interpretation of Duhem) of objects in equilibrium. He proves the law of the lever by means of the principle of work. The De ratione ponderis also proves the conditions of equilibrium of unequal weights on planes inclined at different angles – long before Galileo
Galileo Galilei
Galileo Galilei , was an Italian physicist, mathematician, astronomer, and philosopher who played a major role in the Scientific Revolution. His achievements include improvements to the telescope and consequent astronomical observations and support for Copernicanism...
.
The Elementa super demonstrationem ponderum seems to be the one work which can definitely be ascribed to Jordanus; and the first of the series. Jordanus took what Joseph Brown has called the "Logician’s Abstract of On the Karaston" (a skillful compression of the conclusions of Thābit ibn Qurra
Thabit ibn Qurra
' was a mathematician, physician, astronomer and translator of the Islamic Golden Age.Ibn Qurra made important discoveries in algebra, geometry and astronomy...
’s Liber karastonis) and created a new treatise (7 axioms and 9 propositions) in order to establish a mathematical basis for the four propositions on the Roman
Ancient Rome
Ancient Rome was a thriving civilization that grew on the Italian Peninsula as early as the 8th century BC. Located along the Mediterranean Sea and centered on the city of Rome, it expanded to one of the largest empires in the ancient world....
balance called the Liber de canonio. An early commentary on this (which also contains a necessary correction to Proposition 9) is the “Corpus Christi Commentary”.
The Liber de ponderibus fuses the seven axioms and nine propositions of the Elementa to the four propositions of the De canonio. There are at least two commentary traditions to the Liber de ponderibus which improve some of the demonstrations and better integrate the two sources.
The De ratione ponderis is a skillfully corrected and expanded version (45 propositions) of the Elementa. This is usually ascribed to Jordanus, but more likely it is the work of an unidentified mathematician because the citations by Jordanus of his other works are deleted.
Related to these treatises is an anonymous set of comments, each of which begins with the words “Aliud commentum” (and thus known as the “Aliud commentum” version). This commentary surpasses all others, especially the commentary on Proposition 1.
Algorismi treatises
There are 5 algorismAlgorism
Algorism is the technique of performing basic arithmetic by writing numbers in place value form and applying a set of memorized rules and facts to the digits. One who practices algorism is known as an algorist...
i treatises in this category, examined by Gustaf Eneström
Gustaf Eneström
Gustaf Hjalmar Eneström was a Swedish mathematician, statistician and historian of mathematics known for introducing the Eneström index, which was used to identify Euler's writings...
early in the twentieth century, dealing with practical arithmetic
Arithmetic
Arithmetic or arithmetics is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple day-to-day counting to advanced science and business calculations. It involves the study of quantity, especially as the result of combining numbers...
.
The Communis et consuetus (its opening words) appears to be the earliest form of the work, closely related to the much expanded Demonstratio de algorismo. Eneström believed that the Communis et consuetus was certainly by Jordanus.
The later Demonstratio de algorismo contains 21 definitions and 34 propositions. This is probably a later version of the Communis et consuetus, made either by Jordanus himself or by some other thirteenth-century mathematician.
The Tractatus minutiarum on fractions seems to be a second part of the Communis et consuetus – they are often found together in the manuscripts.
The Demonstratio de minutiius likewise is linked to the Demonstratio de algorismo, and contains and expands the propositions found in the Tractatus minutiarum – again a re-edition of the original text.
The Algorismus demonstratus is a spurious attribution although for a long time this item was ascribed to Jordanus. Up until Eneström began to sort out the various treatises, the Algorismus demonstratus – since it was the only one published (ed. Johannes Schöner
Johannes Schöner
Johannes Schöner was a renowned and respected German polymath...
, Nuremberg, 1543) – was the heading under which all the treatises were grouped. Eneström thought it highly unlikely, however, that this version was the work of Jordanus since no manuscript ascribes it to him (if they give an author, it is generally a Magister Gernarus, or Gerhardus or Gernandus). The first part of this treatise (also known as the Algorismus de integris) contains definitions, axioms and 43 propositions. The second part (the Algorismus de minutiis) contains definitions and 42 propositions. Eneström shows that while different from the algorismi treatises of Jordanus, the Algorismus demonstratus is still closely related to them.
Arithmetic: The De elementis arismetice artis
This treatise on arithmeticArithmetic
Arithmetic or arithmetics is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple day-to-day counting to advanced science and business calculations. It involves the study of quantity, especially as the result of combining numbers...
contains over 400 propositions divided into ten books. There are three versions or editions in manuscript form, the second one with different or expanded proofs than found in the first, and a number of propositions added at the end; the third version inserts the added propositions into their logical position in the text, and again changed some of the proofs. Jordanus’ aim was to write a complete summary of arithmetic, similar to what Euclid
Euclid
Euclid , fl. 300 BC, also known as Euclid of Alexandria, was a Greek mathematician, often referred to as the "Father of Geometry". He was active in Alexandria during the reign of Ptolemy I...
had done for geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
.
Jordanus collected and organized the whole field of arithmetic, based both on Euclid’s work and on that of Boethius
Anicius Manlius Severinus Boethius
Anicius Manlius Severinus Boëthius, commonly called Boethius was a philosopher of the early 6th century. He was born in Rome to an ancient and important family which included emperors Petronius Maximus and Olybrius and many consuls. His father, Flavius Manlius Boethius, was consul in 487 after...
. Definitions, axioms and postulates lead to propositions with proofs which are somewhat sketchy at times, leaving the reader to complete the argument. Here also Jordanus uses letters to represent numbers, but numerical examples, of the type found in the De numeris datis, are not given.
Algebra: The De numeris datis
The editor of this treatise on algebraAlgebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
, Barnabas Hughes, has found two sets of manuscripts for this text, one containing 95 propositions, the other, 113. As well some of the common propositions have different proofs. There are also 4 digests or revisions in manuscript form.
Jordanus’ De numeris datis was the first treatise in advanced algebra composed in Western Europe, building on elementary algebra provided in twelfth-century translations from Arabic sources. It anticipates by 350 years the introduction of algebraic analysis by François Viète
François Viète
François Viète , Seigneur de la Bigotière, was a French mathematician whose work on new algebra was an important step towards modern algebra, due to its innovative use of letters as parameters in equations...
into Renaissance
Renaissance
The Renaissance was a cultural movement that spanned roughly the 14th to the 17th century, beginning in Italy in the Late Middle Ages and later spreading to the rest of Europe. The term is also used more loosely to refer to the historical era, but since the changes of the Renaissance were not...
mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
. Jordanus used a system similar to that of Viète (although couched on non-symbolic terms) of formulating the equation (setting out the problem in terms of what is known and of what is to be found), of transforming the initial given equation into a solution, and the introduction of specific numbers that fulfil the conditions set by the problem.
Geometry: Liber philotegni and the De triangulis
This is medieval geometryGeometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
at its best. It contains propositions on such topics as the ratios of sides and angles of triangles; the division of straight lines, triangles, and quadrangles under different conditions; the ratio of arcs and plane segments in the same or in different circles; trisecting an angle; the area of triangles given the length of the sides; squaring the circle.
Again there are two versions of this text: the shorter and presumably first edition (the Liber philotegni Iordani de Nemore) and a longer version (Liber de triangulis Iordani) which divides the text into books, re-arranges and expands book 2, and adds propositions 4-12 to 4-28. This latter set of 17 propositions also circulated separately. While the longer version may not be by Jordanus, it was certainly complete by the end of the thirteenth century.
Stereographic projection: Demonstratio de plana spera
Stereographic projection
The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it...
(used in planispheric astrolabes). The first and historically the most important proposition proves for all cases that circles on the surface of a sphere when projected stereographically on a plane remain circles (or a circle of infinite radius, i.e., a straight line). While this property was known long before Jordanus, it had never been proved.
There are three versions of the treatise: the basic text, a second version with an introduction and a much expanded text, and a third, only slightly expanded. The introduction is sometimes found with version 1 and 3, but it was obviously written by someone else.
Dubious and spurious works
The De proportionibus (on ratios), the Isoperimetra (on figures with equal perimeters), the Demonstrationes pro astrolapsu (on astrolabeAstrolabe
An astrolabe is an elaborate inclinometer, historically used by astronomers, navigators, and astrologers. Its many uses include locating and predicting the positions of the Sun, Moon, planets, and stars, determining local time given local latitude and longitude, surveying, triangulation, and to...
engraving), and the Pre-exercitamina (“a short introductory exercise”?) are dubiously ascribed to Jordanus. A number of other texts including a Liber de speculis and a Compositum astrolabii are spurious ascriptions.
Editions of Jordanus’ works
Most of Jordanus' works have been published in critical editions in the twentieth century.1. Mechanics: The three main treatises and the “Aliud commentum” version (Latin and English) are published in The Medieval Science of Weights, ed. Ernest A. Moody and Marshall Clagett (Madison: University of Wisconsin Press, 1952). The commentaries are also found in Joseph E. Brown, “The ‘Scientia de ponderibus’ in the Later Middle Ages,” PhD. Dissertation, University of Wisconsin, 1967. The Liber de ponderibus and the “Aliud commentum” version were published by Petrus Apianus
Petrus Apianus
Petrus Apianus , also known as Peter Apian, was a German humanist, known for his works in mathematics, astronomy and cartography.The lunar crater Apianus and minor planet 19139 Apian are named in his honour....
(= Peter Bienewitz) in Nuremberg, 1533; and the De ratione ponderis was published by Nicolò Tartaglia
Niccolò Fontana Tartaglia
Niccolò Fontana Tartaglia was a mathematician, an engineer , a surveyor and a bookkeeper from the then-Republic of Venice...
in Venice, 1565.
2. The Algorismi treatises: The articles by Gustaf Eneström, which contain the Latin text of the introductions, definitions and propositions, but only some of the proofs, were published in Biblioteca Mathematica, ser 3, vol. 7 (1906–07), 24-37; 8 (1907–08), 135-153; 13 (1912–13), 289-332; 14 (1913–14) 41-54 and 99-149.
3. Arithmetic (the De elementis arithmetice artis): Jacques Lefèvre d’Étaples (1455–1536) published a version (with his own demonstrations and comments) in Paris in 1496; this was reprinted Paris, 1514. The modern edition is: H. L. L. Busard, Jordanus de Nemore, De elementis arithmetice artis. A Medieval Treatise on Number Theory (Stuttgart: Franz Steiner Verlag, 1991), 2 parts.
4. Algebra (De numeris data): The text was published in the 19th century, but a critical edition now exists: Jordanus de Nemore, De numeris datis, ed. Barnabas B. Hughes (Berkeley: University of California Press, 1981).
5. Geometry: "De triangulis" was first published by M.Curtze in "Mittheilungen des Copernicusvereins für Wissenschaft und Kunst" Heft VI - Thorn, 1887. See in Kujawsko-Pomorska Digital Library: http://kpbc.umk.pl/dlibra/docmetadata?id=39881. More recently, the Liber philotegni Iordani and the Liber de triangulis Iordani have been critically edited and translated in: Marshall Clagett, Archimedes in the Middle Ages (Philadelphia: American Philosophical Society, 1984), 5: 196-293 and 346-477, which is much improved over Curtze's edition.
6. Stereographic projection: The text of version 3 of the Demonstratio de plana spera and the introduction were published in the sixteenth century – Basel, 1536 and Venice, 1558. All versions are edited and translated in: Ron B. Thomson, Jordanus de Nemore and the Mathematics of Astrolabes: De Plana Spera (Toronto: Pontifical Institute of Mediaeval Studies, 1978).