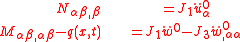Kirchhoff–Love plate theory
Encyclopedia
The Kirchhoff–Love theory of plates is a two-dimensional mathematical model
that is used to determine the stresses and deformation
s in thin plate
s subjected to force
s and moment
s. This theory is an extension of Euler-Bernoulli beam theory and was developed in 1888 by Love
using assumptions proposed by Kirchhoff
. The theory assumes that a mid-surface plane can be used to represent a three-dimensional plate in two-dimensional form.
The following kinematic assumptions that are made in this theory:
 . Then
. Then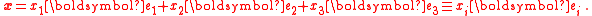
The vectors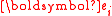 form a Cartesian
form a Cartesian
basis
with origin on the mid-surface of the plate,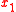 and
and 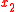 are the Cartesian coordinates on the mid-surface of the undeformed plate, and
are the Cartesian coordinates on the mid-surface of the undeformed plate, and 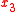 is the coordinate for the thickness direction.
is the coordinate for the thickness direction.
Let the displacement
of a point in the plate be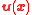 . Then
. Then
This displacement can be decomposed into a vector sum of the mid-surface displacement and an out-of-plane displacement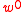 in the
in the 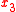 direction. We can write the in-plane displacement of the mid-surface as
direction. We can write the in-plane displacement of the mid-surface as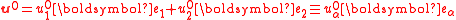
Note that the index takes the values 1 and 2 but not 3.
takes the values 1 and 2 but not 3.
Then the Kirchhoff hypothesis implies that
If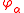 are the angles of rotation of the normal
are the angles of rotation of the normal
to the mid-surface, then in the Kirchhoff-Love theory
Note that we can think of the expression for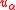 as the first order Taylor series
as the first order Taylor series
expansion of the displacement around the mid-surface.
to situations where moderate rotations could be expected.
 the strains-displacement relations are
the strains-displacement relations are
Using the kinematic assumptions we have
Therefore the only non-zero strains are in the in-plane directions.
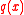 these equations are
these equations are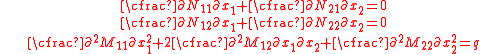
where the thickness of the plate is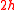 . In index notation,
. In index notation,
where are the stress
are the stress
es.
Note that the quantity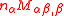 is an effective shear force.
is an effective shear force.
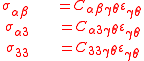
Since and
and 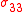 do not appear in the equilibrium equations it is implicitly assumed that these quantities do not have any effect on the momentum balance and are neglected. The remaining stress-strain relations, in matrix form, can be written as
do not appear in the equilibrium equations it is implicitly assumed that these quantities do not have any effect on the momentum balance and are neglected. The remaining stress-strain relations, in matrix form, can be written as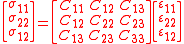
Then,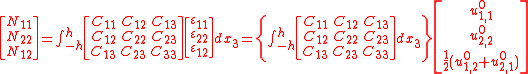
and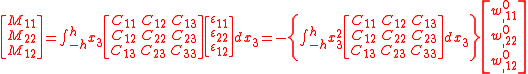
The extensional stiffnesses are the quantities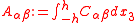
The bending stiffnesses (also called flexural rigidity) are the quantities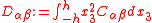
The Kirchhoff-Love constitutive assumptions lead to zero shear forces. As a result, the equilibrium equations for the plate have to be used to determine the shear forces in thin Kirchhoff-Love plates. For isotropic plates, these equations lead to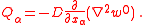
Alternatively, these shear forces can be expressed as
where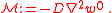
 to 15
to 15 , the strain-displacement relations can be approximated as
, the strain-displacement relations can be approximated as
Then the kinematic assumptions of Kirchhoff-Love theory lead to the classical plate theory with von Kármán
strains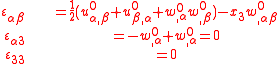
This theory is nonlinear because of the quadratic terms in the strain-displacement relations.
If the strain-displacement relations take the von Karman form, the equilibrium equations can be expressed as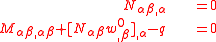
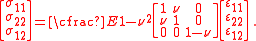
The moments corresponding to these stresses are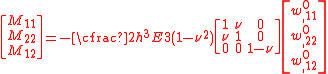
In expanded form,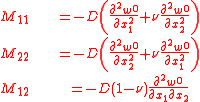
where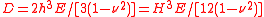 for plates of thickness
for plates of thickness  . Using the stress-strain relations for the plates, we can show that the stresses and moments are related by
. Using the stress-strain relations for the plates, we can show that the stresses and moments are related by
At the top of the plate where , the stresses are
, the stresses are
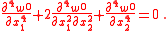
Here we have assumed that the in-plane displacements are do not vary with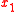 and
and 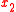 . In index notation,
. In index notation,
and in direct notation
The bending moments are given by
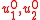 is applied to the plate, the governing equation is
is applied to the plate, the governing equation is  . Following the procedure shown in the previous section we get
. Following the procedure shown in the previous section we get
In rectangular Cartesian coordinates, the governing equation is
and in cylindrical coordinates it takes the form
Solutions of this equation for various geometries and boundary conditions can be found in the article on bending of plates
.
 . In that case
. In that case
and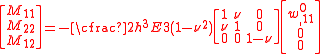
and the governing equations become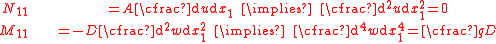
where, for a plate with density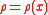 ,
,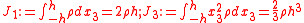
and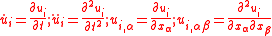
Solutions of these equations for some special cases can be found in the article on vibrations of plates
. The figures below show some vibrational modes of a circular plate.
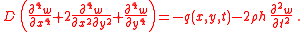
where is the bending stiffness of the plate. For a uniform plate of thickness
is the bending stiffness of the plate. For a uniform plate of thickness 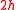 ,
,
In direct notation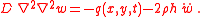
For free vibrations, the governing equation becomes
Mathematical model
A mathematical model is a description of a system using mathematical concepts and language. The process of developing a mathematical model is termed mathematical modeling. Mathematical models are used not only in the natural sciences and engineering disciplines A mathematical model is a...
that is used to determine the stresses and deformation
Deformation (mechanics)
Deformation in continuum mechanics is the transformation of a body from a reference configuration to a current configuration. A configuration is a set containing the positions of all particles of the body...
s in thin plate
Plate
Plate may refer to:* Plate , a broad, mainly flat vessel on which food is served* Silver or the plate, dishware and cutlery made of sterling, Britannia or Sheffield plate silver* Plating, the deposition of metallic layers...
s subjected to force
Force
In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...
s and moment
Moment
- Science, engineering, and mathematics :* Moment , used in probability theory and statistics* Moment , several related concepts, including:** Angular momentum or moment of momentum, the rotational analog of momentum...
s. This theory is an extension of Euler-Bernoulli beam theory and was developed in 1888 by Love
Augustus Edward Hough Love
Augustus Edward Hough Love FRS , often known as A. E. H. Love, was a mathematician famous for his work on the mathematical theory of elasticity...
using assumptions proposed by Kirchhoff
Gustav Kirchhoff
Gustav Robert Kirchhoff was a German physicist who contributed to the fundamental understanding of electrical circuits, spectroscopy, and the emission of black-body radiation by heated objects...
. The theory assumes that a mid-surface plane can be used to represent a three-dimensional plate in two-dimensional form.
The following kinematic assumptions that are made in this theory:
- straight lines normal to the mid-surface remain straight after deformation
- straight lines normal to the mid-surface remain normal to the mid-surface after deformation
- the thickness of the plate does not change during a deformation.
Assumed displacement field
Let the position vector of a point in the undeformed plate be . Then
. Then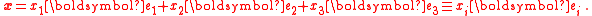
The vectors
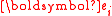 form a Cartesian
form a CartesianCartesian coordinate system
A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
basis
Basis (linear algebra)
In linear algebra, a basis is a set of linearly independent vectors that, in a linear combination, can represent every vector in a given vector space or free module, or, more simply put, which define a "coordinate system"...
with origin on the mid-surface of the plate,
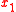 and
and 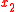 are the Cartesian coordinates on the mid-surface of the undeformed plate, and
are the Cartesian coordinates on the mid-surface of the undeformed plate, and 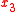 is the coordinate for the thickness direction.
is the coordinate for the thickness direction.Let the displacement
Displacement (vector)
A displacement is the shortest distance from the initial to the final position of a point P. Thus, it is the length of an imaginary straight path, typically distinct from the path actually travelled by P...
of a point in the plate be
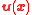 . Then
. Then
This displacement can be decomposed into a vector sum of the mid-surface displacement and an out-of-plane displacement
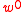 in the
in the 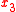 direction. We can write the in-plane displacement of the mid-surface as
direction. We can write the in-plane displacement of the mid-surface as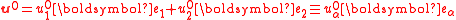
Note that the index
 takes the values 1 and 2 but not 3.
takes the values 1 and 2 but not 3.Then the Kirchhoff hypothesis implies that
If
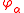 are the angles of rotation of the normal
are the angles of rotation of the normalNormal
Normal may refer to:* Normality , conformance to an average* Norm , social norms, expected patterns of behavior studied within the context of sociology* Normal distribution , the Gaussian continuous probability distribution...
to the mid-surface, then in the Kirchhoff-Love theory

Note that we can think of the expression for
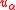 as the first order Taylor series
as the first order Taylor seriesTaylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
expansion of the displacement around the mid-surface.
Quasistatic Kirchhoff-Love plates
The original theory developed by Love was valid for infinitesimal strains and rotations. The theory was extended by von KármánTheodore von Karman
Theodore von Kármán was a Hungarian-American mathematician, aerospace engineer and physicist who was active primarily in the fields of aeronautics and astronautics. He is responsible for many key advances in aerodynamics, notably his work on supersonic and hypersonic airflow characterization...
to situations where moderate rotations could be expected.
Strain-displacement relations
For the situation where the strains in the plate are infinitesimal and the rotations of the mid-surface normals are less than 10 the strains-displacement relations are
the strains-displacement relations are
Using the kinematic assumptions we have
Therefore the only non-zero strains are in the in-plane directions.
Equilibrium equations
The equilibrium equations for the plate can be derived from the principle of virtual work. For a thin plate under a quasistatic transverse load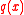 these equations are
these equations are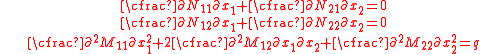
where the thickness of the plate is
 . In index notation,
. In index notation,where
 are the stress
are the stressStress (physics)
In continuum mechanics, stress is a measure of the internal forces acting within a deformable body. Quantitatively, it is a measure of the average force per unit area of a surface within the body on which internal forces act. These internal forces are a reaction to external forces applied on the body...
es.
| Derivation of equilibrium equations for small rotations |
|---|
For the situation where the strains and rotations of the plate are small the virtual internal energy is given by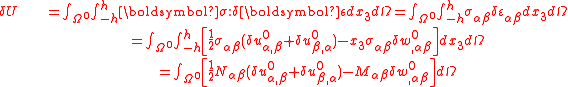 where the thickness of the plate is  and the stress resultants and stress moment resultants are defined as and the stress resultants and stress moment resultants are defined as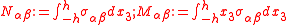 Integration by parts leads to 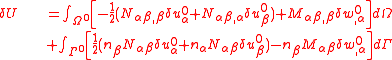 The symmetry of the stress tensor implies that  . Hence, . Hence,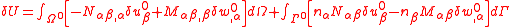 Another integration by parts gives 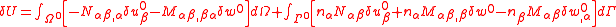 For the case where there are no prescribed external forces, the principle of virtual work implies that 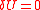 . The equilibrium equations for the plate are then given by . The equilibrium equations for the plate are then given by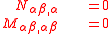 If the plate is loaded by an external distributed load 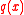 that is normal to the mid-surface and directed in the positive that is normal to the mid-surface and directed in the positive 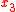 direction, the external virtual work due to the load is direction, the external virtual work due to the load is The principle of virtual work then leads to the equilibrium equations 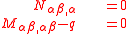 |
Boundary conditions
The boundary conditions that are needed to solve the equilibrium equations of plate theory can be obtained from the boundary terms in the principle of virtual work. In the absence of external forces on the boundary, the boundary conditions are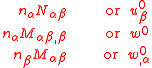
Note that the quantity
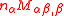 is an effective shear force.
is an effective shear force.Constitutive relations
The stress-strain relations for a linear elastic Kirchhoff plate are given by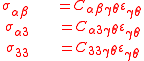
Since
 and
and 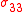 do not appear in the equilibrium equations it is implicitly assumed that these quantities do not have any effect on the momentum balance and are neglected. The remaining stress-strain relations, in matrix form, can be written as
do not appear in the equilibrium equations it is implicitly assumed that these quantities do not have any effect on the momentum balance and are neglected. The remaining stress-strain relations, in matrix form, can be written as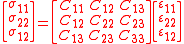
Then,
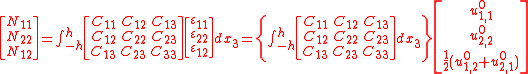
and
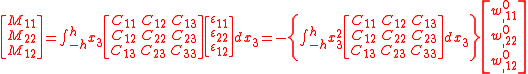
The extensional stiffnesses are the quantities
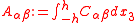
The bending stiffnesses (also called flexural rigidity) are the quantities
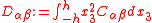
The Kirchhoff-Love constitutive assumptions lead to zero shear forces. As a result, the equilibrium equations for the plate have to be used to determine the shear forces in thin Kirchhoff-Love plates. For isotropic plates, these equations lead to
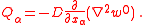
Alternatively, these shear forces can be expressed as

where
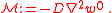
Small strains and moderate rotations
If the rotations of the normals to the mid-surface are in the range of 10 to 15
to 15 , the strain-displacement relations can be approximated as
, the strain-displacement relations can be approximated as
Then the kinematic assumptions of Kirchhoff-Love theory lead to the classical plate theory with von Kármán
Theodore von Karman
Theodore von Kármán was a Hungarian-American mathematician, aerospace engineer and physicist who was active primarily in the fields of aeronautics and astronautics. He is responsible for many key advances in aerodynamics, notably his work on supersonic and hypersonic airflow characterization...
strains
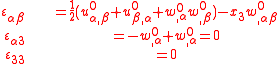
This theory is nonlinear because of the quadratic terms in the strain-displacement relations.
If the strain-displacement relations take the von Karman form, the equilibrium equations can be expressed as
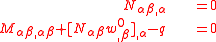
Isotropic quasistatic Kirchhoff-Love plates
For an isotropic and homogeneous plate, the stress-strain relations are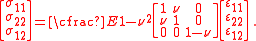
The moments corresponding to these stresses are
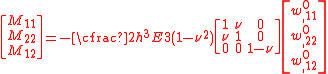
In expanded form,
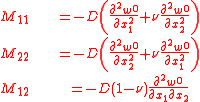
where
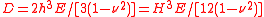 for plates of thickness
for plates of thickness  . Using the stress-strain relations for the plates, we can show that the stresses and moments are related by
. Using the stress-strain relations for the plates, we can show that the stresses and moments are related by
At the top of the plate where
 , the stresses are
, the stresses are
Pure bending
For an isotropic and homogeneous plate under pure bending, the governing equations reduce to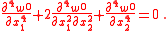
Here we have assumed that the in-plane displacements are do not vary with
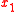 and
and 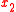 . In index notation,
. In index notation,
and in direct notation
The bending moments are given by

| Derivation of equilibrium equations for pure bending |
|---|
For an isotropic, homogeneous plate under pure bending the governing equations are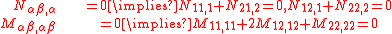 and the stress-strain relations are 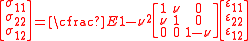 Then, 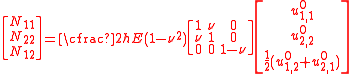 and 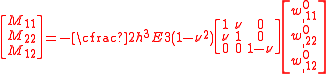 Differentiation gives  and  Plugging into the governing equations leads to 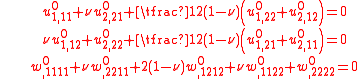 Since the order of differentiation is irrelevant we have 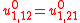 , ,  , and , and 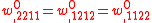 . Hence . Hence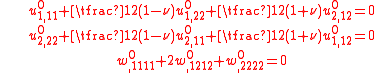 In direct tensor notation, the governing equation of the plate is  where we have assumed that the displacements  are constant. are constant. |
Bending under transverse load
If a distributed transverse load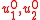 is applied to the plate, the governing equation is
is applied to the plate, the governing equation is  . Following the procedure shown in the previous section we get
. Following the procedure shown in the previous section we getIn rectangular Cartesian coordinates, the governing equation is

and in cylindrical coordinates it takes the form

Solutions of this equation for various geometries and boundary conditions can be found in the article on bending of plates
Bending of plates
Bending of plates or plate bending refers to the deflection of a plate perpendicular to the plane of the plate under the action of external forces and moments. The amount of deflection can be determined by solving the differential equations of an appropriate plate theory. The stresses in the...
.
| Derivation of equilibrium equations for transverse loading |
|---|
or a transversely loaded plate without axial deformations, the governing equation has the form where 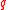 is a distributed transverse load (per unit area). Substitution of the expressions for the derivatives of is a distributed transverse load (per unit area). Substitution of the expressions for the derivatives of 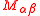 into the governing equation gives into the governing equation gives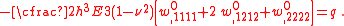 Noting that the bending stiffness is the quantity  we can write the governing equation in the form In cylindrical coordinates 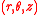 , ,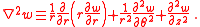 For symmetrically loaded circular plates,  , and we have , and we have |
Cylindrical bending
Under certain loading conditions a flat plate can be bent into the shape of the surface of a cylinder. This type of bending is called cylindrical bending and represents the special situation where . In that case
. In that case
and
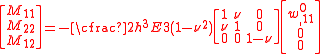
and the governing equations become
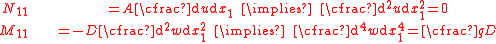
Dynamics of Kirchhoff-Love plates
The dynamic theory of thin plates determines the propagation of waves in the plates, and the study of standing waves and vibration modes.Governing equations
The governing equations for the dynamics of a Kirchhoff-Love plate arewhere, for a plate with density
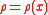 ,
,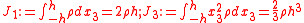
and
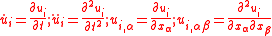
| Derivation of equations governing the dynamics of Kirchhoff-Love plates |
|---|
| The total kinetic energy of the plate is given by |
Solutions of these equations for some special cases can be found in the article on vibrations of plates
Vibration of plates
The vibration of plates is a special case of the more general problem of mechanical vibrations. The equations governing the motion of plates are simpler than those for general three-dimensional objects because one of the dimensions of a plate is much smaller than the other two...
. The figures below show some vibrational modes of a circular plate.
Isotropic plates
The governing equations simplify considerably for isotropic and homogeneous plates for which the in-plane deformations can be neglected. In that case we are left with one equation of the following form (in rectangular Cartesian coordinates):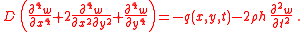
where
 is the bending stiffness of the plate. For a uniform plate of thickness
is the bending stiffness of the plate. For a uniform plate of thickness 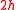 ,
,
In direct notation
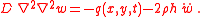
For free vibrations, the governing equation becomes

| Derivation of dynamic governing equations for isotropic Kirchhoff-Love plates |
|---|
For an isotropic and homogeneous plate, the stress-strain relations are 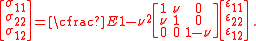 where 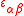 are the in-plane strains. The strain-displacement relations are the in-plane strains. The strain-displacement relationsfor Kirchhoff-Love plates are 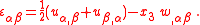 Therefore, the resultant moments corresponding to these stresses are 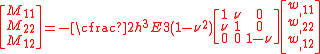 The governing equation for an isotropic and homogeneous plate of uniform thickness  in the in theabsence of in-plane displacements is 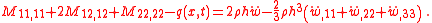 Differentiation of the expressions for the moment resultants gives us 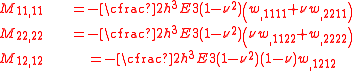 Plugging into the governing equations leads to 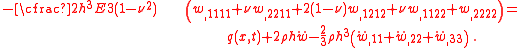 Since the order of differentiation is irrelevant we have 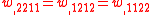 . Hence . Hence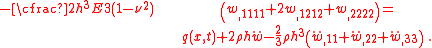 If the flexural stiffness of the plate is defined as  we have  For small deformations, we often neglect the spatial derivatives of the transverse acceleration of the plate and we are left with 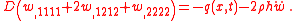 Then, in direct tensor notation, the governing equation of the plate is 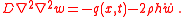 |
See also
- BendingBendingIn engineering mechanics, bending characterizes the behavior of a slender structural element subjected to an external load applied perpendicularly to a longitudinal axis of the element. The structural element is assumed to be such that at least one of its dimensions is a small fraction, typically...
- Bending of platesBending of platesBending of plates or plate bending refers to the deflection of a plate perpendicular to the plane of the plate under the action of external forces and moments. The amount of deflection can be determined by solving the differential equations of an appropriate plate theory. The stresses in the...
- Infinitesimal strain theory
- Linear elasticityLinear elasticityLinear elasticity is the mathematical study of how solid objects deform and become internally stressed due to prescribed loading conditions. Linear elasticity models materials as continua. Linear elasticity is a simplification of the more general nonlinear theory of elasticity and is a branch of...
- Plate theoryPlate theoryIn continuum mechanics, plate theories are mathematical descriptions of the mechanics of flat plates that draws on the theory of beams. Plates are defined as plane structural elements with a small thickness compared to the planar dimensions . The typical thickness to width ratio of a plate...
- Stress (mechanics)
- Vibration of platesVibration of platesThe vibration of plates is a special case of the more general problem of mechanical vibrations. The equations governing the motion of plates are simpler than those for general three-dimensional objects because one of the dimensions of a plate is much smaller than the other two...


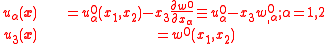

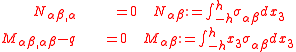
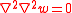

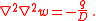
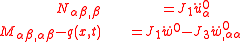
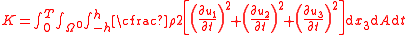
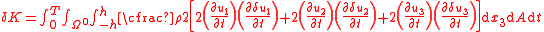
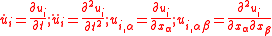
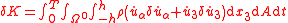
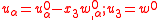
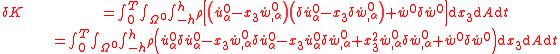
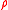 through the thickness of the plate,
through the thickness of the plate,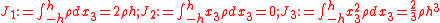
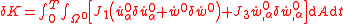
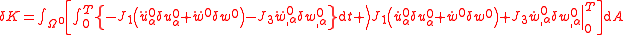
 and
and  are zero at
are zero at  and
and  .
.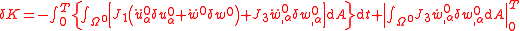
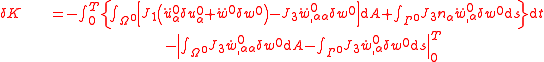


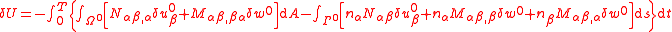
 acting normal to the surface of the plate, the virtual external work done is
acting normal to the surface of the plate, the virtual external work done is
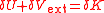 . Hence the governing balance equations for the plate are
. Hence the governing balance equations for the plate are