List of spherical symmetry groups
Encyclopedia
Spherical symmetry groups are also called point groups in three dimensions
, however this article is limitied to the finite symmetries.
There are five fundamental symmetry classes which have triangular fundamental domains: dihedral, cyclic, tetrahedral
, octahedral
, and icosahedral
symmetry.
This article lists the groups by Schoenflies notation
, Coxeter notation
, orbifold notation, and order. John Conway
uses a variation of the Schoenflies notation, named by one or two upper case letters, and whole number subscripts. The group order is defined as the subscript, unless the order is doubled for symbols with a plus or minus, "±", prefix.
Hermann–Mauguin notation (International notation) is also given. The crystallography
groups, 32 in total, are a subset with element orders 2, 3, 4 and 6.
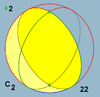
, 2-fold rotational symmetry, and central point symmetry.
families, with n=2 or higher. (n may be 1 as a special case)
families, with n as 2 or higher. (n may be 1 as a special case)
, octahedral symmetry
, and icosahedral symmetry
, named after the triangle-faced regular polyhedra with these symmetries.
Point groups in three dimensions
In geometry, a point group in three dimensions is an isometry group in three dimensions that leaves the origin fixed, or correspondingly, an isometry group of a sphere. It is a subgroup of the orthogonal group O, the group of all isometries that leave the origin fixed, or correspondingly, the group...
, however this article is limitied to the finite symmetries.
There are five fundamental symmetry classes which have triangular fundamental domains: dihedral, cyclic, tetrahedral
Tetrahedral symmetry
150px|right|thumb|A regular [[tetrahedron]], an example of a solid with full tetrahedral symmetryA regular tetrahedron has 12 rotational symmetries, and a symmetry order of 24 including transformations that combine a reflection and a rotation.The group of all symmetries is isomorphic to the group...
, octahedral
Octahedral symmetry
150px|thumb|right|The [[cube]] is the most common shape with octahedral symmetryA regular octahedron has 24 rotational symmetries, and a symmetry order of 48 including transformations that combine a reflection and a rotation...
, and icosahedral
Icosahedral symmetry
A regular icosahedron has 60 rotational symmetries, and a symmetry order of 120 including transformations that combine a reflection and a rotation...
symmetry.
This article lists the groups by Schoenflies notation
Schoenflies notation
The Schoenflies notation or Schönflies notation, named after the German mathematician Arthur Moritz Schoenflies, is one of two conventions commonly used to describe Point groups. This notation is used in spectroscopy. The other convention is the Hermann–Mauguin notation, also known as the...
, Coxeter notation
Coxeter notation
In geometry, Coxeter notation is a system of classifying symmetry groups, describing the angles between with fundamental reflections of a Coxeter group. It uses a bracketed notation, with modifiers to indicate certain subgroups. The notation is named after H. S. M...
, orbifold notation, and order. John Conway
John Horton Conway
John Horton Conway is a prolific mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory...
uses a variation of the Schoenflies notation, named by one or two upper case letters, and whole number subscripts. The group order is defined as the subscript, unless the order is doubled for symbols with a plus or minus, "±", prefix.
Hermann–Mauguin notation (International notation) is also given. The crystallography
Crystallography
Crystallography is the experimental science of the arrangement of atoms in solids. The word "crystallography" derives from the Greek words crystallon = cold drop / frozen drop, with its meaning extending to all solids with some degree of transparency, and grapho = write.Before the development of...
groups, 32 in total, are a subset with element orders 2, 3, 4 and 6.
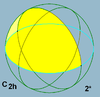
Involutional symmetry
There are four involutional groups: no symmetry, reflection symmetryReflection symmetry
Reflection symmetry, reflectional symmetry, line symmetry, mirror symmetry, mirror-image symmetry, or bilateral symmetry is symmetry with respect to reflection. That is, a figure which does not change upon undergoing a reflection has reflectional symmetry.In 2D there is a line of symmetry, in 3D a...
, 2-fold rotational symmetry, and central point symmetry.
|
|
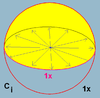
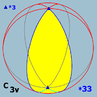
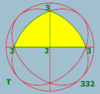
Cyclic symmetry
There are four infinite cyclic symmetryCyclic symmetries
This article deals with the four infinite series of point groups in three dimensions with n-fold rotational symmetry about one axis , and no other rotational symmetry :Chiral:*Cn of order n - n-fold rotational symmetry...
families, with n=2 or higher. (n may be 1 as a special case)
|
|
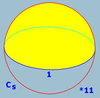
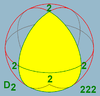
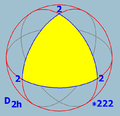
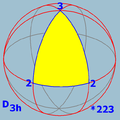
Dihedral symmetry
There are three infinite dihedral symmetryDihedral symmetry in three dimensions
This article deals with three infinite sequences of point groups in three dimensions which have a symmetry group that as abstract group is a dihedral group Dihn .See also point groups in two dimensions.Chiral:...
families, with n as 2 or higher. (n may be 1 as a special case)
|
|
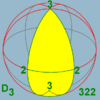
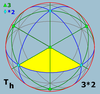
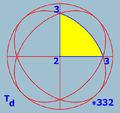
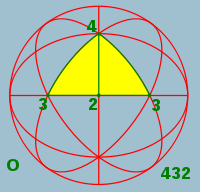
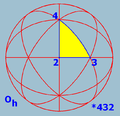
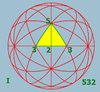
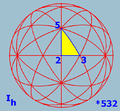
Polyhedral symmetry
There are three types of polyhedral symmetry: tetrahedral symmetryTetrahedral symmetry
150px|right|thumb|A regular [[tetrahedron]], an example of a solid with full tetrahedral symmetryA regular tetrahedron has 12 rotational symmetries, and a symmetry order of 24 including transformations that combine a reflection and a rotation.The group of all symmetries is isomorphic to the group...
, octahedral symmetry
Octahedral symmetry
150px|thumb|right|The [[cube]] is the most common shape with octahedral symmetryA regular octahedron has 24 rotational symmetries, and a symmetry order of 48 including transformations that combine a reflection and a rotation...
, and icosahedral symmetry
Icosahedral symmetry
A regular icosahedron has 60 rotational symmetries, and a symmetry order of 120 including transformations that combine a reflection and a rotation...
, named after the triangle-faced regular polyhedra with these symmetries.
|
|
See also
- Crystallographic point groupCrystallographic point groupIn crystallography, a crystallographic point group is a set of symmetry operations, like rotations or reflections, that leave a central point fixed while moving other directions and faces of the crystal to the positions of features of the same kind...
- Triangle groupTriangle groupIn mathematics, a triangle group is a group that can be realized geometrically by sequences of reflections across the sides of a triangle. The triangle can be an ordinary Euclidean triangle, a triangle on the sphere, or a hyperbolic triangle...
- List of planar symmetry groups
External links
- Finite spherical symmetry groups
- Simplest Canonical Polyhedra of Each Symmetry Type, by David I. McCooey