P-derivation
Encyclopedia
In mathematics
, more specifically differential algebra
, a p-derivation (for p a prime number) on a ring
R, is a mapping from R to R that satisfies certain conditions outlined directly below. The notion of a p-derivation is related to that of a derivation
in differential algebra.
is a map of sets 
that satisfies the following "product rule
":
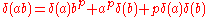
and "sum rule":
.
Note that in the "sum rule" we are not really dividing by p, since all the relevant binomial coefficients in the numerator are divisible by p, so this definition applies in the case when
has p-torsion.
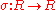is a lift of the Frobenius endomorphism
provided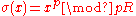
. An example such lift could come from the Artin map.
If
is a ring with a p-derivation, then the map
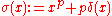defines a ring endomorphism which is a lift of the frobenius endomorphism. When the ring R is p-torsion free the correspondence is a bijection.
The quotient is well-defined because of Fermat's Little Theorem
.
defines a p-derivation.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, more specifically differential algebra
Differential algebra
In mathematics, differential rings, differential fields, and differential algebras are rings, fields, and algebras equipped with a derivation, which is a unary function that is linear and satisfies the Leibniz product law...
, a p-derivation (for p a prime number) on a ring
Ring (mathematics)
In mathematics, a ring is an algebraic structure consisting of a set together with two binary operations usually called addition and multiplication, where the set is an abelian group under addition and a semigroup under multiplication such that multiplication distributes over addition...
R, is a mapping from R to R that satisfies certain conditions outlined directly below. The notion of a p-derivation is related to that of a derivation
Derivation
Derivation may refer to:* Derivation , a function on an algebra which generalizes certain features of the derivative operator* Derivation * Derivation in differential algebra, a unary function satisfying the Leibniz product law...
in differential algebra.
Definition
Let p be a prime number. A p-derivation on a ring is a map of sets that satisfies the following "product ruleProduct rule
In calculus, the product rule is a formula used to find the derivatives of products of two or more functions. It may be stated thus:'=f'\cdot g+f\cdot g' \,\! or in the Leibniz notation thus:...
":
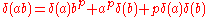
and "sum rule":
.Note that in the "sum rule" we are not really dividing by p, since all the relevant binomial coefficients in the numerator are divisible by p, so this definition applies in the case when
has p-torsion.Relation to Frobenius Endomorphisms
A map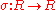 is a lift of the Frobenius endomorphismFrobenius endomorphism
In commutative algebra and field theory, the Frobenius endomorphism is a special endomorphism of commutative rings with prime characteristic p, an important class which includes finite fields. The endomorphism maps every element to its pth power...
provided
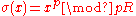. An example such lift could come from the Artin map.If
is a ring with a p-derivation, then the map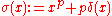 defines a ring endomorphism which is a lift of the frobenius endomorphism. When the ring R is p-torsion free the correspondence is a bijection.Examples
- For
the unique p-derivation is the map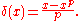
The quotient is well-defined because of Fermat's Little Theorem
Fermat's little theorem
Fermat's little theorem states that if p is a prime number, then for any integer a, a p − a will be evenly divisible by p...
.
- If R is any p-torsion free ring and
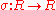is a lift of the Frobenius endomorphism then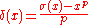
defines a p-derivation.