
Plasma modeling
Encyclopedia
Plasma Modeling refers to solving equations of motion that describe the state of a plasma
. It is generally coupled with Maxwell's Equations
for electromagnetic field
s (or Poisson's Equation
for electrostatic fields). There are several main types of plasma models: single particle, kinetic, fluid, hybrid kinetic/fluid, gyrokinetic and as system of many particles.
In many cases of practical interest, this motion can be treated as the superposition of a relatively fast circular motion around a point called the guiding center
and a relatively slow drift of this point.
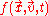
where the independent variables and
and  are position and velocity
are position and velocity
, respectively.
A kinetic description is achieved by solving the Boltzmann equation
or, when the correct description of long-range Coulomb interaction
is necessary, by the Vlasov equation
which contains self-consistent collective electromagnetic field, or by the Fokker-Planck equation
, in which approximations have been used to derive manageable collision terms. The charges and currents produced by the distribution functions self-consistently determine the electromagnetic fields via Maxwell's equations
.
or the Vlasov equation
. The fluid equations are not closed without the determination of transport coefficients such as mobility, diffusion coefficient, averaged collision frequencies, and so on. To determine the transport coefficients, the velocity distribution function must be assumed/chosen. But this assumption can lead to a failure of capturing some physics.
, which is appropriate to systems with a strong background magnetic field, the kinetic equations are averaged over the fast circular motion of the gyroradius. This model has been used extensively for simulation of tokamak
plasma instabilities (for example, the GYRO
and Gyrokinetic ElectroMagnetic
codes), and more recently in astrophysical applications.
Plasma (physics)
In physics and chemistry, plasma is a state of matter similar to gas in which a certain portion of the particles are ionized. Heating a gas may ionize its molecules or atoms , thus turning it into a plasma, which contains charged particles: positive ions and negative electrons or ions...
. It is generally coupled with Maxwell's Equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
for electromagnetic field
Electromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
s (or Poisson's Equation
Poisson's equation
In mathematics, Poisson's equation is a partial differential equation of elliptic type with broad utility in electrostatics, mechanical engineering and theoretical physics...
for electrostatic fields). There are several main types of plasma models: single particle, kinetic, fluid, hybrid kinetic/fluid, gyrokinetic and as system of many particles.
Single Particle Description
The single particle model describes the plasma as individual electrons and ions moving in imposed (rather than self-consistent) electric and magnetic fields. The motion of each particle is thus described by the Lorentz Force Law.In many cases of practical interest, this motion can be treated as the superposition of a relatively fast circular motion around a point called the guiding center
Guiding center
In many cases of practical interest, the motion in a magnetic field of an electrically charged particle can be treated as the superposition of a relatively fast circular motion around a point called the guiding center and a relatively slow drift of this point...
and a relatively slow drift of this point.
Kinetic Description
The kinetic model is the most fundamental way to describe a plasma, resultantly producing a distribution functionDistribution function
In molecular kinetic theory in physics, a particle's distribution function is a function of seven variables, f, which gives the number of particles per unit volume in phase space. It is the number of particles per unit volume having approximately the velocity near the place and time...
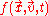
where the independent variables
 and
and  are position and velocity
are position and velocityVelocity
In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...
, respectively.
A kinetic description is achieved by solving the Boltzmann equation
Boltzmann equation
The Boltzmann equation, also often known as the Boltzmann transport equation, devised by Ludwig Boltzmann, describes the statistical distribution of one particle in rarefied gas...
or, when the correct description of long-range Coulomb interaction
Coulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
is necessary, by the Vlasov equation
Vlasov equation
The Vlasov equation is a differential equation describing time evolution of the distribution function of plasma consisting of charged particles with long-range interaction...
which contains self-consistent collective electromagnetic field, or by the Fokker-Planck equation
Fokker-Planck equation
The Fokker–Planck equation describes the time evolution of the probability density function of the velocity of a particle, and can be generalized to other observables as well.It is named after Adriaan Fokkerand Max Planck...
, in which approximations have been used to derive manageable collision terms. The charges and currents produced by the distribution functions self-consistently determine the electromagnetic fields via Maxwell's equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
.
Fluid Description
To reduce the complexities in the kinetic description, the fluid model describes the plasma based on macroscopic quantities (velocity moments of the distribution such as density, mean velocity, and mean energy). The equations for macroscopic quantities, called fluid equations, are obtained by taking velocity moments of the Boltzmann equationBoltzmann equation
The Boltzmann equation, also often known as the Boltzmann transport equation, devised by Ludwig Boltzmann, describes the statistical distribution of one particle in rarefied gas...
or the Vlasov equation
Vlasov equation
The Vlasov equation is a differential equation describing time evolution of the distribution function of plasma consisting of charged particles with long-range interaction...
. The fluid equations are not closed without the determination of transport coefficients such as mobility, diffusion coefficient, averaged collision frequencies, and so on. To determine the transport coefficients, the velocity distribution function must be assumed/chosen. But this assumption can lead to a failure of capturing some physics.
Hybrid Kinetic/Fluid Description
Although the kinetic model describes the physics accurately, it is more complex (and in the case of numerical simulations, more computationally intensive) than the fluid model. The hybrid model is a combination of fluid and kinetic models, treating some components of the system as a fluid, and others kinetically.Gyrokinetic Description
In the gyrokinetic modelGyrokinetics
Gyrokinetics is a branch of plasma physics derived from kinetics and electromagnetism used to describe the low-frequency phenomena in a plasma. The trajectory of charged particles in a magnetic field is a helix that winds around the field line...
, which is appropriate to systems with a strong background magnetic field, the kinetic equations are averaged over the fast circular motion of the gyroradius. This model has been used extensively for simulation of tokamak
Tokamak
A tokamak is a device using a magnetic field to confine a plasma in the shape of a torus . Achieving a stable plasma equilibrium requires magnetic field lines that move around the torus in a helical shape...
plasma instabilities (for example, the GYRO
GYRO
GYRO is a computational plasma physics code developed and maintained at General Atomics. It solves the 5-D coupled gyrokinetic-Maxwell equations using a combination of finite difference, finite element and spectral methods. Given plasma equilibrium data, GYRO can determine the rate of turbulent...
and Gyrokinetic ElectroMagnetic
Gyrokinetic ElectroMagnetic
GEM is short for Gyrokinetic ElectroMagnetic. GEM is a gyrokinetic plasma turbulence simulation that uses the \delta f particle-in-cell method. It is used to study waves, instabilities and nonlinear behavior of tokamak fusion plasmas...
codes), and more recently in astrophysical applications.

