
Runcinated 5-cube
Encyclopedia
5-cube |
Runcinated 5-cube |
Runcinated 5-orthoplex Runcinated 5-orthoplex In six-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation of the regular 5-orthoplex.There are 8 runcinations of the 5-orthoplex with permutations of truncations, and cantellations... |
Runcitruncated 5-cube |
Runcicantellated 5-cube |
Runcicantitruncated 5-cube |
Runcitruncated 5-orthoplex |
Runcicantellated 5-orthoplex |
Runcicantitruncated 5-orthoplex |
| Orthogonal projections in BC5 Coxeter plane | ||
|---|---|---|
In five-dimensional geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, a runcinated 5-cube is a convex uniform 5-polytope that is a runcination
Runcination
In geometry, runcination is an operation that cuts a regular polytope simultaneously along the faces, edges and vertices, creating new facets in place of the original face, edge, and vertex centers....
(a 3rd order truncation) of the regular 5-cube.
There are unique 8 degrees of runcinations of the 5-cube, along with permutations of truncations, and cantellations. Four are more simply constructed relative to the 5-orthoplex.
Runcinated 5-cube
| Runcinated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,3{4,3,3,3} | |
| Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors... |
colspan=2| | |
| 4-faces | 162 | |
| Cells | 1200 | |
| Faces | 2160 | |
| Edges | 1440 | |
| Vertices | 320 | |
| Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:... |
 |
|
| Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example... |
BC5 [4,3,3,3] | |
| Properties | convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... |
|
Coordinates
The Cartesian coordinates of the vertices of a runcinated 5-cube having edge length 2 are all permutations of:
Runcitruncated 5-cube
| Runcitruncated 5-cube | |
|---|---|
| Type | uniform polyteron Uniform polyteron In geometry, a uniform polyteron is a five-dimensional uniform polytope. By definition, a uniform polyteron is vertex-transitive and constructed from uniform polychoron facets.... |
| Schläfli symbol | t0,1,3{4,3,3,3} |
| Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors... s |
|
| 4-faces | 162 |
| Cells | 1440 |
| Faces | 3680 |
| Edges | 3360 |
| Vertices | 960 |
| Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:... |
|
| Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example... s |
BC5, [3,3,3,4] |
| Properties | convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... |
Alternate names
- Runcitruncated penteract
- Prismatotruncated penteract (Acronym: pattin) (Jonathan Bowers)
Construction and coordinates
The Cartesian coordinates of the vertices of a truncated 5-cube having edge length 2 are all permutations of: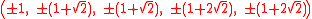
Runcicantellated 5-cube
| Runcicantellated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,2,3{4,3,3,3} | |
| Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors... |
colspan=2| | |
| 4-faces | 162 | |
| Cells | 1200 | |
| Faces | 2960 | |
| Edges | 2880 | |
| Vertices | 960 | |
| Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:... |
||
| Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example... |
BC5 [4,3,3,3] | |
| Properties | convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... |
|
Alternate names
- Runcicantellated penteract
- Prismatorhombated penteract (Acronym: prin) (Jonathan Bowers)
Coordinates
The Cartesian coordinates of the vertices of a runcicantellated 5-cube having edge length 2 are all permutations of:
Runcicantitruncated 5-cube
| Runcicantitruncated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,1,2,3{4,3,3,3} | |
| Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors... |
||
| 4-faces | 162 | |
| Cells | 1440 | |
| Faces | 4160 | |
| Edges | 4800 | |
| Vertices | 1920 | |
| Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:... |
||
| Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example... |
BC5 [4,3,3,3] | |
| Properties | convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... , isogonal Isogonal Isogonal is a mathematical term which means "having similar angles". It occurs in several contexts:*Isogonal polygon, polyhedron, polytope or tiling.*Isogonal trajectory in curve theory.*Isogonal conjugate in triangle geometry.... |
|
Alternate names
- Runcicantitruncated penteract
- Biruncicantitruncated 16-cell / Biruncicantitruncated pentacross
- great prismated penteract (gippin) (Jonathan Bowers)
Coordinates
The Cartesian coordinates of the vertices of an runcicantitruncated tesseract having an edge length of 2 are given by all permutations of coordinates and sign of: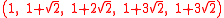
Related polytopes
These polytopes are a part of a set of 31 uniform polytera generated from the regular 5-cube or 5-orthoplex.External links
- Polytopes of Various Dimensions, Jonathan Bowers
- Runcinated uniform polytera (spid), Jonathan Bowers
- Multi-dimensional Glossary

