
Static spherically symmetric perfect fluid
Encyclopedia
In metric theories of gravitation, particularly general relativity
, a static spherically symmetric perfect fluid solution (a term which is often abbreviated as ssspf) is a spacetime
equipped with suitable tensor field
s which models a static round ball of a fluid with isotropic pressure
.
 Such solutions are often used as idealized models of star
Such solutions are often used as idealized models of star
s, especially compact objects such as white dwarf
s and especially neutron star
s. In general relativity, a model of an isolated star (or other fluid ball) generally consists of a fluid-filled interior region, which is technically a perfect fluid
solution of the Einstein field equation, and an exterior region, which is an asymptotically flat vacuum solution
. These two pieces must be carefully matched across the world sheet of a spherical surface, the surface of zero pressure. (There are various mathematical criteria called matching conditions for checking that the required matching has been successfully achieved.) Similar statements hold for other metric theories of gravitation, such as the Brans-Dicke theory
.
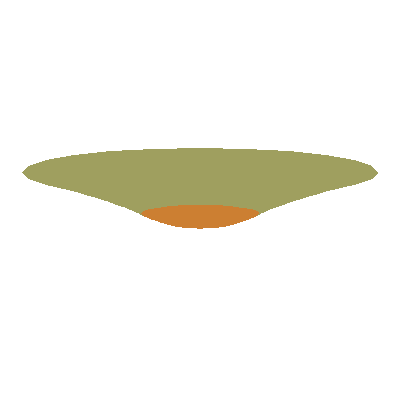
In this article, we will focus on the construction of exact ssspf solutions in our current Gold Standard theory of gravitation, the theory of general relativity. To anticipate, the figure at right depicts (by means of an embedding diagram) the spatial geometry of a simple example of a stellar model in general relativity. The euclidean space in which this two-dimensional Riemannian manifold (standing in for a three-dimensional Riemannian manifold) is embedded has no physical significance, it is merely a visual aid to help convey a quick impression of the kind of geometrical features we will we encounter.
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, a static spherically symmetric perfect fluid solution (a term which is often abbreviated as ssspf) is a spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
equipped with suitable tensor field
Tensor field
In mathematics, physics and engineering, a tensor field assigns a tensor to each point of a mathematical space . Tensor fields are used in differential geometry, algebraic geometry, general relativity, in the analysis of stress and strain in materials, and in numerous applications in the physical...
s which models a static round ball of a fluid with isotropic pressure
Pressure
Pressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
.

Star
A star is a massive, luminous sphere of plasma held together by gravity. At the end of its lifetime, a star can also contain a proportion of degenerate matter. The nearest star to Earth is the Sun, which is the source of most of the energy on Earth...
s, especially compact objects such as white dwarf
White dwarf
A white dwarf, also called a degenerate dwarf, is a small star composed mostly of electron-degenerate matter. They are very dense; a white dwarf's mass is comparable to that of the Sun and its volume is comparable to that of the Earth. Its faint luminosity comes from the emission of stored...
s and especially neutron star
Neutron star
A neutron star is a type of stellar remnant that can result from the gravitational collapse of a massive star during a Type II, Type Ib or Type Ic supernova event. Such stars are composed almost entirely of neutrons, which are subatomic particles without electrical charge and with a slightly larger...
s. In general relativity, a model of an isolated star (or other fluid ball) generally consists of a fluid-filled interior region, which is technically a perfect fluid
Fluid solution
In general relativity, a fluid solution is an exact solution of the Einstein field equation in which the gravitational field is produced entirely by the mass, momentum, and stress density of a fluid....
solution of the Einstein field equation, and an exterior region, which is an asymptotically flat vacuum solution
Vacuum solution (general relativity)
In general relativity, a vacuum solution is a Lorentzian manifold whose Einstein tensor vanishes identically. According to the Einstein field equation, this means that the stress-energy tensor also vanishes identically, so that no matter or non-gravitational fields are present.More generally, a...
. These two pieces must be carefully matched across the world sheet of a spherical surface, the surface of zero pressure. (There are various mathematical criteria called matching conditions for checking that the required matching has been successfully achieved.) Similar statements hold for other metric theories of gravitation, such as the Brans-Dicke theory
Brans-Dicke theory
In theoretical physics, the Brans–Dicke theory of gravitation is a theoretical framework to explain gravitation. It is a well-known competitor of Einstein's more popular theory of general relativity...
.
In this article, we will focus on the construction of exact ssspf solutions in our current Gold Standard theory of gravitation, the theory of general relativity. To anticipate, the figure at right depicts (by means of an embedding diagram) the spatial geometry of a simple example of a stellar model in general relativity. The euclidean space in which this two-dimensional Riemannian manifold (standing in for a three-dimensional Riemannian manifold) is embedded has no physical significance, it is merely a visual aid to help convey a quick impression of the kind of geometrical features we will we encounter.
Short history
We list here a few milestones in the history of exact ssspf solutions in general relativity:- 1916: Schwarzschild fluid solution,
- 1939: The relativistic equation of hydrostatic equilibriumHydrostatic equilibriumHydrostatic equilibrium or hydrostatic balance is the condition in fluid mechanics where a volume of a fluid is at rest or at constant velocity. This occurs when compression due to gravity is balanced by a pressure gradient force...
, the Oppenheimer-Volkov equation, is introduced, - 1939: Tolman gives seven ssspf solutions, two of which are suitable for stellar models,
- 1949: Wyman ssspf and first generating function method,
- 1958: Buchdahl ssspf, a relativistic generalization of a Newtonian polytrope,
- 1967: Kuchowicz ssspf,
- 1969: Heintzmann ssspf,
- 1978: Goldman ssspf,
- 1982: Stewart ssspf,
- 1998: major reviews by Finch & Skea and by Delgaty & Lake,
- 2000: Fodor shows how to generate ssspf solutions using one generating function and differentiation and algebraic operations, but no integrations,
- 2001: Nilsson & Ugla reduce the definition of ssspf solutions with either linearLinearIn mathematics, a linear map or function f is a function which satisfies the following two properties:* Additivity : f = f + f...
or polytropic equations of stateEquation of stateIn physics and thermodynamics, an equation of state is a relation between state variables. More specifically, an equation of state is a thermodynamic equation describing the state of matter under a given set of physical conditions...
to a system of regular ODEs suitable for stability analysis, - 2002: Rahman & Visser give a generating function method using one differentiation, one square root, and one definite integral, in isotropic coordinatesIsotropic coordinatesIn the theory of Lorentzian manifolds, spherically symmetric spacetimes admit a family of nested round spheres. There are several different types of coordinate chart which are adapted to this family of nested spheres; the best known is the Schwarzschild chart, but the isotropic chart is also often...
, with various physical requirements satisfied automatically, and show that every ssspf can be put in Rahman-Visser form, - 2003: Lake extends the long-neglected generating function method of Wyman, for either Schwarzschild coordinatesSchwarzschild coordinatesIn the theory of Lorentzian manifolds, spherically symmetric spacetimes admit a family of nested round spheres. In such a spacetime, a particularly important kind of coordinate chart is the Schwarzschild chart, a kind of polar spherical coordinate chart on a static and spherically symmetric...
or isotropic coordinates, - 2004: Martin & Visser algorithm, another generating function method which uses Schwarzschild coordinates,
- 2004: Martin gives three simple new solutions, one of which is suitable for stellar models,
- 2005: BVW algorithm, apparently the simplest variant now known

