Unitary transformation
Encyclopedia
In mathematics, a unitary transformation may be informally defined as a transformation
that respects the inner product: the inner product of two vectors before the transformation is equal to their inner product after the transformation.
More precisely, a unitary transformation is an isomorphism
between two Hilbert space
s. In other words, a unitary transformation is a bijective function
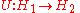
where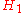
and 
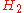are Hilbert spaces, such that
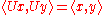
for all
and 
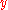in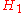 
. A unitary transformation is an isometry
, as one can see by setting
in this formula.
In the case when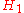
and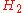 
are the same space, a unitary transformation is an automorphism
of that Hilbert space, and then it is also called a unitary operator
.
A closely related notion is that of antiunitary transformation, which is a bijective function
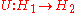
between two complex
Hilbert spaces such that
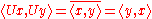
for all
and 
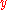in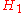 
, where the horizontal bar represents the complex conjugate
.
Transformation (mathematics)
In mathematics, a transformation could be any function mapping a set X on to another set or on to itself. However, often the set X has some additional algebraic or geometric structure and the term "transformation" refers to a function from X to itself that preserves this structure.Examples include...
that respects the inner product: the inner product of two vectors before the transformation is equal to their inner product after the transformation.
More precisely, a unitary transformation is an isomorphism
Isomorphism
In abstract algebra, an isomorphism is a mapping between objects that shows a relationship between two properties or operations. If there exists an isomorphism between two structures, the two structures are said to be isomorphic. In a certain sense, isomorphic structures are...
between two Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
s. In other words, a unitary transformation is a bijective function
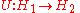
where
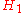and 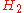are Hilbert spaces, such that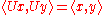for all
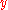and in 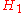. A unitary transformation is an isometryIsometry
In mathematics, an isometry is a distance-preserving map between metric spaces. Geometric figures which can be related by an isometry are called congruent.Isometries are often used in constructions where one space is embedded in another space...
, as one can see by setting
in this formula.In the case when
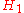and 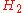are the same space, a unitary transformation is an automorphismAutomorphism
In mathematics, an automorphism is an isomorphism from a mathematical object to itself. It is, in some sense, a symmetry of the object, and a way of mapping the object to itself while preserving all of its structure. The set of all automorphisms of an object forms a group, called the automorphism...
of that Hilbert space, and then it is also called a unitary operator
Unitary operator
In functional analysis, a branch of mathematics, a unitary operator is a bounded linear operator U : H → H on a Hilbert space H satisfyingU^*U=UU^*=I...
.
A closely related notion is that of antiunitary transformation, which is a bijective function
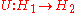
between two complex
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
Hilbert spaces such that
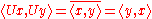
for all
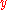and in 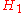, where the horizontal bar represents the complex conjugateComplex conjugate
In mathematics, complex conjugates are a pair of complex numbers, both having the same real part, but with imaginary parts of equal magnitude and opposite signs...
.
See also
- Time reversalTime reversalTime reversal may refer to:* In physics, T-symmetry - the study of thermodynamics and the symmetry of certain physical laws where the concept of time is reversed — ie...
- antiunitary
- Unitary groupUnitary groupIn mathematics, the unitary group of degree n, denoted U, is the group of n×n unitary matrices, with the group operation that of matrix multiplication. The unitary group is a subgroup of the general linear group GL...
- Unitary operatorUnitary operatorIn functional analysis, a branch of mathematics, a unitary operator is a bounded linear operator U : H → H on a Hilbert space H satisfyingU^*U=UU^*=I...
- Unitary matrix
- Wigner's TheoremWigner's theoremWigner's theorem, proved by Eugene Wigner in 1931, is a cornerstone of the mathematical formulation of quantum mechanics. The theorem specifies how physical symmetries such as rotations, translations, and CPT act on the Hilbert space of states....