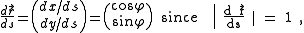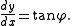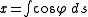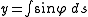The Whewell equation of a plane curve

Plane curve

In mathematics, a plane curve is a curve in a Euclidean plane . The most frequently studied cases are smooth plane curves , and algebraic plane curves....

 is an equation

Equation

An equation is a mathematical statement that asserts the equality of two expressions. In modern notation, this is written by placing the expressions on either side of an equals sign , for examplex + 3 = 5\,asserts that x+3 is equal to 5...

 that relates the tangential angle

Tangential angle

In geometry, the tangential angle of a curve in the Cartesian plane, at a specific point, is the angle between the tangent line to the curve at the given point and the x-axis. In geometry, the tangential angle of a curve in the Cartesian plane, at a specific point, is the angle between the tangent...

 () with arclength (), where the tangential angle is angle between the tangent to the curve and the x-axis and the arc length is the distance along the curve from a fixed point. These quantities do not depend on the coordinate system used except for the choice of the direction of the x-axis, so this is an intrinsic equation of the curve, or, less precisely, the intrinsic equation. If a curve is obtained from another by translation then their Whewell equations will be the same.

When the relation is a function, so that tangential angle is given as a function of arclength, certain properties become easy to manipulate. In particular, the derivative of the tangential angle with respect to arclength is equal to the curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

. Thus, taking the derivative of the Whewell equation yields a Cesàro equation

Cesàro equation

In geometry, the Cesàro equation of a plane curve is an equation relating curvature to arc length . It may also be given as an equation relating the radius of curvature to arc length. Two congruent curves will have the same Cesàro equation...

 for the same curve.

The term is named after William Whewell

William Whewell

William Whewell was an English polymath, scientist, Anglican priest, philosopher, theologian, and historian of science. He was Master of Trinity College, Cambridge.-Life and career:Whewell was born in Lancaster...

, who introduced the concept in 1849, in a paper in the Cambridge Philosophical Transactions

Cambridge Philosophical Society

The Cambridge Philosophical Society is a scientific society at University of Cambridge. It was founded in 1819. The name derives from the medieval use of the word philosophy to denote any research undertaken outside the fields of theology and medicine...

. In his conception, the angle used is the deviation from the direction of the curve at some fixed starting point, and this convention is sometimes used by other authors as well. This is equivalent to the definition given here by the addition of a constant to the angle or by rotating the curve.

Properties

If the curve is given parametrically in terms of the arc length , then 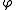is determined by

which implies

Parametric equations for the curve can be obtained by integrating:

Since the curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

 is defined by

the Cesàro equation

Cesàro equation

In geometry, the Cesàro equation of a plane curve is an equation relating curvature to arc length . It may also be given as an equation relating the radius of curvature to arc length. Two congruent curves will have the same Cesàro equation...

 is easily obtained by differentiating the Whewell equation.

Examples

Curve	Equation
Line Line (mathematics) The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
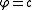Circle Circle A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
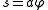Catenary Catenary In physics and geometry, the catenary is the curve that an idealised hanging chain or cable assumes when supported at its ends and acted on only by its own weight. The curve is the graph of the hyperbolic cosine function, and has a U-like shape, superficially similar in appearance to a parabola...
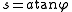