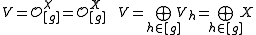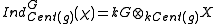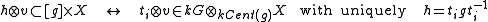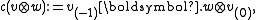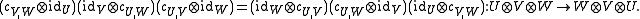Definition

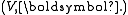• is a left H-module
  Module (mathematics)
  In abstract algebra, the concept of a module over a ring is a generalization of the notion of vector space, wherein the corresponding scalars are allowed to lie in an arbitrary ring...
  
  , where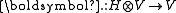 denotes the left action of H on V and ⊗ denotes a tensor product
  Tensor product
  In mathematics, the tensor product, denoted by ⊗, may be applied in different contexts to vectors, matrices, tensors, vector spaces, algebras, topological vector spaces, and modules, among many other structures or objects. In each case the significance of the symbol is the same: the most general...
  
  ,
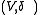• is a left H-comodule
  Comodule
  In mathematics, a comodule or corepresentation is a concept dual to a module. The definition of a comodule over a coalgebra is formed by dualizing the definition of a module over an associative algebra.-Formal definition:...
  
  , where denotes the left coaction of H on V,
• the maps and satisfy the compatibility condition

for all ,

where, using Sweedler notation

Coalgebra

In mathematics, coalgebras or cogebras are structures that are dual to unital associative algebras. The axioms of unital associative algebras can be formulated in terms of commutative diagrams...

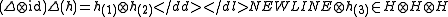, denotes the twofold coproduct of 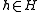, and .

Examples

• Any left H-module over a cocommutative Hopf algebra H is a Yetter–Drinfel'd module with the trivial left coaction .
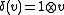• The trivial module 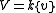with 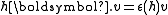, , 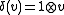is a Yetter–Drinfel'd module for all Hopf algebras H.
• If H is the group algebra
  Group ring
  In algebra, a group ring is a free module and at the same time a ring, constructed in a natural way from any given ring and any given group. As a free module, its ring of scalars is the given ring and its basis is one-to-one with the given group. As a ring, its addition law is that of the free...
  
   kG of an abelian group
  Abelian group
  In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
  
   G, then Yetter–Drinfel'd modules over H are precisely the G-graded G-modules. This means that

,

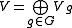where each 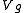is a G-submodule of V.

• More generally, if the group G is not abelian, then Yetter–Drinfel'd modules over H=kG are G-modules with a G-gradation

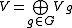, such that .

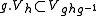• Over the basfield 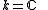all finite-dimensional, irreducible/simple Yetter–Drinfel'd modules over a (nonabelian) group H=kG are uniquely given through a conjugacy class
  Conjugacy class
  In mathematics, especially group theory, the elements of any group may be partitioned into conjugacy classes; members of the same conjugacy class share many properties, and study of conjugacy classes of non-abelian groups reveals many important features of their structure...
  
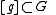    together with 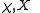(character of) an irreducible group representation of the centralizer
  Centralizer and normalizer
  In group theory, the centralizer and normalizer of a subset S of a group G are subgroups of G which have a restricted action on the elements of S and S as a whole, respectively...
  
    of some representing :

  

  • As G-module take 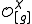to be the induced module
    Induced representation
    In mathematics, and in particular group representation theory, the induced representation is one of the major general operations for passing from a representation of a subgroup H to a representation of the group G itself. It was initially defined as a construction by Frobenius, for linear...
    
     of :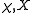

  

  (this can be proven easily not to depend on the choice of g)

  • To define the G-graduation (comodule) assign any element to the graduation layer:

  

  • It is very custom to directly construct as direct sum of X´s and write down the G-action by choice of a specific set of representatives 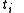for the 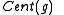-cosets
    Coset
    In mathematics, if G is a group, and H is a subgroup of G, and g is an element of G, thenA coset is a left or right coset of some subgroup in G...
    
    . From this approach, one often writes

  

  (this notation emphasizes the graduation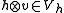, rather than the module structure)

Braiding

Let H be a Hopf algebra with invertible antipode S, and let V, W be Yetter–Drinfel'd modules over H. Then the map ,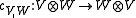

is invertible with inverse

Further, for any three Yetter–Drinfel'd modules U, V, W the map c satisfies the braid relation

Yetter–Drinfel'd category

A monoidal category

Monoidal category

In mathematics, a monoidal category is a category C equipped with a bifunctorwhich is associative, up to a natural isomorphism, and an object I which is both a left and right identity for ⊗, again up to a natural isomorphism...

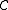consisting of Yetter–Drinfel'd modules over a Hopf algebra H with bijective antipode is called a Yetter–Drinfel'd category. It is a braided monoidal category with the braiding c above. The category of Yetter–Drinfel'd modules over a Hopf algebra H with bijective antipode is denoted by .

The source of this article is wikipedia, the free encyclopedia.  The text of this article is licensed under the GFDL.