Atomic spectral line - AbsoluteAstronomy.com

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

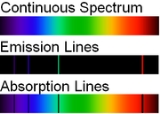
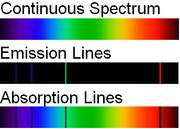
, atomic spectral lines are of two types:

An emission line is formed when an electron makes a transition from a particular discrete energy level
Energy level
A quantum mechanical system or particle that is bound -- that is, confined spatially—can only take on certain discrete values of energy. This contrasts with classical particles, which can have any energy. These discrete values are called energy levels...

of an atom, to a lower energy state, emitting a photon of a particular energy and wavelength. A spectrum of many such photons will show an emission spike at the wavelength associated with these photons.
An absorption line is formed when an electron makes a transition from a lower to a higher discrete energy state, with a photon being absorbed in the process. These absorbed photons generally come from background continuum radiation and a spectrum will show a drop in the continuum radiation at the wavelength associated with the absorbed photons.

The two states must be bound state

Bound state

In physics, a bound state describes a system where a particle is subject to a potential such that the particle has a tendency to remain localised in one or more regions of space...

s in which the electron is bound to the atom, so the transition is sometimes referred to as a "bound–bound" transition, as opposed to a transition in which the electron is ejected out of the atom completely ("bound–free" transition) into a continuum

Continuous spectrum

The spectrum of a linear operator is commonly divided into three parts: point spectrum, continuous spectrum, and residual spectrum.If H is a topological vector space and A:H \to H is a linear map, the spectrum of A is the set of complex numbers \lambda such that A - \lambda I : H \to H is not...

state, leaving an ionized

Ionization

Ionization is the process of converting an atom or molecule into an ion by adding or removing charged particles such as electrons or other ions. This is often confused with dissociation. A substance may dissociate without necessarily producing ions. As an example, the molecules of table sugar...

atom, and generating continuum radiation.

A photon

Photon

In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...

with an energy equal to the energy difference between the levels is released or absorbed in the process. The frequency

at which the spectral line occurs is related to the photon energy

by Planck's law

where

is Planck's constant.

Emission and absorption coefficients

An atomic spectral line refers to emission and absorption events in a gas in which

is the density of atoms in the upper energy state for the line, and

is the density of atoms in the lower energy state for the line.

The emission of atomic line radiation may be described by an emission coefficient

with units of energy/time/volume/solid angle. ε dt dV dΩ is then the energy emitted by a volume element

in time

into solid angle

. For atomic line radiation:

where

is the Einstein coefficient for spontaneous emission, which is fixed by the intrinsic properties of the relevant atom for the two relevant energy levels.

The absorption of atomic line radiation may be described by an absorption coefficient

with units of 1/length. The expression κ' dx gives the fraction of intensity absorbed for a light beam at frequency ν while traveling distance dx. The absorption coefficient is given by:

where

and

are the Einstein coefficients for photo absorption and induced emission respectively. Like the coefficient

, these are also fixed by the intrinsic properties of the relevant atom for the two relevant energy levels. For thermodynamics and for the application of Kirchhoff's law, it is necessary that the total absorption be expressed as the algebraic sum of two components, described respectively by

and

, which may be regarded as positive and negative absorption, respectively the photo absorption and what is commonly called stimulated or induced emission.

The above equations have ignored the influence of the spectral line shape. To be accurate, the above equations need to be multiplied by the (normalized) spectral line shape, in which case the units will change to include a 1/Hz term.

The number densities

and

and the Einstein coefficients provide sufficient information to determine the absorption and emission rates, without further need for information about the physical state of the gas or about the local radiation field. Thus the above absorption and emission coefficients work for any state of the gas, so for equilibrium states and even for laser conditions.

In contrast to the Einstein coefficients, the number densities, however, are not set simply by the intrinsic properties of the relevant atom.

Equilibrium conditions

The number densities

and

are set by the physical state of the gas in which the spectral line occurs, including the local specific radiative intensity

Specific radiative intensity

Specific intensity is a fundamental quantity used in theoretical physics that fully describes the field of classical electromagnetic radiation of any kind, including thermal radiation and light. It is a description based on radiometry rather than Maxwellian electromagnetic fields or photon...

. When that state is either one of strict thermodynamic equilibrium

Thermodynamic equilibrium

In thermodynamics, a thermodynamic system is said to be in thermodynamic equilibrium when it is in thermal equilibrium, mechanical equilibrium, radiative equilibrium, and chemical equilibrium. The word equilibrium means a state of balance...

, or one of so-called 'local thermodynamic equilibrium', then the distribution of atomic states of excitation (which includes

and

) determines the rates of atomic emissions and absorptions to be such that Kirchhoff's law of equality of radiative absorptivity and emissivity

Kirchhoff's law of thermal radiation

In thermodynamics, Kirchhoff's law of thermal radiation, or Kirchhoff's law for short, is a general statement equating emission and absorption in heated objects, proposed by Gustav Kirchhoff in 1859, following from general considerations of thermodynamic equilibrium and detailed balance.An object...

shall hold. In strict thermodynamic equilibrium, the radiation field is said to be black body

Black body

A black body is an idealized physical body that absorbs all incident electromagnetic radiation. Because of this perfect absorptivity at all wavelengths, a black body is also the best possible emitter of thermal radiation, which it radiates incandescently in a characteristic, continuous spectrum...

radiation, and is described by Planck's law. For local thermodynamic equilibrium, the radiation field does not have be a black body field, but the rate of interatomic collisions must vastly exceed the rates of absorption and emission of quanta of light, so that the interatomic collisions entirely dominate the distribution of states of atomic excitation.

In the cases of thermodynamic equilibrium

Thermodynamic equilibrium

and of local thermodynamic equilibrium, the densities of the atoms, both excited and unexcited, may be calculated from the Maxwell–Boltzmann distribution, but for other cases, (e.g. laser

Laser

A laser is a device that emits light through a process of optical amplification based on the stimulated emission of photons. The term "laser" originated as an acronym for Light Amplification by Stimulated Emission of Radiation...

s) the calculation is more complicated.

The Einstein coefficients

In 1916, Albert Einstein

Albert Einstein

Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...

proposed that there are essentially three processes occurring in the formation of an atomic spectral line. The three processes are referred to as spontaneous emission, stimulated emission and absorption and with each is associated an Einstein coefficient which is a measure of the probability of that particular process occurring.

Spontaneous emission

Spontaneous emission is the process by which an electron "spontaneously" (i.e. without any outside influence) decays from a higher energy level to a lower one. The process is described by the Einstein coefficient A₂₁ (s⁻¹) which gives the probability per unit time that an electron in state 2 with energy

will decay spontaneously to state 1 with energy

, emitting a photon with an energy

. Due to the energy-time uncertainty principle, the transition actually produces photons within a narrow range of frequencies called the spectral linewidth

Spectral linewidth

The spectral linewidth characterizes the width of a spectral line, such as in the electromagnetic emission spectrum of an atom, or the frequency spectrum of an acoustic or electronic system...

. If

is the number density of atoms in state i then the change in the number density of atoms in state 1 per unit time due to spontaneous emission will be:

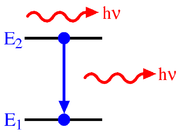
Stimulated emission

In optics, stimulated emission is the process by which an atomic electron interacting with an electromagnetic wave of a certain frequency may drop to a lower energy level, transferring its energy to that field. A photon created in this manner has the same phase, frequency, polarization, and...

(also known as induced emission) is the process by which an electron is induced to jump from a higher energy level to a lower one by the presence of electromagnetic radiation at (or near) the frequency of the transition. The process is described by the Einstein coefficient

(sr·m²·Hz·W⁻¹·s⁻¹ = sr·m²·J⁻¹·s⁻¹), which gives the probability per unit time per unit spectral radiance of the radiation field that an electron in state 2 with energy

will decay to state 1 with energy

, emitting a photon with an energy

. The change in the number density of atoms in state 1 per unit time due to induced emission will be:

where

is the radiation density of the radiation field at the frequency of the transition (see Planck's law).

Stimulated emission is one of the fundamental processes that led to the development of the laser

Laser

Photo absorption

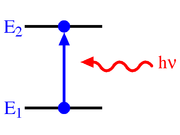
Absorption is the process by which a photon is absorbed by the atom, causing an electron to jump from a lower energy level to a higher one. The process is described by the Einstein coefficient

(sr·m²·Hz·W⁻¹·s⁻¹ = sr·m²·J⁻¹·s⁻¹), which gives the probability per unit time per unit spectral radiance of the radiation field that an electron in state 1 with energy

will absorb a photon with an energy

and jump to state 2 with energy

. The change in the number density of atoms in state 1 per unit time due to absorption will be:

Detailed balancing

The Einstein coefficients are fixed probabilities associated with each atom, and do not depend on the state of the gas of which the atoms are a part. Therefore, any relationship that we can derive between the coefficients at, say, thermal equilibrium will be valid universally.

At equilibrium, we will have a simple balancing, in which the net change in the number of any excited atoms is zero, being balanced by loss and gain due to all processes. With respect to bound-bound transitions, we will have detailed balancing

Detailed balance

The principle of detailed balance is formulated for kinetic systems which are decomposed into elementary processes : At equilibrium, each elementary process should be equilibrated by its reverse process....

as well, which states that the net exchange between any two levels will be balanced. This is because the probabilities of transition cannot be affected by the presence or absence of other excited atoms. Detailed balance (valid only at equilibrium) requires that the change in time of the number of atoms in level 1 due to the above three processes be zero:

Along with detailed balancing, we may use our knowledge of the equilibrium energy distribution of the atoms, as stated in the Maxwell–Boltzmann distribution , and the equilibrium distribution of the photons, as stated in Planck's law of black body radiation

Planck's law of black body radiation

In physics, Planck's law describes the amount of energy emitted by a black body in radiation of a certain wavelength . The law is named after Max Planck, who originally proposed it in 1900. The law was the first to accurately describe black body radiation, and resolved the ultraviolet catastrophe...

to derive universal relationships between the Einstein coefficients.

From the Maxwell–Boltzmann distribution we have for the number of excited atomic specie i:

where n is the total density of the atomic specie, excited and unexcited, k is Boltzmann's constant, T is the temperature

Temperature

Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...

is the degeneracy of state i, and Z is the partition function

Partition function (statistical mechanics)

Partition functions describe the statistical properties of a system in thermodynamic equilibrium. It is a function of temperature and other parameters, such as the volume enclosing a gas...

. From Planck's law of black body radiation we have for the spectral radiance at frequency

where:

where

is the speed of light

Speed of light

The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...

and

is Planck's constant.
Note that in some treatments, the blackbody energy density is used rather than the spectral radiance, in which case:

Substituting these expressions into the equation of detailed balancing and remembering that

yields:

separating to:

The above equation must hold at any temperature, so

Therefore the three Einstein coefficients are interrelated by:

and

When this relation is inserted into the original equation, one can also find a relation between

and

, involving Planck's law.

Oscillator strengths

The oscillator strength

is defined by the following relation to the cross section

for absorption:

where

is the electron charge and

is the electron mass. This allows all three Einstein coefficients to be expressed in terms of the single oscillator strength associated with the particular atomic spectral line:

External links

Emission Spectra from various light sources

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.

Emission and absorption coefficients

Equilibrium conditions

The Einstein coefficients

Spontaneous emission

Stimulated emission

Photo absorption

Detailed balancing

Oscillator strengths

See also

External links