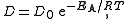Diffusivity (biology)
Encyclopedia
Diffusivity or diffusion
coefficient is a proportionality constant between the molar flux due to molecular diffusion and the gradient in the concentration of the species (or the driving force for diffusion). Diffusivity is encountered in Fick's law and numerous other equations of physical chemistry
.
It is generally prescribed for a given pair of species. For a multi-component system, it is prescribed for each pair of species in the system.
The higher the diffusivity (of one substance with respect to another), the faster they diffuse into each other.
This coefficient has an SI unit of m2/s (length2/time).
The diffusion coefficient in solids at different temperatures is often found to be well predicted by
where
An equation of this form is known as the Arrhenius equation
.
An approximate dependence of the diffusion coefficient on temperature in liquids can often be found using Stokes–Einstein equation, which predicts that:
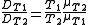
where:
The dependence of the diffusion coefficient on temperature for gases can be expressed using the Chapman–Enskog theory (predictions accurate on average to about 8%):
where:
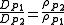
where:
in nature, because it is not individual pores but the entire pore space that needs to be considered. The effective diffusion coefficient for transport through the pores, De, is estimated as follows:
where:
The transport-available porosity
equals the total porosity less the pores which, due to their size, are not accessible to the diffusing particles, and less dead-end and blind pores (i.e., pores without being connected to the rest of the pore system). The constrictivity describes the slowing down of diffusion by increasing the viscosity
in narrow pores as a result of greater proximity to the average pore wall. It is a function of pore diameter and the size of the diffusing particles.
Diffusion
Molecular diffusion, often called simply diffusion, is the thermal motion of all particles at temperatures above absolute zero. The rate of this movement is a function of temperature, viscosity of the fluid and the size of the particles...
coefficient is a proportionality constant between the molar flux due to molecular diffusion and the gradient in the concentration of the species (or the driving force for diffusion). Diffusivity is encountered in Fick's law and numerous other equations of physical chemistry
Physical chemistry
Physical chemistry is the study of macroscopic, atomic, subatomic, and particulate phenomena in chemical systems in terms of physical laws and concepts...
.
It is generally prescribed for a given pair of species. For a multi-component system, it is prescribed for each pair of species in the system.
The higher the diffusivity (of one substance with respect to another), the faster they diffuse into each other.
This coefficient has an SI unit of m2/s (length2/time).
Temperature dependence of the diffusion coefficient
Typically, a compound's diffusion coefficient is ~10,000× as great in air than in water. Carbon dioxide in air has a diffusion coefficient of 16 mm2/s, and in water its coefficient is 0.0016 mm2/s.The diffusion coefficient in solids at different temperatures is often found to be well predicted by
where
-
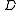is the diffusion coefficient -
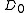is the maximum diffusion coefficient (at infinite temperature) -
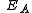is the activation energyActivation energyIn chemistry, activation energy is a term introduced in 1889 by the Swedish scientist Svante Arrhenius that is defined as the energy that must be overcome in order for a chemical reaction to occur. Activation energy may also be defined as the minimum energy required to start a chemical reaction...
for diffusion in dimensions of [energy (amount of substance)−1] -
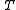is the temperature in units of [absolute temperature] (kelvinKelvinThe kelvin is a unit of measurement for temperature. It is one of the seven base units in the International System of Units and is assigned the unit symbol K. The Kelvin scale is an absolute, thermodynamic temperature scale using as its null point absolute zero, the temperature at which all...
s or degrees Rankine) -
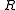is the gas constantGas constantThe gas constant is a physical constant which is featured in many fundamental equations in the physical sciences, such as the ideal gas law and the Nernst equation. It is equivalent to the Boltzmann constant, but expressed in units of energy The gas constant (also known as the molar, universal,...
in dimensions of [energy temperature−1 (amount of substance)−1]
An equation of this form is known as the Arrhenius equation
Arrhenius equation
The Arrhenius equation is a simple, but remarkably accurate, formula for the temperature dependence of the reaction rate constant, and therefore, rate of a chemical reaction. The equation was first proposed by the Dutch chemist J. H. van 't Hoff in 1884; five years later in 1889, the Swedish...
.
An approximate dependence of the diffusion coefficient on temperature in liquids can often be found using Stokes–Einstein equation, which predicts that:
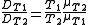
where:
- T1 and T2 denote temperatures 1 and 2, respectively
- D is the diffusion coefficient (cm2/s)
- T is the absolute temperature (K),
- μ is the dynamic viscosity of the solvent (Pa·s)
The dependence of the diffusion coefficient on temperature for gases can be expressed using the Chapman–Enskog theory (predictions accurate on average to about 8%):
where:
- 1 and 2 index the two kinds of molecules present in the gaseous mixture
- T – temperature (K)
- M – molar mass (g/mol)
- p – pressure (atm)
-
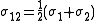– the average collision diameter (the values are tabulated) (Å) - Ω – a temperature-dependent collision integral (the values are tabulated but usually of order 1) (dimensionless).
- D – diffusion coefficient (which is expressed in cm2/s when the other magnitudes are expressed in the units as given above).
Pressure dependence of the diffusion coefficient
For self-diffusion in gases at two different pressures (but the same temperature), the following empirical equation has been suggested: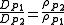where:
- P1 and P2 denote pressures 1 and 2, respectively
- D is the diffusion coefficient (m2/s)
- ρ is the gas mass density (kg/m3)
Effective diffusivity in porous media
The effective diffusion coefficient describes diffusion through the pore space of porous media. It is macroscopicMacroscopic
The macroscopic scale is the length scale on which objects or processes are of a size which is measurable and observable by the naked eye.When applied to phenomena and abstract objects, the macroscopic scale describes existence in the world as we perceive it, often in contrast to experiences or...
in nature, because it is not individual pores but the entire pore space that needs to be considered. The effective diffusion coefficient for transport through the pores, De, is estimated as follows:
where:
- D is the diffusion coefficient in gas or liquid filling the pores (m2s−1)
- εt is the porosityPorosityPorosity or void fraction is a measure of the void spaces in a material, and is a fraction of the volume of voids over the total volume, between 0–1, or as a percentage between 0–100%...
available for the transport (dimensionless) - δ is the constrictivityConstrictivityConstrictivity is a dimensionless parameter used to describe transport processes in porous media.Constrictivity is viewed to depend on the ratio of the diameter of the diffusing particle to the pore diameter. The value of constrictivity is always less than 1...
(dimensionless) - τ is the tortuosityTortuosityTortuosity is a property of curve being tortuous . There have been several attempts to quantify this property. Tortuosity is commonly used to describe diffusion in porous media...
(dimensionless)
The transport-available porosity
Porosity
Porosity or void fraction is a measure of the void spaces in a material, and is a fraction of the volume of voids over the total volume, between 0–1, or as a percentage between 0–100%...
equals the total porosity less the pores which, due to their size, are not accessible to the diffusing particles, and less dead-end and blind pores (i.e., pores without being connected to the rest of the pore system). The constrictivity describes the slowing down of diffusion by increasing the viscosity
Viscosity
Viscosity is a measure of the resistance of a fluid which is being deformed by either shear or tensile stress. In everyday terms , viscosity is "thickness" or "internal friction". Thus, water is "thin", having a lower viscosity, while honey is "thick", having a higher viscosity...
in narrow pores as a result of greater proximity to the average pore wall. It is a function of pore diameter and the size of the diffusing particles.
See also
- Atomic diffusionAtomic diffusionAtomic diffusion is a diffusion process whereby the random thermally-activated movement of atoms in a solid results in the net transport of atoms. For example, helium atoms inside a balloon can diffuse through the wall of the balloon and escape, resulting in the balloon slowly deflating. Other air...
- Effective diffusion coefficientEffective diffusion coefficientThe effective diffusion coefficient of a diffusant in atomic diffusion of solid polycrystalline materials like metal alloys is often represented as a weighted average of the grain boundary diffusion coefficient and the lattice diffusion coefficient...
- Lattice diffusion coefficientLattice diffusion coefficientLattice diffusion refers to atomic diffusion within a crystalline lattice . Diffusion within the crystal lattice occurs by either interstitial or substitutional mechanisms and is referred to as lattice diffusion...
- Knudsen diffusionKnudsen diffusionKnudsen diffusion is a means of diffusion that occurs in a long pore with a narrow diameter because molecules frequently collide with the pore wall.Consider the diffusion of gas molecules through very small capillary pores...