
Earth ellipsoid
Encyclopedia
An Earth ellipsoid is a mathematical figure approximating the shape of the Earth
, used as a reference frame
for computations in geodesy, astronomy and the geosciences. Various different ellipsoids have been used as approximations.
It is an ellipsoid of rotation, whose short (polar) axis (connecting the two flattest spots called geographical north and south poles) is approximately aligned with the rotation axis of the Earth. The ellipsoid is defined by the equatorial axis a and the polar axis b; their difference is about 21 km or 0,3 per cent. Additional parameters are the mass function
J2, the correspondent gravity formula, and the rotation period (usually 86164 seconds).
Many methods exist for determination of the axes of an Earth ellipsoid, ranging from meridian arc
s up to modern satellite geodesy
or the analysis and interconnection of continental geodetic network
s. Amongst the different set of data used in national surveys are several of special importance: the Bessel ellipsoid
of 1841, the international Hayford ellipsoid
of 1924, and (for GPS positioning) the WGS84 ellipsoid.
of the Earth's surface curvature is called the mean Earth Ellipsoid. It refers to a theoretical coherence between the geographic latitude and the meridional curvature of the geoid
. The latter is close to the mean sea level, and therefore an ideal Earth ellipsoid has the same volume
as the geoid.
While the mean Earth ellipsoid is the ideal basis of global geodesy, for regional
networks a so called reference ellipsoid
may be the better choice. When geodetic measurements have to be computed on a mathematical reference surface, this surface should have a similar curvature as the regional geoid. Otherwise the reduction
of the measurements would get small distortions.
This is the reason for the "long life" of former reference ellipsoids like the Hayford
or the Bessel ellipsoid
, despite of the fact that their main axes deviate by several hundred meters from the modern values. Another reason is a juridical one: the coordinates of millions of boundary stones should remain fixed for a long period. If their reference surface would change, the coordinates themselves would also change.
However, for international
networks, GPS positioning or astronautics
, these regional reasons are less relevant. As the knowledge of Earth's figure
is increasingly accurate, the International Geoscientific Union IUGG usually adopts the axes of the Earth ellipsoid to the best available data.
Figure of the Earth
The expression figure of the Earth has various meanings in geodesy according to the way it is used and the precision with which the Earth's size and shape is to be defined. The actual topographic surface is most apparent with its variety of land forms and water areas. This is, in fact, the surface...
, used as a reference frame
Frame of reference
A frame of reference in physics, may refer to a coordinate system or set of axes within which to measure the position, orientation, and other properties of objects in it, or it may refer to an observational reference frame tied to the state of motion of an observer.It may also refer to both an...
for computations in geodesy, astronomy and the geosciences. Various different ellipsoids have been used as approximations.
It is an ellipsoid of rotation, whose short (polar) axis (connecting the two flattest spots called geographical north and south poles) is approximately aligned with the rotation axis of the Earth. The ellipsoid is defined by the equatorial axis a and the polar axis b; their difference is about 21 km or 0,3 per cent. Additional parameters are the mass function
Probability mass function
In probability theory and statistics, a probability mass function is a function that gives the probability that a discrete random variable is exactly equal to some value...
J2, the correspondent gravity formula, and the rotation period (usually 86164 seconds).
Many methods exist for determination of the axes of an Earth ellipsoid, ranging from meridian arc
Meridian arc
In geodesy, a meridian arc measurement is a highly accurate determination of the distance between two points with the same longitude. Two or more such determinations at different locations then specify the shape of the reference ellipsoid which best approximates the shape of the geoid. This...
s up to modern satellite geodesy
Satellite geodesy
Satellite geodesy is the measurement of the form and dimensions of the Earth, the location of objects on its surface and the figure of the Earth's gravity field by means of artificial satellite techniques—geodesy by means of artificial satellites...
or the analysis and interconnection of continental geodetic network
Geodetic network
A geodetic network is a network of triangles which are measured exactly by techniques of terrestrial surveying or by satellite geodesy.In "classical geodesy" this is done by triangulation, based on measurements of angles and of some spare distances; the precise orientation to the geographic north...
s. Amongst the different set of data used in national surveys are several of special importance: the Bessel ellipsoid
Bessel ellipsoid
The Bessel ellipsoid is an important reference ellipsoid of geodesy. It is currently used by several countries for their national geodetic surveys, in Europe and on other continents, but will be replaced in the next decades by modern ellipsoids of satellite geodesy.The Bessel ellipsoid was derived...
of 1841, the international Hayford ellipsoid
Hayford ellipsoid
The Hayford ellipsoid is a geodetic reference ellipsoid, named after the US geodesist John Fillmore Hayford , which was introduced in 1910. The Hayford ellipsoid was also referred to as the International ellipsoid 1924 after it had been adopted by the International Union of Geodesy and Geophysics...
of 1924, and (for GPS positioning) the WGS84 ellipsoid.
Historical Earth ellipsoids
The following table lists 9 ellipsoids which (except Clarke's) were the best estimation of the Earth's figure when they were published:| Name | Equatorial axis (m) | Polar axis (m) | Inverse flattening,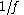 |
|---|---|---|---|
| Delambre, France 1810 | 6,376,985.0 | 308,6465 | |
| Airy 1830 George Biddell Airy Sir George Biddell Airy PRS KCB was an English mathematician and astronomer, Astronomer Royal from 1835 to 1881... |
6,377,563.4 | 6,356,256.91 | 299.3249646 |
| Bessel 1841 Bessel ellipsoid The Bessel ellipsoid is an important reference ellipsoid of geodesy. It is currently used by several countries for their national geodetic surveys, in Europe and on other continents, but will be replaced in the next decades by modern ellipsoids of satellite geodesy.The Bessel ellipsoid was derived... |
6,377,397.155 | 6,356,078.963 | 299.1528128 |
| Alexander Ross Clarke Alexander Ross Clarke Alexander Ross Clarke was a British geodesist, primarily remembered for his work defining different reference ellipsoids approximating the shape of the geoid.Clarke was born on December 16, 1828 in Reading, Berkshire, England... |
6,378,206.4 | 6,356,583.8 | 294.9786982 |
| Helmert 1906 Friedrich Robert Helmert Friedrich Robert Helmert was a German geodesist and an important writer on the theory of errors.Helmert was born in Freiberg, Kingdom of Saxony. After schooling in Freiberg and Dresden, he entered the Polytechnische Schule, now Technische Universität, in Dresden to study engineering science in 1859... |
6,378,200.0 | (close to WGS84!) | 298.30 |
| International 1924 | 6,378,388.0 | 6,356,911.9 | 297.0 |
| Krassowski 1940 SK-42 Reference System The SK-42 reference system also known as the Krasovsky ellipsoid, is a coordinate system established in the Soviet Union in 1942 as Systema koordinat , and provides parameters which are linked to the geocentric Cartesian coordinate system PZ-90... |
6,378,245.00 | (for Eastern Europe) | 298.3 |
| Internat. 1967 Luzern | 6,378,165.00 | (incl. Sat.Geodesy) | 298.25 |
| WGS 1984 | 6,378,137.00 | 6,356,752.3142 | 298.257223563 |
Mean Earth ellipsoid and reference ellipsoids
A data set which describes the global averageAverage
In mathematics, an average, or central tendency of a data set is a measure of the "middle" value of the data set. Average is one form of central tendency. Not all central tendencies should be considered definitions of average....
of the Earth's surface curvature is called the mean Earth Ellipsoid. It refers to a theoretical coherence between the geographic latitude and the meridional curvature of the geoid
Geoid
The geoid is that equipotential surface which would coincide exactly with the mean ocean surface of the Earth, if the oceans were in equilibrium, at rest , and extended through the continents . According to C.F...
. The latter is close to the mean sea level, and therefore an ideal Earth ellipsoid has the same volume
Volume
Volume is the quantity of three-dimensional space enclosed by some closed boundary, for example, the space that a substance or shape occupies or contains....
as the geoid.
While the mean Earth ellipsoid is the ideal basis of global geodesy, for regional
Régional
Régional Compagnie Aérienne Européenne, or Régional for short, is a subsidiary airline wholly owned by Air France which connects hubs at Paris, Lyon, Clermont-Ferrand, and Bordeaux to 49 airports in Europe. The airline operates in Air France livery, retaining its name in small titles and logo on...
networks a so called reference ellipsoid
Reference ellipsoid
In geodesy, a reference ellipsoid is a mathematically-defined surface that approximates the geoid, the truer figure of the Earth, or other planetary body....
may be the better choice. When geodetic measurements have to be computed on a mathematical reference surface, this surface should have a similar curvature as the regional geoid. Otherwise the reduction
Reduction (mathematics)
In mathematics, reduction refers to the rewriting of an expression into a simpler form. For example, the process of rewriting a fraction into one with the smallest whole-number denominator possible is called "reducing a fraction"...
of the measurements would get small distortions.
This is the reason for the "long life" of former reference ellipsoids like the Hayford
Hayford ellipsoid
The Hayford ellipsoid is a geodetic reference ellipsoid, named after the US geodesist John Fillmore Hayford , which was introduced in 1910. The Hayford ellipsoid was also referred to as the International ellipsoid 1924 after it had been adopted by the International Union of Geodesy and Geophysics...
or the Bessel ellipsoid
Bessel ellipsoid
The Bessel ellipsoid is an important reference ellipsoid of geodesy. It is currently used by several countries for their national geodetic surveys, in Europe and on other continents, but will be replaced in the next decades by modern ellipsoids of satellite geodesy.The Bessel ellipsoid was derived...
, despite of the fact that their main axes deviate by several hundred meters from the modern values. Another reason is a juridical one: the coordinates of millions of boundary stones should remain fixed for a long period. If their reference surface would change, the coordinates themselves would also change.
However, for international
International
----International mostly means something that involves more than one country. The term international as a word means involvement of, interaction between or encompassing more than one nation, or generally beyond national boundaries...
networks, GPS positioning or astronautics
Astronautics
Astronautics, and related astronautical engineering, is the theory and practice of navigation beyond the Earth's atmosphere. In other words, it is the science and technology of space flight....
, these regional reasons are less relevant. As the knowledge of Earth's figure
Figure of the Earth
The expression figure of the Earth has various meanings in geodesy according to the way it is used and the precision with which the Earth's size and shape is to be defined. The actual topographic surface is most apparent with its variety of land forms and water areas. This is, in fact, the surface...
is increasingly accurate, the International Geoscientific Union IUGG usually adopts the axes of the Earth ellipsoid to the best available data.
See also
- Reference ellipsoidReference ellipsoidIn geodesy, a reference ellipsoid is a mathematically-defined surface that approximates the geoid, the truer figure of the Earth, or other planetary body....
- History of geodesyHistory of geodesyGeodesy ,[1] also named geodetics, is the scientific discipline that deals with the measurement and representation of the Earth.Humanity has always been interested in the Earth...
- geodetic datum

