Euler–Mascheroni constant
Encyclopedia
| Binary Binary numeral system The binary numeral system, or base-2 number system, represents numeric values using two symbols, 0 and 1. More specifically, the usual base-2 system is a positional notation with a radix of 2... |
|
| Decimal Decimal The decimal numeral system has ten as its base. It is the numerical base most widely used by modern civilizations.... |
|
| Hexadecimal Hexadecimal In mathematics and computer science, hexadecimal is a positional numeral system with a radix, or base, of 16. It uses sixteen distinct symbols, most often the symbols 0–9 to represent values zero to nine, and A, B, C, D, E, F to represent values ten to fifteen... |
|
| Continued fraction Continued fraction In mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer part and another reciprocal, and so on... |
(This continued fraction is not known to be periodic Periodic function In mathematics, a periodic function is a function that repeats its values in regular intervals or periods. The most important examples are the trigonometric functions, which repeat over intervals of length 2π radians. Periodic functions are used throughout science to describe oscillations,... . Shown in linear notation) |
The Euler–Mascheroni constant (also called Euler's constant) is a mathematical constant
Mathematical constant
A mathematical constant is a special number, usually a real number, that is "significantly interesting in some way". Constants arise in many different areas of mathematics, with constants such as and occurring in such diverse contexts as geometry, number theory and calculus.What it means for a...
recurring in analysis
Mathematical analysis
Mathematical analysis, which mathematicians refer to simply as analysis, has its beginnings in the rigorous formulation of infinitesimal calculus. It is a branch of pure mathematics that includes the theories of differentiation, integration and measure, limits, infinite series, and analytic functions...
and number theory
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
, usually denoted by the lowercase Greek letter (gamma
Gamma
Gamma is the third letter of the Greek alphabet. In the system of Greek numerals it has a value of 3. It was derived from the Phoenician letter Gimel . Letters that arose from Gamma include the Roman C and G and the Cyrillic letters Ge Г and Ghe Ґ.-Greek:In Ancient Greek, gamma represented a...
).
It is defined as the limiting
Limit of a sequence
The limit of a sequence is, intuitively, the unique number or point L such that the terms of the sequence become arbitrarily close to L for "large" values of n...
difference between the harmonic series
Harmonic series (mathematics)
In mathematics, the harmonic series is the divergent infinite series:Its name derives from the concept of overtones, or harmonics in music: the wavelengths of the overtones of a vibrating string are 1/2, 1/3, 1/4, etc., of the string's fundamental wavelength...
and the natural logarithm
Natural logarithm
The natural logarithm is the logarithm to the base e, where e is an irrational and transcendental constant approximately equal to 2.718281828...
:
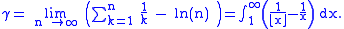
Here, ⌊x⌋ represents the floor function. The numerical value of this constant, to 50 decimal places, is
- .
should not be confused with the base of the natural logarithmNatural logarithm
The natural logarithm is the logarithm to the base e, where e is an irrational and transcendental constant approximately equal to 2.718281828...
, e
E (mathematical constant)
The mathematical constant ' is the unique real number such that the value of the derivative of the function at the point is equal to 1. The function so defined is called the exponential function, and its inverse is the natural logarithm, or logarithm to base...
, which is sometimes called Euler's number.
History
The constant first appeared in a 1734 paper by the Swiss mathematician Leonhard EulerLeonhard Euler
Leonhard Euler was a pioneering Swiss mathematician and physicist. He made important discoveries in fields as diverse as infinitesimal calculus and graph theory. He also introduced much of the modern mathematical terminology and notation, particularly for mathematical analysis, such as the notion...
, titled De Progressionibus harmonicis observationes (Eneström Index 43). Euler used the notations C and O for the constant. In 1790, Italian
Italy
Italy , officially the Italian Republic languages]] under the European Charter for Regional or Minority Languages. In each of these, Italy's official name is as follows:;;;;;;;;), is a unitary parliamentary republic in South-Central Europe. To the north it borders France, Switzerland, Austria and...
mathematician Lorenzo Mascheroni
Lorenzo Mascheroni
Lorenzo Mascheroni was an Italian mathematician.He was born near Bergamo, Lombardy. At first mainly interested in the humanities , he eventually became professor of mathematics at Pavia....
used the notations A and a for the constant. The notation γ appears nowhere in the writings of either Euler or Mascheroni, and was chosen at a later time because of the constant's connection to the gamma function
Gamma function
In mathematics, the gamma function is an extension of the factorial function, with its argument shifted down by 1, to real and complex numbers...
. For example, the German mathematician Carl Anton Bretschneider
Carl Anton Bretschneider
Carl Anton Bretschneider was a mathematician from Gotha, Germany. Bretschneider worked in geometry, number theory, and history of geometry. He also worked on logarithmic integrals and mathematical tables. He was one of the first mathematicians to use the symbol γ for Euler's constant when he...
used the notation γ in 1835 and Augustus De Morgan
Augustus De Morgan
Augustus De Morgan was a British mathematician and logician. He formulated De Morgan's laws and introduced the term mathematical induction, making its idea rigorous. The crater De Morgan on the Moon is named after him....
used it in a textbook published in parts from 1836 to 1842.
Appearances
The Euler–Mascheroni constant appears, among other places, in the following ('*' means that this entry contains an explicit equation):- Expressions involving the exponential integralExponential integralIn mathematics, the exponential integral is a special function defined on the complex plane given the symbol Ei.-Definitions:For real, nonzero values of x, the exponential integral Ei can be defined as...
* - The Laplace transform of the natural logarithmNatural logarithmThe natural logarithm is the logarithm to the base e, where e is an irrational and transcendental constant approximately equal to 2.718281828...
- The first term of the Taylor seriesTaylor seriesIn mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
expansion for the Riemann zeta function*, where it is the first of the Stieltjes constants* - Calculations of the digamma function
- A product formula for the gamma functionGamma functionIn mathematics, the gamma function is an extension of the factorial function, with its argument shifted down by 1, to real and complex numbers...
- An inequality for Euler's totient functionEuler's totient functionIn number theory, the totient \varphi of a positive integer n is defined to be the number of positive integers less than or equal to n that are coprime to n In number theory, the totient \varphi(n) of a positive integer n is defined to be the number of positive integers less than or equal to n that...
- The growth rate of the divisor functionDivisor functionIn mathematics, and specifically in number theory, a divisor function is an arithmetical function related to the divisors of an integer. When referred to as the divisor function, it counts the number of divisors of an integer. It appears in a number of remarkable identities, including relationships...
- The calculation of the Meissel–Mertens constant
- The third of Mertens' theoremsMertens' theoremsIn number theory, Mertens' theorems are three 1874 results related to the density of prime numbers proved by Franz Mertens...
* - Solution of the second kind to Bessel's equation
- In Dimensional regularizationDimensional regularizationIn theoretical physics, dimensional regularization is a method introduced by Giambiagi and Bollini for regularizing integrals in the evaluation of Feynman diagrams; in other words, assigning values to them that are meromorphic functions of an auxiliary complex parameter d, called the...
of Feynman diagramFeynman diagramFeynman diagrams are a pictorial representation scheme for the mathematical expressions governing the behavior of subatomic particles, first developed by the Nobel Prize-winning American physicist Richard Feynman, and first introduced in 1948...
s in Quantum Field TheoryQuantum field theoryQuantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and... - The meanMeanIn statistics, mean has two related meanings:* the arithmetic mean .* the expected value of a random variable, which is also called the population mean....
of the Gumbel distribution - The information entropyInformation entropyIn information theory, entropy is a measure of the uncertainty associated with a random variable. In this context, the term usually refers to the Shannon entropy, which quantifies the expected value of the information contained in a message, usually in units such as bits...
of the Weibull and Lévy distributions, and, implicitly, of the Chi-squared distribution for one or two degrees of freedom. - The answer to the Coupon collector's problemCoupon collector's problemIn probability theory, the coupon collector's problem describes the "collect all coupons and win" contests. It asks the following question: Suppose that there are n coupons, from which coupons are being collected with replacement...
* - A definition of the cosine integral*
For more information of this nature, see Gourdon and Sebah (2004).
Properties
The number γ has not been proved algebraicAlgebraic number
In mathematics, an algebraic number is a number that is a root of a non-zero polynomial in one variable with rational coefficients. Numbers such as π that are not algebraic are said to be transcendental; almost all real numbers are transcendental...
or transcendental
Transcendental number
In mathematics, a transcendental number is a number that is not algebraic—that is, it is not a root of a non-constant polynomial equation with rational coefficients. The most prominent examples of transcendental numbers are π and e...
. In fact, it is not even known whether γ is irrational
Irrational number
In mathematics, an irrational number is any real number that cannot be expressed as a ratio a/b, where a and b are integers, with b non-zero, and is therefore not a rational number....
. Continued fraction
Continued fraction
In mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer part and another reciprocal, and so on...
analysis reveals that if γ is rational
Rational number
In mathematics, a rational number is any number that can be expressed as the quotient or fraction a/b of two integers, with the denominator b not equal to zero. Since b may be equal to 1, every integer is a rational number...
, its denominator must be greater than 10242080. The ubiquity of γ revealed by the large number of equations below makes the irrationality of γ a major open question in mathematics. Also see Sondow (2003a).
For more equations of the sort shown below, see Gourdon and Sebah (2002).
Relation to gamma function
γ is related to the digamma function Ψ, and hence the derivative of the gamma functionGamma function
In mathematics, the gamma function is an extension of the factorial function, with its argument shifted down by 1, to real and complex numbers...
Γ, when both functions are evaluated at 1. Thus:
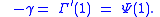
This is equal to the limits:
Further limit results are (Krämer, 2005):
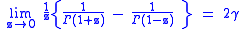
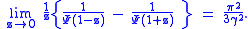
A limit related to the Beta function (expressed in terms of gamma function
Gamma function
In mathematics, the gamma function is an extension of the factorial function, with its argument shifted down by 1, to real and complex numbers...
s) is
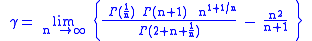
Relation to the zeta function
γ can also be expressed as an infinite sumSeries (mathematics)
A series is the sum of the terms of a sequence. Finite sequences and series have defined first and last terms, whereas infinite sequences and series continue indefinitely....
whose terms involve the Riemann zeta function evaluated at positive integers:
Other series related to the zeta function include:
The error term in the last equation is a rapidly decreasing function of n. As a result, the formula is well-suited for efficient computation of the constant to high precision.
Other interesting limits equaling the Euler–Mascheroni constant are the antisymmetric limit (Sondow, 1998)
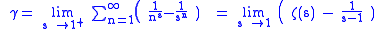
and
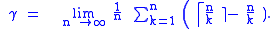
Closely related to this is the rational zeta series
Rational zeta series
In mathematics, a rational zeta series is the representation of an arbitrary real number in terms of a series consisting of rational numbers and the Riemann zeta function or the Hurwitz zeta function...
expression. By peeling off the first few terms of the series above, one obtains an estimate for the classical series limit:
where ζ(s,k) is the Hurwitz zeta function. The sum in this equation involves the harmonic numbers, Hn. Expanding some of the terms in the Hurwitz zeta function gives:
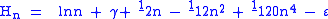, where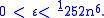 
Integrals
γ equals the value of a number of definite integralIntegral
Integration is an important concept in mathematics and, together with its inverse, differentiation, is one of the two main operations in calculus...
s:
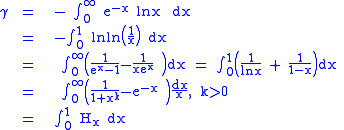
where
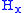is the fractional Harmonic number.Definite integrals in which γ appears include:
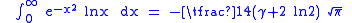
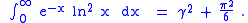
One can express γ using a special case of Hadjicostas's formula
Hadjicostas's formula
In mathematics, Hadjicostas's formula is a formula relating a certain double integral to values of the Gamma function and the Riemann zeta function.-Statement:Let s be a complex number with Re > −2...
as a double integral (Sondow 2003a, 2005) with equivalent series:
-
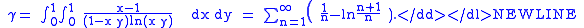
An interesting comparison by J. Sondow (2005) is the double integral and alternating series
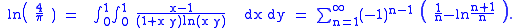
It shows that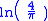
may be thought of as an "alternating Euler constant".
The two constants are also related by the pair of series (see Sondow 2005 #2)
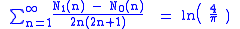
where N1(n) and N0(n) are the number of 1's and 0's, respectively, in the base 2 expansion of n.
We have also Catalan's 1875 integral (see Sondow and Zudilin)
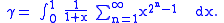
Series expansions
Euler showed that the following infinite series approaches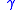
:
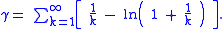
The series for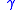
is equivalent to series Nielsen found in 1897:
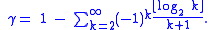
In 1910, Vacca found the closely related series:
where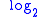
is the logarithmLogarithmThe logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: More generally, if x = by, then y is the logarithm of x to base b, and is written...
to the base 2 and
is the floor functionFloor functionIn mathematics and computer science, the floor and ceiling functions map a real number to the largest previous or the smallest following integer, respectively...
.
In 1926 he found a second series:
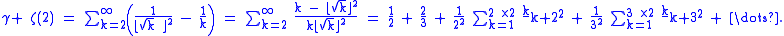
From the Kummer-expansion of the gamma function we get: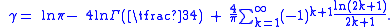
Series of prime numbers:
Asymptotic expansions
γ equals the following asymptotic formulas (where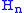
is the nth harmonic number.)
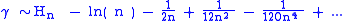
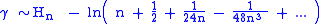
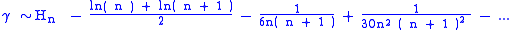
The third formula is also called the Ramanujan expansion.
Relations with the reciprocal logarithm
The reciprocal logarithm function (Krämer, 2005)
has a deep connection with Euler's constant and was studied by James Gregory in connection with numerical integrationNumerical integrationIn numerical analysis, numerical integration constitutes a broad family of algorithms for calculating the numerical value of a definite integral, and by extension, the term is also sometimes used to describe the numerical solution of differential equations. This article focuses on calculation of...
. The coefficients
are called Gregory coefficients; the first six were given in a letter to John CollinsJohn Collins (mathematician)John Collins was an English mathematician. He is most known for his extensive correspondence with leading scientists and mathematicians such as Giovanni Alfonso Borelli, Gottfried Leibniz, Isaac Newton, and John Wallis...
in 1670. From the recursionRecursionRecursion is the process of repeating items in a self-similar way. For instance, when the surfaces of two mirrors are exactly parallel with each other the nested images that occur are a form of infinite recursion. The term has a variety of meanings specific to a variety of disciplines ranging from...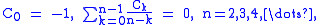
we get the tablen 1 2 3 4 5 6 7 8 9 10 OEIS sequence Cn 
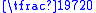
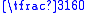
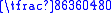
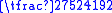
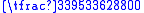
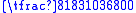
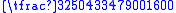
(numerators), (demominators)
Gregory coefficients are similar to Bernoulli numbers and satisfy the asymptotic relation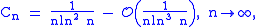
and the integral representation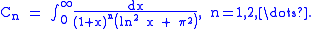
Euler's constant has the integral representations
A very important expansion of Gregorio FontanaGregorio FontanaGregorio Fontana was an Italian mathematician. He was chair of mathematics at the university of Pavia succeeding Roger Joseph Boscovich. He has been credited with the introduction of polar coordinates....
(1780) is: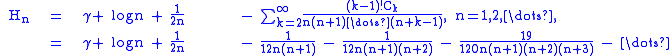
which is convergent for all n.
Weighted sums of the Gregory coefficients give different constants:
eγ
The constant eγ is important in number theory. Some authors denote this quantity simply as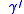
. eγ equals the following limitLimit of a sequenceThe limit of a sequence is, intuitively, the unique number or point L such that the terms of the sequence become arbitrarily close to L for "large" values of n...
, where pn is the n-th prime numberPrime numberA prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
:
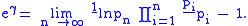
This restates the third of Mertens' theoremsMertens' theoremsIn number theory, Mertens' theorems are three 1874 results related to the density of prime numbers proved by Franz Mertens...
. The numerical value of eγ is:- 1.78107241799019798523650410310717954916964521430343 … .
Other infinite products relating to eγ include:
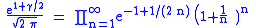
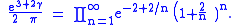
These products result from the Barnes G-functionBarnes G-functionIn mathematics, the Barnes G-function G is a function that is an extension of superfactorials to the complex numbers. It is related to the Gamma function, the K-function and the Glaisher-Kinkelin constant, and was named after mathematician Ernest William Barnes...
.
We also have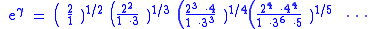
where the nth factor is the (n+1)st root of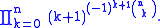
This infinite product, first discovered by Ser in 1926, was rediscovered by Sondow (2003) using hypergeometric functions.
Continued fraction
The continued fractionContinued fractionIn mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer part and another reciprocal, and so on...
expansion of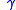
is of the form [0; 1, 1, 2, 1, 2, 1, 4, 3, 13, 5, 1, 1, 8, 1, 2, 4, 1, 1, 40, ...] , and has at least 470,000 terms.
Generalizations
Euler's generalized constants are given by
for 0 < α < 1, with γ as the special case α = 1. This can be further generalized to
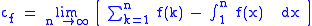
for some arbitrary decreasing function f. For example,
gives rise to the Stieltjes constants, and
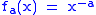
gives
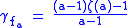
where again the limit
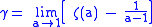
appears.
A two-dimensional limit generalization is the Masser–Gramain constant.
Published digits
Euler initially calculated the constant's value to 6 decimal places. In 1781, he calculated it to 16 decimal places. Mascheroni attempted to calculate the constant to 32 decimal places, but made errors in the 20th-22nd decimal places; starting from the 20th digit, he calculated ...1811209008239 when the correct value is ...0651209008240.
Published Decimal Expansions of γ Date Decimal digits Author 1734 5 Leonhard Euler Leonhard EulerLeonhard Euler was a pioneering Swiss mathematician and physicist. He made important discoveries in fields as diverse as infinitesimal calculus and graph theory. He also introduced much of the modern mathematical terminology and notation, particularly for mathematical analysis, such as the notion...1735 15 Leonhard Euler 1790 19 Lorenzo Mascheroni Lorenzo MascheroniLorenzo Mascheroni was an Italian mathematician.He was born near Bergamo, Lombardy. At first mainly interested in the humanities , he eventually became professor of mathematics at Pavia....1809 22 Johann G. von Soldner Johann Georg von SoldnerJohann Georg von Soldner was a German physicist, mathematician and astronomer, first in Berlin and later in 1808 in Munich.-Life:...1811 22 Carl Friedrich Gauss Carl Friedrich GaussJohann Carl Friedrich Gauss was a German mathematician and scientist who contributed significantly to many fields, including number theory, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy and optics.Sometimes referred to as the Princeps mathematicorum...1812 40 Friedrich Bernhard Gottfried Nicolai Friedrich Bernhard Gottfried NicolaiFriedrich Bernhard Gottfried Nicolai was a German astronomer.Born in Braunschweig, Nicolai was educated at Göttingen. In 1812, he calculated the Euler–Mascheroni constant to 40 decimal places. In 1816, he joined the Mannheim observatory where he became the director.The crater Nicolai on the Moon...1857 34 Christian Fredrik Lindman 1861 41 Ludwig Oettinger 1867 49 William Shanks William ShanksWilliam Shanks was a British amateur mathematician.Shanks is famous for his calculation of π to 707 places, accomplished in 1873, which, however, was only correct up to the first 527 places. This error was highlighted in 1944 by D. F...1871 99 James W.L. Glaisher James Whitbread Lee GlaisherJames Whitbread Lee Glaisher son of James Glaisher, the meteorologist, was a prolific English mathematician.He was educated at St Paul's School and Trinity College, Cambridge, where he was second wrangler in 1871...1871 101 William Shanks 1877 262 J. C. Adams John Couch AdamsJohn Couch Adams was a British mathematician and astronomer. Adams was born in Laneast, near Launceston, Cornwall, and died in Cambridge. The Cornish name Couch is pronounced "cooch"....1952 328 John William Wrench, Jr. John WrenchJohn William Wrench, Jr. was an American mathematician who worked primarily in numerical analysis. He was a pioneer in using computers for mathematical calculations, and is noted for work done with Daniel Shanks to calculate the mathematical constant pi to 100,000 decimal places.-Life and...1961 1050 Helmut Fischer and Karl Zeller 1962 1,271 Donald Knuth Donald KnuthDonald Ervin Knuth is a computer scientist and Professor Emeritus at Stanford University.He is the author of the seminal multi-volume work The Art of Computer Programming. Knuth has been called the "father" of the analysis of algorithms...1962 3,566 Dura W. Sweeney 1973 4,879 William A. Beyer and Michael S. Waterman 1977 20,700 Richard P. Brent Richard Brent (scientist)Richard Peirce Brent is an Australian mathematician and computer scientist, born in 1946. He holds the position of Distinguished Professor of Mathematics and Computer Science with a joint appointment in the Mathematical Sciences Institute and the College of Engineering and Computer Science at...1980 30,100 Richard P. Brent & Edwin M. McMillan Edwin McMillanEdwin Mattison McMillan was an American physicist and Nobel laureate credited with being the first ever to produce a transuranium element. He shared the Nobel Prize in Chemistry with Glenn Seaborg in 1951....1993 172,000 Jonathan Borwein Jonathan BorweinJonathan Michael Borwein is a Scottish mathematician who holds an appointment as Laureate Professor of mathematics at the University of Newcastle, Australia. Noted for his prolific and creative work throughout the international mathematical community, he is a close associate of David H...2009 29,844,489,545 Alexander J. Yee & Raymond Chan
External links
- Krämer, Stefan "Euler's Constant γ=0.577... Its Mathematics and History."
- Jonathan Sondow.