Formal scheme
Encyclopedia
In mathematics
, specifically in algebraic geometry
, a formal scheme is a type of space which includes data about its surroundings. Unlike an ordinary scheme
, a formal scheme includes infinitesimal data that, in effect, points in a direction off of the scheme. For this reason, formal schemes frequently appear in topics such as deformation theory
.
case. While there have been several definitions of non-Noetherian formal schemes, these encounter technical problems. Consequently we will make the assumption that all rings are Noetherian.
All rings will be assumed to be commutative
and with unit. Let A be a (Noetherian) topological ring
, that is, a ring A which is a topological space
such that the operations of addition and multiplication are continuous. A is linearly topologized if zero has a base
consisting of ideal
s. An ideal of definition
for a linearly topologized ring is an open ideal such that for every open neighborhood V of 0, there exists a positive integer n such that 
. A linearly topologized ring is preadmissible if it admits an ideal of definition, and it is admissible if it is also complete
. (In the terminology of Bourbaki, this is "complete and separated".)
Assume that A is admissible, and let
be an ideal of definition. A prime ideal is open if and only if it contains 
. The set of open prime ideals of A, or equivalently the set of prime ideals of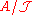 
, is the underlying topological space of the formal spectrum of A, denoted Spf A. Spf A has a structure sheaf which is defined using the structure sheaf of the spectrum of a ring
. Let
be a neighborhood basis for zero consisting of ideals of definition. All the spectra of 
have the same underlying topological space but a different structure sheaf. The structure sheaf of Spf A is the projective limit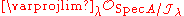 
.
It can be shown that if f ∈ A and Df is the set of all open prime ideals of A not containing f, then
, where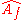 
is the completion of the localization
Af.
Finally, a Noetherian formal scheme is a topologically ringed space
(that is, a ringed space
whose sheaf of rings is a sheaf of topological rings) such that each point of
admits an open neighborhood isomorphic (as topologically ringed spaces) to the formal spectrum of a noetherian ring.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, specifically in algebraic geometry
Algebraic geometry
Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, a formal scheme is a type of space which includes data about its surroundings. Unlike an ordinary scheme
Scheme (mathematics)
In mathematics, a scheme is an important concept connecting the fields of algebraic geometry, commutative algebra and number theory. Schemes were introduced by Alexander Grothendieck so as to broaden the notion of algebraic variety; some consider schemes to be the basic object of study of modern...
, a formal scheme includes infinitesimal data that, in effect, points in a direction off of the scheme. For this reason, formal schemes frequently appear in topics such as deformation theory
Deformation theory
In mathematics, deformation theory is the study of infinitesimal conditions associated with varying a solution P of a problem to slightly different solutions Pε, where ε is a small number, or vector of small quantities. The infinitesimal conditions are therefore the result of applying the approach...
.
Definition
Formal schemes are usually defined only in the NoetherianNoetherian ring
In mathematics, more specifically in the area of modern algebra known as ring theory, a Noetherian ring, named after Emmy Noether, is a ring in which every non-empty set of ideals has a maximal element...
case. While there have been several definitions of non-Noetherian formal schemes, these encounter technical problems. Consequently we will make the assumption that all rings are Noetherian.
All rings will be assumed to be commutative
Commutative ring
In ring theory, a branch of abstract algebra, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra....
and with unit. Let A be a (Noetherian) topological ring
Topological ring
In mathematics, a topological ring is a ring R which is also a topological space such that both the addition and the multiplication are continuous as mapswhere R × R carries the product topology.- General comments :...
, that is, a ring A which is a topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
such that the operations of addition and multiplication are continuous. A is linearly topologized if zero has a base
Base (topology)
In mathematics, a base B for a topological space X with topology T is a collection of open sets in T such that every open set in T can be written as a union of elements of B. We say that the base generates the topology T...
consisting of ideal
Ideal (ring theory)
In ring theory, a branch of abstract algebra, an ideal is a special subset of a ring. The ideal concept allows the generalization in an appropriate way of some important properties of integers like "even number" or "multiple of 3"....
s. An ideal of definition
for a linearly topologized ring is an open ideal such that for every open neighborhood V of 0, there exists a positive integer n such that . A linearly topologized ring is preadmissible if it admits an ideal of definition, and it is admissible if it is also completeCompletion (ring theory)
In abstract algebra, a completion is any of several related functors on rings and modules that result in complete topological rings and modules. Completion is similar to localization, and together they are among the most basic tools in analysing commutative rings. Complete commutative rings have...
. (In the terminology of Bourbaki, this is "complete and separated".)
Assume that A is admissible, and let
be an ideal of definition. A prime ideal is open if and only if it contains . The set of open prime ideals of A, or equivalently the set of prime ideals of 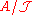, is the underlying topological space of the formal spectrum of A, denoted Spf A. Spf A has a structure sheaf which is defined using the structure sheaf of the spectrum of a ringSpectrum of a ring
In abstract algebra and algebraic geometry, the spectrum of a commutative ring R, denoted by Spec, is the set of all proper prime ideals of R...
. Let
be a neighborhood basis for zero consisting of ideals of definition. All the spectra of have the same underlying topological space but a different structure sheaf. The structure sheaf of Spf A is the projective limit 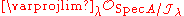.It can be shown that if f ∈ A and Df is the set of all open prime ideals of A not containing f, then
, where 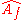is the completion of the localizationLocalization of a ring
In abstract algebra, localization is a systematic method of adding multiplicative inverses to a ring. Given a ring R and a subset S, one wants to construct some ring R* and ring homomorphism from R to R*, such that the image of S consists of units in R*...
Af.
Finally, a Noetherian formal scheme is a topologically ringed space
(that is, a ringed spaceRinged space
In mathematics, a ringed space is, intuitively speaking, a space together with a collection of commutative rings, the elements of which are "functions" on each open set of the space...
whose sheaf of rings is a sheaf of topological rings) such that each point of
admits an open neighborhood isomorphic (as topologically ringed spaces) to the formal spectrum of a noetherian ring.