Frieze group
Encyclopedia
A frieze group is a mathematical concept to classify designs on two-dimensional surfaces which are repetitive in one direction, based on the symmetries
in the pattern. Such patterns occur frequently in architecture
and decorative art
. The mathematical study of such patterns reveals that exactly 7 different types of patterns can occur.
Frieze groups are two-dimensional line groups
, from having only one direction of repeat, and they are related to the more complex wallpaper groups
, which classify patterns that are repetitive in two directions.
As with wallpaper groups, a frieze group is often visualised by a simple periodic pattern in the category concerned.
s for patterns on a strip (infinitely wide rectangle), hence a class of groups
of isometries
of the plane, or of a strip. There are seven different frieze groups. The actual symmetry groups within a frieze group are characterized by the smallest translation distance, and, for the frieze groups 4-7, by a shifting parameter. In the case of symmetry groups in the plane, additional parameters are the direction of the translation vector, and, for the frieze groups 2, 3, 5, 6, and 7, the positioning perpendicular to the translation vector. Thus there are two degrees of freedom
for group 1, three for groups 2, 3, and 4, and four for groups 5, 6, and 7. Many authors present the frieze groups in a different order.
A symmetry group of a frieze group necessarily contains translation
s and may contain glide reflection
s. Other possible group elements are reflection
s along the long axis of the strip, reflections along the narrow axis of the strip and 180° rotation
s. For two of the seven frieze groups (numbers 1 and 2 below) the symmetry groups are singly generated
, for four (numbers 3–6) they have a pair of generators, and for number 7 the symmetry groups require three generators.
A symmetry group in frieze group 1, 3, 4, or 5 is a subgroup
of a symmetry group in the last frieze group with the same translational distance. A symmetry group in frieze group 2 or 6 is a subgroup of a symmetry group in the last frieze group with half the translational distance. This last frieze group contains the symmetry groups of the simplest periodic patterns in the strip (or the plane), a row of dots. Any transformation of the plane leaving this pattern invariant can be decomposed into a translation, (x,y) → (n+x,y), optionally followed by a reflection in either the horizontal axis, (x,y) → (x,−y), or the vertical axis, (x,y) → (−x,y), provided that this axis is chosen through or midway between two dots, or a rotation by 180°, (x,y) → (−x,−y) (ditto). Therefore, in a way, this frieze group contains the "largest" symmetry groups, which consist of all such transformations.
The inclusion of the discrete condition is to exclude the group containing all translations, and groups containing arbitrarily small translations (e.g. the group of horizontal translations by rational distances). Even apart from scaling and shifting, there are infinitely many cases, e.g. by considering rational numbers of which the denominators are powers of a given prime number.
The inclusion of the infinite condition is to exclude groups that have no translations:
or IUC notation, orbifold notation, Coxeter notation
, and Schönflies notation:
Summarized:
As we have seen, up to isomorphism
, there are four groups, two abelian
, and two non-abelian.
The groups can be classified by their type of two-dimensional grid or lattice:
The lattice being oblique means that the second direction need not be orthogonal to the direction of repeat. The groups' order in this table is their order in the International Tables for Crystallography, which differs from orders given elsewhere.
Symmetry
Symmetry generally conveys two primary meanings. The first is an imprecise sense of harmonious or aesthetically pleasing proportionality and balance; such that it reflects beauty or perfection...
in the pattern. Such patterns occur frequently in architecture
Architecture
Architecture is both the process and product of planning, designing and construction. Architectural works, in the material form of buildings, are often perceived as cultural and political symbols and as works of art...
and decorative art
Decorative art
The decorative arts is traditionally a term for the design and manufacture of functional objects. It includes interior design, but not usually architecture. The decorative arts are often categorized in opposition to the "fine arts", namely, painting, drawing, photography, and large-scale...
. The mathematical study of such patterns reveals that exactly 7 different types of patterns can occur.
Frieze groups are two-dimensional line groups
Line group
A line group is a mathematical way of describing symmetries associated with moving along a line. These symmetries include repeating along that line, making that line a one-dimensional lattice...
, from having only one direction of repeat, and they are related to the more complex wallpaper groups
Wallpaper group
A wallpaper group is a mathematical classification of a two-dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art...
, which classify patterns that are repetitive in two directions.
As with wallpaper groups, a frieze group is often visualised by a simple periodic pattern in the category concerned.
General
Formally, a frieze group is a class of infinite discrete symmetry groupSymmetry group
The symmetry group of an object is the group of all isometries under which it is invariant with composition as the operation...
s for patterns on a strip (infinitely wide rectangle), hence a class of groups
Group (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
of isometries
Isometry
In mathematics, an isometry is a distance-preserving map between metric spaces. Geometric figures which can be related by an isometry are called congruent.Isometries are often used in constructions where one space is embedded in another space...
of the plane, or of a strip. There are seven different frieze groups. The actual symmetry groups within a frieze group are characterized by the smallest translation distance, and, for the frieze groups 4-7, by a shifting parameter. In the case of symmetry groups in the plane, additional parameters are the direction of the translation vector, and, for the frieze groups 2, 3, 5, 6, and 7, the positioning perpendicular to the translation vector. Thus there are two degrees of freedom
Degrees of freedom (physics and chemistry)
A degree of freedom is an independent physical parameter, often called a dimension, in the formal description of the state of a physical system...
for group 1, three for groups 2, 3, and 4, and four for groups 5, 6, and 7. Many authors present the frieze groups in a different order.
A symmetry group of a frieze group necessarily contains translation
Translation (geometry)
In Euclidean geometry, a translation moves every point a constant distance in a specified direction. A translation can be described as a rigid motion, other rigid motions include rotations and reflections. A translation can also be interpreted as the addition of a constant vector to every point, or...
s and may contain glide reflection
Glide reflection
In geometry, a glide reflection is a type of isometry of the Euclidean plane: the combination of a reflection in a line and a translation along that line. Reversing the order of combining gives the same result...
s. Other possible group elements are reflection
Reflection (mathematics)
In mathematics, a reflection is a mapping from a Euclidean space to itself that is an isometry with a hyperplane as set of fixed points; this set is called the axis or plane of reflection. The image of a figure by a reflection is its mirror image in the axis or plane of reflection...
s along the long axis of the strip, reflections along the narrow axis of the strip and 180° rotation
Rotation
A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...
s. For two of the seven frieze groups (numbers 1 and 2 below) the symmetry groups are singly generated
Generating set of a group
In abstract algebra, a generating set of a group is a subset that is not contained in any proper subgroup of the group. Equivalently, a generating set of a group is a subset such that every element of the group can be expressed as the combination of finitely many elements of the subset and their...
, for four (numbers 3–6) they have a pair of generators, and for number 7 the symmetry groups require three generators.
A symmetry group in frieze group 1, 3, 4, or 5 is a subgroup
Subgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
of a symmetry group in the last frieze group with the same translational distance. A symmetry group in frieze group 2 or 6 is a subgroup of a symmetry group in the last frieze group with half the translational distance. This last frieze group contains the symmetry groups of the simplest periodic patterns in the strip (or the plane), a row of dots. Any transformation of the plane leaving this pattern invariant can be decomposed into a translation, (x,y) → (n+x,y), optionally followed by a reflection in either the horizontal axis, (x,y) → (x,−y), or the vertical axis, (x,y) → (−x,y), provided that this axis is chosen through or midway between two dots, or a rotation by 180°, (x,y) → (−x,−y) (ditto). Therefore, in a way, this frieze group contains the "largest" symmetry groups, which consist of all such transformations.
The inclusion of the discrete condition is to exclude the group containing all translations, and groups containing arbitrarily small translations (e.g. the group of horizontal translations by rational distances). Even apart from scaling and shifting, there are infinitely many cases, e.g. by considering rational numbers of which the denominators are powers of a given prime number.
The inclusion of the infinite condition is to exclude groups that have no translations:
- the group with the identity only (isomorphic to C1, the trivial groupTrivial groupIn mathematics, a trivial group is a group consisting of a single element. All such groups are isomorphic so one often speaks of the trivial group. The single element of the trivial group is the identity element so it usually denoted as such, 0, 1 or e depending on the context...
of order 1). - the group consisting of the identity and reflection in the horizontal axis (isomorphic to C2, the cyclic groupCyclic groupIn group theory, a cyclic group is a group that can be generated by a single element, in the sense that the group has an element g such that, when written multiplicatively, every element of the group is a power of g .-Definition:A group G is called cyclic if there exists an element g...
of order 2). - the groups each consisting of the identity and reflection in a vertical axis (ditto)
- the groups each consisting of the identity and 180° rotation about a point on the horizontal axis (ditto)
- the groups each consisting of the identity, reflection in a vertical axis, reflection in the horizontal axis, and 180° rotation about the point of intersection (isomorphic to the Klein four-groupKlein four-groupIn mathematics, the Klein four-group is the group Z2 × Z2, the direct product of two copies of the cyclic group of order 2...
)
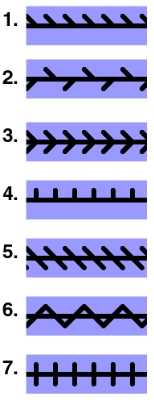
Descriptions of the seven frieze groups
There are seven distinct subgroups (up to scaling and shifting of patterns) in the discrete frieze group generated by a translation, reflection (along the same axis) and a 180° rotation. Each of these subgroups is the symmetry group of a frieze pattern, and sample patterns are shown in Fig. 1. The seven different groups correspond to the 7 infinite series of axial point groups in three dimensions, with n = ∞. They are identified using Hermann-Mauguin notationHermann-Mauguin notation
Hermann–Mauguin notation is used to represent the symmetry elements in point groups, plane groups and space groups. It is named after the German crystallographer Carl Hermann and the French mineralogist Charles-Victor Mauguin...
or IUC notation, orbifold notation, Coxeter notation
Coxeter notation
In geometry, Coxeter notation is a system of classifying symmetry groups, describing the angles between with fundamental reflections of a Coxeter group. It uses a bracketed notation, with modifiers to indicate certain subgroups. The notation is named after H. S. M...
, and Schönflies notation:
Summarized:
- p1: T (translationTranslation (geometry)In Euclidean geometry, a translation moves every point a constant distance in a specified direction. A translation can be described as a rigid motion, other rigid motions include rotations and reflections. A translation can also be interpreted as the addition of a constant vector to every point, or...
only, in the horizontal direction) - p11g: TG (translation and glide reflectionGlide reflectionIn geometry, a glide reflection is a type of isometry of the Euclidean plane: the combination of a reflection in a line and a translation along that line. Reversing the order of combining gives the same result...
) - p11m: THG (translation, horizontal line reflectionReflection (mathematics)In mathematics, a reflection is a mapping from a Euclidean space to itself that is an isometry with a hyperplane as set of fixed points; this set is called the axis or plane of reflection. The image of a figure by a reflection is its mirror image in the axis or plane of reflection...
, and glide reflection) - p2m1: TV (translation and vertical line reflection)
- p2: TR (translation and 180° rotationRotationA rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...
) - p2mg: TRVG (translation, 180° rotation, vertical line reflection, and glide reflection)
- p2mm: TRHVG (translation, 180° rotation, horizontal line reflection, vertical line reflection, and glide reflection)
As we have seen, up to isomorphism
Group isomorphism
In abstract algebra, a group isomorphism is a function between two groups that sets up a one-to-one correspondence between the elements of the groups in a way that respects the given group operations. If there exists an isomorphism between two groups, then the groups are called isomorphic...
, there are four groups, two abelian
Abelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
, and two non-abelian.
The groups can be classified by their type of two-dimensional grid or lattice:
| Lattice type | # | Groups |
|---|---|---|
| Oblique | 1-2 | p1, p211 |
| Rectangular | 3-7 | p1m1, p11m, p11g, p2mm, p2mg |
The lattice being oblique means that the second direction need not be orthogonal to the direction of repeat. The groups' order in this table is their order in the International Tables for Crystallography, which differs from orders given elsewhere.
See also
- Symmetry groups in one dimensionSymmetry groups in one dimensionA one-dimensional symmetry group is a mathematical group that describes symmetries in one dimension .A pattern in 1D can be represented as a function f for, say, the color at position x....
- Line groupLine groupA line group is a mathematical way of describing symmetries associated with moving along a line. These symmetries include repeating along that line, making that line a one-dimensional lattice...
- Rod groupRod groupIn mathematics, a rod group is a three-dimensional line group whose point group is one of the axial crystallographic point groups. This constraint means that the point group must be the symmetry of some three-dimensional lattice....
- Wallpaper groupWallpaper groupA wallpaper group is a mathematical classification of a two-dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art...
- Space groupSpace groupIn mathematics and geometry, a space group is a symmetry group, usually for three dimensions, that divides space into discrete repeatable domains.In three dimensions, there are 219 unique types, or counted as 230 if chiral copies are considered distinct...
Web demo and software
There exist software graphic tools that will let you create 2D patterns using frieze groups. Usually, you can edit the original strip and its copies in the entire pattern are updated automatically.- Kali, a free and open source softwareFree and open source softwareFree and open-source software or free/libre/open-source software is software that is liberally licensed to grant users the right to use, study, change, and improve its design through the availability of its source code...
application for wallpaper, frieze and other patterns. - Kali, free downloadable Kali for Windows and Mac Classic.
- Tess, a nagware tessellation program for multiple platforms, supports all wallpaper, frieze, and rosette groups, as well as Heesch tilings.
- FriezingWorkz, a freeware Hypercard stack for the Classic Mac platform that supports all frieze groups.
External links
- Frieze Patterns at cut-the-knotCut-the-knotCut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...
- Illuminations: Frieze Patterns

