
Irreducible element
Encyclopedia
In abstract algebra
, a non-zero non-unit
element in an integral domain is said to be irreducible if it is not a product of two non-units.
Irreducible elements should not be confused with prime element
s. (A non-unit element in a commutative ring
in a commutative ring
 is called prime if whenever
is called prime if whenever 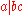 for some
for some  and
and  in
in  , then
, then 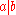 or
or 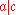 .) Every prime element is irreducible, but the converse is not true in general. The converse is true for UFD
.) Every prime element is irreducible, but the converse is not true in general. The converse is true for UFD
s (or, more generally, GCD domain
s.)
Moreover, while an ideal generated by a prime element is a prime ideal
, it is not true in general that an ideal generated by an irreducible element is an irreducible ideal
. However, if is a GCD domain, and
is a GCD domain, and  is an irreducible element of
is an irreducible element of  , then the ideal generated by
, then the ideal generated by  is an irreducible ideal of
is an irreducible ideal of  .
.
 , it can be shown using norm
, it can be shown using norm
arguments that the number 3 is irreducible. However, it is not a prime in this ring since, for example,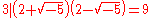
but does not divide either of the two factors.
does not divide either of the two factors.
Abstract algebra
Abstract algebra is the subject area of mathematics that studies algebraic structures, such as groups, rings, fields, modules, vector spaces, and algebras...
, a non-zero non-unit
Unit (ring theory)
In mathematics, an invertible element or a unit in a ring R refers to any element u that has an inverse element in the multiplicative monoid of R, i.e. such element v that...
element in an integral domain is said to be irreducible if it is not a product of two non-units.
Irreducible elements should not be confused with prime element
Prime element
In abstract algebra, an element p of a commutative ring R is said to be prime if it is not zero, not a unit and whenever p divides ab for some a and b in R, then p divides a or p divides b...
s. (A non-unit element
 in a commutative ring
in a commutative ringCommutative ring
In ring theory, a branch of abstract algebra, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra....
 is called prime if whenever
is called prime if whenever 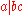 for some
for some  and
and  in
in  , then
, then 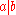 or
or 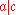 .) Every prime element is irreducible, but the converse is not true in general. The converse is true for UFD
.) Every prime element is irreducible, but the converse is not true in general. The converse is true for UFDUnique factorization domain
In mathematics, a unique factorization domain is, roughly speaking, a commutative ring in which every element, with special exceptions, can be uniquely written as a product of prime elements , analogous to the fundamental theorem of arithmetic for the integers...
s (or, more generally, GCD domain
GCD domain
In mathematics, a GCD domain is an integral domain R with the property that any two non-zero elements have a greatest common divisor . Equivalently, any two non-zero elements of R have a least common multiple ....
s.)
Moreover, while an ideal generated by a prime element is a prime ideal
Prime ideal
In algebra , a prime ideal is a subset of a ring which shares many important properties of a prime number in the ring of integers...
, it is not true in general that an ideal generated by an irreducible element is an irreducible ideal
Irreducible ideal
In mathematics, an ideal of a commutative ring is said to be irreducible if it cannot be written as a finite intersection of ideals properly containing it....
. However, if
 is a GCD domain, and
is a GCD domain, and  is an irreducible element of
is an irreducible element of  , then the ideal generated by
, then the ideal generated by  is an irreducible ideal of
is an irreducible ideal of  .
.Example
In the quadratic integer ring , it can be shown using norm
, it can be shown using normField norm
In mathematics, the norm is a mapping defined in field theory, to map elements of a larger field into a smaller one.-Formal definitions:1. Let K be a field and L a finite extension of K...
arguments that the number 3 is irreducible. However, it is not a prime in this ring since, for example,
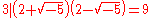
but
 does not divide either of the two factors.
does not divide either of the two factors.

