
Langlands–Shahidi method
Encyclopedia
In mathematics, the Langlands–Shahidi method provides the means to define automorphic L-functions in many cases that arise with connected reductive groups
over a number field
. This includes Rankin–Selberg
products for cuspidal automorphic representations of general linear groups
. The method develops the theory of the local coefficient, which links to the global theory via Eisenstein series
. The resulting L-functions satisfy a number of analytic properties, including an important functional equation.
 , together with a Levi subgroup
, together with a Levi subgroup 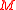 , defined over a local field
, defined over a local field
 . For example, if
. For example, if  is a classical group
is a classical group
of rank , its maximal Levi subgroups are of the form
, its maximal Levi subgroups are of the form  ,
,  . F. Shahidi
. F. Shahidi
develops the theory of the local coefficient for irreducible generic representations of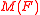 . The local coefficient is defined by means of the uniqueness property of Whittaker models
. The local coefficient is defined by means of the uniqueness property of Whittaker models
paired with the theory of intertwining operators for representations obtained by parabolic induction from generic representations.
The global intertwining operator appearing in the functional equation of Langlands
' theory of Eisenstein series can be decomposed as a product of local intertwining operators. When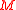 is a maximal Levi subgroup, local coefficients arise from Fourier coefficients of appropriately chosen Eisenstein series and satisfy a crude functional equation involving a product of partial L-functions.
is a maximal Levi subgroup, local coefficients arise from Fourier coefficients of appropriately chosen Eisenstein series and satisfy a crude functional equation involving a product of partial L-functions.
 to individual functional equations of partial
to individual functional equations of partial 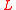 -functions and
-functions and  -factors:
-factors:
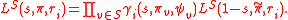
The details are technical: is a finite set of places (of the underlying global field) with
is a finite set of places (of the underlying global field) with 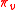 unramified for
unramified for 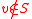 , and
, and  is the adjoint action of
is the adjoint action of 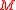 on the complex Lie algebra of a specific subgroup of the Langlands dual group
on the complex Lie algebra of a specific subgroup of the Langlands dual group
of . When
. When  is the special linear group
is the special linear group
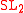 , and
, and  is the maximal torus of diagonal matrices,
is the maximal torus of diagonal matrices,  -factors (
-factors ( is a character of idèle classes in this situation) are the local factors of Tate's thesis
is a character of idèle classes in this situation) are the local factors of Tate's thesis
.
The -factors are uniquely characterized by their role in the functional equation and a list of local properties, including multiplicativity with respect to parabolic induction. They satisfy a relationship involving Artin L-functions
-factors are uniquely characterized by their role in the functional equation and a list of local properties, including multiplicativity with respect to parabolic induction. They satisfy a relationship involving Artin L-functions
and Artin root numbers when gives an archimedean local field or when
gives an archimedean local field or when  is non-archimedean and
is non-archimedean and 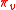 is a constituent of an unramified principal series representation of
is a constituent of an unramified principal series representation of 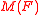 . Local
. Local 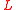 -functions and root numbers ε
-functions and root numbers ε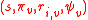 are then defined at every place, including
are then defined at every place, including 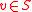 , by means of Langlands classification for
, by means of Langlands classification for  -adic groups. The functional equation takes the form
-adic groups. The functional equation takes the form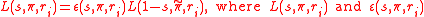
are the completed global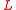 -function and root number.
-function and root number.
A full list of Langlands–Shahidi L-functions depends on the quasi-split group and maximal Levi subgroup
and maximal Levi subgroup 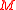 . More specifically, the decomposition of the adjoint action
. More specifically, the decomposition of the adjoint action  can be classified using Dynkin diagrams.
can be classified using Dynkin diagrams.
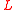 -functions are said to be nice if they satisfy:
-functions are said to be nice if they satisfy:
Langlands–Shahidi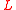 -functions satisfy the functional equation. Progress towards boundedness in vertical strips was made by S. S. Gelbart and F. Shahidi. And, after incorporating twists by highly ramified characters, Langlands–Shahidi
-functions satisfy the functional equation. Progress towards boundedness in vertical strips was made by S. S. Gelbart and F. Shahidi. And, after incorporating twists by highly ramified characters, Langlands–Shahidi 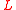 -functions do become entire.
-functions do become entire.
Another result is the non-vanishing of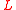 -functions. For Rankin–Selberg products of general linear groups it states that
-functions. For Rankin–Selberg products of general linear groups it states that  is non-zero for every real number t.
is non-zero for every real number t.
Applications to functoriality and to representation theory of
Here,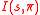 is obtained by unitary parabolic induction from
is obtained by unitary parabolic induction from
Reductive group
In mathematics, a reductive group is an algebraic group G over an algebraically closed field such that the unipotent radical of G is trivial . Any semisimple algebraic group is reductive, as is any algebraic torus and any general linear group...
over a number field
Algebraic number field
In mathematics, an algebraic number field F is a finite field extension of the field of rational numbers Q...
. This includes Rankin–Selberg
Rankin–Selberg method
In mathematics, the Rankin–Selberg method, introduced by and , also known as the theory of integral representations of L-functions, is a technique for directly constructing and analytically continuing several important examples of automorphic L-functions. Some authors reserve the term for a...
products for cuspidal automorphic representations of general linear groups
General linear group
In mathematics, the general linear group of degree n is the set of n×n invertible matrices, together with the operation of ordinary matrix multiplication. This forms a group, because the product of two invertible matrices is again invertible, and the inverse of an invertible matrix is invertible...
. The method develops the theory of the local coefficient, which links to the global theory via Eisenstein series
Eisenstein series
Eisenstein series, named after German mathematician Gotthold Eisenstein, are particular modular forms with infinite series expansions that may be written down directly...
. The resulting L-functions satisfy a number of analytic properties, including an important functional equation.
The local coefficient
The setting is in the generality of a connected quasi-split reductive group , together with a Levi subgroup
, together with a Levi subgroup 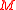 , defined over a local field
, defined over a local fieldLocal field
In mathematics, a local field is a special type of field that is a locally compact topological field with respect to a non-discrete topology.Given such a field, an absolute value can be defined on it. There are two basic types of local field: those in which the absolute value is archimedean and...
 . For example, if
. For example, if  is a classical group
is a classical groupClassical group
In mathematics, the classical Lie groups are four infinite families of Lie groups closely related to the symmetries of Euclidean spaces. Their finite analogues are the classical groups of Lie type...
of rank
 , its maximal Levi subgroups are of the form
, its maximal Levi subgroups are of the form  ,
,  . F. Shahidi
. F. ShahidiFreydoon Shahidi
Freydoon Shahidi is an Iranian mathematician who is currently a Distinguished Professor of Mathematics at Purdue University in the U.S. He is known for a method of automorphic L-functions which is now known as the Langlands–Shahidi method. He is a member of the American Academy of Arts and...
develops the theory of the local coefficient for irreducible generic representations of
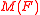 . The local coefficient is defined by means of the uniqueness property of Whittaker models
. The local coefficient is defined by means of the uniqueness property of Whittaker modelsWhittaker model
In representation theory, a branch of mathematics, the Whittaker model is a realization of a representation of a reductive algebraic group such as GL2 over a finite or local or global field on a space of functions on the group. It is named after E. T...
paired with the theory of intertwining operators for representations obtained by parabolic induction from generic representations.
The global intertwining operator appearing in the functional equation of Langlands
Robert Langlands
Robert Phelan Langlands is a mathematician, best known as the founder of the Langlands program. He is an emeritus professor at the Institute for Advanced Study...
' theory of Eisenstein series can be decomposed as a product of local intertwining operators. When
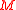 is a maximal Levi subgroup, local coefficients arise from Fourier coefficients of appropriately chosen Eisenstein series and satisfy a crude functional equation involving a product of partial L-functions.
is a maximal Levi subgroup, local coefficients arise from Fourier coefficients of appropriately chosen Eisenstein series and satisfy a crude functional equation involving a product of partial L-functions.Local factors and functional equation
An induction step refines the crude functional equation of a globally generic cuspidal automorphic representation to individual functional equations of partial
to individual functional equations of partial 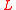 -functions and
-functions and  -factors:
-factors: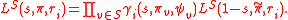
The details are technical:
 is a finite set of places (of the underlying global field) with
is a finite set of places (of the underlying global field) with 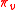 unramified for
unramified for 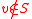 , and
, and  is the adjoint action of
is the adjoint action of 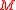 on the complex Lie algebra of a specific subgroup of the Langlands dual group
on the complex Lie algebra of a specific subgroup of the Langlands dual groupDual group
In mathematics, the dual group may be:* The Pontryagin dual of a locally compact abelian group* The Langlands dual of a reductive algebraic group* The Deligne-Lusztig dual of a reductive group over a finite field....
of
 . When
. When  is the special linear group
is the special linear groupSpecial linear group
In mathematics, the special linear group of degree n over a field F is the set of n×n matrices with determinant 1, with the group operations of ordinary matrix multiplication and matrix inversion....
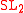 , and
, and  is the maximal torus of diagonal matrices,
is the maximal torus of diagonal matrices,  -factors (
-factors ( is a character of idèle classes in this situation) are the local factors of Tate's thesis
is a character of idèle classes in this situation) are the local factors of Tate's thesisJohn Tate
John Torrence Tate Jr. is an American mathematician, distinguished for many fundamental contributions in algebraic number theory, arithmetic geometry and related areas in algebraic geometry.-Biography:...
.
The
 -factors are uniquely characterized by their role in the functional equation and a list of local properties, including multiplicativity with respect to parabolic induction. They satisfy a relationship involving Artin L-functions
-factors are uniquely characterized by their role in the functional equation and a list of local properties, including multiplicativity with respect to parabolic induction. They satisfy a relationship involving Artin L-functionsArtin L-function
In mathematics, an Artin L-function is a type of Dirichlet series associated to a linear representation ρ of a Galois group G. These functions were introduced in the 1923 by Emil Artin, in connection with his research into class field theory. Their fundamental properties, in particular the Artin...
and Artin root numbers when
 gives an archimedean local field or when
gives an archimedean local field or when  is non-archimedean and
is non-archimedean and 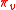 is a constituent of an unramified principal series representation of
is a constituent of an unramified principal series representation of 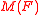 . Local
. Local 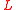 -functions and root numbers ε
-functions and root numbers ε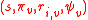 are then defined at every place, including
are then defined at every place, including 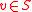 , by means of Langlands classification for
, by means of Langlands classification for  -adic groups. The functional equation takes the form
-adic groups. The functional equation takes the form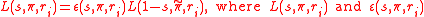
are the completed global
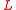 -function and root number.
-function and root number.Examples of automorphic L-functions
-
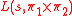 , the Rankin–Selberg
, the Rankin–Selberg  -function of cuspidal automorphic representations
-function of cuspidal automorphic representations  of
of 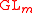 and
and  of
of 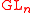 .
.
-
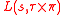 , where
, where  is a cuspidal automorphic representation of
is a cuspidal automorphic representation of  and
and  is a globally generic cuspidal automorphic representation of a classical group
is a globally generic cuspidal automorphic representation of a classical group  .
.
-
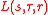 , with
, with  as before and
as before and  a symmetric square, an exterior square, or an Asai representation of the dual group of
a symmetric square, an exterior square, or an Asai representation of the dual group of 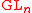 .
.
A full list of Langlands–Shahidi L-functions depends on the quasi-split group
 and maximal Levi subgroup
and maximal Levi subgroup 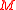 . More specifically, the decomposition of the adjoint action
. More specifically, the decomposition of the adjoint action  can be classified using Dynkin diagrams.
can be classified using Dynkin diagrams.Analytic properties of L-functions
Global -functions are said to be nice if they satisfy:
-functions are said to be nice if they satisfy:-
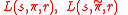 extend to entire functions of the complex variable
extend to entire functions of the complex variable  .
. -
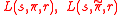 are bounded in vertical strips.
are bounded in vertical strips. - (Functional Equation)
 .
.
Langlands–Shahidi
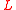 -functions satisfy the functional equation. Progress towards boundedness in vertical strips was made by S. S. Gelbart and F. Shahidi. And, after incorporating twists by highly ramified characters, Langlands–Shahidi
-functions satisfy the functional equation. Progress towards boundedness in vertical strips was made by S. S. Gelbart and F. Shahidi. And, after incorporating twists by highly ramified characters, Langlands–Shahidi 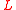 -functions do become entire.
-functions do become entire.Another result is the non-vanishing of
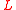 -functions. For Rankin–Selberg products of general linear groups it states that
-functions. For Rankin–Selberg products of general linear groups it states that  is non-zero for every real number t.
is non-zero for every real number t. Applications to functoriality and to representation theory of 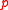 -adic groups
-adic groups
- Functoriality for the classical groups: A cuspidal globally generic automorphic representation of a classical group admits a Langlands functorialLanglands programThe Langlands program is a web of far-reaching and influential conjectures that relate Galois groups in algebraic number theory to automorphic forms and representation theory of algebraic groups over local fields and adeles. It was proposed by ....
lift to an automorphic representation of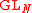 , where
, where  depends on the classical group. Then, the Ramanujan bounds of W. Luo, Z. Rudnick and P. Sarnak for
depends on the classical group. Then, the Ramanujan bounds of W. Luo, Z. Rudnick and P. Sarnak for 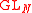 over number fields yield non-trivial bounds for the generalized Ramanujan conjecture of the classical groups.
over number fields yield non-trivial bounds for the generalized Ramanujan conjecture of the classical groups.
- Symmetric powers for
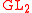 : Proofs of functoriality for the symmetric cube and for the symmetric fourth powers of cuspidal automorphic representations of
: Proofs of functoriality for the symmetric cube and for the symmetric fourth powers of cuspidal automorphic representations of 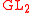 were made possible by the Langlands–Shahidi method. Progress towards higher Symmetric powers leads to the best possible bounds towards the Ramanujan–Peterson conjecture of automorphic cusp forms of
were made possible by the Langlands–Shahidi method. Progress towards higher Symmetric powers leads to the best possible bounds towards the Ramanujan–Peterson conjecture of automorphic cusp forms of 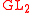 .
.
- Representations of
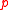 -adic groups: Applications involving Harish-ChandraHarish-ChandraHarish-Chandra was an Indian mathematician, who did fundamental work in representation theory, especially Harmonic analysis on semisimple Lie groups. -Life:...
-adic groups: Applications involving Harish-ChandraHarish-ChandraHarish-Chandra was an Indian mathematician, who did fundamental work in representation theory, especially Harmonic analysis on semisimple Lie groups. -Life:...
 functions (from the Plancherel formula) and to complementary series of
functions (from the Plancherel formula) and to complementary series of 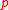 -adic reductive groups are possible. For example,
-adic reductive groups are possible. For example, 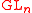 appears as the Siegel Levi subgroup of a classical group G. If
appears as the Siegel Levi subgroup of a classical group G. If  is a smooth irreducible ramified supercuspidal representation of
is a smooth irreducible ramified supercuspidal representation of 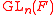 over a field
over a field  of
of 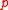 -adic numbers, and
-adic numbers, and 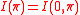 is irreducible, then:
is irreducible, then:
-
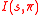 is irreducible and in the complementary series for
is irreducible and in the complementary series for 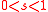 ;
; -
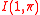 is reducible and has a unique generic non-supercuspidal discrete series subrepresentation;
is reducible and has a unique generic non-supercuspidal discrete series subrepresentation; -
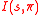 is irreducible and never in the complementary series for
is irreducible and never in the complementary series for  .
.
Here,
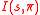 is obtained by unitary parabolic induction from
is obtained by unitary parabolic induction from
 if
if 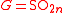 ,
, 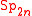 , or
, or  ;
; if
if  , or
, or 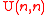 .
.

