
Macbeath surface
Encyclopedia
In Riemann surface
theory and hyperbolic geometry
, the Macbeath surface, also called Macbeath's curve or the Fricke–Macbeath curve, is the genus-7 Hurwitz surface
.
The automorphism group of the Macbeath surface is the simple group
PSL(2,8)
, consisting of 504 symmetries.
can be constructed as the principal congruence subgroup of the (2,3,7) triangle group
in a suitable tower of principal congruence subgroups. Here the choices of quaternion algebra and Hurwitz quaternion order
are described at the triangle group page. Choosing the ideal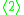 in the ring of integers, the corresponding principal congruence subgroup defines this surface of genus 7. Its systole is about 5.796, and the number of systolic loops is 126 according to R. Vogeler's calculations.
in the ring of integers, the corresponding principal congruence subgroup defines this surface of genus 7. Its systole is about 5.796, and the number of systolic loops is 126 according to R. Vogeler's calculations.
in a 24.vii.1990 letter to Abhyankar".
Riemann surface
In mathematics, particularly in complex analysis, a Riemann surface, first studied by and named after Bernhard Riemann, is a one-dimensional complex manifold. Riemann surfaces can be thought of as "deformed versions" of the complex plane: locally near every point they look like patches of the...
theory and hyperbolic geometry
Hyperbolic geometry
In mathematics, hyperbolic geometry is a non-Euclidean geometry, meaning that the parallel postulate of Euclidean geometry is replaced...
, the Macbeath surface, also called Macbeath's curve or the Fricke–Macbeath curve, is the genus-7 Hurwitz surface
Hurwitz surface
In Riemann surface theory and hyperbolic geometry, a Hurwitz surface, named after Adolf Hurwitz, is a compact Riemann surface with preciselyautomorphisms, where g is the genus of the surface. This number is maximal by virtue of Hurwitz's theorem on automorphisms...
.
The automorphism group of the Macbeath surface is the simple group
Simple group
In mathematics, a simple group is a nontrivial group whose only normal subgroups are the trivial group and the group itself. A group that is not simple can be broken into two smaller groups, a normal subgroup and the quotient group, and the process can be repeated...
PSL(2,8)
Projective linear group
In mathematics, especially in the group theoretic area of algebra, the projective linear group is the induced action of the general linear group of a vector space V on the associated projective space P...
, consisting of 504 symmetries.
Triangle group construction
The surface's Fuchsian groupFuchsian group
In mathematics, a Fuchsian group is a discrete subgroup of PSL. The group PSL can be regarded as a group of isometries of the hyperbolic plane, or conformal transformations of the unit disc, or conformal transformations of the upper half plane, so a Fuchsian group can be regarded as a group acting...
can be constructed as the principal congruence subgroup of the (2,3,7) triangle group
(2,3,7) triangle group
In the theory of Riemann surfaces and hyperbolic geometry, the triangle group is particularly important. This importance stems from its connection to Hurwitz surfaces, namely Riemann surfaces of genus g with the largest possible order, 84, of its automorphism group.A note on terminology – the "...
in a suitable tower of principal congruence subgroups. Here the choices of quaternion algebra and Hurwitz quaternion order
Hurwitz quaternion order
The Hurwitz quaternion order is a specific order in a quaternion algebra over a suitable number field. The order is of particular importance in Riemann surface theory, in connection with surfaces with maximal symmetry, namely the Hurwitz surfaces. The Hurwitz quaternion order was studied in 1967...
are described at the triangle group page. Choosing the ideal
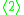 in the ring of integers, the corresponding principal congruence subgroup defines this surface of genus 7. Its systole is about 5.796, and the number of systolic loops is 126 according to R. Vogeler's calculations.
in the ring of integers, the corresponding principal congruence subgroup defines this surface of genus 7. Its systole is about 5.796, and the number of systolic loops is 126 according to R. Vogeler's calculations.Historical note
This surface was originally discovered by , but named after Alexander Murray Macbeath due to his later independent rediscovery of the same curve. Elkies writes that the equivalence between the curves studied by Fricke and Macbeath "may first have been observed by SerreJean-Pierre Serre
Jean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
in a 24.vii.1990 letter to Abhyankar".

