Riemann surface
Encyclopedia
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, particularly in complex analysis
Complex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is useful in many branches of mathematics, including number theory and applied mathematics; as well as in physics,...
, a Riemann surface, first studied by and named after Bernhard Riemann
Bernhard Riemann
Georg Friedrich Bernhard Riemann was an influential German mathematician who made lasting contributions to analysis and differential geometry, some of them enabling the later development of general relativity....
, is a one-dimensional complex manifold
Complex manifold
In differential geometry, a complex manifold is a manifold with an atlas of charts to the open unit disk in Cn, such that the transition maps are holomorphic....
. Riemann surfaces can be thought of as "deformed versions" of the complex plane
Complex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
: locally near every point they look like patches of the complex plane, but the global topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
can be quite different. For example, they can look like a sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
or a torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
or a couple of sheets glued together.
The main point of Riemann surfaces is that holomorphic function
Holomorphic function
In mathematics, holomorphic functions are the central objects of study in complex analysis. A holomorphic function is a complex-valued function of one or more complex variables that is complex differentiable in a neighborhood of every point in its domain...
s may be defined between them. Riemann surfaces are nowadays considered the natural setting for studying the global behavior of these functions, especially multi-valued functions such as the square root
Square root
In mathematics, a square root of a number x is a number r such that r2 = x, or, in other words, a number r whose square is x...
and other algebraic function
Algebraic function
In mathematics, an algebraic function is informally a function that satisfies a polynomial equation whose coefficients are themselves polynomials with rational coefficients. For example, an algebraic function in one variable x is a solution y for an equationwhere the coefficients ai are polynomial...
s, or the logarithm
Natural logarithm
The natural logarithm is the logarithm to the base e, where e is an irrational and transcendental constant approximately equal to 2.718281828...
.
Every Riemann surface is a two-dimensional real analytic manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
(i.e., a surface
Surface
In mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
), but it contains more structure (specifically a complex structure) which is needed for the unambiguous definition of holomorphic functions. A two-dimensional real manifold can be turned into a Riemann surface (usually in several inequivalent ways) if and only if it is orientable and metrizable. So the sphere and torus admit complex structures, but the Möbius strip
Möbius strip
The Möbius strip or Möbius band is a surface with only one side and only one boundary component. The Möbius strip has the mathematical property of being non-orientable. It can be realized as a ruled surface...
, Klein bottle
Klein bottle
In mathematics, the Klein bottle is a non-orientable surface, informally, a surface in which notions of left and right cannot be consistently defined. Other related non-orientable objects include the Möbius strip and the real projective plane. Whereas a Möbius strip is a surface with boundary, a...
and projective plane
Projective plane
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect...
do not.
Geometrical facts about Riemann surfaces are as "nice" as possible, and they often provide the intuition and motivation for generalizations to other curves, manifolds or varieties. The Riemann–Roch theorem
Riemann–Roch theorem
The Riemann–Roch theorem is an important tool in mathematics, specifically in complex analysis and algebraic geometry, for the computation of the dimension of the space of meromorphic functions with prescribed zeroes and allowed poles...
is a prime example of this influence.
Definitions
There are several equivalent definitions of a Riemann surface.- A Riemann surface X is a complex manifoldComplex manifoldIn differential geometry, a complex manifold is a manifold with an atlas of charts to the open unit disk in Cn, such that the transition maps are holomorphic....
of complex dimensionDimensionIn physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...
one. This means that X is a HausdorffHausdorff spaceIn topology and related branches of mathematics, a Hausdorff space, separated space or T2 space is a topological space in which distinct points have disjoint neighbourhoods. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" is the most frequently...
topological spaceTopological spaceTopological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
endowed with an atlasAtlas (topology)In mathematics, particularly topology, one describesa manifold using an atlas. An atlas consists of individualcharts that, roughly speaking, describe individual regionsof the manifold. If the manifold is the surface of the Earth,...
: for every point x ∈ X there is a neighbourhoodOpen setThe concept of an open set is fundamental to many areas of mathematics, especially point-set topology and metric topology. Intuitively speaking, a set U is open if any point x in U can be "moved" a small amount in any direction and still be in the set U...
containing x homeomorphic to the unit disk of the complex planeComplex planeIn mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
. The map carrying the structure of the complex plane to the Riemann surface is called a chart. Additionally, the transition maps between two overlapping charts are required to be holomorphicHolomorphic functionIn mathematics, holomorphic functions are the central objects of study in complex analysis. A holomorphic function is a complex-valued function of one or more complex variables that is complex differentiable in a neighborhood of every point in its domain...
. - A Riemann surface is a manifoldManifoldIn mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
of (real) dimension two – a surfaceSurfaceIn mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
– together with a conformal structure. Again, manifold means that locally at any point x of X, the space is supposed to be like the real plane. The supplement "Riemann" signifies that X is endowed with an additional structure which allows angleAngleIn geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...
measurement on the manifold, namely an equivalence class of so-called Riemannian metrics. Two such metrics are considered equivalent if the angles they measure are the same. Choosing an equivalence class of metrics on X is the additional datum of the conformal structure.
A complex structure gives rise to a conformal structure by choosing the standard Euclidean metric given on the complex plane and transporting it to X by means of the charts. Showing that a conformal structure determines a complex structure is more difficult.
Examples
- The complex planeComplex planeIn mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
C is the most basic Riemann surface. The map f(z) = z (the identity map) defines a chart for C, and {f} is an atlasAtlas (topology)In mathematics, particularly topology, one describesa manifold using an atlas. An atlas consists of individualcharts that, roughly speaking, describe individual regionsof the manifold. If the manifold is the surface of the Earth,...
for C. The map g(z) = z* (the conjugateComplex conjugateIn mathematics, complex conjugates are a pair of complex numbers, both having the same real part, but with imaginary parts of equal magnitude and opposite signs...
map) also defines a chart on C and {g} is an atlas for C. The charts f and g are not compatible, so this endows C with two distinct Riemann surface structures. In fact, given a Riemann surface X and its atlas A, the conjugate atlas B = {f* : f ∈ A} is never compatible with A, and endows X with a distinct, incompatible Riemann structure.
- In an analogous fashion, every open subset of the complex plane can be viewed as a Riemann surface in a natural way. More generally, every open subset of a Riemann surface is a Riemann surface.
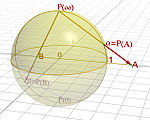
- Let S = C ∪ {∞} and let f(z) = z where z is in S \ {∞} and g(z) = 1 / z where z is in S \ {0} and 1/∞ is defined to be 0. Then f and g are charts, they are compatible, and { f, g } is an atlas for S, making S into a Riemann surface. This particular surface is called the Riemann sphereRiemann sphereIn mathematics, the Riemann sphere , named after the 19th century mathematician Bernhard Riemann, is the sphere obtained from the complex plane by adding a point at infinity...
because it can be interpreted as wrapping the complex plane around the sphere. Unlike the complex plane, it is compactCompact spaceIn mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
.
- The theory of compact Riemann surfaces can be shown to be equivalent to that of projective algebraic curveAlgebraic curveIn algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.- Plane algebraic curves...
s that are defined over the complex numbers and non-singular. For example, the torusTorusIn geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
C/(Z + τ Z), where τ is a complex non-real number, corresponds, via the Weierstrass elliptic function associated to the latticeLattice (group)In mathematics, especially in geometry and group theory, a lattice in Rn is a discrete subgroup of Rn which spans the real vector space Rn. Every lattice in Rn can be generated from a basis for the vector space by forming all linear combinations with integer coefficients...
Z + τ Z, to an elliptic curveElliptic curveIn mathematics, an elliptic curve is a smooth, projective algebraic curve of genus one, on which there is a specified point O. An elliptic curve is in fact an abelian variety — that is, it has a multiplication defined algebraically with respect to which it is a group — and O serves as the identity...
given by an equation
-
- y2 = x3 + a x + b.
- Tori are the only Riemann surfaces of genusGenus (mathematics)In mathematics, genus has a few different, but closely related, meanings:-Orientable surface:The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It...
one, surfaces of higher genera g are provided by the hyperelliptic surfaceHyperelliptic surfaceIn mathematics, a hyperelliptic surface, or bi-elliptic surface, is a surface with an elliptic fibration over an elliptic curve. Any such surface can be written as the quotient of a product of two elliptic curves by a finite abelian group....
s- y2 = P(x),
- where P is a complex polynomialPolynomialIn mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...
of degree 2g + 1.
- Important examples of non-compact Riemann surfaces are provided by analytic continuationAnalytic continuationIn complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which...
.
Further definitions and properties
As with any map between complex manifolds, a functionFunction (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
f: M → N between two Riemann surfaces M and N is called holomorphic if for every chart g in the atlas
Atlas (topology)
In mathematics, particularly topology, one describesa manifold using an atlas. An atlas consists of individualcharts that, roughly speaking, describe individual regionsof the manifold. If the manifold is the surface of the Earth,...
of M and every chart h in the atlas of N, the map h o f o g−1 is holomorphic (as a function from C to C) wherever it is defined. The composition of two holomorphic maps is holomorphic. The two Riemann surfaces M and N are called biholomorphic (or conformally equivalent to emphasize the conformal point of view) if there exists a bijective holomorphic function from M to N whose inverse is also holomorphic (it turns out that the latter condition is automatic and can therefore be omitted). Two conformally equivalent Riemann surfaces are for all practical purposes identical.
Orientability
We noted in the preamble that all Riemann surfaces, like all complex manifolds, are orientable as a real manifold. The reason is that for complex charts f and g with transition function h = f(g−1(z)) we can consider h as a map from an open set of R2 to R2 whose JacobianJacobian
In vector calculus, the Jacobian matrix is the matrix of all first-order partial derivatives of a vector- or scalar-valued function with respect to another vector. Suppose F : Rn → Rm is a function from Euclidean n-space to Euclidean m-space...
in a point z is just the real linear map given by multiplication by the complex number h
Determinant
In linear algebra, the determinant is a value associated with a square matrix. It can be computed from the entries of the matrix by a specific arithmetic expression, while other ways to determine its value exist as well...
of multiplication by a complex number α equals |α|2, so the Jacobian of h has positive determinant. Consequently the complex atlas is an oriented atlas.
Functions
Every non-compact Riemann surface admits non-constant holomorphic functions (with values in C). In fact, every non-compact Riemann surface is a Stein manifoldStein manifold
In mathematics, a Stein manifold in the theory of several complex variables and complex manifolds is a complex submanifold of the vector space of n complex dimensions. The name is for Karl Stein.- Definition :...
.
In contrast, on a compact Riemann surface X every holomorphic function with value in C is constant due to the maximum principle
Maximum principle
In mathematics, the maximum principle is a property of solutions to certain partial differential equations, of the elliptic and parabolic types. Roughly speaking, it says that the maximum of a function in a domain is to be found on the boundary of that domain...
. However, there always exists non-constant meromorphic functions (holomorphic functions with values in the Riemann sphere
Riemann sphere
In mathematics, the Riemann sphere , named after the 19th century mathematician Bernhard Riemann, is the sphere obtained from the complex plane by adding a point at infinity...
C ∪ {∞}). More precisely, the function field
Function field of an algebraic variety
In algebraic geometry, the function field of an algebraic variety V consists of objects which are interpreted as rational functions on V...
of X is a finite extension
Field extension
In abstract algebra, field extensions are the main object of study in field theory. The general idea is to start with a base field and construct in some manner a larger field which contains the base field and satisfies additional properties...
of C(t), the function field in one variable, i.e. any two meromorphic functions are algebraically dependent. This statement generalizes to higher dimensions, see .
Analytic vs. algebraic
The above fact about existence of nonconstant meromorphic functions can be used to show that any compact Riemann surface is a projective variety, i.e. can be given by polynomialPolynomial
In mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...
equations inside a projective space
Projective space
In mathematics a projective space is a set of elements similar to the set P of lines through the origin of a vector space V. The cases when V=R2 or V=R3 are the projective line and the projective plane, respectively....
. Actually, it can be shown that every compact Riemann surface can be embedded into complex projective 3-space
Complex projective space
In mathematics, complex projective space is the projective space with respect to the field of complex numbers. By analogy, whereas the points of a real projective space label the lines through the origin of a real Euclidean space, the points of a complex projective space label the complex lines...
. This is a surprising theorem: Riemann surfaces are given by locally patching charts. If one global condition, namely compactness, is added, the surface is necessarily algebraic. This feature of Riemann surfaces allows to study them with either the means of analytic
Analytic geometry
Analytic geometry, or analytical geometry has two different meanings in mathematics. The modern and advanced meaning refers to the geometry of analytic varieties...
or algebraic geometry
Algebraic geometry
Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
. The corresponding statement for higher-dimensional objects is false, i.e. there are compact complex 2-manifolds which are not algebraic. On the other hand, every projective complex manifold is necessarily algebraic, see Chow's theorem.
As an example, consider the torus T := C/(Z + τ Z). The Weierstrass function
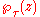belonging to the lattice Z + τ Z is a meromorphic functionMeromorphic function
In complex analysis, a meromorphic function on an open subset D of the complex plane is a function that is holomorphic on all D except a set of isolated points, which are poles for the function...
on T. This function and its derivative
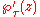generateGenerating set
In mathematics, the expressions generator, generate, generated by and generating set can have several closely related technical meanings:...
the function field of T. There is an equation
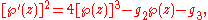
where the coefficients g2 and g3 depend on τ, thus giving an elliptic curve Eτ in the sense of algebraic geometry. Reversing this is accomplished by the j-invariant
J-invariant
In mathematics, Klein's j-invariant, regarded as a function of a complex variable τ, is a modular function defined on the upper half-plane of complex numbers.We haveThe modular discriminant \Delta is defined as \Delta=g_2^3-27g_3^2...
j(E), which can be used to determine τ and hence a torus.
Classification of Riemann surfaces
The realm of Riemann surfaces can be divided into three regimes: hyperbolic, parabolic and elliptic Riemann surfaces, with the distinction given by the uniformization theoremUniformization theorem
In mathematics, the uniformization theorem says that any simply connected Riemann surface is conformally equivalent to one of the three domains: the open unit disk, the complex plane, or the Riemann sphere. In particular it admits a Riemannian metric of constant curvature...
. Geometrically, these correspond to negative curvature, zero curvature/flat, and positive curvature: stating the uniformization theorem in terms of conformal geometry, every connected Riemann surface X admits a unique complete 2-dimensional real Riemann metric
Riemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
with constant curvature
Curvature
In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...
−1, 0 or 1 inducing the same conformal structure – every metric is conformally equivalent to a constant curvature metric. The surface X is called hyperbolic, parabolic, and elliptic, respectively.
For simply connected Riemann surfaces, the uniformization theorem states that every simply connected Riemann surface is conformally equivalent to one of the following:
elliptic: the Riemann sphere
C ∪ {∞}, also denoted P1Cparabolic: the complex plane C, or
hyperbolic: the open disk D := {z ∈ C : |z| < 1} or equivalently the upper half-plane H := {z ∈ C : Im(z) > 0}.
The existence of these three types parallels the several non-Euclidean geometries
Non-Euclidean geometry
Non-Euclidean geometry is the term used to refer to two specific geometries which are, loosely speaking, obtained by negating the Euclidean parallel postulate, namely hyperbolic and elliptic geometry. This is one term which, for historical reasons, has a meaning in mathematics which is much...
.
The general technique of associating to a manifold X its universal cover Y, and expressing the original X as the quotient of Y by the group of deck transformations gives a first overview over Riemann surfaces.
Elliptic Riemann surfaces
By definition, these are the surfaces X with constant curvature +1. The Riemann sphereRiemann sphere
In mathematics, the Riemann sphere , named after the 19th century mathematician Bernhard Riemann, is the sphere obtained from the complex plane by adding a point at infinity...
C ∪ {∞} is the only example. (Elliptic function
Elliptic function
In complex analysis, an elliptic function is a function defined on the complex plane that is periodic in two directions and at the same time is meromorphic...
s are examples of parabolic Riemann surfaces. The naming comes from the history: elliptic functions are associated to elliptic integrals, which in turn show up in calculating the circumference
Circumference
The circumference is the distance around a closed curve. Circumference is a special perimeter.-Circumference of a circle:The circumference of a circle is the length around it....
of ellipse
Ellipse
In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
s).
Parabolic Riemann surfaces
By definition, these are the surfaces X with constant curvature 0. Equivalently, by the uniformization theorem, the universal cover of X has to be the complex plane.There are then three possibilities for X. It can be the plane itself, an annulus
Annulus (mathematics)
In mathematics, an annulus is a ring-shaped geometric figure, or more generally, a term used to name a ring-shaped object. Or, it is the area between two concentric circles...
, or a torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
- T := C / (Z ⊕ τZ).
The set of representatives of the cosets are called fundamental domain
Fundamental domain
In geometry, the fundamental domain of a symmetry group of an object is a part or pattern, as small or irredundant as possible, which determines the whole object based on the symmetry. More rigorously, given a topological space and a group acting on it, the images of a single point under the group...
s.
Care must be taken insofar as two tori are always homeomorphic, but in general not biholomorphic to each other. This is the first appearance of the problem of moduli. The modulus of a torus can be captured by a single complex number τ with positive imaginary part. In fact, the marked moduli space (Teichmüller space
Teichmüller space
In mathematics, the Teichmüller space TX of a topological surface X, is a space that parameterizes complex structures on X up to the action of homeomorphisms that are isotopic to the identity homeomorphism...
) of the torus is biholomorphic to the upper half-plane or equivalently the open unit disk.
Hyperbolic Riemann surfaces
The Riemann surfaces with curvature −1 are called hyperbolic. This group is the "biggest" one.The celebrated Riemann mapping theorem states that any simply connected strict subset of the complex plane is biholomorphic to the unit disk. Therefore the open disk with the Poincaré-metric of constant curvature −1 is the local model of any hyperbolic Riemann surface. According to the uniformization theorem above, all hyperbolic surfaces are quotients of the unit disk.
Examples include all surfaces with genus g > 1 such as hyper-elliptic curves.
For every hyperbolic Riemann surface, the fundamental group is isomorphic to a Fuchsian group
Fuchsian group
In mathematics, a Fuchsian group is a discrete subgroup of PSL. The group PSL can be regarded as a group of isometries of the hyperbolic plane, or conformal transformations of the unit disc, or conformal transformations of the upper half plane, so a Fuchsian group can be regarded as a group acting...
, and thus the surface can be modelled by a Fuchsian model
Fuchsian model
In mathematics, a Fuchsian model is a construction of a hyperbolic Riemann surface R as a quotient of the upper half-plane H. By the uniformization theorem, every Riemann surface is either elliptic, parabolic or hyperbolic. Every hyperbolic Riemann surface has a non-trivial fundamental group \pi_1...
H/Γ where H is the upper half-plane and Γ is the Fuchsian group. The set of representatives of the cosets of H/Γ are free regular set
Free regular set
In mathematics, a free regular set is a subset of a topological space that is acted upon disjointly under a given group action.To be more precise, let X be a topological space. Let G be a group of homeomorphisms from X to X...
s and can be fashioned into metric fundamental polygon
Fundamental polygon
In mathematics, each closed surface in the sense of geometric topology can be constructed from an even-sided oriented polygon, called a fundamental polygon, by pairwise identification of its edges....
s. Quotient structures as H/Γ are generalized to Shimura varieties
Shimura variety
In number theory, a Shimura variety is a higher-dimensional analogue of a modular curve that arises as a quotient of a Hermitian symmetric space by a congruence subgroup of a reductive algebraic group defined over Q. The term "Shimura variety" applies to the higher-dimensional case, in the case of...
.
Unlike elliptic and parabolic surfaces, no classification of the hyperbolic surfaces is possible. Any connected open strict subset of the plane gives a hyperbolic surface; consider the plane minus a Cantor set
Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of remarkable and deep properties. It was discovered in 1875 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883....
. A classification is possible for surfaces of finite type: those isomorphic to a compact surface with a finite number of points removed. Any one of these has a finite number of moduli and so a finite dimensional Teichmüller space. The problem of moduli
Moduli space
In algebraic geometry, a moduli space is a geometric space whose points represent algebro-geometric objects of some fixed kind, or isomorphism classes of such objects...
(solved by Lars Ahlfors and extended by Lipman Bers
Lipman Bers
Lipman Bers was an American mathematician born in Riga who created the theory of pseudoanalytic functions and worked on Riemann surfaces and Kleinian groups.-Biography:...
) was to justify Riemann's claim that for a closed surface of genus g, 3g − 3 complex parameters suffice.
When a hyperbolic surface is compact, then the total area of the surface is 4π(g − 1), where g is the genus
Genus (mathematics)
In mathematics, genus has a few different, but closely related, meanings:-Orientable surface:The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It...
of the surface; the area is obtained by applying the Gauss-Bonnet theorem to the area of the fundamental polygon.
Maps between Riemann surfaces
The geometric classification is reflected in maps between Riemann surfaces,as detailed in Liouville's theorem
Liouville's theorem (complex analysis)
In complex analysis, Liouville's theorem, named after Joseph Liouville, states that every bounded entire function must be constant. That is, every holomorphic function f for which there exists a positive number M such that |f| ≤ M for all z in C is constant.The theorem is considerably improved by...
and the Little Picard theorem: maps from hyperbolic to parabolic to elliptic are easy, but maps from elliptic to parabolic or parabolic to hyperbolic are very constrained (indeed, generally constant!). There are inclusions of the disc in the plane in the sphere:
but any meromorphic map from the sphere to the plane is constant, any holomorphic map from the plane into the unit disk is constant (Liouville's theorem), and in fact any holomorphic map from the plane into the plane minus two points is constant (Little Picard theorem)!Punctured spheres
These statements are clarified by considering the type of a Riemann sphere
with a number of punctures. With no punctures, it is the Riemann sphere, which is elliptic. With one puncture, which can be placed at infinity, it is the complex plane, which is parabolic. With two punctures, it is the punctured plane or alternatively annulus or cylinder, which is parabolic. With three or more punctures, it is hyperbolic – compare pair of pantsPair of pants
In mathematics, a pair of pants is a simple two-dimensional surface resembling a pair of pants: topologically, it is a sphere with three holes in it...
. One can map from one puncture to two, via the exponential map (which is entire and has an essential singularity at infinity, so not defined at infinity, and misses zero and infinity), but all maps from zero punctures to one or more, or one or two punctures to three or more are constant.
Ramified covering spaces
Continuing in this vein, compact Riemann surfaces can map to surfaces of lower genus, but not to higher genus, except as constant maps. This is because holomorphic and meromorphic maps behave locally like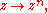
so non-constant maps are ramified covering maps, and for compact Riemann surfaces these are constrained by the Riemann–Hurwitz formula in algebraic topologyAlgebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
, which relates the Euler characteristic
Euler characteristic
In mathematics, and more specifically in algebraic topology and polyhedral combinatorics, the Euler characteristic is a topological invariant, a number that describes a topological space's shape or structure regardless of the way it is bent...
of a space and a ramified cover.
For example, hyperbolic Riemann surfaces are ramified covering spaces of the sphere (they have non-constant meromorphic functions), but the sphere does not cover or otherwise map to higher genus surfaces, except as a constant.
Isometries of Riemann surfaces
The isometry group of a uniformized Riemann surface (equivalently, the conformal automorphism group) reflects its geometry:- genus 0 – the isometry group of the sphere is the Möbius group of projective transforms of the complex line,
- the isometry group of the plane is the subgroup fixing infinity, and of the punctured plane is the subgroup fixing infinity and zero as a set: either fixing them both, or interchanging them (1/z).
- the isometry group of the upper half-planePoincaré half-plane modelIn non-Euclidean geometry, the Poincaré half-plane model is the upper half-plane , together with a metric, the Poincaré metric, that makes it a model of two-dimensional hyperbolic geometry....
is the real Möbius group; this is conjugate to the automorphism group of the disk. - genus 1 – the isometry group of a torus is in general translations (as an Abelian varietyAbelian varietyIn mathematics, particularly in algebraic geometry, complex analysis and number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functions...
), though the square lattice and hexagonal lattice have addition symmetries from rotation by 90° and 60°. - For genus ≥ 2, the isometry group is finite, and has order at most
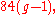by Hurwitz's automorphisms theorem; surfaces that realize this bound are called Hurwitz surfaces. - It's known that every finite group can be realized as the full group of isometries of some riemann surface.
- For genus 2 the order is maximized by the Bolza surfaceBolza surfaceIn mathematics, the Bolza surface, alternatively, complex algebraic Bolza curve , is a compact Riemann surface of genus 2 with the highest possible order of the conformal automorphism group in this genus, namely 48. An affine model for the Bolza surface can be obtained as the locus of the...
, with order 48. - For genus 3 the order is maximized by the Klein quarticKlein quarticIn hyperbolic geometry, the Klein quartic, named after Felix Klein, is a compact Riemann surface of genus 3 with the highest possible order automorphism group for this genus, namely order 168 orientation-preserving automorphisms, and 336 automorphisms if orientation may be reversed...
, with order 168; this is the first Hurwitz surface, and its automorphism group is isomorphic to PSL(2,7)PSL(2,7)In mathematics, the projective special linear group PSL is a finite simple group that has important applications in algebra, geometry, and number theory. It is the automorphism group of the Klein quartic as well as the symmetry group of the Fano plane...
, the second-smallest non-abelian simple group. - For genus 4, Bring's surface is a highly symmetric surface.
- For genus 7 the order is maximized by the Macbeath surfaceMacbeath surfaceIn Riemann surface theory and hyperbolic geometry, the Macbeath surface, also called Macbeath's curve or the Fricke–Macbeath curve, is the genus-7 Hurwitz surface....
, with order 504; this is the second Hurwitz surface, and its automorphism group is isomorphic to PSL(2,8), the fourth-smallest non-abelian simple group.
- For genus 2 the order is maximized by the Bolza surface
Function-theoretic classification
The classification scheme above is typically used by geometers. There is a different classification for Riemann surfaces which is typically used by complex analysts. It employs a different definition for "parabolic" and "hyperbolic". In this alternative classification scheme, a Riemann surface is called parabolic if there are no nonconstant negative subharmonic functions on the surface and is otherwise called hyperbolic. This class of hyperbolic surfaces is further subdivided into subclasses according to whether function spaces other than the negative subharmonic functions are degenerate, e.g. Riemann surfaces on which all bounded holomorphic functions are constant, or on which all bounded harmonic functions are constant, or on which all positive harmonic functions are constant, etc.To avoid confusion, call the classification based on metrics of constant curvature the geometric classification, and the one based on degeneracy of function spaces the function-theoretic classification. For example, the Riemann surface consisting of "all complex numbers but 0 and 1" is parabolic in the function-theoretic classification but it is hyperbolic in the geometric classification.