Musical acoustics
Encyclopedia
Musical acoustics or music acoustics is the branch of acoustics
concerned with researching and describing the physics
of music
– how sound
s employed as music work. Examples of areas of study are the function of musical instruments, the human voice
(the physics of speech
and singing
), computer analysis of melody
, and in the clinical use of music in music therapy
.
Whenever two different pitches are played at the same time, their sound waves interact with each other – the highs and lows in the air pressure reinforce each other to produce a different sound wave. As a result, any given sound wave which is more complicated than a sine wave can be modelled by many different sine waves of the appropriate frequencies and amplitudes (a frequency spectrum
). In human
s the hearing
apparatus (composed of the ear
s and brain
) can usually isolate these tones and hear them distinctly. When two or more tones are played at once, a variation of air pressure at the ear "contains" the pitches of each, and the ear and/or brain isolate and decode them into distinct tones.
When the original sound sources are perfectly periodic, the note
consists of several related sine waves (which mathematically add to each other) called the fundamental
and the harmonic
s, partial
s, or overtone
s. The sounds have harmonic
frequency spectra
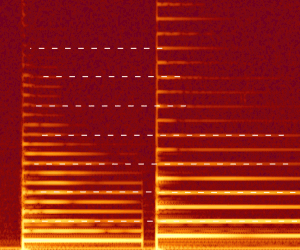
. The lowest frequency present is the fundamental, and is the frequency at which the entire wave vibrates. The overtones vibrate faster than the fundamental, but must vibrate at integer multiples of the fundamental frequency in order for the total wave to be exactly the same each cycle. Real instruments are close to periodic, but the frequencies of the overtones are slightly imperfect, so the shape of the wave changes slightly over time.
against the ear
drum, and the subsequent physical and neurological processing and interpretation, give rise to the subjective experience called sound
. Most sound that people recognize as music
al is dominated by periodic
or regular vibrations rather than non-periodic ones; that is, musical sounds typically have a definite pitch). The transmission of these variations through air is via a sound wave
. In a very simple case, the sound of a sine wave
, which is considered to be the most basic model of a sound waveform, causes the air pressure to increase and decrease in a regular fashion, and is heard as a very pure tone. Pure tones can be produced by tuning fork
s or whistling
. The rate at which the air pressure oscillates is the frequency
of the tone, which is measured in oscillations per second, called hertz
. Frequency is the primary determinant of the perceived pitch
. Frequency of musical instruments can change with altitude due to changes in air pressure.
s. Together they form the harmonic series
.
Overtones which are perfect integer multiples of the fundamental are called harmonic
s. When an overtone is near to being harmonic, but not exact, it is sometimes called a harmonic partial, although they are often referred to simply as harmonics. Sometimes overtones are created that are not anywhere near a harmonic, and are just called partials or inharmonic overtones.
The fundamental frequency is considered the first harmonic and the first partial. The numbering of the partials and harmonics is then usually the same; the second partial is the second harmonic, etc. But if there are inharmonic partials, the numbering no longer coincides. Overtones are numbered as they appear above the fundamental. So strictly speaking, the first overtone is the second partial (and usually the second harmonic). As this can result in confusion, only harmonics are usually referred to by their numbers, and overtones and partials are described by their relationships to those harmonics.
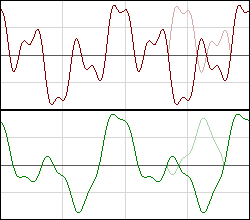When a periodic wave is composed of a fundamental and only odd harmonics (f, 3f, 5f, 7f, ...), the summed wave is half-wave symmetric; it can be inverted and phase shifted and be exactly the same. If the wave has any even harmonics (0f, 2f, 4f, 6f, ...), it will be asymmetrical; the top half will not be a mirror image of the bottom.
Conversely, a system which changes the shape of the wave (beyond simple scaling or shifting) creates additional harmonics (harmonic distortion). This is called a non-linear system. If it affects the wave symmetrically, the harmonics produced will only be odd, if asymmetrically, at least one even harmonic will be produced (and probably also odd).
s that are simple fractions (e.g. 2/1, 3/2 or 5/4), then the composite wave will still be periodic with a short period, and the combination will sound consonant
. For instance, a note vibrating at 200 Hz and a note vibrating at 300 Hz (a perfect fifth
, or 3/2 ratio, above 200 Hz) will add together to make a wave that repeats at 100 Hz: every 1/100 of a second, the 300 Hz wave will repeat thrice and the 200 Hz wave will repeat twice. Note that the total wave repeats at 100 Hz, but there is not actually a 100 Hz sinusoidal component present.
Additionally, the two notes will have many of the same partials. For instance, a note with a fundamental frequency of 200 Hz will have harmonics at: 400, 600, 800, 1000, 1200, …
A note with fundamental frequency of 300 Hz will have harmonics at: 600, 900, 1200, 1500, …
The two notes have the harmonics 600 and 1200 in common, and more will coincide further up the series.
The combination of composite waves with short fundamental frequencies and shared or closely related partials is what causes the sensation of harmony.
When two frequencies are near to a simple fraction, but not exact, the composite wave cycles slowly enough to hear the cancellation of the waves as a steady pulsing instead of a tone. This is called beating
, and is considered to be unpleasant, or dissonant
.
The frequency of beating is calculated as the difference between the frequencies of the two notes. For the example above, |200 Hz - 300 Hz| = 100 Hz. As another example, a combination of 3425 Hz and 3426 Hz would beat once per second (|3425 Hz - 3426 Hz| = 1 Hz). This follows from modulation
theory.
The difference between consonance and dissonance is not clearly defined, but the higher the beat frequency, the more likely the interval to be dissonant. Helmholtz
proposed that maximum dissonance would arise between two pure tones when the beat rate is roughly 35 Hz. http://www.music-cog.ohio-state.edu/Music829B/roughness.html
. Because most people cannot adequately determine absolute
frequencies, the identity of a scale lies in the ratios of frequencies between its tones (known as intervals
).
The diatonic scale
appears in writing throughout history, consisting of seven tones in each octave
. In just intonation
the diatonic scale may be easily constructed using the three simplest intervals within the octave, the perfect fifth
(3/2), perfect fourth
(4/3), and the major third
(5/4). As forms of the fifth and third are naturally present in the overtone series of harmonic resonators, this is a very simple process.
The following table shows the ratios between the frequencies of all the notes of the just major scale
and the fixed frequency of the first note of the scale.
There are other scales available through just intonation, for example the minor scale
. Scales which do not adhere to just intonation, and instead have their intervals adjusted to meet other needs are known as temperaments
, of which equal temperament
is the most used. Temperaments, though they obscure the acoustical purity of just intervals often have other desirable properties, such as a closed circle of fifths
.
Acoustics
Acoustics is the interdisciplinary science that deals with the study of all mechanical waves in gases, liquids, and solids including vibration, sound, ultrasound and infrasound. A scientist who works in the field of acoustics is an acoustician while someone working in the field of acoustics...
concerned with researching and describing the physics
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
of music
Music
Music is an art form whose medium is sound and silence. Its common elements are pitch , rhythm , dynamics, and the sonic qualities of timbre and texture...
– how sound
Sound
Sound is a mechanical wave that is an oscillation of pressure transmitted through a solid, liquid, or gas, composed of frequencies within the range of hearing and of a level sufficiently strong to be heard, or the sensation stimulated in organs of hearing by such vibrations.-Propagation of...
s employed as music work. Examples of areas of study are the function of musical instruments, the human voice
Human voice
The human voice consists of sound made by a human being using the vocal folds for talking, singing, laughing, crying, screaming, etc. Its frequency ranges from about 60 to 7000 Hz. The human voice is specifically that part of human sound production in which the vocal folds are the primary...
(the physics of speech
Interpersonal communication
Interpersonal communication is usually defined by communication scholars in numerous ways, usually describing participants who are dependent upon one another. It...
and singing
Singing
Singing is the act of producing musical sounds with the voice, and augments regular speech by the use of both tonality and rhythm. One who sings is called a singer or vocalist. Singers perform music known as songs that can be sung either with or without accompaniment by musical instruments...
), computer analysis of melody
Melody
A melody , also tune, voice, or line, is a linear succession of musical tones which is perceived as a single entity...
, and in the clinical use of music in music therapy
Music therapy
Music therapy is an allied health profession and one of the expressive therapies, consisting of an interpersonal process in which a trained music therapist uses music and all of its facets—physical, emotional, mental, social, aesthetic, and spiritual—to help clients to improve or maintain their...
.
Methods and fields of study
- Frequency Range of MusicRange (music)In music, the range of a musical instrument is the distance from the lowest to the highest pitch it can play. For a singing voice, the equivalent is vocal range...
- Frequency analysisFrequency analysisIn cryptanalysis, frequency analysis is the study of the frequency of letters or groups of letters in a ciphertext. The method is used as an aid to breaking classical ciphers....
- Computer analysisMusical analysisMusical analysis is the attempt to answer the question how does this music work?. The method employed to answer this question, and indeed exactly what is meant by the question, differs from analyst to analyst, and according to the purpose of the analysis. According to Ian Bent , analysis is "an...
of musical structure - Synthesis of musical sounds
- Music cognitionMusic cognitionMusic cognition is an interdisciplinary approach to understanding the mental processes that support musical behaviors, including perception, comprehension, memory, attention, and performance...
, based on physics (also known as psychoacousticsPsychoacousticsPsychoacoustics is the scientific study of sound perception. More specifically, it is the branch of science studying the psychological and physiological responses associated with sound...
)
Physical aspects
Frequency spectrum
The frequency spectrum of a time-domain signal is a representation of that signal in the frequency domain. The frequency spectrum can be generated via a Fourier transform of the signal, and the resulting values are usually presented as amplitude and phase, both plotted versus frequency.Any signal...
). In human
Human
Humans are the only living species in the Homo genus...
s the hearing
Hearing (sense)
Hearing is the ability to perceive sound by detecting vibrations through an organ such as the ear. It is one of the traditional five senses...
apparatus (composed of the ear
Ear
The ear is the organ that detects sound. It not only receives sound, but also aids in balance and body position. The ear is part of the auditory system....
s and brain
Brain
The brain is the center of the nervous system in all vertebrate and most invertebrate animals—only a few primitive invertebrates such as sponges, jellyfish, sea squirts and starfishes do not have one. It is located in the head, usually close to primary sensory apparatus such as vision, hearing,...
) can usually isolate these tones and hear them distinctly. When two or more tones are played at once, a variation of air pressure at the ear "contains" the pitches of each, and the ear and/or brain isolate and decode them into distinct tones.
When the original sound sources are perfectly periodic, the note
Note
In music, the term note has two primary meanings:#A sign used in musical notation to represent the relative duration and pitch of a sound;#A pitched sound itself....
consists of several related sine waves (which mathematically add to each other) called the fundamental
Fundamental
Fundamental may refer to:* Foundation of reality* Fundamental frequency, as in music or phonetics, often referred to as simply a "fundamental"...
and the harmonic
Harmonic
A harmonic of a wave is a component frequency of the signal that is an integer multiple of the fundamental frequency, i.e. if the fundamental frequency is f, the harmonics have frequencies 2f, 3f, 4f, . . . etc. The harmonics have the property that they are all periodic at the fundamental...
s, partial
Partial
Partial may refer to:*partial derivative, in mathematics** ∂, the partial derivative symbol, often read as "partial"*partial function, in mathematics*partial algorithm, in computer science*part score, in contract bridge...
s, or overtone
Overtone
An overtone is any frequency higher than the fundamental frequency of a sound. The fundamental and the overtones together are called partials. Harmonics are partials whose frequencies are whole number multiples of the fundamental These overlapping terms are variously used when discussing the...
s. The sounds have harmonic
Harmonic
A harmonic of a wave is a component frequency of the signal that is an integer multiple of the fundamental frequency, i.e. if the fundamental frequency is f, the harmonics have frequencies 2f, 3f, 4f, . . . etc. The harmonics have the property that they are all periodic at the fundamental...
frequency spectra
Frequency spectrum
The frequency spectrum of a time-domain signal is a representation of that signal in the frequency domain. The frequency spectrum can be generated via a Fourier transform of the signal, and the resulting values are usually presented as amplitude and phase, both plotted versus frequency.Any signal...
. The lowest frequency present is the fundamental, and is the frequency at which the entire wave vibrates. The overtones vibrate faster than the fundamental, but must vibrate at integer multiples of the fundamental frequency in order for the total wave to be exactly the same each cycle. Real instruments are close to periodic, but the frequencies of the overtones are slightly imperfect, so the shape of the wave changes slightly over time.
Subjective aspects
Variations in air pressurePressure
Pressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
against the ear
Ear
The ear is the organ that detects sound. It not only receives sound, but also aids in balance and body position. The ear is part of the auditory system....
drum, and the subsequent physical and neurological processing and interpretation, give rise to the subjective experience called sound
Sound
Sound is a mechanical wave that is an oscillation of pressure transmitted through a solid, liquid, or gas, composed of frequencies within the range of hearing and of a level sufficiently strong to be heard, or the sensation stimulated in organs of hearing by such vibrations.-Propagation of...
. Most sound that people recognize as music
Music
Music is an art form whose medium is sound and silence. Its common elements are pitch , rhythm , dynamics, and the sonic qualities of timbre and texture...
al is dominated by periodic
Periodic function
In mathematics, a periodic function is a function that repeats its values in regular intervals or periods. The most important examples are the trigonometric functions, which repeat over intervals of length 2π radians. Periodic functions are used throughout science to describe oscillations,...
or regular vibrations rather than non-periodic ones; that is, musical sounds typically have a definite pitch). The transmission of these variations through air is via a sound wave
Wave
In physics, a wave is a disturbance that travels through space and time, accompanied by the transfer of energy.Waves travel and the wave motion transfers energy from one point to another, often with no permanent displacement of the particles of the medium—that is, with little or no associated mass...
. In a very simple case, the sound of a sine wave
Sine wave
The sine wave or sinusoid is a mathematical function that describes a smooth repetitive oscillation. It occurs often in pure mathematics, as well as physics, signal processing, electrical engineering and many other fields...
, which is considered to be the most basic model of a sound waveform, causes the air pressure to increase and decrease in a regular fashion, and is heard as a very pure tone. Pure tones can be produced by tuning fork
Tuning fork
A tuning fork is an acoustic resonator in the form of a two-pronged fork with the prongs formed from a U-shaped bar of elastic metal . It resonates at a specific constant pitch when set vibrating by striking it against a surface or with an object, and emits a pure musical tone after waiting a...
s or whistling
Whistling
Human whistling is the production of sound by means of carefully controlling a stream of air flowing through a small hole. Whistling can be achieved by creating a small opening with one's lips and then blowing or sucking air through the hole...
. The rate at which the air pressure oscillates is the frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
of the tone, which is measured in oscillations per second, called hertz
Hertz
The hertz is the SI unit of frequency defined as the number of cycles per second of a periodic phenomenon. One of its most common uses is the description of the sine wave, particularly those used in radio and audio applications....
. Frequency is the primary determinant of the perceived pitch
Pitch (music)
Pitch is an auditory perceptual property that allows the ordering of sounds on a frequency-related scale.Pitches are compared as "higher" and "lower" in the sense associated with musical melodies,...
. Frequency of musical instruments can change with altitude due to changes in air pressure.
Harmonics, partials, and overtones
The fundamental is the frequency at which the entire wave vibrates. Overtones are other sinusoidal components present at frequencies above the fundamental. All of the frequency components that make up the total waveform, including the fundamental and the overtones, are called partialPartial
Partial may refer to:*partial derivative, in mathematics** ∂, the partial derivative symbol, often read as "partial"*partial function, in mathematics*partial algorithm, in computer science*part score, in contract bridge...
s. Together they form the harmonic series
Harmonic series (music)
Pitched musical instruments are often based on an approximate harmonic oscillator such as a string or a column of air, which oscillates at numerous frequencies simultaneously. At these resonant frequencies, waves travel in both directions along the string or air column, reinforcing and canceling...
.
Overtones which are perfect integer multiples of the fundamental are called harmonic
Harmonic
A harmonic of a wave is a component frequency of the signal that is an integer multiple of the fundamental frequency, i.e. if the fundamental frequency is f, the harmonics have frequencies 2f, 3f, 4f, . . . etc. The harmonics have the property that they are all periodic at the fundamental...
s. When an overtone is near to being harmonic, but not exact, it is sometimes called a harmonic partial, although they are often referred to simply as harmonics. Sometimes overtones are created that are not anywhere near a harmonic, and are just called partials or inharmonic overtones.
The fundamental frequency is considered the first harmonic and the first partial. The numbering of the partials and harmonics is then usually the same; the second partial is the second harmonic, etc. But if there are inharmonic partials, the numbering no longer coincides. Overtones are numbered as they appear above the fundamental. So strictly speaking, the first overtone is the second partial (and usually the second harmonic). As this can result in confusion, only harmonics are usually referred to by their numbers, and overtones and partials are described by their relationships to those harmonics.
Harmonics and non-linearities
Conversely, a system which changes the shape of the wave (beyond simple scaling or shifting) creates additional harmonics (harmonic distortion). This is called a non-linear system. If it affects the wave symmetrically, the harmonics produced will only be odd, if asymmetrically, at least one even harmonic will be produced (and probably also odd).
Harmony
If two notes are simultaneously played, with frequency ratioRatio
In mathematics, a ratio is a relationship between two numbers of the same kind , usually expressed as "a to b" or a:b, sometimes expressed arithmetically as a dimensionless quotient of the two which explicitly indicates how many times the first number contains the second In mathematics, a ratio is...
s that are simple fractions (e.g. 2/1, 3/2 or 5/4), then the composite wave will still be periodic with a short period, and the combination will sound consonant
Consonance
Consonance is a stylistic device, most commonly used in poetry and songs, characterized by the repetition of the same consonant two or more times in short succession, as in "pitter patter" or in "all mammals named Sam are clammy".Consonance should not be confused with assonance, which is the...
. For instance, a note vibrating at 200 Hz and a note vibrating at 300 Hz (a perfect fifth
Perfect fifth
In classical music from Western culture, a fifth is a musical interval encompassing five staff positions , and the perfect fifth is a fifth spanning seven semitones, or in meantone, four diatonic semitones and three chromatic semitones...
, or 3/2 ratio, above 200 Hz) will add together to make a wave that repeats at 100 Hz: every 1/100 of a second, the 300 Hz wave will repeat thrice and the 200 Hz wave will repeat twice. Note that the total wave repeats at 100 Hz, but there is not actually a 100 Hz sinusoidal component present.
Additionally, the two notes will have many of the same partials. For instance, a note with a fundamental frequency of 200 Hz will have harmonics at: 400, 600, 800, 1000, 1200, …
A note with fundamental frequency of 300 Hz will have harmonics at: 600, 900, 1200, 1500, …
The two notes have the harmonics 600 and 1200 in common, and more will coincide further up the series.
The combination of composite waves with short fundamental frequencies and shared or closely related partials is what causes the sensation of harmony.
When two frequencies are near to a simple fraction, but not exact, the composite wave cycles slowly enough to hear the cancellation of the waves as a steady pulsing instead of a tone. This is called beating
Beat (acoustics)
In acoustics, a beat is an interference between two sounds of slightly different frequencies, perceived as periodic variations in volume whose rate is the difference between the two frequencies....
, and is considered to be unpleasant, or dissonant
Consonance and dissonance
In music, a consonance is a harmony, chord, or interval considered stable, as opposed to a dissonance , which is considered to be unstable...
.
The frequency of beating is calculated as the difference between the frequencies of the two notes. For the example above, |200 Hz - 300 Hz| = 100 Hz. As another example, a combination of 3425 Hz and 3426 Hz would beat once per second (|3425 Hz - 3426 Hz| = 1 Hz). This follows from modulation
Modulation
In electronics and telecommunications, modulation is the process of varying one or more properties of a high-frequency periodic waveform, called the carrier signal, with a modulating signal which typically contains information to be transmitted...
theory.
The difference between consonance and dissonance is not clearly defined, but the higher the beat frequency, the more likely the interval to be dissonant. Helmholtz
Hermann von Helmholtz
Hermann Ludwig Ferdinand von Helmholtz was a German physician and physicist who made significant contributions to several widely varied areas of modern science...
proposed that maximum dissonance would arise between two pure tones when the beat rate is roughly 35 Hz. http://www.music-cog.ohio-state.edu/Music829B/roughness.html
Scales
The material of a musical composition is usually taken from a collection of pitches known as a scaleMusical scale
In music, a scale is a sequence of musical notes in ascending and descending order. Most commonly, especially in the context of the common practice period, the notes of a scale will belong to a single key, thus providing material for or being used to conveniently represent part or all of a musical...
. Because most people cannot adequately determine absolute
Absolute pitch
Absolute pitch , widely referred to as perfect pitch, is the ability of a person to identify or re-create a given musical note without the benefit of an external reference.-Definition:...
frequencies, the identity of a scale lies in the ratios of frequencies between its tones (known as intervals
Interval (music)
In music theory, an interval is a combination of two notes, or the ratio between their frequencies. Two-note combinations are also called dyads...
).
The diatonic scale
Diatonic scale
In music theory, a diatonic scale is a seven note, octave-repeating musical scale comprising five whole steps and two half steps for each octave, in which the two half steps are separated from each other by either two or three whole steps...
appears in writing throughout history, consisting of seven tones in each octave
Octave
In music, an octave is the interval between one musical pitch and another with half or double its frequency. The octave relationship is a natural phenomenon that has been referred to as the "basic miracle of music", the use of which is "common in most musical systems"...
. In just intonation
Just intonation
In music, just intonation is any musical tuning in which the frequencies of notes are related by ratios of small whole numbers. Any interval tuned in this way is called a just interval. The two notes in any just interval are members of the same harmonic series...
the diatonic scale may be easily constructed using the three simplest intervals within the octave, the perfect fifth
Perfect fifth
In classical music from Western culture, a fifth is a musical interval encompassing five staff positions , and the perfect fifth is a fifth spanning seven semitones, or in meantone, four diatonic semitones and three chromatic semitones...
(3/2), perfect fourth
Perfect fourth
In classical music from Western culture, a fourth is a musical interval encompassing four staff positions , and the perfect fourth is a fourth spanning five semitones. For example, the ascending interval from C to the next F is a perfect fourth, as the note F lies five semitones above C, and there...
(4/3), and the major third
Major third
In classical music from Western culture, a third is a musical interval encompassing three staff positions , and the major third is one of two commonly occurring thirds. It is qualified as major because it is the largest of the two: the major third spans four semitones, the minor third three...
(5/4). As forms of the fifth and third are naturally present in the overtone series of harmonic resonators, this is a very simple process.
The following table shows the ratios between the frequencies of all the notes of the just major scale
Major scale
In music theory, the major scale or Ionian scale is one of the diatonic scales. It is made up of seven distinct notes, plus an eighth which duplicates the first an octave higher. In solfege these notes correspond to the syllables "Do, Re, Mi, Fa, Sol, La, Ti/Si, ", the "Do" in the parenthesis at...
and the fixed frequency of the first note of the scale.
| C | D | E | F | G | A | B | C |
|---|---|---|---|---|---|---|---|
| 1 | 9/8 | 5/4 | 4/3 | 3/2 | 5/3 | 15/8 | 2 |
There are other scales available through just intonation, for example the minor scale
Minor scale
A minor scale in Western music theory includes any scale that contains, in its tonic triad, at least three essential scale degrees: 1) the tonic , 2) a minor-third, or an interval of a minor third above the tonic, and 3) a perfect-fifth, or an interval of a perfect fifth above the tonic, altogether...
. Scales which do not adhere to just intonation, and instead have their intervals adjusted to meet other needs are known as temperaments
Musical temperament
In musical tuning, a temperament is a system of tuning which slightly compromises the pure intervals of just intonation in order to meet other requirements of the system. Most instruments in modern Western music are tuned in the equal temperament system...
, of which equal temperament
Equal temperament
An equal temperament is a musical temperament, or a system of tuning, in which every pair of adjacent notes has an identical frequency ratio. As pitch is perceived roughly as the logarithm of frequency, this means that the perceived "distance" from every note to its nearest neighbor is the same for...
is the most used. Temperaments, though they obscure the acoustical purity of just intervals often have other desirable properties, such as a closed circle of fifths
Circle of fifths
In music theory, the circle of fifths shows the relationships among the 12 tones of the chromatic scale, their corresponding key signatures, and the associated major and minor keys...
.
See also
- Mathematics of musical scales
- Music therapyMusic therapyMusic therapy is an allied health profession and one of the expressive therapies, consisting of an interpersonal process in which a trained music therapist uses music and all of its facets—physical, emotional, mental, social, aesthetic, and spiritual—to help clients to improve or maintain their...
- Vibrating stringVibrating stringA vibration in a string is a wave. Usually a vibrating string produces a sound whose frequency in most cases is constant. Therefore, since frequency characterizes the pitch, the sound produced is a constant note....
- Open tubeOpen tubeIn the field of acoustics, a tone is created by the periodic vibrations of air. There are several ways in music to create such vibrations. One of these is to use a tube and to blow across the end. This creates a note of a given frequency, depending on the length of the tube and the pressure of the...
- Closed tubeClosed tubeIn the field of acoustics, a tone is created by the periodic vibrations of air applied to a resonator. There are several ways in music to create such vibrations. One of these is to use a closed tube and to blow across the end. This creates a Bernoulli, or "siphon", effect just below the open end or...
- String resonance (music)String resonance (music)String resonance occurs on string instruments. Strings or parts of strings may resonate at their fundamental or overtone frequencies when other strings are sounded...
- 3rd Bridge3rd BridgeThe 3rd bridge is an extended playing technique used on some string instruments , that allows a musician to produce distinctive timbres and overtones that are unavailable on a conventional string instrument with two bridges...
(harmonic resonance based on equal string divisions)
External links
- Physics of music course - links and illustrations - University of Maryland
- Music acoustics - sound files, animations and illustrations - University of New South Wales
- Acoustics collection - descriptions, photos, and video clips of the apparatus for research in musical acoustics by Prof. Dayton MillerDayton MillerDayton Clarence Miller was an American physicist, astronomer, acoustician, and accomplished amateur flautist...
- The Technical Committee on Musical Acoustics (TCMU) of the Acoustical Society of America (ASA)
- The Musical Acoustics Research Library (MARL)
- Acoustics and Music Technology courses - University of Edinburgh
- The music acoustics group at Speech, Music and Hearing KTH
- The physics of harpsichord sound
- Equal beating tuning
- Music & Acoustics
- Sound & Hearing
- Visual music
- Acoustics, audio and video group - University of Salford
- Savart Journal - The open access online journal of science and technology of stringed musical instruments
- Audio Engineering online course under Creative CommonsCreative CommonsCreative Commons is a non-profit organization headquartered in Mountain View, California, United States devoted to expanding the range of creative works available for others to build upon legally and to share. The organization has released several copyright-licenses known as Creative Commons...
Licence