In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, a near-miss Johnson solid is a strictly convex

Convex set

In Euclidean space, an object is convex if for every pair of points within the object, every point on the straight line segment that joins them is also within the object...

 polyhedron

Polyhedron

In elementary geometry a polyhedron is a geometric solid in three dimensions with flat faces and straight edges...

, where every face is a regular or nearly regular polygon

Regular polygon

A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...

, and excluding the 5 Platonic solid

Platonic solid

In geometry, a Platonic solid is a convex polyhedron that is regular, in the sense of a regular polygon. Specifically, the faces of a Platonic solid are congruent regular polygons, with the same number of faces meeting at each vertex; thus, all its edges are congruent, as are its vertices and...

s, the 13 Archimedean solid

Archimedean solid

In geometry an Archimedean solid is a highly symmetric, semi-regular convex polyhedron composed of two or more types of regular polygons meeting in identical vertices...

s, the infinite set of prism

Prism (geometry)

In geometry, a prism is a polyhedron with an n-sided polygonal base, a translated copy , and n other faces joining corresponding sides of the two bases. All cross-sections parallel to the base faces are the same. Prisms are named for their base, so a prism with a pentagonal base is called a...

s, the infinite set of antiprism

Antiprism

In geometry, an n-sided antiprism is a polyhedron composed of two parallel copies of some particular n-sided polygon, connected by an alternating band of triangles...

s, and the 92 Johnson solid

Johnson solid

In geometry, a Johnson solid is a strictly convex polyhedron, each face of which is a regular polygon, but which is not uniform, i.e., not a Platonic solid, Archimedean solid, prism or antiprism. There is no requirement that each face must be the same polygon, or that the same polygons join around...

s.

The set of near-misses is not exactly defined, but can be loosely defined as convex polyhedra that can be approximately constructed from rigid regular polygon faces as a physical model. Because of the "fuzziness" of this definition, the exact number of near-misses is not known.

Examples

These four convex polyhedra can be modelled physically with regular polygon faces with various degrees of adjustment. If they could be built exactly, they would be Johnson solids.

Name	Image	verfs Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...	V	E	F	F3	F4	F5	F6	F8	F10	Symmetry
Truncated triakis tetrahedron Truncated triakis tetrahedron The truncated triakis tetrahedron is a convex polyhedron with 16 faces: 4 sets of 3 pentagons arranged in a tetrahedral arrangement, with 4 hexagons in the gaps. It is constructed from taking a triakis tetrahedron by truncating the order-6 vertices...		4 (5.5.5) 24 (5.5.6)	28	42	16			12	4			Td
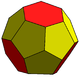
--		6 (5.5.5) 9 (3.5.3.5) 12 (3.3.5.5)	27	51	26	14		12				D3h
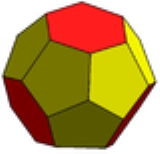
Tetrated dodecahedron Tetrated dodecahedron The tetrated dodecahedron is a near-miss Johnson solid. It was first discovered in 2002 by Alex Doskey. It was then independently rediscovered in 2003 and named by Robert Austin....		4 (5.5.5) 12 (3.5.3.5) 12 (3.3.5.5)	28	54	28	16		12				Td
--		12 (5.5.6) 6 (3.5.3.5) 12 (3.3.5.5)	30	54	26	12		12	2			D6h

Possible vertex figures

The near-misses, like all convex polyhedra made of regular polygons, have a countably infinite set of vertex figure

Vertex figure

In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...

s that they can use, defined by a positive angle defect

Defect (geometry)

In geometry, the defect means the failure of some angles to add up to the expected amount of 360° or 180°, when such angles in the plane would...

. A secondary constraint for the triples requires the angle sum of the two smaller polygons to exceed the angle of the larger one.

The set of polygons that can create convex vertex figures include:

• Triples p.q.r:
  • 3.3.(3-5), 3.4.(4-11), 3.5.(5-7), 3.6.(6+), 3.7.(7-41), 3.8.(8-23), 3.9.(9-17), 3.10.(10-14), 3.11.(11-13), 4.4.(4+), 4.5.(5-19), 4.6.(6-11), 4.7.(7-9), 5.5.(5-9), 5.6.(6-7).
• Quadruples p.q.r.s:
  • 3.3.3.(3+), 3.3.4.(4-11), 3.3.5.(5-7), 3.4.4.(4-5)
• Quintuples p.q.r.s.t:
  • 3.3.3.3.(3-5)

NOTE:

• (a-b) means any polygon for which the number of sides is between a and b inclusive.
• (n+) means any polygon with n or more sides.

Permutation

Permutation

In mathematics, the notion of permutation is used with several slightly different meanings, all related to the act of permuting objects or values. Informally, a permutation of a set of objects is an arrangement of those objects into a particular order...

s of these polygon lists further extend possible vertex figures.

Each vertex figure has an angle defect, and a convex polyhedron will have a combined angle defect of 720 degrees.

These vertex figures and angle defect sums constrain the possible existence of convex polyhedra of regular or near regular polygon faces.

See Vertex configuration for the convex vertex figures used in the regular and semiregular solids.

See also

• Platonic solid
  Platonic solid
  In geometry, a Platonic solid is a convex polyhedron that is regular, in the sense of a regular polygon. Specifically, the faces of a Platonic solid are congruent regular polygons, with the same number of faces meeting at each vertex; thus, all its edges are congruent, as are its vertices and...
  
  
• Semiregular polyhedron
  Semiregular polyhedron
  The term semiregular polyhedron is used variously by different authors.In its original definition, it is a polyhedron with regular faces and a symmetry group which is transitive on its vertices, which is more commonly referred to today as a uniform polyhedron...
  
  
  • Archimedean solid
    Archimedean solid
    In geometry an Archimedean solid is a highly symmetric, semi-regular convex polyhedron composed of two or more types of regular polygons meeting in identical vertices...
    
    
  • prism
    Prism (geometry)
    In geometry, a prism is a polyhedron with an n-sided polygonal base, a translated copy , and n other faces joining corresponding sides of the two bases. All cross-sections parallel to the base faces are the same. Prisms are named for their base, so a prism with a pentagonal base is called a...
    
    
  • antiprism
    Antiprism
    In geometry, an n-sided antiprism is a polyhedron composed of two parallel copies of some particular n-sided polygon, connected by an alternating band of triangles...
    
    
• Johnson solids
• Geodesic dome
  Geodesic dome
  A geodesic dome is a spherical or partial-spherical shell structure or lattice shell based on a network of great circles on the surface of a sphere. The geodesics intersect to form triangular elements that have local triangular rigidity and also distribute the stress across the structure. When...
  
  
• Truncated rhombic dodecahedron
  Truncated rhombic dodecahedron
  The truncated rhombic dodecahedron is a convex polyhedron constructed from the rhombic dodecahedron by truncating the 6 vertices.The 6 vertices are truncated such that all edges are equal length. The original 12 rhombic faces become flattened hexagons, and the truncated vertices become squares.The...
  
  

External links

• Near Misses
• Symmetrohedra: Polyhedra from Symmetric Placement of Regular Polygons
• 24 Johnson Solid Near Misses