Non-uniform circular motion
Encyclopedia
Non-uniform circular motion is any case in which an object moving in a circular path has a varying speed
. Some examples of non-uniform circular motion include a roller coaster, a vertical pendulum
, and a car riding over a hill. All of these situations include an object traveling at different speeds in a circular path. For the roller coaster, its cars travel faster during its trip down than its trip up. The pendulum’s mass swings in a semicircle in which its trip up slows down to 0 m/s and comes back down. A car riding over a hill will experience different speeds at different points of the hill.
. In uniform circular motion, the magnitude of the tangential acceleration
is always equal to zero assuming speed remains constant. The radial acceleration in uniform circular motion is equal to the centripetal acceleration, which is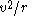
towards the center of the circle. In non-uniform circular motion, the radial
acceleration is the same and equal to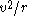
towards the center of the circle. What is different is the tangential acceleration, since speed is non-zero and changing.
Since there is a non-zero tangential acceleration, there are forces that act on an object in addition to its centripetal force
(composed of the mass and radial acceleration). These forces on the object include forces such as weight, normal force
, and other forces acted on the object due to the environment it is in such as friction
.
concepts. For example, in many situations weight is countered by its normal force. When weight points down, normal force points up. In non-uniform circular motion, normal force does not always point in the opposite direction of weight
. Here is an example with an object traveling in a straight path then loops a loop back into a straight path again.
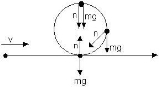
This diagram shows the normal force pointing in other directions rather than opposite to the weight force. The normal force is actually the sum of the radial and tangential forces that help to counteract the weight force and contribute to the centripetal force. The normal force can be broken down into horizontal and vertical components such as in the diagram to the right.
From this diagram, the horizontal component of normal force is what contributes to the centripetal force. The vertical component of the normal force is what counteracts the weight force of the object.
One characteristic that is in non-uniform circular motion that may seem illogical is the fact that normal force and weight can point in the same direction. Both forces can point down, yet the object will remain in a circular path without falling straight down. First let’s see why normal force can point down in the first place. In the first diagram, let's say the object is a person sitting inside a plane, the two forces point down only when it reaches the top of the circle. The reason for this is that the normal force is the sum of the weight and centripetal force. Since both weight and centripetal force points down at the top of the circle, normal force will point down as well. From a logical standpoint, a person who is traveling in the plane will be upside down at the top of the circle. At that moment, the person’s seat is actually pushing down on the person, which is the normal force.
The reason why the object does not fall down when subjected to only downward forces is a simple one. Think about what keeps an object up after it is thrown. Once an object is thrown into the air, there is only the downward force of earth’s gravity that acts on the object. That does not mean that once an object is thrown in the air, it will fall instantly. What keeps that object up in the air is its velocity
. The first of Newton's laws of motion
states that an object’s inertia
keeps it in motion, and since the object in the air has a velocity, it will tend to keep moving in that direction.
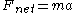
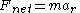
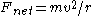
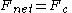
Notice that radial acceleration is used when calculating the total force. Tangential acceleration is not used in calculating total force because it is not responsible for keeping the object in a circular path. The only acceleration responsible for keeping an object moving in a circle is the radial acceleration. Also, keep in mind that since the sum of all forces is equal to the centripetal force, drawing centripetal force into a free body diagram is not necessary and usually not recommended.
Using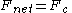
, we can draw free body diagrams to list all the forces acting on an object then set it equal to 
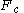. Afterwards, we can solve for what ever is unknown (this can be mass, velocity, radius of curvature, coefficient of friction, normal force, etc.). For example, the visual above showing an object at the top of a semicircle would be expressed as 
.
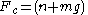In uniform circular motion, total acceleration of an object in a circular path is equal to the radial acceleration. Due to the presence of tangential acceleration in non uniform circular motion, that does not hold true any more. To find the total acceleration of an object in non uniform circular, find the vector sum of the tangential acceleration and the radial acceleration.
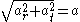
Radial acceleration is always equal to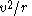
. The tangential acceleration is simply the derivative of the velocity at the given point.
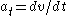
Speed
In kinematics, the speed of an object is the magnitude of its velocity ; it is thus a scalar quantity. The average speed of an object in an interval of time is the distance traveled by the object divided by the duration of the interval; the instantaneous speed is the limit of the average speed as...
. Some examples of non-uniform circular motion include a roller coaster, a vertical pendulum
Pendulum
A pendulum is a weight suspended from a pivot so that it can swing freely. When a pendulum is displaced from its resting equilibrium position, it is subject to a restoring force due to gravity that will accelerate it back toward the equilibrium position...
, and a car riding over a hill. All of these situations include an object traveling at different speeds in a circular path. For the roller coaster, its cars travel faster during its trip down than its trip up. The pendulum’s mass swings in a semicircle in which its trip up slows down to 0 m/s and comes back down. A car riding over a hill will experience different speeds at different points of the hill.
Differences from uniform circular motion
The way to express non-uniform circular motion is not that different from the techniques used in calculating uniform circular motionUniform circular motion
In physics, uniform circular motion describes the motion of a body traversing a circular path at constant speed. The distance of the body from the axis of rotation remains constant at all times. Though the body's speed is constant, its velocity is not constant: velocity, a vector quantity, depends...
. In uniform circular motion, the magnitude of the tangential acceleration
Acceleration
In physics, acceleration is the rate of change of velocity with time. In one dimension, acceleration is the rate at which something speeds up or slows down. However, since velocity is a vector, acceleration describes the rate of change of both the magnitude and the direction of velocity. ...
is always equal to zero assuming speed remains constant. The radial acceleration in uniform circular motion is equal to the centripetal acceleration, which is
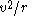towards the center of the circle. In non-uniform circular motion, the radialRadius
In classical geometry, a radius of a circle or sphere is any line segment from its center to its perimeter. By extension, the radius of a circle or sphere is the length of any such segment, which is half the diameter. If the object does not have an obvious center, the term may refer to its...
acceleration is the same and equal to
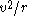towards the center of the circle. What is different is the tangential acceleration, since speed is non-zero and changing.Since there is a non-zero tangential acceleration, there are forces that act on an object in addition to its centripetal force
Centripetal force
Centripetal force is a force that makes a body follow a curved path: it is always directed orthogonal to the velocity of the body, toward the instantaneous center of curvature of the path. The mathematical description was derived in 1659 by Dutch physicist Christiaan Huygens...
(composed of the mass and radial acceleration). These forces on the object include forces such as weight, normal force
Normal force
In mechanics, the normal force F_n\ is the component, perpendicular to the surface of contact, of the contact force exerted on an object by, for example, the surface of a floor or wall, preventing the object from penetrating the surface.The normal force is one of the components of the ground...
, and other forces acted on the object due to the environment it is in such as friction
Friction
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and/or material elements sliding against each other. There are several types of friction:...
.
Important facts
An interesting characteristic of the additional forces that non-uniform circular motion takes into account is that they do not always follow traditional forceForce
In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...
concepts. For example, in many situations weight is countered by its normal force. When weight points down, normal force points up. In non-uniform circular motion, normal force does not always point in the opposite direction of weight
Weight
In science and engineering, the weight of an object is the force on the object due to gravity. Its magnitude , often denoted by an italic letter W, is the product of the mass m of the object and the magnitude of the local gravitational acceleration g; thus:...
. Here is an example with an object traveling in a straight path then loops a loop back into a straight path again.
This diagram shows the normal force pointing in other directions rather than opposite to the weight force. The normal force is actually the sum of the radial and tangential forces that help to counteract the weight force and contribute to the centripetal force. The normal force can be broken down into horizontal and vertical components such as in the diagram to the right.
From this diagram, the horizontal component of normal force is what contributes to the centripetal force. The vertical component of the normal force is what counteracts the weight force of the object.
One characteristic that is in non-uniform circular motion that may seem illogical is the fact that normal force and weight can point in the same direction. Both forces can point down, yet the object will remain in a circular path without falling straight down. First let’s see why normal force can point down in the first place. In the first diagram, let's say the object is a person sitting inside a plane, the two forces point down only when it reaches the top of the circle. The reason for this is that the normal force is the sum of the weight and centripetal force. Since both weight and centripetal force points down at the top of the circle, normal force will point down as well. From a logical standpoint, a person who is traveling in the plane will be upside down at the top of the circle. At that moment, the person’s seat is actually pushing down on the person, which is the normal force.
The reason why the object does not fall down when subjected to only downward forces is a simple one. Think about what keeps an object up after it is thrown. Once an object is thrown into the air, there is only the downward force of earth’s gravity that acts on the object. That does not mean that once an object is thrown in the air, it will fall instantly. What keeps that object up in the air is its velocity
Velocity
In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...
. The first of Newton's laws of motion
Newton's laws of motion
Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...
states that an object’s inertia
Inertia
Inertia is the resistance of any physical object to a change in its state of motion or rest, or the tendency of an object to resist any change in its motion. It is proportional to an object's mass. The principle of inertia is one of the fundamental principles of classical physics which are used to...
keeps it in motion, and since the object in the air has a velocity, it will tend to keep moving in that direction.
Applications involving non-uniform circular motion
Solving applications dealing with non-uniform circular motion involves force analysis. With uniform circular motion, the only force acting upon an object traveling in a circle is the centripetal force. In non-uniform circular motion, there are additional forces acting on the object due to a non-zero tangential acceleration. 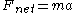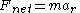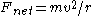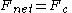Although there are additional forces acting upon the object, the sum of all the forces acting on the object will have to equal to the centripetal force.Notice that radial acceleration is used when calculating the total force. Tangential acceleration is not used in calculating total force because it is not responsible for keeping the object in a circular path. The only acceleration responsible for keeping an object moving in a circle is the radial acceleration. Also, keep in mind that since the sum of all forces is equal to the centripetal force, drawing centripetal force into a free body diagram is not necessary and usually not recommended.
Using
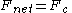, we can draw free body diagrams to list all the forces acting on an object then set it equal to 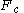. Afterwards, we can solve for what ever is unknown (this can be mass, velocity, radius of curvature, coefficient of friction, normal force, etc.). For example, the visual above showing an object at the top of a semicircle would be expressed as 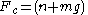.In uniform circular motion, total acceleration of an object in a circular path is equal to the radial acceleration. Due to the presence of tangential acceleration in non uniform circular motion, that does not hold true any more. To find the total acceleration of an object in non uniform circular, find the vector sum of the tangential acceleration and the radial acceleration.
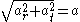
Radial acceleration is always equal to
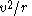. The tangential acceleration is simply the derivative of the velocity at the given point.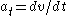See also
- Fictitious forceFictitious forceA fictitious force, also called a pseudo force, d'Alembert force or inertial force, is an apparent force that acts on all masses in a non-inertial frame of reference, such as a rotating reference frame....
- Uniform circular motionUniform circular motionIn physics, uniform circular motion describes the motion of a body traversing a circular path at constant speed. The distance of the body from the axis of rotation remains constant at all times. Though the body's speed is constant, its velocity is not constant: velocity, a vector quantity, depends...
- Centrifugal forceCentrifugal forceCentrifugal force can generally be any force directed outward relative to some origin. More particularly, in classical mechanics, the centrifugal force is an outward force which arises when describing the motion of objects in a rotating reference frame...
- Centripetal forceCentripetal forceCentripetal force is a force that makes a body follow a curved path: it is always directed orthogonal to the velocity of the body, toward the instantaneous center of curvature of the path. The mathematical description was derived in 1659 by Dutch physicist Christiaan Huygens...
- Circular motionCircular motionIn physics, circular motion is rotation along a circular path or a circular orbit. It can be uniform, that is, with constant angular rate of rotation , or non-uniform, that is, with a changing rate of rotation. The rotation around a fixed axis of a three-dimensional body involves circular motion of...