Population inversion
Encyclopedia
In physics
, specifically statistical mechanics
, a population inversion occurs when a system (such as a group of atom
s or molecule
s) exists in state with more members in an excited state
than in lower energy states. The concept is of fundamental importance in laser science
because the production of a population inversion is a necessary step in the workings of a standard laser
.
and the way that light
interacts with matter
. To do so, it is useful to consider a very simple assembly of atoms forming a laser medium
.
Assume there are a group of N atoms, each of which is capable of being in one of two energy
states, either
The number of these atoms which are in the ground state is given by N1, and the number in the excited state N2. Since there are N atoms in total,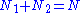
The energy difference between the two states, given by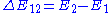 ,
,
determines the characteristic frequency
ν12 of light which will interact with the atoms; This is given by the relation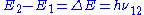 ,
,
h being Planck's constant.
If the group of atoms is in thermal equilibrium
, it can be shown from thermodynamics
that the ratio of the number of atoms in each state is given by a Boltzmann distribution
: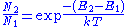 ,
,
where T is the thermodynamic temperature
of the group of atoms, and k is Boltzmann's constant.
We may calculate the ratio of the populations of the two states at room temperature (T ≈ 300 K
) for an energy difference ΔE that corresponds to light of a frequency corresponding to visible light (ν ≈ 5×1014 Hz). In this case ΔE = E2 - E1 ≈ 2.07 eV, and kT ≈ 0.026 eV. Since E2 - E1 ≫ kT, it follows that the argument of the exponential in the equation above is a large negative number, and as such N2/N1 is vanishingly small; i.e., there are almost no atoms in the excited state. When in thermal equilibrium, then, it is seen that the lower energy state is more populated than the higher energy state, and this is the normal state of the system. As T increases, the number of electrons in the high-energy state (N2) increases, but N2 never exceeds N1 for a system at thermal equilibrium; rather, at infinite temperature, the populations N2 and N1 become equal. In other words, a population inversion (N2/N1 > 1) can never exist for a system at thermal equilibrium. To achieve population inversion therefore requires pushing the system into a non-equilibrated state.
s) of frequency
ν12 pass through the group of atoms, there is a possibility of the light being absorbed by atoms which are in the ground state, which will cause them to be excited to the higher energy state. The probability of absorption is proportional
to the radiation intensity of the light, and also to the number of atoms currently in the ground state, N1.
The photons are emitted stochastic
ally, and there is no fixed phase
relationship between photons emitted from a group of excited atoms; in other words, spontaneous emission is incoherent
. In the absence of other processes, the number of atoms in the excited state at time t, is given by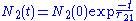 ,
,
where N2(0) is the number of excited atoms at time t = 0, and τ21 is the lifetime of the transition between the two states.
ν21 corresponding to the energy gap ΔE of the excited state to ground state transition. In this case, the excited atom relaxes to the ground state, and is induced to produce a second photon of frequency ν21. The original photon is not absorbed by the atom, and so the result is two photons of the same frequency. This process is known as stimulated emission.
Specifically, an excited atom will act like a small electric dipole which will oscillate with the external field provided. One of the consequences of this oscillation is that it encourages electrons to decay to the lowest energy state. When this happens due to the presence of the electromagnetic field from a photon, a photon is released in the same phase and direction as the "stimulating" photon, and is called stimulated emission.
The rate at which stimulated emission occurs is proportional to the number of atoms N2 in the excited state, and the radiation density of the light. The base probability of a photon causing stimulated emission in a single excited atom was shown by Albert Einstein
to be exactly equal to the probability of a photon being absorbed by an atom in the ground state. Therefore, when the numbers of atoms in the ground and excited states are equal, the rate of stimulated emission is equal to the rate of absorption for a given radiation density.
The critical detail of stimulated emission is that the induced photon has the same frequency
and phase
as the incident photon. In other words, the two photons are coherent
. It is this property that allows optical amplification
, and the production of a laser
system. During the operation of a laser, all three light-matter interactions described above are taking place. Initially, atoms are energized from the ground state to the excited state by a process called pumping, described below. Some of these atoms decay via spontaneous emission, releasing incoherent light as photons of frequency, ν. These photons are fed back into the laser medium, usually by an optical resonator
. Some of these photons are absorbed by the atoms in the ground state, and the photons are lost to the laser process. However, some photons cause stimulated emission in excited-state atoms, releasing another coherent photon. In effect, this results in optical amplification.
If the number of photons being amplified per unit time is greater than the number of photons being absorbed, then the net result is a continuously increasing number of photons being produced; the laser medium is said to have a gain of greater than unity.
Recall from the descriptions of absorption and stimulated emission above that the rates of these two processes are proportional to the number of atoms in the ground and excited states, N1 and N2, respectively. If the ground state has a higher population than the excited state (N1 > N2), the process of absorption dominates and there is a net attenuation of photons. If the populations of the two states are the same (N1 = N2), the rate of absorption of light exactly balances the rate of emission; the medium is then said to be optically transparent.
If the higher energy state has a greater population than the lower energy state (N1 < N2), then the emission process dominates, and light in the system undergoes a net increase in intensity. It is thus clear that to produce a faster rate of stimulated emissions than absorptions, it is required that the ratio of the populations of the two states is such that
N2/N1 > 1; In other words, a population inversion is required for laser operation.
s, which describe the conditions under which a radiative transition is allowed. For instance, transitions are only allowed if ΔS=0, S being the total spin angular momentum of the system. In real materials other effects, such as interactions with the crystal lattice, intervene to circumvent the formal rules. In these systems the forbidden transitions can occur, but usually at slower rates than allowed transitions. A classic example is phosphorescence
where a material has a ground state with S=0, an excited state with S=0, and an intermediate state with S=1. The transition from the intermediate state to the ground state by emission of light is slow because of the selection rules. Thus emission may continue after the external illumination is removed. In contrast fluorescence
in materials is characterized by emission which ceases when the external illumination is removed.
Transitions which do not involve the absorption or emission of radiation are not affected by selection rules. Radiationless transition between levels, such as between the excited S=0 and S=1 states, may proceed quickly enough to siphon off a portion of the S=0 population before it spontaneously returns to the ground state.
The existence of intermediate states in materials, as we will see, is essential to the technique of optical pumping of lasers.
operation, but cannot be achieved in our theoretical group of atoms with two energy-levels when they are in thermal equilibrium. In fact, any method by which the atoms are directly and continuously excited from the ground state to the excited state (such as optical absorption) will eventually reach equilibrium with the de-exciting processes of spontaneous and stimulated emission. At best, an equal population of the two states, N1 = N2 = N/2, can be achieved, resulting in optical transparency but no net optical gain.
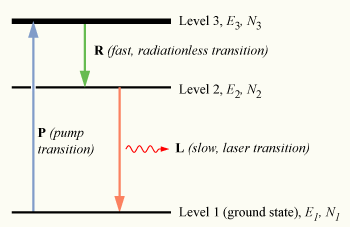 To achieve non-equilibrium conditions, an indirect method of populating the excited state must be used. To understand how this is done, we may use a slightly more realistic model, that of a three-level laser. Again consider a group of N atoms, this time with each atom able to exist in any of three energy states, levels 1, 2 and 3, with energies E1, E2, and E3, and populations N1, N2, and N3, respectively.
To achieve non-equilibrium conditions, an indirect method of populating the excited state must be used. To understand how this is done, we may use a slightly more realistic model, that of a three-level laser. Again consider a group of N atoms, this time with each atom able to exist in any of three energy states, levels 1, 2 and 3, with energies E1, E2, and E3, and populations N1, N2, and N3, respectively.
Note that E1 < E2 < E3; that is, the energy of level 2 lies between that of the ground state and level 3.
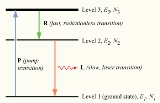
Initially, the system of atoms is at thermal equilibrium, and the majority of the atoms will be in the ground state, i.e., N1 ≈ N, N2 ≈ N3 ≈ 0. If we now subject the atoms to light of a frequency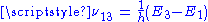 , the process of optical absorption will excite the atoms from the ground state to level 3. This process is called pumping and does not necessarily always directly involve light absorption; other methods of exciting the laser medium, such as electrical discharge or chemical reactions, may be used. The level 3 is sometimes referred to as the pump level or pump band, and the energy transition E1 → E3 as the pump transition, which is shown as the arrow marked P in the diagram on the right.
, the process of optical absorption will excite the atoms from the ground state to level 3. This process is called pumping and does not necessarily always directly involve light absorption; other methods of exciting the laser medium, such as electrical discharge or chemical reactions, may be used. The level 3 is sometimes referred to as the pump level or pump band, and the energy transition E1 → E3 as the pump transition, which is shown as the arrow marked P in the diagram on the right.
If we continue pumping the atoms, we will excite an appreciable number of them into level 3, such that N3 > 0. In a medium suitable for laser operation, we require these excited atoms to quickly decay to level 2. The energy released in this transition may be emitted as a photon (spontaneous emission), however in practice the 3→2 transition (labeled R in the diagram) is usually radiationless, with the energy being transferred to vibrational motion (heat
) of the host material surrounding the atoms, without the generation of a photon.
An atom in level 2 may decay by spontaneous emission to the ground state, releasing a photon of frequency ν12 (given by E2 – E1 = hν12), which is shown as the transition L, called the laser transition in the diagram. If the lifetime of this transition, τ21 is much longer than the lifetime of the radiationless 3 → 2 transition τ32 (if τ21 ≫ τ32, known as a favourable lifetime ratio), the population of the E3 will be essentially zero (N3 ≈ 0) and a population of excited state atoms will accumulate in level 2 (N2 > 0). If over half the N atoms can be accumulated in this state, this will exceed the population of the ground state N1. A population inversion (N2 > N1 ) has thus been achieved between level 1 and 2, and optical amplification at the frequency ν21 can be obtained.
Because at least half the population of atoms must be excited from the ground state to obtain a population inversion, the laser medium must be very strongly pumped. This makes three-level lasers rather inefficient, despite being the first type of laser to be discovered (based on a ruby
laser medium, by Theodore Maiman in 1960). A three-level system could also have a radiative transition between level 3 and 2, and a non-radiative transition between 2 and 1. In this case, the pumping requirements are weaker. In practice, most lasers are four-level lasers, described below.
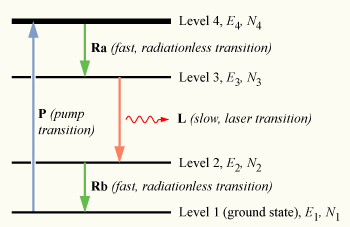 Here, there are four energy levels, energies E1, E2, E3, E4, and populations N1, N2, N3, N4, respectively. The energies of each level are such that E1 < E2 < E3 < E4.
Here, there are four energy levels, energies E1, E2, E3, E4, and populations N1, N2, N3, N4, respectively. The energies of each level are such that E1 < E2 < E3 < E4.
In this system, the pumping transition P excites the atoms in the ground state (level 1) into the pump band (level 4). From level 4, the atoms again decay by a fast, non-radiative transition Ra into the level 3. Since the lifetime of the laser transition L is long compared to that of Ra (τ32 ≫ τ43), a population accumulates in level 3 (the upper laser level), which may relax by spontaneous or stimulated emission into level 2 (the lower laser level). This level likewise has a fast, non-radiative decay Rb into the ground state.
As before, the presence of a fast, radiationless decay transitions result in population of the pump band being quickly depleted (N4 ≈ 0). In a four-level system, any atom in the lower laser level E2 is also quickly de-excited, leading to a negligible population in that state (N2 ≈ 0). This is important, since any appreciable population accumulating in level 3, the upper laser level, will form a population inversion with respect to level 2. That is, as long as N3 > 0, then N3 > N2 and a population inversion is achieved. Thus optical amplification, and laser operation, can take place at a frequency of ν32 (E3-E2 = hν32).
Since only a few atoms must be excited into the upper laser level to form a population inversion, a four-level laser is much more efficient than a three-level one, and most practical lasers are of this type. In reality, many more than four energy levels may be involved in the laser process, with complex excitation and relaxation processes involved between these levels. In particular, the pump band may consist of several distinct energy levels, or a continuum of levels, which allow optical pumping of the medium over a wide range of wavelengths.
Note that in both three- and four-level lasers, the energy of the pumping transition is greater than that of the laser transition. This means that, if the laser is optically pumped, the frequency of the pumping light must be greater than that of the resulting laser light. In other words, the pump wavelength is shorter than the laser wavelength. It is possible in some media to use multiple photon absorptions between multiple lower-energy transitions to reach the pump level; such lasers are called up-conversion lasers.
While in many lasers the laser process involves the transition of atoms between different electron
ic energy states, as described in the model above, this is not the only mechanism that can result in laser action. For example, there are many common lasers (e.g., dye lasers, carbon dioxide laser
s) where the laser medium consists of complete molecules, and energy states correspond to vibrational and rotational modes of oscillation of the molecules. This is the case with water maser
s, that occur in nature.
In some media it is possible, by imposing an additional optical or microwave field, to use quantum coherence effects to reduce the likelihood of an excited-state to ground-state transition. This technique, known as lasing without inversion, allows optical amplification to take place without producing a population inversion between the two states.
for Microwave Amplification by Stimulated Emission of Radiation. In the microwave region, the Boltzmann distribution of molecules among energy states is such that, at room temperature all states are populated almost equally.
To create a population inversion under these conditions, it is necessary to selectively remove some atoms or molecules from the system based on differences in properties. For instance, in a hydrogen Maser, the well-known "21cm wave" transition in atomic hydrogen, where the lone electron flips its spin state from parallel to the nuclear spin to antiparallel, can be used to create a population inversion because the parallel state has a magnetic moment and the antiparallel state does not. A strong inhomogeneous magnetic field will separate out atoms in the higher energy state from a beam of mixed state atoms. The separated population represents a population inversion which can exhibit stimulated emissions.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, specifically statistical mechanics
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
, a population inversion occurs when a system (such as a group of atom
Atom
The atom is a basic unit of matter that consists of a dense central nucleus surrounded by a cloud of negatively charged electrons. The atomic nucleus contains a mix of positively charged protons and electrically neutral neutrons...
s or molecule
Molecule
A molecule is an electrically neutral group of at least two atoms held together by covalent chemical bonds. Molecules are distinguished from ions by their electrical charge...
s) exists in state with more members in an excited state
Excited state
Excitation is an elevation in energy level above an arbitrary baseline energy state. In physics there is a specific technical definition for energy level which is often associated with an atom being excited to an excited state....
than in lower energy states. The concept is of fundamental importance in laser science
Laser science
Laser science or laser physics is a branch of optics that describes the theory and practice of lasers.Laser science is principally concerned with quantum electronics, laser construction, optical cavity design, the physics of producing a population inversion in laser media, and the temporal...
because the production of a population inversion is a necessary step in the workings of a standard laser
Laser
A laser is a device that emits light through a process of optical amplification based on the stimulated emission of photons. The term "laser" originated as an acronym for Light Amplification by Stimulated Emission of Radiation...
.
Boltzmann distributions and thermal equilibrium
To understand the concept of a population inversion, it is necessary to understand some thermodynamicsThermodynamics
Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...
and the way that light
Light
Light or visible light is electromagnetic radiation that is visible to the human eye, and is responsible for the sense of sight. Visible light has wavelength in a range from about 380 nanometres to about 740 nm, with a frequency range of about 405 THz to 790 THz...
interacts with matter
Matter
Matter is a general term for the substance of which all physical objects consist. Typically, matter includes atoms and other particles which have mass. A common way of defining matter is as anything that has mass and occupies volume...
. To do so, it is useful to consider a very simple assembly of atoms forming a laser medium
Active laser medium
The active laser medium is the source of optical gain within a laser. The gain results from the stimulated emission of electronic or molecular transitions to a lower energy state from a higher energy state...
.
Assume there are a group of N atoms, each of which is capable of being in one of two energy
Energy
In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
states, either
- The ground state, with energy E1; or
- The excited state, with energy E2, with E2 > E1.
The number of these atoms which are in the ground state is given by N1, and the number in the excited state N2. Since there are N atoms in total,
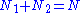
The energy difference between the two states, given by
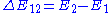 ,
,determines the characteristic frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
ν12 of light which will interact with the atoms; This is given by the relation
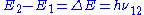 ,
,h being Planck's constant.
If the group of atoms is in thermal equilibrium
Thermal equilibrium
Thermal equilibrium is a theoretical physical concept, used especially in theoretical texts, that means that all temperatures of interest are unchanging in time and uniform in space...
, it can be shown from thermodynamics
Thermodynamics
Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...
that the ratio of the number of atoms in each state is given by a Boltzmann distribution
Boltzmann distribution
In chemistry, physics, and mathematics, the Boltzmann distribution is a certain distribution function or probability measure for the distribution of the states of a system. It underpins the concept of the canonical ensemble, providing its underlying distribution...
:
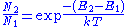 ,
,where T is the thermodynamic temperature
Thermodynamic temperature
Thermodynamic temperature is the absolute measure of temperature and is one of the principal parameters of thermodynamics. Thermodynamic temperature is an "absolute" scale because it is the measure of the fundamental property underlying temperature: its null or zero point, absolute zero, is the...
of the group of atoms, and k is Boltzmann's constant.
We may calculate the ratio of the populations of the two states at room temperature (T ≈ 300 K
Kelvin
The kelvin is a unit of measurement for temperature. It is one of the seven base units in the International System of Units and is assigned the unit symbol K. The Kelvin scale is an absolute, thermodynamic temperature scale using as its null point absolute zero, the temperature at which all...
) for an energy difference ΔE that corresponds to light of a frequency corresponding to visible light (ν ≈ 5×1014 Hz). In this case ΔE = E2 - E1 ≈ 2.07 eV, and kT ≈ 0.026 eV. Since E2 - E1 ≫ kT, it follows that the argument of the exponential in the equation above is a large negative number, and as such N2/N1 is vanishingly small; i.e., there are almost no atoms in the excited state. When in thermal equilibrium, then, it is seen that the lower energy state is more populated than the higher energy state, and this is the normal state of the system. As T increases, the number of electrons in the high-energy state (N2) increases, but N2 never exceeds N1 for a system at thermal equilibrium; rather, at infinite temperature, the populations N2 and N1 become equal. In other words, a population inversion (N2/N1 > 1) can never exist for a system at thermal equilibrium. To achieve population inversion therefore requires pushing the system into a non-equilibrated state.
The interaction of light with matter
There are three types of possible interactions between a system of atoms and light that are of interest:Absorption
If light (photonPhoton
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
s) of frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
ν12 pass through the group of atoms, there is a possibility of the light being absorbed by atoms which are in the ground state, which will cause them to be excited to the higher energy state. The probability of absorption is proportional
Proportionality (mathematics)
In mathematics, two variable quantities are proportional if one of them is always the product of the other and a constant quantity, called the coefficient of proportionality or proportionality constant. In other words, are proportional if the ratio \tfrac yx is constant. We also say that one...
to the radiation intensity of the light, and also to the number of atoms currently in the ground state, N1.
Spontaneous emission
If a collection of atoms are in the excited state, spontaneous decay events to the ground state will occur at a rate proportional to N2, the number of atoms in the excited state. The energy difference between the two states ΔE21 is emitted from the atom as a photon of frequency ν21 as given by the frequency-energy relation above.The photons are emitted stochastic
Stochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
ally, and there is no fixed phase
Phase (waves)
Phase in waves is the fraction of a wave cycle which has elapsed relative to an arbitrary point.-Formula:The phase of an oscillation or wave refers to a sinusoidal function such as the following:...
relationship between photons emitted from a group of excited atoms; in other words, spontaneous emission is incoherent
Coherence (physics)
In physics, coherence is a property of waves that enables stationary interference. More generally, coherence describes all properties of the correlation between physical quantities of a wave....
. In the absence of other processes, the number of atoms in the excited state at time t, is given by
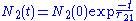 ,
,where N2(0) is the number of excited atoms at time t = 0, and τ21 is the lifetime of the transition between the two states.
Stimulated emission
If an atom is already in the excited state, it may be perturbed by the passage of a photon that has a frequencyFrequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
ν21 corresponding to the energy gap ΔE of the excited state to ground state transition. In this case, the excited atom relaxes to the ground state, and is induced to produce a second photon of frequency ν21. The original photon is not absorbed by the atom, and so the result is two photons of the same frequency. This process is known as stimulated emission.
Specifically, an excited atom will act like a small electric dipole which will oscillate with the external field provided. One of the consequences of this oscillation is that it encourages electrons to decay to the lowest energy state. When this happens due to the presence of the electromagnetic field from a photon, a photon is released in the same phase and direction as the "stimulating" photon, and is called stimulated emission.
The rate at which stimulated emission occurs is proportional to the number of atoms N2 in the excited state, and the radiation density of the light. The base probability of a photon causing stimulated emission in a single excited atom was shown by Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
to be exactly equal to the probability of a photon being absorbed by an atom in the ground state. Therefore, when the numbers of atoms in the ground and excited states are equal, the rate of stimulated emission is equal to the rate of absorption for a given radiation density.
The critical detail of stimulated emission is that the induced photon has the same frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
and phase
Phase (waves)
Phase in waves is the fraction of a wave cycle which has elapsed relative to an arbitrary point.-Formula:The phase of an oscillation or wave refers to a sinusoidal function such as the following:...
as the incident photon. In other words, the two photons are coherent
Coherence (physics)
In physics, coherence is a property of waves that enables stationary interference. More generally, coherence describes all properties of the correlation between physical quantities of a wave....
. It is this property that allows optical amplification
Optical amplifier
An optical amplifier is a device that amplifies an optical signal directly, without the need to first convert it to an electrical signal. An optical amplifier may be thought of as a laser without an optical cavity, or one in which feedback from the cavity is suppressed...
, and the production of a laser
Laser
A laser is a device that emits light through a process of optical amplification based on the stimulated emission of photons. The term "laser" originated as an acronym for Light Amplification by Stimulated Emission of Radiation...
system. During the operation of a laser, all three light-matter interactions described above are taking place. Initially, atoms are energized from the ground state to the excited state by a process called pumping, described below. Some of these atoms decay via spontaneous emission, releasing incoherent light as photons of frequency, ν. These photons are fed back into the laser medium, usually by an optical resonator
Laser construction
A laser is constructed from three principal parts:*An energy source ,*A gain medium or laser medium, and*Two or more mirrors that form an optical resonator.-Pump source:...
. Some of these photons are absorbed by the atoms in the ground state, and the photons are lost to the laser process. However, some photons cause stimulated emission in excited-state atoms, releasing another coherent photon. In effect, this results in optical amplification.
If the number of photons being amplified per unit time is greater than the number of photons being absorbed, then the net result is a continuously increasing number of photons being produced; the laser medium is said to have a gain of greater than unity.
Recall from the descriptions of absorption and stimulated emission above that the rates of these two processes are proportional to the number of atoms in the ground and excited states, N1 and N2, respectively. If the ground state has a higher population than the excited state (N1 > N2), the process of absorption dominates and there is a net attenuation of photons. If the populations of the two states are the same (N1 = N2), the rate of absorption of light exactly balances the rate of emission; the medium is then said to be optically transparent.
If the higher energy state has a greater population than the lower energy state (N1 < N2), then the emission process dominates, and light in the system undergoes a net increase in intensity. It is thus clear that to produce a faster rate of stimulated emissions than absorptions, it is required that the ratio of the populations of the two states is such that
N2/N1 > 1; In other words, a population inversion is required for laser operation.
Selection rules
Many transitions involving electromagnetic radiation are strictly forbidden under quantum mechanics. The allowed transitions are described by so-called selection ruleSelection rule
In physics and chemistry a selection rule, or transition rule, formally constrains the possible transitions of a system from one state to another. Selection rules have been derived for electronic, vibrational, and rotational transitions...
s, which describe the conditions under which a radiative transition is allowed. For instance, transitions are only allowed if ΔS=0, S being the total spin angular momentum of the system. In real materials other effects, such as interactions with the crystal lattice, intervene to circumvent the formal rules. In these systems the forbidden transitions can occur, but usually at slower rates than allowed transitions. A classic example is phosphorescence
Phosphorescence
Phosphorescence is a specific type of photoluminescence related to fluorescence. Unlike fluorescence, a phosphorescent material does not immediately re-emit the radiation it absorbs. The slower time scales of the re-emission are associated with "forbidden" energy state transitions in quantum...
where a material has a ground state with S=0, an excited state with S=0, and an intermediate state with S=1. The transition from the intermediate state to the ground state by emission of light is slow because of the selection rules. Thus emission may continue after the external illumination is removed. In contrast fluorescence
Fluorescence
Fluorescence is the emission of light by a substance that has absorbed light or other electromagnetic radiation of a different wavelength. It is a form of luminescence. In most cases, emitted light has a longer wavelength, and therefore lower energy, than the absorbed radiation...
in materials is characterized by emission which ceases when the external illumination is removed.
Transitions which do not involve the absorption or emission of radiation are not affected by selection rules. Radiationless transition between levels, such as between the excited S=0 and S=1 states, may proceed quickly enough to siphon off a portion of the S=0 population before it spontaneously returns to the ground state.
The existence of intermediate states in materials, as we will see, is essential to the technique of optical pumping of lasers.
Creating a population inversion
As described above, a population inversion is required for laserLaser
A laser is a device that emits light through a process of optical amplification based on the stimulated emission of photons. The term "laser" originated as an acronym for Light Amplification by Stimulated Emission of Radiation...
operation, but cannot be achieved in our theoretical group of atoms with two energy-levels when they are in thermal equilibrium. In fact, any method by which the atoms are directly and continuously excited from the ground state to the excited state (such as optical absorption) will eventually reach equilibrium with the de-exciting processes of spontaneous and stimulated emission. At best, an equal population of the two states, N1 = N2 = N/2, can be achieved, resulting in optical transparency but no net optical gain.
Three-level lasers

Note that E1 < E2 < E3; that is, the energy of level 2 lies between that of the ground state and level 3.
Initially, the system of atoms is at thermal equilibrium, and the majority of the atoms will be in the ground state, i.e., N1 ≈ N, N2 ≈ N3 ≈ 0. If we now subject the atoms to light of a frequency
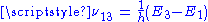 , the process of optical absorption will excite the atoms from the ground state to level 3. This process is called pumping and does not necessarily always directly involve light absorption; other methods of exciting the laser medium, such as electrical discharge or chemical reactions, may be used. The level 3 is sometimes referred to as the pump level or pump band, and the energy transition E1 → E3 as the pump transition, which is shown as the arrow marked P in the diagram on the right.
, the process of optical absorption will excite the atoms from the ground state to level 3. This process is called pumping and does not necessarily always directly involve light absorption; other methods of exciting the laser medium, such as electrical discharge or chemical reactions, may be used. The level 3 is sometimes referred to as the pump level or pump band, and the energy transition E1 → E3 as the pump transition, which is shown as the arrow marked P in the diagram on the right.If we continue pumping the atoms, we will excite an appreciable number of them into level 3, such that N3 > 0. In a medium suitable for laser operation, we require these excited atoms to quickly decay to level 2. The energy released in this transition may be emitted as a photon (spontaneous emission), however in practice the 3→2 transition (labeled R in the diagram) is usually radiationless, with the energy being transferred to vibrational motion (heat
Heat
In physics and thermodynamics, heat is energy transferred from one body, region, or thermodynamic system to another due to thermal contact or thermal radiation when the systems are at different temperatures. It is often described as one of the fundamental processes of energy transfer between...
) of the host material surrounding the atoms, without the generation of a photon.
An atom in level 2 may decay by spontaneous emission to the ground state, releasing a photon of frequency ν12 (given by E2 – E1 = hν12), which is shown as the transition L, called the laser transition in the diagram. If the lifetime of this transition, τ21 is much longer than the lifetime of the radiationless 3 → 2 transition τ32 (if τ21 ≫ τ32, known as a favourable lifetime ratio), the population of the E3 will be essentially zero (N3 ≈ 0) and a population of excited state atoms will accumulate in level 2 (N2 > 0). If over half the N atoms can be accumulated in this state, this will exceed the population of the ground state N1. A population inversion (N2 > N1 ) has thus been achieved between level 1 and 2, and optical amplification at the frequency ν21 can be obtained.
Because at least half the population of atoms must be excited from the ground state to obtain a population inversion, the laser medium must be very strongly pumped. This makes three-level lasers rather inefficient, despite being the first type of laser to be discovered (based on a ruby
Ruby
A ruby is a pink to blood-red colored gemstone, a variety of the mineral corundum . The red color is caused mainly by the presence of the element chromium. Its name comes from ruber, Latin for red. Other varieties of gem-quality corundum are called sapphires...
laser medium, by Theodore Maiman in 1960). A three-level system could also have a radiative transition between level 3 and 2, and a non-radiative transition between 2 and 1. In this case, the pumping requirements are weaker. In practice, most lasers are four-level lasers, described below.
Four-level lasers

In this system, the pumping transition P excites the atoms in the ground state (level 1) into the pump band (level 4). From level 4, the atoms again decay by a fast, non-radiative transition Ra into the level 3. Since the lifetime of the laser transition L is long compared to that of Ra (τ32 ≫ τ43), a population accumulates in level 3 (the upper laser level), which may relax by spontaneous or stimulated emission into level 2 (the lower laser level). This level likewise has a fast, non-radiative decay Rb into the ground state.
As before, the presence of a fast, radiationless decay transitions result in population of the pump band being quickly depleted (N4 ≈ 0). In a four-level system, any atom in the lower laser level E2 is also quickly de-excited, leading to a negligible population in that state (N2 ≈ 0). This is important, since any appreciable population accumulating in level 3, the upper laser level, will form a population inversion with respect to level 2. That is, as long as N3 > 0, then N3 > N2 and a population inversion is achieved. Thus optical amplification, and laser operation, can take place at a frequency of ν32 (E3-E2 = hν32).
Since only a few atoms must be excited into the upper laser level to form a population inversion, a four-level laser is much more efficient than a three-level one, and most practical lasers are of this type. In reality, many more than four energy levels may be involved in the laser process, with complex excitation and relaxation processes involved between these levels. In particular, the pump band may consist of several distinct energy levels, or a continuum of levels, which allow optical pumping of the medium over a wide range of wavelengths.
Note that in both three- and four-level lasers, the energy of the pumping transition is greater than that of the laser transition. This means that, if the laser is optically pumped, the frequency of the pumping light must be greater than that of the resulting laser light. In other words, the pump wavelength is shorter than the laser wavelength. It is possible in some media to use multiple photon absorptions between multiple lower-energy transitions to reach the pump level; such lasers are called up-conversion lasers.
While in many lasers the laser process involves the transition of atoms between different electron
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
ic energy states, as described in the model above, this is not the only mechanism that can result in laser action. For example, there are many common lasers (e.g., dye lasers, carbon dioxide laser
Carbon dioxide laser
The carbon dioxide laser was one of the earliest gas lasers to be developed , and is still one of the most useful. Carbon dioxide lasers are the highest-power continuous wave lasers that are currently available...
s) where the laser medium consists of complete molecules, and energy states correspond to vibrational and rotational modes of oscillation of the molecules. This is the case with water maser
Maser
A maser is a device that produces coherent electromagnetic waves through amplification by stimulated emission. Historically, “maser” derives from the original, upper-case acronym MASER, which stands for "Microwave Amplification by Stimulated Emission of Radiation"...
s, that occur in nature.
In some media it is possible, by imposing an additional optical or microwave field, to use quantum coherence effects to reduce the likelihood of an excited-state to ground-state transition. This technique, known as lasing without inversion, allows optical amplification to take place without producing a population inversion between the two states.
Other methods of creating a population inversion
Stimulated emission was first observed in the microwave region of the electromagnetic spectrum, giving rise to the acronym MASERMaser
A maser is a device that produces coherent electromagnetic waves through amplification by stimulated emission. Historically, “maser” derives from the original, upper-case acronym MASER, which stands for "Microwave Amplification by Stimulated Emission of Radiation"...
for Microwave Amplification by Stimulated Emission of Radiation. In the microwave region, the Boltzmann distribution of molecules among energy states is such that, at room temperature all states are populated almost equally.
To create a population inversion under these conditions, it is necessary to selectively remove some atoms or molecules from the system based on differences in properties. For instance, in a hydrogen Maser, the well-known "21cm wave" transition in atomic hydrogen, where the lone electron flips its spin state from parallel to the nuclear spin to antiparallel, can be used to create a population inversion because the parallel state has a magnetic moment and the antiparallel state does not. A strong inhomogeneous magnetic field will separate out atoms in the higher energy state from a beam of mixed state atoms. The separated population represents a population inversion which can exhibit stimulated emissions.

