
Septic equation
Encyclopedia
In mathematics
, a septic equation, heptic equation or septimic equation is a polynomial
equation
of degree
seven. It is of the form:

The coefficients are members of a field
(typically the rational number
s, the real number
s or the complex number
s), and a ≠ 0.
Because they have an odd degree, normal septic functions appear similar to normal quintic or cubic function
when graphed, except they may possess an additional local maximum
and local minimum each. The derivative
of a septic function is a sextic function.
developed techniques for determining whether a given equation could be solved by radicals which gave rise to the field of Galois theory
. To give an example of an irreducible but solvable septic, one can generalize the solvable de Moivre quintic to get, ,
,
where the auxiliary equation is .
.
This means that the septic is obtained by eliminating u and v between
 ,
,  and
and  .
.
It follows that that the septic's seven roots are given by

where ωk is any of the seven 7th roots of unity
. The Galois group
of this septic is the maximal solvable group of order 42. This is easily generalized to any other degrees k, not necessarily prime.
Another solvable family is,

whose members appear in Kluner's "Database of Number Fields". Its discriminant is,

Note that d = -467 has class number
h(d) = 7. The Galois group
of these septics is the dihedral group
of order 14.
The general septic equation can be solved with the alternating or symmetric
Galois group
s A7 or S7. Such equations require hyperelliptic functions and associated theta functions of genus
3 for their solution. However, these equations were not studied specifically by the nineteenth-century mathematicians studying the solutions of algebraic equations, because the sextic equation
s' solutions were already at the limits of their computational abilities without computers.
Septics are the lowest order equations for which it is not obvious that their solutions may be obtained by superimposing continuous functions of two variables. Hilbert's 13th problem
was the conjecture this was not possible in the general case for seventh-degree equations. Vladimir Arnold
solved this in 1957, demonstrating that this was always possible. However, Arnold himself considered the genuine Hilbert problem to be whether the solutions of septics may be obtained by superimposing algebraic functions of two variables (the problem still being open).
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a septic equation, heptic equation or septimic equation is a polynomial
Polynomial
In mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...
equation
Equation
An equation is a mathematical statement that asserts the equality of two expressions. In modern notation, this is written by placing the expressions on either side of an equals sign , for examplex + 3 = 5\,asserts that x+3 is equal to 5...
of degree
Degree of a polynomial
The degree of a polynomial represents the highest degree of a polynominal's terms , should the polynomial be expressed in canonical form . The degree of an individual term is the sum of the exponents acting on the term's variables...
seven. It is of the form:

The coefficients are members of a field
Field (mathematics)
In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
(typically the rational number
Rational number
In mathematics, a rational number is any number that can be expressed as the quotient or fraction a/b of two integers, with the denominator b not equal to zero. Since b may be equal to 1, every integer is a rational number...
s, the real number
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
s or the complex number
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
s), and a ≠ 0.
Because they have an odd degree, normal septic functions appear similar to normal quintic or cubic function
Cubic function
In mathematics, a cubic function is a function of the formf=ax^3+bx^2+cx+d,\,where a is nonzero; or in other words, a polynomial of degree three. The derivative of a cubic function is a quadratic function...
when graphed, except they may possess an additional local maximum
Maxima and minima
In mathematics, the maximum and minimum of a function, known collectively as extrema , are the largest and smallest value that the function takes at a point either within a given neighborhood or on the function domain in its entirety .More generally, the...
and local minimum each. The derivative
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
of a septic function is a sextic function.
Solvable septics
Some seventh degree equations can be solved by factorizing into radicals, but other septics cannot. Évariste GaloisÉvariste Galois
Évariste Galois was a French mathematician born in Bourg-la-Reine. While still in his teens, he was able to determine a necessary and sufficient condition for a polynomial to be solvable by radicals, thereby solving a long-standing problem...
developed techniques for determining whether a given equation could be solved by radicals which gave rise to the field of Galois theory
Galois theory
In mathematics, more specifically in abstract algebra, Galois theory, named after Évariste Galois, provides a connection between field theory and group theory...
. To give an example of an irreducible but solvable septic, one can generalize the solvable de Moivre quintic to get,
 ,
,where the auxiliary equation is
 .
.This means that the septic is obtained by eliminating u and v between
 ,
,  and
and  .
.It follows that that the septic's seven roots are given by

where ωk is any of the seven 7th roots of unity
Root of unity
In mathematics, a root of unity, or de Moivre number, is any complex number that equals 1 when raised to some integer power n. Roots of unity are used in many branches of mathematics, and are especially important in number theory, the theory of group characters, field theory, and the discrete...
. The Galois group
Galois group
In mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
of this septic is the maximal solvable group of order 42. This is easily generalized to any other degrees k, not necessarily prime.
Another solvable family is,

whose members appear in Kluner's "Database of Number Fields". Its discriminant is,

Note that d = -467 has class number
Class number
In mathematics, class number may refer to* Class number , the number of conjugacy classes of a group*The size of the ideal class group of a number ring*The number of equivalence classes of binary quadratic forms of a given discriminant...
h(d) = 7. The Galois group
Galois group
In mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
of these septics is the dihedral group
Dihedral group
In mathematics, a dihedral group is the group of symmetries of a regular polygon, including both rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry.See also: Dihedral symmetry in three...
of order 14.
The general septic equation can be solved with the alternating or symmetric
Symmetric group
In mathematics, the symmetric group Sn on a finite set of n symbols is the group whose elements are all the permutations of the n symbols, and whose group operation is the composition of such permutations, which are treated as bijective functions from the set of symbols to itself...
Galois group
Galois group
In mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
s A7 or S7. Such equations require hyperelliptic functions and associated theta functions of genus
Genus (mathematics)
In mathematics, genus has a few different, but closely related, meanings:-Orientable surface:The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It...
3 for their solution. However, these equations were not studied specifically by the nineteenth-century mathematicians studying the solutions of algebraic equations, because the sextic equation
Sextic equation
In mathematics, a sextic equation is a polynomial equation of degree six. It is of the form:ax^6+bx^5+cx^4+dx^3+ex^2+fx+g=0,\,where a \neq 0....
s' solutions were already at the limits of their computational abilities without computers.
Septics are the lowest order equations for which it is not obvious that their solutions may be obtained by superimposing continuous functions of two variables. Hilbert's 13th problem
Hilbert's thirteenth problem
Hilbert's thirteenth problem is one of the 23 Hilbert problems set out in a celebrated list compiled in 1900 by David Hilbert. It entails proving whether or not a solution exists for all 7th-degree equations using functions of two arguments...
was the conjecture this was not possible in the general case for seventh-degree equations. Vladimir Arnold
Vladimir Arnold
Vladimir Igorevich Arnold was a Soviet and Russian mathematician. While he is best known for the Kolmogorov–Arnold–Moser theorem regarding the stability of integrable Hamiltonian systems, he made important contributions in several areas including dynamical systems theory, catastrophe theory,...
solved this in 1957, demonstrating that this was always possible. However, Arnold himself considered the genuine Hilbert problem to be whether the solutions of septics may be obtained by superimposing algebraic functions of two variables (the problem still being open).
Galois groups

- Septic equations solvable by radicals have a Galois groupGalois groupIn mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
which is either the cyclic groupCyclic groupIn group theory, a cyclic group is a group that can be generated by a single element, in the sense that the group has an element g such that, when written multiplicatively, every element of the group is a power of g .-Definition:A group G is called cyclic if there exists an element g...
of order 7, or the dihedral groupDihedral groupIn mathematics, a dihedral group is the group of symmetries of a regular polygon, including both rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry.See also: Dihedral symmetry in three...
of order 14 or a metacyclic groupMetacyclic groupIn group theory, a metacyclic group is an extension of a cyclic group by a cyclic group. That is, it is a group G for which there is a short exact sequence1 \rightarrow K \rightarrow G \rightarrow H \rightarrow 1,\,where H and K are cyclic...
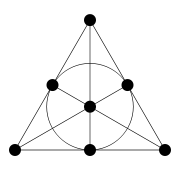
of order 21 or 42. - The L(3, 2) Galois groupGalois groupIn mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
(of order 168) is formed by the permutations of the 7 vertex labels which preserve the 7 "lines" in the Fano planeFano planeIn finite geometry, the Fano plane is the finite projective plane with the smallest possible number of points and lines: 7 each.-Homogeneous coordinates:...
. Septic equations with this Galois groupGalois groupIn mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...
L(3, 2) require elliptic functionElliptic functionIn complex analysis, an elliptic function is a function defined on the complex plane that is periodic in two directions and at the same time is meromorphic...
s but not hyperelliptic functions for their solution. - Otherwise the Galois group of a septic is either the alternating group of order 2520 or the symmetric groupSymmetric groupIn mathematics, the symmetric group Sn on a finite set of n symbols is the group whose elements are all the permutations of the n symbols, and whose group operation is the composition of such permutations, which are treated as bijective functions from the set of symbols to itself...
of order 5040.

