
Square knot (mathematics)
Encyclopedia
In knot theory
, the square knot is a composite knot obtained by taking the connected sum of two trefoil knot
s. It is closely related to the granny knot
, which is also a connected sum of two trefoils. Because the trefoil knot is the simplest nontrivial knot, the square knot and the granny knot are the simplest of all composite knots.
The square knot is the mathematical version of the common reef knot
.
It is important that the original trefoil knots be mirror images of one another. If two identical trefoil knots are used instead, the result is a granny knot.
, meaning that it is indistinguishable from its own mirror image. The crossing number
of a square knot is six, which is the smallest possible crossing number for a composite knot.
The Alexander polynomial
of the square knot is
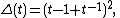
which is simply the square of the Alexander polynomial of a trefoil knot. Similarly, the Conway polynomial
of a square knot is
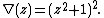
These two polynomials are the same as those for the granny knot. However, the Jones polynomial for the square knot is
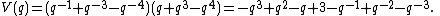
This is the product of the Jones polynomials for the right-handed and left-handed trefoil knots, and is different from the Jones polynomial for a granny knot.
The knot group
of the square knot is given by the presentation
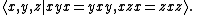
This is isomorphic
to the knot group of the granny knot, and is the simplest example of two different knots with isomorphic knot groups.
Unlike the granny knot, the square knot is a ribbon knot
, and it is therefore also a slice knot.
Knot theory
In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...
, the square knot is a composite knot obtained by taking the connected sum of two trefoil knot
Trefoil knot
In topology, a branch of mathematics, the trefoil knot is the simplest example of a nontrivial knot. The trefoil can be obtained by joining together the two loose ends of a common overhand knot, resulting in a knotted loop...
s. It is closely related to the granny knot
Granny knot (mathematics)
In knot theory, the granny knot is a composite knot obtained by taking the connected sum of two identical trefoil knots. It is closely related to the square knot, which can also be described as a connected sum of two trefoils...
, which is also a connected sum of two trefoils. Because the trefoil knot is the simplest nontrivial knot, the square knot and the granny knot are the simplest of all composite knots.
The square knot is the mathematical version of the common reef knot
Reef knot
The reef knot or square knot is an ancient and simple binding knot used to secure a rope or line around an object. Although the reef knot is often seen used for tying two ropes together, it is not recommended for this purpose due to potential instability of the knot.A reef knot is formed by tying...
.
Construction
The square knot can be constructed from two trefoil knots, one of which must be left-handed and the other right-handed. Each of the two knots is cut, and then the loose ends are joined together pairwise. The resulting connected sum is the square knot.It is important that the original trefoil knots be mirror images of one another. If two identical trefoil knots are used instead, the result is a granny knot.
Properties
The square knot is amphichiralAmphichiral knot
In the mathematical field of knot theory, a chiral knot is a knot that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphichiral knot, also called an achiral knot or amphicheiral knot. The chirality of a knot is a knot invariant...
, meaning that it is indistinguishable from its own mirror image. The crossing number
Crossing number (knot theory)
In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....
of a square knot is six, which is the smallest possible crossing number for a composite knot.
The Alexander polynomial
Alexander polynomial
In mathematics, the Alexander polynomial is a knot invariant which assigns a polynomial with integer coefficients to each knot type. James Waddell Alexander II discovered this, the first knot polynomial, in 1923...
of the square knot is
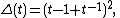
which is simply the square of the Alexander polynomial of a trefoil knot. Similarly, the Conway polynomial
Conway polynomial
In mathematics, Conway polynomial can refer to:* the Alexander–Conway polynomial in knot theory* the Conway polynomial...
of a square knot is
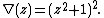
These two polynomials are the same as those for the granny knot. However, the Jones polynomial for the square knot is
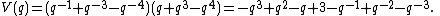
This is the product of the Jones polynomials for the right-handed and left-handed trefoil knots, and is different from the Jones polynomial for a granny knot.
The knot group
Knot group
In mathematics, a knot is an embedding of a circle into 3-dimensional Euclidean space. The knot group of a knot K is defined as the fundamental group of the knot complement of K in R3,\pi_1....
of the square knot is given by the presentation
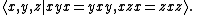
This is isomorphic
Group isomorphism
In abstract algebra, a group isomorphism is a function between two groups that sets up a one-to-one correspondence between the elements of the groups in a way that respects the given group operations. If there exists an isomorphism between two groups, then the groups are called isomorphic...
to the knot group of the granny knot, and is the simplest example of two different knots with isomorphic knot groups.
Unlike the granny knot, the square knot is a ribbon knot
Ribbon knot
In the mathematical area of knot theory, a ribbon knot is a knot that bounds a self-intersecting disc with only ribbon singularities. This type of singularity is a self-intersection along an arc; the preimage of this arc consists of two arcs in the disc, one properly embedded in the disc and the...
, and it is therefore also a slice knot.

