
Sumset
Encyclopedia
In additive combinatorics, the sumset (also called the Minkowski sum
) of two subsets A and B of an abelian group
G (written additively) is defined to be the set of all sums of an element from A with an element from B. That is,
 .
.
The n-fold iterated sumset of A is

where there are n summands.
Many of the questions and results of additive combinatorics and additive number theory
can be phrased in terms of sumsets. For example, Lagrange's four-square theorem
can be written succinctly in the form
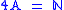
where A is the set of square number
s. A subject that has received a fair amount of study is that of sets with small doubling, where the size of the set A+A is small (compared to the size of A); see for example Freiman's theorem
.
Minkowski addition
In geometry, the Minkowski sum of two sets A and B in Euclidean space is the result of adding every element of A to every element of B, i.e...
) of two subsets A and B of an abelian group
Abelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
G (written additively) is defined to be the set of all sums of an element from A with an element from B. That is,
 .
.The n-fold iterated sumset of A is

where there are n summands.
Many of the questions and results of additive combinatorics and additive number theory
Additive number theory
In number theory, the specialty additive number theory studies subsets of integers and their behavior under addition. More abstractly, the field of "additive number theory" includes the study of Abelian groups and commutative semigroups with an operation of addition. Additive number theory has...
can be phrased in terms of sumsets. For example, Lagrange's four-square theorem
Lagrange's four-square theorem
Lagrange's four-square theorem, also known as Bachet's conjecture, states that any natural number can be represented as the sum of four integer squaresp = a_0^2 + a_1^2 + a_2^2 + a_3^2\ where the four numbers are integers...
can be written succinctly in the form
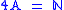
where A is the set of square number
Square number
In mathematics, a square number, sometimes also called a perfect square, is an integer that is the square of an integer; in other words, it is the product of some integer with itself...
s. A subject that has received a fair amount of study is that of sets with small doubling, where the size of the set A+A is small (compared to the size of A); see for example Freiman's theorem
Freiman's theorem
In mathematics, Freiman's theorem is a combinatorial result in number theory. In a sense it accounts for the approximate structure of sets of integers that contain a high proportion of their internal sums, taken two at a time.The formal statement is:...
.
See also
- Minkowski additionMinkowski additionIn geometry, the Minkowski sum of two sets A and B in Euclidean space is the result of adding every element of A to every element of B, i.e...
(geometryGeometryGeometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
) - Restricted sumset
- Sidon set
- Sum-free setSum-free setIn additive combinatorics and number theory, a subset A of an abelian group G is said to be sum-free if the sumset A⊕A is disjoint from A...
- Schnirelmann densitySchnirelmann densityIn additive number theory, the Schnirelmann density of a sequence of numbers is a way to measure how "dense" the sequence is. It is named after Russian mathematician L.G...
- Shapley–Folkman lemmaShapley–Folkman lemmaIn geometry and economics, the Shapley–Folkman lemma describes the Minkowski addition of sets in a vector space. Minkowski addition is defined as the addition of the sets' members: for example, adding the set consisting of the integers zero and one to itself yields the set consisting of...

