Supersymmetry
Encyclopedia
In particle physics
, supersymmetry (often abbreviated SUSY) is a symmetry
that relates elementary particle
s of one spin
to other particles that differ by half a unit of spin and are known as superpartner
s. In a theory with unbroken supersymmetry, for every type of boson
there exists a corresponding type of fermion
with the same mass and internal quantum numbers, and vice-versa.
There is no direct evidence for the existence of supersymmetry. It is motivated by possible solutions to several theoretical problems. Since the superpartners of the Standard Model
particles have not been observed, supersymmetry, if it exists, must be a broken symmetry, allowing the superparticles
to be heavier than the corresponding Standard Model particles.
If supersymmetry exists close to the TeV
energy scale, it allows for a solution of the hierarchy problem
of the Standard Model, i.e., the fact that the Higgs boson
mass is subject to quantum corrections which — barring extremely fine-tuned
cancellations among independent contributions — would make it so large as to undermine the internal consistency of the theory. In supersymmetric theories, on the other hand, the contributions to the quantum corrections coming from Standard Model particles are naturally canceled by the contributions of the corresponding superpartners. Other attractive features of TeV-scale supersymmetry are the fact that it allows for the high-energy unification
of the weak interactions, the strong interactions and electromagnetism
, and the fact that it provides a candidate for Dark Matter
and a natural mechanism for electroweak symmetry breaking. Therefore, scenarios where supersymmetric partners appear with masses not much greater than 1 TeV are considered the most well-motivated by theorists. These scenarios would imply that experimental traces of the superpartners should begin to emerge in high-energy collisions at the LHC
relatively soon. As of September 2011, no meaningful signs of the superpartners have been observed, which is beginning to significantly constrain the most popular incarnations of supersymmetry. However, the total parameter space of consistent supersymmetric extensions of the Standard Model is extremely diverse and can not be definitively ruled out at the LHC.
Another theoretically appealing property of supersymmetry is that it offers the only "loophole" to the Coleman–Mandula theorem, which prohibits spacetime and internal symmetries from being combined in any nontrivial way, for quantum field theories
like the Standard Model under very general assumptions. The Haag-Lopuszanski-Sohnius theorem
demonstrates that supersymmetry is the only way spacetime and internal symmetries can be consistently combined.
In general, supersymmetric quantum field theory
is often much easier to work with, as many more problems become exactly solvable. Supersymmetry is also a feature of most versions of string theory
, though it may exist in nature even if string theory is incorrect.
The Minimal Supersymmetric Standard Model
is one of the best studied candidates for physics beyond the Standard Model. Theories of gravity that are also invariant under supersymmetry are known as supergravity
theories.
In the early 1970s, J. L. Gervais and B. Sakita
(in 1971), Yu. A. Golfand
and E.P. Likhtman (also in 1971), D.V. Volkov and V.P. Akulov (in 1972) and J. Wess
and B. Zumino
(in 1974) independently rediscovered supersymmetry, a radically new type of symmetry of spacetime and fundamental fields, which establishes a relationship between elementary particles of different quantum nature, bosons and fermions, and unifies spacetime and internal symmetries of the microscopic world. Supersymmetry first arose in 1971 in the context of an early version of string theory
by Pierre Ramond
, John H. Schwarz and Andre Neveu
, but the mathematical structure of supersymmetry has subsequently been applied successfully to other areas of physics; firstly by Wess, Zumino, and Abdus Salam
and their fellow researchers to particle physics, and later to a variety of fields, ranging from quantum mechanics
to statistical physics
. It remains a vital part of many proposed theories of physics.
The first realistic supersymmetric version of the Standard Model was proposed in 1981 by Howard Georgi
and Savas Dimopoulos
and is called the Minimal Supersymmetric Standard Model
or MSSM for short. It was proposed to solve the hierarchy problem
and predicts superpartners with masses between 100 GeV and 1 TeV.
As of 2009 there is no irrefutable experimental evidence that supersymmetry is a symmetry of nature. Since 2010, the Large Hadron Collider
at CERN
is producing the world's highest energy collisions and offers the best chance at discovering superparticles for the foreseeable future.
and internal symmetries and the Coleman–Mandula theorem showed that under certain assumptions, the symmetries of the S-matrix must be a direct product of the Poincaré group with a compact
internal symmetry group or if there is no mass gap
, the conformal group
with a compact internal symmetry group.
In 1971 Golfand
and Likhtman were the first to show that the Poincaré algebra can be extended through introduction of four
anticommuting spinor generators (in four dimensions), which later became known as supercharges.
In 1975 the Haag-Lopuszanski-Sohnius theorem
analyzed all possible superalgebras in the general form, including those with an extended number of the supergenerators and central charge
s.
This extended super-Poincaré algebra paved the way for obtaining a very large and important class of supersymmetric field theories.
representations of the Poincaré group
and internal symmetries. Supersymmetries, on the other hand, are generated by objects that transform under the spinor
representations. According to the spin-statistics theorem
, boson
ic fields commute while fermion
ic fields anticommute
. Combining the two kinds of fields into a single algebra
requires the introduction of a Z2-grading
under which the bosons are the even elements and the fermions are the odd elements. Such an algebra is called a Lie superalgebra
.
The simplest supersymmetric extension of the Poincaré algebra is the Super-Poincaré algebra. Expressed in terms of two Weyl spinors, has the following anti-commutation
relation: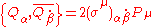
and all other anti-commutation relations between the Qs and commutation relations between the Qs and Ps vanish. In the above expression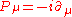
are the generators of translation and 
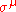are the Pauli matrices
.
There are representations of a Lie superalgebra
that are analogous to representations of a Lie algebra. Each Lie algebra has an associated Lie group and a Lie superalgebra can sometimes be extended into representations of a Lie supergroup.
requires doubling the number of particles since there is no way that any of the particles in the Standard Model can be superpartner
s of each other. With the addition of new particles, there are many possible new interactions. The simplest possible supersymmetric model consistent with the Standard Model is the Minimal Supersymmetric Standard Model
(MSSM) which can include the necessary additional new particles that are able to be superpartner
s of those in the Standard Model
.
One of the main motivations for SUSY comes from the quadratically divergent contributions to the Higgs mass squared. The quantum mechanical interactions of the Higgs boson causes a large renormalization of the Higgs mass and unless there is an accidental cancellation, the natural size of the Higgs mass is the highest scale possible. This problem is known as the hierarchy problem
. Supersymmetry reduces the size of the quantum corrections by having automatic cancellations between fermionic and bosonic Higgs interactions. If supersymmetry is restored at the weak scale, then the Higgs mass is related to supersymmetry breaking which can be induced from small non-perturbative effects explaining the vastly different scales in the weak interactions and gravitational interactions.
In many supersymmetric Standard Models there is a heavy stable particle (such as neutralino
) which could serve as a Weakly interacting massive particle (WIMP) dark matter
candidate. The existence of a supersymmetric dark matter candidate is closely tied to R-parity
.
The standard paradigm for incorporating supersymmetry into a realistic theory is to have the underlying dynamics of the theory be supersymmetric, but the ground state of the theory does not respect the symmetry and supersymmetry is broken spontaneously
. The supersymmetry break can not be done permanently by the particles of the MSSM as they currently appear. This means that there is a new sector of the theory that is responsible for the breaking. The only constraint on this new sector is that it must break supersymmetry permanently and must give superparticles TeV scale masses. There are many models that can do this and most of their details do not currently matter. In order to parameterize the relevant features of supersymmetry breaking, arbitrary soft SUSY breaking
terms are added to the theory which temporarily break SUSY explicitly but could never arise from a complete theory of supersymmetry breaking.
The renormalization group
evolution of the three gauge coupling constant
s of the Standard Model
is somewhat sensitive to the present particle content of the theory. These coupling constants do not quite meet together at a common energy scale if we run the renormalization group using the Standard Model
. With the addition of minimal SUSY joint convergence of the coupling constants is projected at approximately 1016 GeV
.
as opposed to quantum field theory
. Supersymmetric quantum mechanics often comes up when studying the dynamics of supersymmetric solitons and due to the simplified nature of having fields only functions of time (rather than space-time), a great deal of progress has been made in this subject and is now studied in its own right.
SUSY quantum mechanics involves pairs of Hamiltonians
which share a particular mathematical relationship, which are called partner Hamiltonians. (The potential energy
terms which occur in the Hamiltonians are then called partner potentials.) An introductory theorem shows that for every eigenstate of one Hamiltonian, its partner Hamiltonian has a corresponding eigenstate with the same energy. This fact can be exploited to deduce many properties of the eigenstate spectrum. It is analogous to the original description of SUSY, which referred to bosons and fermions. We can imagine a "bosonic Hamiltonian", whose eigenstates are the various bosons of our theory. The SUSY partner of this Hamiltonian would be "fermionic", and its eigenstates would be the theory's fermions. Each boson would have a fermionic partner of equal energy.
SUSY concepts have provided useful extensions to the WKB approximation
. In addition, SUSY has been applied to non-quantum statistical mechanics
through the Fokker-Planck equation
.
s of more realistic theories. A prime example of this has been the demonstration of S-duality in four-dimensional gauge theories that interchanges particles and monopole
s.
The maximal number of supersymmetry generators possible is 32. Theories with more than 32 supersymmetry generators automatically have massless fields with spin greater than 2. It is not known how to make massless fields with spin greater than two interact, so the maximal number of supersymmetry generators considered is 32. This corresponds to an N = 8 supersymmetry theory. Theories with 32 supersymmetries automatically have a graviton
.
In four dimensions there are the following theories, with the corresponding multiplets(CPT adds a copy, whenever they are not invariant under such symmetry)
Chiral multiplet:
(0,)
Vector multiplet:
Gravitino multiplet:
(1,)
Graviton multiplet:
hypermultiplet:
(-,02,)
vector multiplet:
(0,2,1)
supergravity multiplet:
(1,2,2)
Vector multiplet:
(-1,-4,06,4,1)
Supergravity multiplet:
(0,4,16,4,2)
Supergravity multiplet:
(-2,-8,-128,-56,070,56,128,8,2)
and quantum group
s. In particular, it involves a mild form of noncommutativity, namely supercommutativity. See the main article for more details.
(TOE). A significant part of this larger enterprise is the quest for a theory of quantum gravity
, which would unify the classical theory of general relativity
and the Standard Model
, which explains the other three basic forces
in physics (electromagnetism
, the strong interaction
, and the weak interaction
), and provides a palette of fundamental particles upon which all four forces act. Two of the most active approaches to forming a theory of quantum gravity are string theory
and loop quantum gravity
(LQG), although in theory, supersymmetry could be a component of other theoretical approaches as well.
For string theory
to be consistent, supersymmetry appears to be required at some level (although it may be a strongly broken symmetry). In particle theory, supersymmetry is recognized as a way to stabilize the hierarchy
between the unification scale and the electroweak scale (or the Higgs boson
mass), and can also provide a natural dark matter
candidate. String theory also requires extra spatial dimensions which have to be compactified as in Kaluza-Klein theory.
Loop quantum gravity
(LQG), in its current formulation, predicts no additional spatial dimensions, nor anything else about particle physics. These theories can be formulated in three spatial dimensions and one dimension of time, although in some LQG theories dimensionality is an emergent property of the theory, rather than a fundamental assumption of the theory. Also, LQG is a theory of quantum gravity which does not require supersymmetry. Lee Smolin
, one of the originators of LQG, has proposed that a loop quantum gravity theory incorporating either supersymmetry or extra dimensions, or both, be called "loop quantum gravity II".
If experimental evidence confirms supersymmetry in the form of supersymmetric particles such as the neutralino
that is often believed to be the lightest superpartner
, some people believe this would be a major boost to string theory
. Since supersymmetry is a required component of string theory, any discovered supersymmetry would be consistent with string theory. If the Large Hadron Collider
and other major particle physics experiments fail to detect supersymmetric partners or evidence of extra dimensions, many versions of string theory
which had predicted certain low mass superpartners to existing particles may need to be significantly revised. The failure of experiments to discover either supersymmetric partners or extra spatial dimensions, , has encouraged loop quantum gravity
researchers.
had set limits for specific models which have now been exceeded by the Large Hadron Collider
. Searches are only applicable for a finite set of tested points because simulation using the Monte Carlo method
must be made so that limits for that particular model can be calculated. This complicates matters because different experiments have looked at different sets of points. Some extrapolation between points can be made within particular models but it is difficult to set general limits even for the Minimal Supersymmetric Standard Model
.
The first mass limits for squarks and gluinos were made at CERN
by the UA1 experiment and the UA2 experiment at the Super Proton Synchrotron
. LEP later set very strong limits. In 2006 these limits were extended by the D0 experiment The LHC has now extended these limits, by extending the search area, with no sign of supersymmmetry.
Particle physics
Particle physics is a branch of physics that studies the existence and interactions of particles that are the constituents of what is usually referred to as matter or radiation. In current understanding, particles are excitations of quantum fields and interact following their dynamics...
, supersymmetry (often abbreviated SUSY) is a symmetry
Symmetry in physics
In physics, symmetry includes all features of a physical system that exhibit the property of symmetry—that is, under certain transformations, aspects of these systems are "unchanged", according to a particular observation...
that relates elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
s of one spin
Spin (physics)
In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
to other particles that differ by half a unit of spin and are known as superpartner
Superpartner
In particle physics, a superpartner is a hypothetical elementary particle. Supersymmetry is one of the synergistic theories in current high-energy physics which predicts the existence of these "shadow" particles....
s. In a theory with unbroken supersymmetry, for every type of boson
Boson
In particle physics, bosons are subatomic particles that obey Bose–Einstein statistics. Several bosons can occupy the same quantum state. The word boson derives from the name of Satyendra Nath Bose....
there exists a corresponding type of fermion
Fermion
In particle physics, a fermion is any particle which obeys the Fermi–Dirac statistics . Fermions contrast with bosons which obey Bose–Einstein statistics....
with the same mass and internal quantum numbers, and vice-versa.
There is no direct evidence for the existence of supersymmetry. It is motivated by possible solutions to several theoretical problems. Since the superpartners of the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
particles have not been observed, supersymmetry, if it exists, must be a broken symmetry, allowing the superparticles
Superpartner
In particle physics, a superpartner is a hypothetical elementary particle. Supersymmetry is one of the synergistic theories in current high-energy physics which predicts the existence of these "shadow" particles....
to be heavier than the corresponding Standard Model particles.
If supersymmetry exists close to the TeV
Electronvolt
In physics, the electron volt is a unit of energy equal to approximately joule . By definition, it is equal to the amount of kinetic energy gained by a single unbound electron when it accelerates through an electric potential difference of one volt...
energy scale, it allows for a solution of the hierarchy problem
Hierarchy problem
In theoretical physics, a hierarchy problem occurs when the fundamental parameters of some Lagrangian are vastly different than the parameters measured by experiment. This can happen because measured parameters are related to the fundamental parameters by a prescription known as renormalization...
of the Standard Model, i.e., the fact that the Higgs boson
Higgs boson
The Higgs boson is a hypothetical massive elementary particle that is predicted to exist by the Standard Model of particle physics. Its existence is postulated as a means of resolving inconsistencies in the Standard Model...
mass is subject to quantum corrections which — barring extremely fine-tuned
Fine Tuning
Fine Tuning was the name of XM Satellite Radio's eclectic music channel. The program director for Fine Tuning was Ben Smith and the tag line was, "The World's Most Interesting Music"....
cancellations among independent contributions — would make it so large as to undermine the internal consistency of the theory. In supersymmetric theories, on the other hand, the contributions to the quantum corrections coming from Standard Model particles are naturally canceled by the contributions of the corresponding superpartners. Other attractive features of TeV-scale supersymmetry are the fact that it allows for the high-energy unification
Grand unification theory
The term Grand Unified Theory, often abbreviated as GUT, refers to any of several similar candidate models in particle physics in which at high-energy, the three gauge interactions of the Standard Model which define the electromagnetic, weak, and strong interactions, are merged into one single...
of the weak interactions, the strong interactions and electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
, and the fact that it provides a candidate for Dark Matter
Dark matter
In astronomy and cosmology, dark matter is matter that neither emits nor scatters light or other electromagnetic radiation, and so cannot be directly detected via optical or radio astronomy...
and a natural mechanism for electroweak symmetry breaking. Therefore, scenarios where supersymmetric partners appear with masses not much greater than 1 TeV are considered the most well-motivated by theorists. These scenarios would imply that experimental traces of the superpartners should begin to emerge in high-energy collisions at the LHC
LHC
LHC may refer to:* Large Hadron Collider, a particle accelerator and collider located on the Franco-Swiss border near Geneva, SwitzerlandLHC also may refer to:* La hora Chanante, a Spanish comedy television show...
relatively soon. As of September 2011, no meaningful signs of the superpartners have been observed, which is beginning to significantly constrain the most popular incarnations of supersymmetry. However, the total parameter space of consistent supersymmetric extensions of the Standard Model is extremely diverse and can not be definitively ruled out at the LHC.
Another theoretically appealing property of supersymmetry is that it offers the only "loophole" to the Coleman–Mandula theorem, which prohibits spacetime and internal symmetries from being combined in any nontrivial way, for quantum field theories
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
like the Standard Model under very general assumptions. The Haag-Lopuszanski-Sohnius theorem
Haag-Lopuszanski-Sohnius theorem
In theoretical physics, the Haag–Lopuszanski–Sohnius theorem shows that the possible symmetries of a consistent 4-dimensional quantum field theory do not only consist of internal symmetries and Poincaré symmetry, but can also include supersymmetry as a nontrivial extension of the Poincaré algebra...
demonstrates that supersymmetry is the only way spacetime and internal symmetries can be consistently combined.
In general, supersymmetric quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
is often much easier to work with, as many more problems become exactly solvable. Supersymmetry is also a feature of most versions of string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
, though it may exist in nature even if string theory is incorrect.
The Minimal Supersymmetric Standard Model
Minimal Supersymmetric Standard Model
The Minimal Supersymmetric Standard Model is the minimal extension to the Standard Model that realizes supersymmetry, although non-minimal extensions do exist. Supersymmetry pairs bosons with fermions; therefore every Standard Model particle has a partner that has yet to be discovered...
is one of the best studied candidates for physics beyond the Standard Model. Theories of gravity that are also invariant under supersymmetry are known as supergravity
Supergravity
In theoretical physics, supergravity is a field theory that combines the principles of supersymmetry and general relativity. Together, these imply that, in supergravity, the supersymmetry is a local symmetry...
theories.
History
A supersymmetry relating mesons and baryons was first proposed, in the context of hadronic physics, by Hironari Miyazawa in 1966, but his work was ignored at the time.In the early 1970s, J. L. Gervais and B. Sakita
Bunji Sakita
was a Japanese-American theoretical physicist who made important contributions in quantum field theory, superstring theory and discovered supersymmetry in 1971. He was a Distinguished Professor of Physics at the City College of New York.-Early years:...
(in 1971), Yu. A. Golfand
Yuri Golfand
Yuri Golfand was a Russian and Israeli physisist known, in particular, for his 1971 paper where they proposed supersymmetry between bosonic and ferminoic particles by extending the Poincare algebra with anticommuting spinor generators. The algebra they constructed is also called a Super-Poincaré...
and E.P. Likhtman (also in 1971), D.V. Volkov and V.P. Akulov (in 1972) and J. Wess
Julius Wess
Julius Wess was an Austrian theoretical physicist noted as the co-inventor of the Wess–Zumino model and Wess–Zumino–Witten model in the field of supersymmetry...
and B. Zumino
Bruno Zumino
Bruno Zumino is an Italian theoretical physicist and emeritus faculty at the University of California, Berkeley. He got his bachelor degree from the University of Rome in 1945...
(in 1974) independently rediscovered supersymmetry, a radically new type of symmetry of spacetime and fundamental fields, which establishes a relationship between elementary particles of different quantum nature, bosons and fermions, and unifies spacetime and internal symmetries of the microscopic world. Supersymmetry first arose in 1971 in the context of an early version of string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
by Pierre Ramond
Pierre Ramond
Pierre Ramond is a Distinguished Professor of Physics at University of Florida in Gainesville, Florida...
, John H. Schwarz and Andre Neveu
André Neveu
André Neveu is a French physicist working on string theory and quantum field theory who coinvented the Neveu-Schwarz algebra and the Gross-Neveu model.Neveu studied in Paris at the École normale supérieure...
, but the mathematical structure of supersymmetry has subsequently been applied successfully to other areas of physics; firstly by Wess, Zumino, and Abdus Salam
Abdus Salam
Mohammad Abdus Salam, NI, SPk Mohammad Abdus Salam, NI, SPk Mohammad Abdus Salam, NI, SPk (Urdu: محمد عبد السلام, pronounced , (January 29, 1926– November 21, 1996) was a Pakistani theoretical physicist and Nobel laureate in Physics for his work on the electroweak unification of the...
and their fellow researchers to particle physics, and later to a variety of fields, ranging from quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
to statistical physics
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
. It remains a vital part of many proposed theories of physics.
The first realistic supersymmetric version of the Standard Model was proposed in 1981 by Howard Georgi
Howard Georgi
Howard Mason Georgi III, born January 6, 1947 in San Bernardino, California, is Harvard College Professor and Mallinckrodt Professor of Physics at Harvard University...
and Savas Dimopoulos
Savas Dimopoulos
Savas Dimopoulos is a Greek particle physicist at Stanford University. He was born in Istanbul, Turkey and later moved to Athens due to ethnic tensions in Turkey during the 1950s and 1960s. Dimopoulos studied as an undergraduate at the University of Houston...
and is called the Minimal Supersymmetric Standard Model
Minimal Supersymmetric Standard Model
The Minimal Supersymmetric Standard Model is the minimal extension to the Standard Model that realizes supersymmetry, although non-minimal extensions do exist. Supersymmetry pairs bosons with fermions; therefore every Standard Model particle has a partner that has yet to be discovered...
or MSSM for short. It was proposed to solve the hierarchy problem
Hierarchy problem
In theoretical physics, a hierarchy problem occurs when the fundamental parameters of some Lagrangian are vastly different than the parameters measured by experiment. This can happen because measured parameters are related to the fundamental parameters by a prescription known as renormalization...
and predicts superpartners with masses between 100 GeV and 1 TeV.
As of 2009 there is no irrefutable experimental evidence that supersymmetry is a symmetry of nature. Since 2010, the Large Hadron Collider
Large Hadron Collider
The Large Hadron Collider is the world's largest and highest-energy particle accelerator. It is expected to address some of the most fundamental questions of physics, advancing the understanding of the deepest laws of nature....
at CERN
CERN
The European Organization for Nuclear Research , known as CERN , is an international organization whose purpose is to operate the world's largest particle physics laboratory, which is situated in the northwest suburbs of Geneva on the Franco–Swiss border...
is producing the world's highest energy collisions and offers the best chance at discovering superparticles for the foreseeable future.
Extension of possible symmetry groups
One reason that physicists explored supersymmetry is because it offers an extension to the more familiar symmetries of quantum field theory. These symmetries are grouped into the Poincaré groupPoincaré group
In physics and mathematics, the Poincaré group, named after Henri Poincaré, is the group of isometries of Minkowski spacetime.-Simple explanation:...
and internal symmetries and the Coleman–Mandula theorem showed that under certain assumptions, the symmetries of the S-matrix must be a direct product of the Poincaré group with a compact
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
internal symmetry group or if there is no mass gap
Mass gap
In quantum field theory, the mass gap is the difference in energy between the vacuum and the next lowest energy state. The energy of the vacuum is zero by definition, and assuming that all energy states can be thought of as particles in plane-waves, the mass gap is the mass of the lightest...
, the conformal group
Conformal group
In mathematics, the conformal group is the group of transformations from a space to itself that preserve all angles within the space. More formally, it is the group of transformations that preserve the conformal geometry of the space. Several specific conformal groups are particularly important:*...
with a compact internal symmetry group.
In 1971 Golfand
Yuri Golfand
Yuri Golfand was a Russian and Israeli physisist known, in particular, for his 1971 paper where they proposed supersymmetry between bosonic and ferminoic particles by extending the Poincare algebra with anticommuting spinor generators. The algebra they constructed is also called a Super-Poincaré...
and Likhtman were the first to show that the Poincaré algebra can be extended through introduction of four
anticommuting spinor generators (in four dimensions), which later became known as supercharges.
In 1975 the Haag-Lopuszanski-Sohnius theorem
Haag-Lopuszanski-Sohnius theorem
In theoretical physics, the Haag–Lopuszanski–Sohnius theorem shows that the possible symmetries of a consistent 4-dimensional quantum field theory do not only consist of internal symmetries and Poincaré symmetry, but can also include supersymmetry as a nontrivial extension of the Poincaré algebra...
analyzed all possible superalgebras in the general form, including those with an extended number of the supergenerators and central charge
Central charge
In theoretical physics, a central charge is an operator Z that commutes with all the other symmetry operators. The adjective "central" refers to the center of the symmetry group -- the subgroup of elements that commute with all other elements of the original group—or to the center of a Lie algebra...
s.
This extended super-Poincaré algebra paved the way for obtaining a very large and important class of supersymmetric field theories.
The supersymmetry algebra
Traditional symmetries in physics are generated by objects that transform under the tensorTensor
Tensors are geometric objects that describe linear relations between vectors, scalars, and other tensors. Elementary examples include the dot product, the cross product, and linear maps. Vectors and scalars themselves are also tensors. A tensor can be represented as a multi-dimensional array of...
representations of the Poincaré group
Poincaré group
In physics and mathematics, the Poincaré group, named after Henri Poincaré, is the group of isometries of Minkowski spacetime.-Simple explanation:...
and internal symmetries. Supersymmetries, on the other hand, are generated by objects that transform under the spinor
Spinor
In mathematics and physics, in particular in the theory of the orthogonal groups , spinors are elements of a complex vector space introduced to expand the notion of spatial vector. Unlike tensors, the space of spinors cannot be built up in a unique and natural way from spatial vectors...
representations. According to the spin-statistics theorem
Spin-statistics theorem
In quantum mechanics, the spin-statistics theorem relates the spin of a particle to the particle statistics it obeys. The spin of a particle is its intrinsic angular momentum...
, boson
Boson
In particle physics, bosons are subatomic particles that obey Bose–Einstein statistics. Several bosons can occupy the same quantum state. The word boson derives from the name of Satyendra Nath Bose....
ic fields commute while fermion
Fermion
In particle physics, a fermion is any particle which obeys the Fermi–Dirac statistics . Fermions contrast with bosons which obey Bose–Einstein statistics....
ic fields anticommute
Anticommutativity
In mathematics, anticommutativity is the property of an operation that swapping the position of any two arguments negates the result. Anticommutative operations are widely used in algebra, geometry, mathematical analysis and, as a consequence, in physics: they are often called antisymmetric...
. Combining the two kinds of fields into a single algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
requires the introduction of a Z2-grading
Graded algebra
In mathematics, in particular abstract algebra, a graded algebra is an algebra over a field with an extra piece of structure, known as a gradation ....
under which the bosons are the even elements and the fermions are the odd elements. Such an algebra is called a Lie superalgebra
Lie superalgebra
In mathematics, a Lie superalgebra is a generalisation of a Lie algebra to include a Z2-grading. Lie superalgebras are important in theoretical physics where they are used to describe the mathematics of supersymmetry...
.
The simplest supersymmetric extension of the Poincaré algebra is the Super-Poincaré algebra. Expressed in terms of two Weyl spinors, has the following anti-commutation
Commutator
In mathematics, the commutator gives an indication of the extent to which a certain binary operation fails to be commutative. There are different definitions used in group theory and ring theory.-Group theory:...
relation:
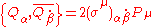
and all other anti-commutation relations between the Qs and commutation relations between the Qs and Ps vanish. In the above expression
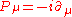are the generators of translation and 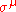are the Pauli matricesPauli matrices
The Pauli matrices are a set of three 2 × 2 complex matrices which are Hermitian and unitary. Usually indicated by the Greek letter "sigma" , they are occasionally denoted with a "tau" when used in connection with isospin symmetries...
.
There are representations of a Lie superalgebra
Representation of a Lie superalgebra
In the mathematical field of representation theory, a representation of a Lie superalgebra is an action of Lie superalgebra L on a Z2-graded vector space V, such that if A and B are any two pure elements of L and X and Y are any two pure elements of V, then[X]=c_1 A[X] + c_2 B[X]\,A[c_1 X + c_2...
that are analogous to representations of a Lie algebra. Each Lie algebra has an associated Lie group and a Lie superalgebra can sometimes be extended into representations of a Lie supergroup.
The Supersymmetric Standard Model
Incorporating supersymmetry into the Standard ModelStandard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
requires doubling the number of particles since there is no way that any of the particles in the Standard Model can be superpartner
Superpartner
In particle physics, a superpartner is a hypothetical elementary particle. Supersymmetry is one of the synergistic theories in current high-energy physics which predicts the existence of these "shadow" particles....
s of each other. With the addition of new particles, there are many possible new interactions. The simplest possible supersymmetric model consistent with the Standard Model is the Minimal Supersymmetric Standard Model
Minimal Supersymmetric Standard Model
The Minimal Supersymmetric Standard Model is the minimal extension to the Standard Model that realizes supersymmetry, although non-minimal extensions do exist. Supersymmetry pairs bosons with fermions; therefore every Standard Model particle has a partner that has yet to be discovered...
(MSSM) which can include the necessary additional new particles that are able to be superpartner
Superpartner
In particle physics, a superpartner is a hypothetical elementary particle. Supersymmetry is one of the synergistic theories in current high-energy physics which predicts the existence of these "shadow" particles....
s of those in the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
.
Hierarchy problem
In theoretical physics, a hierarchy problem occurs when the fundamental parameters of some Lagrangian are vastly different than the parameters measured by experiment. This can happen because measured parameters are related to the fundamental parameters by a prescription known as renormalization...
. Supersymmetry reduces the size of the quantum corrections by having automatic cancellations between fermionic and bosonic Higgs interactions. If supersymmetry is restored at the weak scale, then the Higgs mass is related to supersymmetry breaking which can be induced from small non-perturbative effects explaining the vastly different scales in the weak interactions and gravitational interactions.
In many supersymmetric Standard Models there is a heavy stable particle (such as neutralino
Neutralino
In particle physics, the neutralino is a hypothetical particle predicted by supersymmetry. There are four neutralinos that are fermions and are electrically neutral, the lightest of which is typically stable...
) which could serve as a Weakly interacting massive particle (WIMP) dark matter
Dark matter
In astronomy and cosmology, dark matter is matter that neither emits nor scatters light or other electromagnetic radiation, and so cannot be directly detected via optical or radio astronomy...
candidate. The existence of a supersymmetric dark matter candidate is closely tied to R-parity
R-parity
R-parity is a concept in particle physics. In the supersymmetric extension of the Standard Model, baryon number and lepton number are no longer conserved by all of the renormalizable couplings...
.
The standard paradigm for incorporating supersymmetry into a realistic theory is to have the underlying dynamics of the theory be supersymmetric, but the ground state of the theory does not respect the symmetry and supersymmetry is broken spontaneously
Spontaneous symmetry breaking
Spontaneous symmetry breaking is the process by which a system described in a theoretically symmetrical way ends up in an apparently asymmetric state....
. The supersymmetry break can not be done permanently by the particles of the MSSM as they currently appear. This means that there is a new sector of the theory that is responsible for the breaking. The only constraint on this new sector is that it must break supersymmetry permanently and must give superparticles TeV scale masses. There are many models that can do this and most of their details do not currently matter. In order to parameterize the relevant features of supersymmetry breaking, arbitrary soft SUSY breaking
Soft SUSY breaking
In theoretical physics, soft SUSY breaking is type of supersymmetry breaking that does not cause ultraviolet divergences to appear in scalar masses. These terms are relevant operators—i.e. operators whose coefficients have a positive dimension of mass—though there are some exceptions.A model...
terms are added to the theory which temporarily break SUSY explicitly but could never arise from a complete theory of supersymmetry breaking.
Gauge Coupling Unification
One piece of evidence for supersymmetry existing is gauge coupling unification.The renormalization group
Renormalization group
In theoretical physics, the renormalization group refers to a mathematical apparatus that allows systematic investigation of the changes of a physical system as viewed at different distance scales...
evolution of the three gauge coupling constant
Coupling constant
In physics, a coupling constant, usually denoted g, is a number that determines the strength of an interaction. Usually the Lagrangian or the Hamiltonian of a system can be separated into a kinetic part and an interaction part...
s of the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
is somewhat sensitive to the present particle content of the theory. These coupling constants do not quite meet together at a common energy scale if we run the renormalization group using the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
. With the addition of minimal SUSY joint convergence of the coupling constants is projected at approximately 1016 GeV
GEV
GEV or GeV may stand for:*GeV or gigaelectronvolt, a unit of energy equal to billion electron volts*GEV or Grid Enabled Vehicle that is fully or partially powered by the electric grid, see plug-in electric vehicle...
.
Supersymmetric quantum mechanics
Supersymmetric quantum mechanics adds the SUSY superalgebra to quantum mechanicsQuantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
as opposed to quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
. Supersymmetric quantum mechanics often comes up when studying the dynamics of supersymmetric solitons and due to the simplified nature of having fields only functions of time (rather than space-time), a great deal of progress has been made in this subject and is now studied in its own right.
SUSY quantum mechanics involves pairs of Hamiltonians
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
which share a particular mathematical relationship, which are called partner Hamiltonians. (The potential energy
Potential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
terms which occur in the Hamiltonians are then called partner potentials.) An introductory theorem shows that for every eigenstate of one Hamiltonian, its partner Hamiltonian has a corresponding eigenstate with the same energy. This fact can be exploited to deduce many properties of the eigenstate spectrum. It is analogous to the original description of SUSY, which referred to bosons and fermions. We can imagine a "bosonic Hamiltonian", whose eigenstates are the various bosons of our theory. The SUSY partner of this Hamiltonian would be "fermionic", and its eigenstates would be the theory's fermions. Each boson would have a fermionic partner of equal energy.
SUSY concepts have provided useful extensions to the WKB approximation
WKB approximation
In mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear partial differential equations with spatially varying coefficients...
. In addition, SUSY has been applied to non-quantum statistical mechanics
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
through the Fokker-Planck equation
Fokker-Planck equation
The Fokker–Planck equation describes the time evolution of the probability density function of the velocity of a particle, and can be generalized to other observables as well.It is named after Adriaan Fokkerand Max Planck...
.
Mathematics
SUSY is also sometimes studied mathematically for its intrinsic properties. This is because it describes complex fields satisfying a property known as holomorphy, which allows holomorphic quantities to be exactly computed. This makes supersymmetric models useful toy modelToy model
In physics, a toy model is a simplified set of objects and equations relating them that can nevertheless be used to understand a mechanism that is also useful in the full, non-simplified theory....
s of more realistic theories. A prime example of this has been the demonstration of S-duality in four-dimensional gauge theories that interchanges particles and monopole
Monopole
Monopole may refer to:*Magnetic monopole, or Dirac monopole, a hypothetical particle that may be loosely described as a magnet with only one pole, or related concepts in physics and mathematics:...
s.
General supersymmetry
Supersymmetry appears in many different contexts in theoretical physics that are closely related. It is possible to have multiple supersymmetries and also have supersymmetric extra dimensions.Extended supersymmetry
It is possible to have more than one kind of supersymmetry transformation. Theories with more than one supersymmetry transformation are known as extended supersymmetric theories. The more supersymmetry a theory has, the more constrained the field content and interactions are. Typically the number of copies of a supersymmetry is a power of 2, i.e. 1, 2, 4, 8. In four dimensions, a spinor has four degrees of freedom and thus the minimal number of supersymmetry generators is four in four dimensions and having eight copies of supersymmetry means that there are 32 supersymmetry generators.The maximal number of supersymmetry generators possible is 32. Theories with more than 32 supersymmetry generators automatically have massless fields with spin greater than 2. It is not known how to make massless fields with spin greater than two interact, so the maximal number of supersymmetry generators considered is 32. This corresponds to an N = 8 supersymmetry theory. Theories with 32 supersymmetries automatically have a graviton
Graviton
In physics, the graviton is a hypothetical elementary particle that mediates the force of gravitation in the framework of quantum field theory. If it exists, the graviton must be massless and must have a spin of 2...
.
In four dimensions there are the following theories, with the corresponding multiplets(CPT adds a copy, whenever they are not invariant under such symmetry)
- N = 1
Chiral multiplet:
(0,)
Vector multiplet:
Gravitino multiplet:
(1,)
Graviton multiplet:
- N = 2
hypermultiplet:
(-,02,)
vector multiplet:
(0,2,1)
supergravity multiplet:
(1,2,2)
- N = 4
Vector multiplet:
(-1,-4,06,4,1)
Supergravity multiplet:
(0,4,16,4,2)
- N = 8
Supergravity multiplet:
(-2,-8,-128,-56,070,56,128,8,2)
Supersymmetry in alternate numbers of dimensions
It is possible to have supersymmetry in dimensions other than four. Because the properties of spinors change drastically between different dimensions, each dimension has its characteristic. In d dimensions, the size of spinors is roughly 2d/2 or 2(d − 1)/2. Since the maximum number of supersymmetries is 32, the greatest number of dimensions in which a supersymmetric theory can exist is eleven.Supersymmetry as a quantum group
Supersymmetry can be reinterpreted in the language of noncommutative geometryNoncommutative geometry
Noncommutative geometry is a branch of mathematics concerned with geometric approach to noncommutative algebras, and with construction of spaces which are locally presented by noncommutative algebras of functions...
and quantum group
Quantum group
In mathematics and theoretical physics, the term quantum group denotes various kinds of noncommutative algebra with additional structure. In general, a quantum group is some kind of Hopf algebra...
s. In particular, it involves a mild form of noncommutativity, namely supercommutativity. See the main article for more details.
Supersymmetry in quantum gravity
Supersymmetry is part of a larger enterprise of theoretical physics to unify everything we know about the physical world into a single fundamental framework of physical laws, known as the quest for a Theory of EverythingTheory of everything
A theory of everything is a putative theory of theoretical physics that fully explains and links together all known physical phenomena, and predicts the outcome of any experiment that could be carried out in principle....
(TOE). A significant part of this larger enterprise is the quest for a theory of quantum gravity
Quantum gravity
Quantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
, which would unify the classical theory of general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
and the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
, which explains the other three basic forces
Fundamental interaction
In particle physics, fundamental interactions are the ways that elementary particles interact with one another...
in physics (electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
, the strong interaction
Strong interaction
In particle physics, the strong interaction is one of the four fundamental interactions of nature, the others being electromagnetism, the weak interaction and gravitation. As with the other fundamental interactions, it is a non-contact force...
, and the weak interaction
Weak interaction
Weak interaction , is one of the four fundamental forces of nature, alongside the strong nuclear force, electromagnetism, and gravity. It is responsible for the radioactive decay of subatomic particles and initiates the process known as hydrogen fusion in stars...
), and provides a palette of fundamental particles upon which all four forces act. Two of the most active approaches to forming a theory of quantum gravity are string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
and loop quantum gravity
Loop quantum gravity
Loop quantum gravity , also known as loop gravity and quantum geometry, is a proposed quantum theory of spacetime which attempts to reconcile the theories of quantum mechanics and general relativity...
(LQG), although in theory, supersymmetry could be a component of other theoretical approaches as well.
For string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
to be consistent, supersymmetry appears to be required at some level (although it may be a strongly broken symmetry). In particle theory, supersymmetry is recognized as a way to stabilize the hierarchy
Hierarchy problem
In theoretical physics, a hierarchy problem occurs when the fundamental parameters of some Lagrangian are vastly different than the parameters measured by experiment. This can happen because measured parameters are related to the fundamental parameters by a prescription known as renormalization...
between the unification scale and the electroweak scale (or the Higgs boson
Higgs boson
The Higgs boson is a hypothetical massive elementary particle that is predicted to exist by the Standard Model of particle physics. Its existence is postulated as a means of resolving inconsistencies in the Standard Model...
mass), and can also provide a natural dark matter
Dark matter
In astronomy and cosmology, dark matter is matter that neither emits nor scatters light or other electromagnetic radiation, and so cannot be directly detected via optical or radio astronomy...
candidate. String theory also requires extra spatial dimensions which have to be compactified as in Kaluza-Klein theory.
Loop quantum gravity
Loop quantum gravity
Loop quantum gravity , also known as loop gravity and quantum geometry, is a proposed quantum theory of spacetime which attempts to reconcile the theories of quantum mechanics and general relativity...
(LQG), in its current formulation, predicts no additional spatial dimensions, nor anything else about particle physics. These theories can be formulated in three spatial dimensions and one dimension of time, although in some LQG theories dimensionality is an emergent property of the theory, rather than a fundamental assumption of the theory. Also, LQG is a theory of quantum gravity which does not require supersymmetry. Lee Smolin
Lee Smolin
Lee Smolin is an American theoretical physicist, a researcher at the Perimeter Institute for Theoretical Physics, and an adjunct professor of physics at the University of Waterloo. He is married to Dina Graser, a communications lawyer in Toronto. His brother is David M...
, one of the originators of LQG, has proposed that a loop quantum gravity theory incorporating either supersymmetry or extra dimensions, or both, be called "loop quantum gravity II".
If experimental evidence confirms supersymmetry in the form of supersymmetric particles such as the neutralino
Neutralino
In particle physics, the neutralino is a hypothetical particle predicted by supersymmetry. There are four neutralinos that are fermions and are electrically neutral, the lightest of which is typically stable...
that is often believed to be the lightest superpartner
Superpartner
In particle physics, a superpartner is a hypothetical elementary particle. Supersymmetry is one of the synergistic theories in current high-energy physics which predicts the existence of these "shadow" particles....
, some people believe this would be a major boost to string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
. Since supersymmetry is a required component of string theory, any discovered supersymmetry would be consistent with string theory. If the Large Hadron Collider
Large Hadron Collider
The Large Hadron Collider is the world's largest and highest-energy particle accelerator. It is expected to address some of the most fundamental questions of physics, advancing the understanding of the deepest laws of nature....
and other major particle physics experiments fail to detect supersymmetric partners or evidence of extra dimensions, many versions of string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
which had predicted certain low mass superpartners to existing particles may need to be significantly revised. The failure of experiments to discover either supersymmetric partners or extra spatial dimensions, , has encouraged loop quantum gravity
Loop quantum gravity
Loop quantum gravity , also known as loop gravity and quantum geometry, is a proposed quantum theory of spacetime which attempts to reconcile the theories of quantum mechanics and general relativity...
researchers.
Current Limits
The tightest limits will of course come from direct production at colliders. Both the Large Electron–Positron Collider and TevatronTevatron
The Tevatron is a circular particle accelerator in the United States, at the Fermi National Accelerator Laboratory , just east of Batavia, Illinois, and is the second highest energy particle collider in the world after the Large Hadron Collider...
had set limits for specific models which have now been exceeded by the Large Hadron Collider
Large Hadron Collider
The Large Hadron Collider is the world's largest and highest-energy particle accelerator. It is expected to address some of the most fundamental questions of physics, advancing the understanding of the deepest laws of nature....
. Searches are only applicable for a finite set of tested points because simulation using the Monte Carlo method
Monte Carlo method
Monte Carlo methods are a class of computational algorithms that rely on repeated random sampling to compute their results. Monte Carlo methods are often used in computer simulations of physical and mathematical systems...
must be made so that limits for that particular model can be calculated. This complicates matters because different experiments have looked at different sets of points. Some extrapolation between points can be made within particular models but it is difficult to set general limits even for the Minimal Supersymmetric Standard Model
Minimal Supersymmetric Standard Model
The Minimal Supersymmetric Standard Model is the minimal extension to the Standard Model that realizes supersymmetry, although non-minimal extensions do exist. Supersymmetry pairs bosons with fermions; therefore every Standard Model particle has a partner that has yet to be discovered...
.
The first mass limits for squarks and gluinos were made at CERN
CERN
The European Organization for Nuclear Research , known as CERN , is an international organization whose purpose is to operate the world's largest particle physics laboratory, which is situated in the northwest suburbs of Geneva on the Franco–Swiss border...
by the UA1 experiment and the UA2 experiment at the Super Proton Synchrotron
Super Proton Synchrotron
The Super Proton Synchrotron is a particle accelerator of the synchrotron type at CERN. It is housed in a circular tunnel, in circumference, straddling the border of France and Switzerland near Geneva, Switzerland. The SPS was designed by a team led by John Adams, director-general of what was...
. LEP later set very strong limits. In 2006 these limits were extended by the D0 experiment The LHC has now extended these limits, by extending the search area, with no sign of supersymmmetry.
Further reading
- A Supersymmetry Primer by S. Martin, 2011
- Introduction to Supersymmetry By Joseph D. LykkenJoseph LykkenJoseph David Lykken is a theoretical physicist at the Fermi National Accelerator Laboratory.-Background and education:...
, 1996 - An Introduction to Supersymmetry By Manuel Drees, 1996
- Introduction to Supersymmetry By Adel Bilal, 2001
- An Introduction to Global Supersymmetry by Philip Arygres, 2001
- Weak Scale Supersymmetry by Howard Baer and Xerxes Tata, 2006.
- Cooper, F., A. Khare and U. Sukhatme. "Supersymmetry in Quantum Mechanics." Phys. Rep. 251 (1995) 267-85 (arXiv:hep-th/9405029).
- Junker, G. Supersymmetric Methods in Quantum and Statistical Physics, Springer-Verlag (1996).
- Gordon L. Kane.Supersymmetry: Unveiling the Ultimate Laws of Nature Basic Books, New York (2001). ISBN 0-7382-0489-7.
- Gordon L. Kane and Shifman, M., eds. The Supersymmetric World: The Beginnings of the Theory, World Scientific, Singapore (2000). ISBN 981-02-4522-X.
- D.V. Volkov, V.P. Akulov, Pisma Zh.Eksp.Teor.Fiz. 16 (1972) 621; Phys.Lett. B46 (1973) 109.
- V.P. Akulov, D.V. Volkov, Teor.Mat.Fiz. 18 (1974) 39.
- Weinberg, Steven, The Quantum Theory of Fields, Volume 3: Supersymmetry, Cambridge University Press, Cambridge, (1999). ISBN 0-521-66000-9.
- Wess, Julius, and Jonathan Bagger, Supersymmetry and Supergravity, Princeton University Press, Princeton, (1992). ISBN 0-691-02530-4.
- Brookhaven National Laboratory (Jan. 8, 2004). New g−2 measurement deviates further from Standard Model. Press Release.
- Fermi National Accelerator Laboratory (Sept 25, 2006). Fermilab's CDF scientists have discovered the quick-change behavior of the B-sub-s meson. Press Release.
External links
- What do current LHC results (mid-August 2011) imply about supersymmetry? Matt Strassler
- ATLAS Experiment Supersymmetry search documents
- CMS Experiment Supersymmetry search documents
- "Particle wobble shakes up supersymmetry", Cosmos magazine, September 2006
- LHC results put supersymmetry theory 'on the spot' BBC news 27/8/2011