Gauss's Theorema Egregium (Latin: "Remarkable Theorem") is a foundational result in differential geometry proved by Carl Friedrich Gauss

Carl Friedrich Gauss

Johann Carl Friedrich Gauss was a German mathematician and scientist who contributed significantly to many fields, including number theory, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy and optics.Sometimes referred to as the Princeps mathematicorum...

 that concerns the curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

 of surfaces. The theorem says that the Gaussian curvature

Gaussian curvature

In differential geometry, the Gaussian curvature or Gauss curvature of a point on a surface is the product of the principal curvatures, κ1 and κ2, of the given point. It is an intrinsic measure of curvature, i.e., its value depends only on how distances are measured on the surface, not on the way...

 of a surface can be determined entirely by measuring angles, distances and their rates on the surface itself, without further reference to the particular way in which the surface is embedded

Embedding

In mathematics, an embedding is one instance of some mathematical structure contained within another instance, such as a group that is a subgroup....

 in the ambient 3-dimensional Euclidean space. Thus the Gaussian curvature is an intrinsic invariant

Invariant (mathematics)

In mathematics, an invariant is a property of a class of mathematical objects that remains unchanged when transformations of a certain type are applied to the objects. The particular class of objects and type of transformations are usually indicated by the context in which the term is used...

 of a surface.

Gauss presented the theorem in this way (translated from Latin):

Thus the formula of the preceding article leads itself to the remarkable Theorem. If a curved surface is developed upon any other surface whatever, the measure of curvature in each point remains unchanged.

The theorem is "remarkable" because the starting definition of Gaussian curvature makes direct use of position of the surface in space. So it is quite surprising that the end result does not depend on its embedding in spite of all bending and twisting deformations undergone.

In modern mathematical language, the theorem may be stated as follows:

The Gaussian curvature

Gaussian curvature

In differential geometry, the Gaussian curvature or Gauss curvature of a point on a surface is the product of the principal curvatures, κ1 and κ2, of the given point. It is an intrinsic measure of curvature, i.e., its value depends only on how distances are measured on the surface, not on the way...

 of a surface is invariant under local isometry

Isometry (Riemannian geometry)

In the study of Riemannian geometry in mathematics, a local isometry from one Riemannian manifold to another is a map which pulls back the metric tensor on the second manifold to the metric tensor on the first...

.

Elementary applications

A sphere

Sphere

A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...

 of radius R has constant Gaussian curvature which is equal to 1/R². At the same time, a plane has zero Gaussian curvature. As a corollary of Theorema Egregium, a piece of paper cannot be bent onto a sphere without crumpling. Conversely, the surface of a sphere cannot be unfolded onto a flat plane without distorting the distances. If one were to step on an empty egg shell, its edges have to split in expansion before being flattened. Mathematically speaking, a sphere and a plane are not isometric

Isometry

In mathematics, an isometry is a distance-preserving map between metric spaces. Geometric figures which can be related by an isometry are called congruent.Isometries are often used in constructions where one space is embedded in another space...

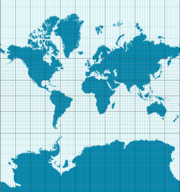
, even locally. This fact is of enormous significance for cartography

Cartography

Cartography is the study and practice of making maps. Combining science, aesthetics, and technique, cartography builds on the premise that reality can be modeled in ways that communicate spatial information effectively.The fundamental problems of traditional cartography are to:*Set the map's...

: it implies that no planar (flat) map of Earth can be perfect, even for a portion of the Earth's surface. Thus every cartographic projection

Map projection

A map projection is any method of representing the surface of a sphere or other three-dimensional body on a plane. Map projections are necessary for creating maps. All map projections distort the surface in some fashion...

 necessarily distorts at least some distances.

The catenoid

Catenoid

A catenoid is a three-dimensional surface made by rotating a catenary curve about its directrix. Not counting the plane, it is the first minimal surface to be discovered. It was found and proved to be minimal by Leonhard Euler in 1744. Early work on the subject was published also by Jean Baptiste...

 and the helicoid

Helicoid

The helicoid, after the plane and the catenoid, is the third minimal surface to be known. It was first discovered by Jean Baptiste Meusnier in 1776. Its name derives from its similarity to the helix: for every point on the helicoid there is a helix contained in the helicoid which passes through...

 are two very different-looking surfaces. Nevertheless, each of them can be continuously bent into the other: they are locally isometric. It follows from Theorema Egregium that under this bending the Gaussian curvature at any two corresponding points of the catenoid and helicoid is always the same. Thus isometry is simply bending and twisting of a surface without internal crumpling or tearing, in other words without extra tension, compression or shear.

An application of the Theorema Egregium is seen in a common pizza

Pizza

Pizza is an oven-baked, flat, disc-shaped bread typically topped with a tomato sauce, cheese and various toppings.Originating in Italy, from the Neapolitan cuisine, the dish has become popular in many parts of the world. An establishment that makes and sells pizzas is called a "pizzeria"...

-eating strategy: A slice of pizza can be seen as a surface with constant Gaussian curvature 0. Gently bending a slice must then roughly maintain this curvature (assuming the bend is roughly a local isometry). If one bends a slice horizontally along a radius, non-zero principal curvatures are created along the bend, dictating that the other principal curvature at these points must be zero. This creates rigidity in the direction perpendicular to the fold, an attribute desirable when eating pizza, as it holds its shape long enough to be consumed without a mess. This same principle is used for strengthening in corrugated

Corrugated

The term corrugated, describing a series of parallel ridges and furrows, may refer to the following:*Corrugated fiberboard also called corrugated cardboard*Corrugated galvanised iron*Corrugated plastic*Corrugated Frog...

 materials, most familiarly corrugated fiberboard and corrugated galvanised iron

Corrugated galvanised iron

Corrugated galvanised iron is a building material composed of sheets of hot-dip galvanised mild steel, cold-rolled to produce a linear corrugated pattern in them...

.

See also

• Second fundamental form
• Gaussian curvature
  Gaussian curvature
  In differential geometry, the Gaussian curvature or Gauss curvature of a point on a surface is the product of the principal curvatures, κ1 and κ2, of the given point. It is an intrinsic measure of curvature, i.e., its value depends only on how distances are measured on the surface, not on the way...
  
  
• Differential geometry of surfaces
  Differential geometry of surfaces
  In mathematics, the differential geometry of surfaces deals with smooth surfaces with various additional structures, most often, a Riemannian metric....