
Triangular orthobicupola
Encyclopedia
In geometry
, the triangular orthobicupola is one of the Johnson solid
s (J27). As the name suggests, it can be constructed by attaching two triangular cupola
s (J3) along their bases. It has an equal number of squares and triangles at each vertex; however, it is not vertex-transitive
.
The triangular orthobicupola is the first in an infinite set of orthobicupolae
.
The triangular orthobicupola has a superficial resemblance to the cuboctahedron
, which would be known as the triangular gyrobicupola in the nomenclature of Johnson solids — the difference is that the two triangular cupolas which make up the triangular orthobicupola are joined so that pairs of matching sides abut (hence, "ortho"); the cuboctahedron is joined so that triangles abut squares and vice versa. Given a triangular orthobicupola, a 60-degree rotation of one cupola before the joining yields a cuboctahedron.
The elongated triangular orthobicupola
(J35), which is constructed by elongating this solid, has a (different) special relationship with the rhombicuboctahedron
.
The 92 Johnson solids were named and described by Norman Johnson in 1966.
The dual of the triangular orthobicupola is called a trapezoid-rhombic dodecahedron. It has 6 rhombic and 6 trapezoidal faces. It is similar to the rhombic dodecahedron
and both of them are space-filling polyhedra.
e for volume
, surface area
, and circumradius
can be used if all faces
are regular
, with edge length a:
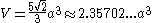
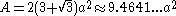
The circumradius of a triangular orthobicupola is the same as the edge length (C=a).
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the triangular orthobicupola is one of the Johnson solid
Johnson solid
In geometry, a Johnson solid is a strictly convex polyhedron, each face of which is a regular polygon, but which is not uniform, i.e., not a Platonic solid, Archimedean solid, prism or antiprism. There is no requirement that each face must be the same polygon, or that the same polygons join around...
s (J27). As the name suggests, it can be constructed by attaching two triangular cupola
Triangular cupola
In geometry, the triangular cupola is one of the Johnson solids . It can be seen as half a cuboctahedron.The 92 Johnson solids were named and described by Norman Johnson in 1966.-Formulae:...
s (J3) along their bases. It has an equal number of squares and triangles at each vertex; however, it is not vertex-transitive
Vertex-transitive
In geometry, a polytope is isogonal or vertex-transitive if, loosely speaking, all its vertices are the same...
.
The triangular orthobicupola is the first in an infinite set of orthobicupolae
Bicupola (geometry)
In geometry, a bicupola is a solid formed by connecting two cupolae on their bases.There are two classes of bicupola because each cupola half is bordered by alternating triangles and squares...
.
The triangular orthobicupola has a superficial resemblance to the cuboctahedron
Cuboctahedron
In geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron,...
, which would be known as the triangular gyrobicupola in the nomenclature of Johnson solids — the difference is that the two triangular cupolas which make up the triangular orthobicupola are joined so that pairs of matching sides abut (hence, "ortho"); the cuboctahedron is joined so that triangles abut squares and vice versa. Given a triangular orthobicupola, a 60-degree rotation of one cupola before the joining yields a cuboctahedron.
The elongated triangular orthobicupola
Elongated triangular orthobicupola
In geometry, the elongated triangular orthobicupola is one of the Johnson solids . As the name suggests, it can be constructed by elongating a triangular orthobicupola by inserting a hexagonal prism between its two halves...
(J35), which is constructed by elongating this solid, has a (different) special relationship with the rhombicuboctahedron
Rhombicuboctahedron
In geometry, the rhombicuboctahedron, or small rhombicuboctahedron, is an Archimedean solid with eight triangular and eighteen square faces. There are 24 identical vertices, with one triangle and three squares meeting at each. Note that six of the squares only share vertices with the triangles...
.
The 92 Johnson solids were named and described by Norman Johnson in 1966.
The dual of the triangular orthobicupola is called a trapezoid-rhombic dodecahedron. It has 6 rhombic and 6 trapezoidal faces. It is similar to the rhombic dodecahedron
Rhombic dodecahedron
In geometry, the rhombic dodecahedron is a convex polyhedron with 12 rhombic faces. It is an Archimedean dual solid, or a Catalan solid. Its dual is the cuboctahedron.-Properties:...
and both of them are space-filling polyhedra.
Formulae
The following formulaFormula
In mathematics, a formula is an entity constructed using the symbols and formation rules of a given logical language....
e for volume
Volume
Volume is the quantity of three-dimensional space enclosed by some closed boundary, for example, the space that a substance or shape occupies or contains....
, surface area
Surface area
Surface area is the measure of how much exposed area a solid object has, expressed in square units. Mathematical description of the surface area is considerably more involved than the definition of arc length of a curve. For polyhedra the surface area is the sum of the areas of its faces...
, and circumradius
Circumscribed sphere
In geometry, a circumscribed sphere of a polyhedron is a sphere that contains the polyhedron and touches each of the polyhedron's vertices. The word circumsphere is sometimes used to mean the same thing...
can be used if all faces
Face (geometry)
In geometry, a face of a polyhedron is any of the polygons that make up its boundaries. For example, any of the squares that bound a cube is a face of the cube...
are regular
Regular polygon
A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...
, with edge length a:
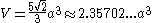
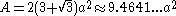
The circumradius of a triangular orthobicupola is the same as the edge length (C=a).

