Wind turbine aerodynamics
Encyclopedia
The primary application of wind turbines is to extract energy from the wind. Hence, the aerodynamics is a very important aspect of wind turbines. Like many machines, there are many different types all based on different energy extraction concepts. Similarly, the aerodynamics of one wind turbine to the next can be very different.
Overall the details of the aerodynamics depend very much on the topology. There are still some fundamental concepts that apply to all turbines. Every topology has a maximum power for a given flow, and some topologies are better than others. The method used to extract power has a strong influence on this. In general all turbines can be grouped as being lift
based, or drag
based with the former being more efficient. The difference between these groups is the aerodynamic force that is used to extract the energy.
The most common topology is the Horizontal-axis wind turbine. It is a lift based wind turbine with very good performance, accordingly it is a popular for commercial applications and much research has been applied to this turbine. In the latter part of the 20th century the Darrieus wind turbine
was another popular lift based alternative but is rarely used today. The Savonius wind turbine
is the most common drag type turbine, despite its low efficiency it is used because it is simple to build and maintain and very robust.
The force F is generated by the wind interacting with the blade. The primary focus of wind turbine aerodynamics is the magnitude and distribution of this force. The most familiar type of aerodynamic force is Drag, this is the same force that is felt pushing against you on a windy day. Another type of force is lift, this is the same force that allow most aircraft to fly. The direction of the drag force is parallel to the relative wind, while the lift force is perpendicular. Typically, the wind turbine parts are moving so this alters the flow around the part. An example of relative wind is the wind one would feel cycling on a calm day.
To extract power, the turbine part must move in the direction of the force. In the drag force case, the relative wind speed decreases subsequently so does the drag force. The relative wind aspect dramatically limits the maximum power that can be extracted by a drag based wind turbine. Lift based wind turbine typically have lifting surfaces moving perpendicular to the flow. Here, the relative wind will not decrease in fact it increases with rotor speed. Thus the maximum power limits of these machines is much higher than drag based machines.
is applied to various qualities. One of the qualities of nondimensionalization is that when geometrically similar turbines will produce the same non-dimensional results, while because of other factors (difference in scale, wind properties) produce very different dimensional properties. This allows one to make comparisons between different turbines, while eliminating the effect of things like size and wind conditions from the comparison.
The coefficient of power is the most important variable in wind turbine aerodynamics. Buckingham π theorem can be applied to show that non-dimensional variable for power is given by the equation below. This equation is similar to efficiency, so values between 0 and less than one are typical. However this is not the exactly the same as efficiency so in practice some turbines can exhibit greater than unity power coefficients. In these circumstances one cannot conclude the first law of thermodynamics is violated because this is not an efficiency term by the strict definition of efficiency.
Equation shows two important dependents. The first is the speed (U) that the machine is going at. The speed at the tip of the blade is usually used for this purpose, and is written as the product of the blade radius and the rotational speed of the wind (U=omega*r).[please clarify] This variable is nondimensionalized by the wind speed, to get the speed ratio:
The force vector is not straightforward, as stated earlier there are two types of aerodynamic forces, lift and drag. Accordingly there are two non-dimensional parameters. However both variables are non-dimensionalized in a similar way. The formula for lift is given below, the formula for drag is given after:
The aerodynamic forces have a dependency on W, this speed is the relative speed and it is given by the equation below. Note that this is vector subtraction.
The formulas and are applied to express in nondimensional form:
It can be shown through calculus that equation achieves a maximum at
. By inspection one can see that equation will achieve larger values for 
. In these circumstances, the scalar product in equation makes the result negative. Thus, one can conclude that the maximum power is given by:
Experimentally it has been determined that a large
is 1.2, thus the maximum 
is approximately 0.1778.
Similarly, this is non-dimensionalized with equations and . However in this derivation the parameter
is also used:
Solving the optimal speed ratio is complicated by the dependency on
and the fact that the optimal speed ratio is a solution to a cubic polynomial. Numerical methods can then be applied to determine this solution and the corresponding 
solution for a range of 
results. Some sample solutions are given in the table below.
Experiments have shown that it is not unreasonable to achieve a drag ratio (
) of approximately 0.01 at a lift coefficient of 0.6. This would give a 
of about 889. This is substantially better than the best drag based machine, hence why lift based machines are superior.
In the analysis given here, there is an inconsistency compared to typical wind turbine non-dimensionalization. As stated in the preceding section the A in the
non-dimensionalization is not always the same as the A in the force equations and . Typically for 
the A is the area swept by the rotor blade in its motion. For 
and 
A is the area of the turbine wing section. For drag based machines, these two areas are almost identical so there is little difference. To make the lift based results comparable to the drag results, the area of the wing section was used to non-dimensionalize power. The results here could be interpreted as power per unit of material. Given that the material represents the cost (wind is free), this is a better variable for comparison.
If one were to apply conventional non-dimensionalization, more information on the motion of the blade would be required. However the discussion on Horizontal Axis Wind Turbines will show that the maximum
there is 16/27. Thus, even by conventional non-dimensional analysis lift based machines are superior to drag based machines.
There are several idealizations to the analysis. In any lift based machine (aircraft included) with finite wings, there is a wake that affects the incoming flow and creates induced drag. This phenomenon exists in wind turbines and was neglected in this analysis. Including induced drag requires information specific to the topology, In these cases it is expected that both the optimal speed ratio and the optimal
would be less. The analysis focused on the aerodynamic potential, but neglected structural aspects. In reality most optimal wind turbine design becomes a compromise between optimal aerodynamic design, and optimal structural design.
of a wind turbine
at the rotor surface exhibit phenomena that are rarely seen in other aerodynamic fields.
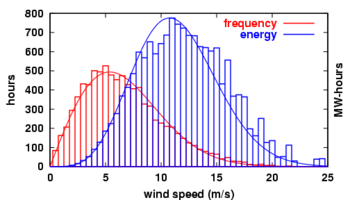Energy in fluid is contained in four different forms: gravitational potential energy, thermodynamic pressure
, kinetic energy
from the velocity and finally thermal energy
. Gravitational and thermal energy have a negligible effect on the energy extraction process. From a macroscopic point of view, the air flow about the wind turbine is at atmospheric pressure. If pressure is constant then only kinetic energy is extracted. However up close near the rotor itself the air velocity is constant as it passes through the rotor plane. This is because of conservation of mass
. The air that passes through the rotor cannot slow down because it needs to stay out of the way of the air behind it. So at the rotor the energy is extracted by a pressure drop. The air directly behind the wind turbine is at sub-atmospheric pressure
; the air in front is under greater than atmospheric pressure. It is this high pressure in front of the wind turbine that deflects some of the upstream air around the turbine.
Frederick W. Lanchester was the first to study this phenomenon in application to ship propellers, five years later Nikolai Yegorovich Zhukovsky and Albert Betz
independently arrived at the same results . It is believed that each researcher was not aware of the others work because of World War One and the Bolshevik Revolution. Thus formally, the proceeding limit should be referred to as the Lanchester-Betz-Joukowsky limit. In general Albert Betz
is credited for this accomplishment because he published his work in a journal that had a wider circulation, while the other two published it in the publication associated with their respective institution, thus it is widely known as simply the Betz Limit.
This is derived by looking at the axial momentum of the air passing through the wind turbine. As stated above some of the air is deflected away from the turbine. This causes the air passing through the rotor plane to have a smaller velocity than the free stream velocity. The ratio of this reduction to that of the air velocity far away from the wind turbine is called the axial induction factor. It is defined as below:
The first step to deriving the Betz limit is applying conservation of axial momentum
. As stated above the wind loses speed after the wind turbine compared to the speed far away from the turbine. This would violate the conservation of momentum if the wind turbine was not applying a thrust force on the flow. This thrust force manifests itself through the pressure drop across the rotor. The front operates at high pressure while the back operates at low pressure. The pressure difference from the front to back causes the thrust force. The momentum lost in the turbine is balanced by the thrust force.
Another equation is needed to relate the pressure difference to the velocity of the flow near the turbine. Here the Bernoulli equation
is used between the field flow and the flow near the wind turbine. There is one limitation to the Bernoulli equation: the equation cannot be applied to fluid passing through the wind turbine. Instead conservation of mass is used to relate the incoming air to the outlet air. Betz used these equations and managed to solve the velocities of the flow in the far wake and near the wind turbine in terms of the far field flow and the axial induction factor. The velocities are given below as:
U4 is introduced here as the wind velocity in the far wake. This is important because the power extracted from the turbine is defined by the following equation. However the Betz limit is given in terms of the coefficient of power
. The coefficient of power is similar to efficiency but not the same. The formula for the coefficient of power is given beneath the formula for power:
Betz was able to develop an expression for
in terms of the induction factors. This is done by the velocity relations being substituted into power and power is substituted into the coefficient of power definition. The relationship Betz developed is given below:
The Betz limit is defined by the maximum value that can be given by the above formula. This is found by taking the derivative with respect to the axial induction factor, setting it to zero and solving for the axial induction factor. Betz was able to show that the optimum axial induction factor is one third. The optimum axial induction factor was then used to find the maximum coefficient of power. This maximum coefficient is the Betz limit. Betz was able to show that the maximum coefficient of power of a wind turbine is 16/27. Airflow operating at higher thrust will cause the axial induction factor to rise above the optimum value. Higher thrust cause more air to be deflected away from the turbine. When the axial induction factor falls below the optimum value the wind turbine is not extracting all the energy it can. This reduces pressure around the turbine and allows more air to pass through the turbine, but not enough to account for lack of energy being extracted.
The derivation of the Betz limit shows a simple analysis of wind turbine aerodynamics. In reality there is a lot more. A more rigorous analysis would include wake rotation, the effect of variable geometry. The effect of airfoils on the flow is a major component of wind turbine aerodynamics. Within airfoils alone, the wind turbine aerodynamicist has to consider the effect of surface roughness, dynamic stall tip losses, solidity, among other problems.
s and no losses. The tip speed ratio is ratio of the speed of the tip relative to the free stream flow. This turbine is not too far from actual wind turbines. Actual turbines are rotating blades. They typically operate at high tip speed ratios. At high tip speed ratios three blades are sufficient to interact with all the air passing through the rotor plane. Actual turbines still produce considerable thrust forces.
One key difference between actual turbines and the actuator disk, is that the energy is extracted through torque. The wind imparts a torque on the wind turbine, thrust is a necessary by-product of torque. Newtonian physics dictates that for every action there is an equal and opposite reaction. If the wind imparts a torque on the blades then the blades must be imparting a torque on the wind. This torque would then cause the flow to rotate. Thus the flow in the wake has two components, axial and tangential. This tangential flow is referred to as wake rotation.
Torque is necessary for energy extraction. However wake rotation is considered a loss. Accelerating the flow in the tangential direction increases the absolute velocity. This in turn increases the amount of kinetic energy in the near wake. This rotational energy is not dissipated in any form that would allow for a greater pressure drop (Energy extraction). Thus any rotational energy in the wake is energy that is lost and unavailable.
This loss is minimized by allowing the rotor to rotate very quickly. To the observer it may seem like the rotor is not moving fast; however, it is common for the tips to be moving through the air at 6 times the speed of the free stream. Newtonian mechanics defines power as torque multiplied by the rotational speed. The same amount of power can be extracted by allowing the rotor to rotate faster and produce less torque. Less torque means that there is less wake rotation. Less wake rotation means there is more energy available to extract.
(HAWT) aerodynamics is Blade Element
Momentum (BEM) theory
. The theory is based on the assumption that the flow at a given annulus does not affect the flow at adjacent annuli. This allows the rotor blade to be analyzed in sections, where the resulting forces are summed over all sections to get the overall forces of the rotor. The theory uses both axial and angular momentum balances to determine the flow and the resulting forces at the blade.
The momentum equations for the far field flow dictate that the thrust and torque will induce a secondary flow in the approaching wind. This in turn affects the flow geometry at the blade. The blade itself is the source of these thrust and torque forces. The force response of the blades is governed by the geometry of the flow, or better known as the angle of attack. Refer to the Airfoil
article for more information on how airfoils create lift and drag forces at various angles of attack. This interplay between the far field momentum balances and the local blade forces requires one to solve the momentum equations and the airfoil equations simultaneously. Typically computers and numerical methods are employed to solve these models.
There is a lot of variation between different version of BEM theory. First, one can consider the effect of wake rotation or not. Second, one can go further and consider the pressure drop induced in wake rotation. Third, the tangential induction factors can be solved with a momentum equation, an energy balance or orthognal geometric constraint; the latter a result of Biot-Savart law
in vortex methods. These all lead to different set of equations that need to be solved. The simplest and most widely used equations are those that consider wake rotation with the momentum equation but ignore the pressure drop from wake rotation. Those equations are given below. a is the axial component of the induced flow, a' is the tangential component of the induced flow.
is the solidity of the rotor, 
is the local inflow angle. 
and 
are the coefficient of normal force and the coefficient of tangential force respectively. Both these coefficients are defined with the resulting lift and drag coefficients of the airfoil:
The effect of the discrete number of blades is dealt with by applying the Prandtl tip loss factor. The most common form of this factor is given below where B is the number of blades, R is the outer radius and r is the local radius. The definition of F is based on actuator disk models and not directly applicable to BEM. However the most common application multiplies induced velocity term by F in the momentum equations. As in the momentum equation there are many variations for applying F, some argue that the mass flow should be corrected in either the axial equation, or both axial and tangential equations. Others have suggested a second tip loss term to account for the reduced blade forces at the tip. Shown below are the above momentum equations with the most common application of 'F':
The typical momentum theory applied in BEM is only effective for axial induction factors up to 0.4 (thrust coefficient of 0.96). Beyond this point the wake collapses and turbulent mixing occurs. This state is highly transient and largely unpredictable by theoretical means. Accordingly, several empirical relations have been developed. As the usual case there are several version, however a simple one that is commonly used is a linear curve fit given below, with
. The turbulent wake function given excludes the tip loss function, however the tip loss is applied simply by multiplying the resulting axial induction by the tip loss function.
The terms
and 
represent different quantities. The first one is the thrust coefficient of the rotor, which is the one which should be corrected for high rotor loading (i.e. for high values of 
), while the second one (
) is the tangential aerodynamic coefficient of an individual blade element, which is given by the aerodynamic lift and drag coefficients.
solvers based on Reynolds Averaged Navier Stokes
(RANS) and other similar three-dimensional models such as free vortex methods. These are very computationally intensive simulations to perform for several reasons. First, the solver must accurately model the far-field flow conditions, which can extend several rotor diameters up- and down-stream and include atmospheric boundary layer turbulence, while at the same time resolving the small-scale boundary-layer
flow conditions at the blades' surface (necessary to capture blade stall). In addition, many CFD solvers have difficulty meshing parts that move and deform, such as the rotor blades. Finally, there are many dynamic flow phenomena that are not easily modelled by RANS, such as dynamic stall and tower shadow. Due to the computational complexity, it is not currently practical to use these advanced methods for wind turbine design, though research continues in these and other areas related to helicopter and wind turbine aerodynamics.
Free vortex models (FVM) and Lagrangian particle vortex methods (LPVM) are both active areas of research that seek to increase modelling accuracy by accounting for more of the three-dimensional and unsteady flow effects than either BEM or RANS. FVM is similar to lifting line theory in that it assumes that the wind turbine rotor is shedding either a continuous vortex filament from the blade tips (and often the root), or a continuous vortex sheet from the blades' trailing edges. LPVM can use a variety of methods to introduce vorticity into the wake. Biot-Savart summation is used to determine the induced flow field of these wake vorticies' circulations, allowing for better approximations of the local flow over the rotor blades. These methods have largely confirmed much of the applicability of BEM and shed insight into the structure of wind turbine wakes. FVM has limitations due to its origin in potential flow theory, such as not explicitly modelling model viscous behavior, though LPVM is a fully viscous method. LPVM is more computationally intensive than either FVM or RANS, and FVM still relies on blade element theory for the blade forces.
Overall the details of the aerodynamics depend very much on the topology. There are still some fundamental concepts that apply to all turbines. Every topology has a maximum power for a given flow, and some topologies are better than others. The method used to extract power has a strong influence on this. In general all turbines can be grouped as being lift
Lift (force)
A fluid flowing past the surface of a body exerts a surface force on it. Lift is the component of this force that is perpendicular to the oncoming flow direction. It contrasts with the drag force, which is the component of the surface force parallel to the flow direction...
based, or drag
Drag (physics)
In fluid dynamics, drag refers to forces which act on a solid object in the direction of the relative fluid flow velocity...
based with the former being more efficient. The difference between these groups is the aerodynamic force that is used to extract the energy.
The most common topology is the Horizontal-axis wind turbine. It is a lift based wind turbine with very good performance, accordingly it is a popular for commercial applications and much research has been applied to this turbine. In the latter part of the 20th century the Darrieus wind turbine
Darrieus wind turbine
The Darrieus wind turbine is a type of vertical axis wind turbine used to generate electricity from the energy carried in the wind. The turbine consists of a number of aerofoils usually—but not always—vertically mounted on a rotating shaft or framework...
was another popular lift based alternative but is rarely used today. The Savonius wind turbine
Savonius wind turbine
Savonius wind turbines are a type of vertical-axis wind turbine , used for converting the force of the wind into torque on a rotating shaft...
is the most common drag type turbine, despite its low efficiency it is used because it is simple to build and maintain and very robust.
General aerodynamic considerations
The governing equation for power extraction is given below:- where: P is the power, F is the force vector, and U is the speed of the moving wind turbine part.
The force F is generated by the wind interacting with the blade. The primary focus of wind turbine aerodynamics is the magnitude and distribution of this force. The most familiar type of aerodynamic force is Drag, this is the same force that is felt pushing against you on a windy day. Another type of force is lift, this is the same force that allow most aircraft to fly. The direction of the drag force is parallel to the relative wind, while the lift force is perpendicular. Typically, the wind turbine parts are moving so this alters the flow around the part. An example of relative wind is the wind one would feel cycling on a calm day.
To extract power, the turbine part must move in the direction of the force. In the drag force case, the relative wind speed decreases subsequently so does the drag force. The relative wind aspect dramatically limits the maximum power that can be extracted by a drag based wind turbine. Lift based wind turbine typically have lifting surfaces moving perpendicular to the flow. Here, the relative wind will not decrease in fact it increases with rotor speed. Thus the maximum power limits of these machines is much higher than drag based machines.
Characteristic parameters
Different wind turbines will come in different sizes. Then once the wind turbine is operating it will experience a wide range of conditions. This variability complicates the comparison of different types of turbines. To deal with this, nondimensionalizationNondimensionalization
Nondimensionalization is the partial or full removal of units from an equation involving physical quantities by a suitable substitution of variables. This technique can simplify and parameterize problems where measured units are involved. It is closely related to dimensional analysis...
is applied to various qualities. One of the qualities of nondimensionalization is that when geometrically similar turbines will produce the same non-dimensional results, while because of other factors (difference in scale, wind properties) produce very different dimensional properties. This allows one to make comparisons between different turbines, while eliminating the effect of things like size and wind conditions from the comparison.
The coefficient of power is the most important variable in wind turbine aerodynamics. Buckingham π theorem can be applied to show that non-dimensional variable for power is given by the equation below. This equation is similar to efficiency, so values between 0 and less than one are typical. However this is not the exactly the same as efficiency so in practice some turbines can exhibit greater than unity power coefficients. In these circumstances one cannot conclude the first law of thermodynamics is violated because this is not an efficiency term by the strict definition of efficiency.
- where:
is the coefficient of power, 
is the air density, A is the area of the wind turbine, finally V is the wind speed.
Equation shows two important dependents. The first is the speed (U) that the machine is going at. The speed at the tip of the blade is usually used for this purpose, and is written as the product of the blade radius and the rotational speed of the wind (U=omega*r).[please clarify] This variable is nondimensionalized by the wind speed, to get the speed ratio:
The force vector is not straightforward, as stated earlier there are two types of aerodynamic forces, lift and drag. Accordingly there are two non-dimensional parameters. However both variables are non-dimensionalized in a similar way. The formula for lift is given below, the formula for drag is given after:
- where:
is the lift coefficient, 
is the drag coefficient, 
is the relative wind as experienced by the wind turbine blade, A is the area but may not be the same area used in the power non-dimensionalization of power.
The aerodynamic forces have a dependency on W, this speed is the relative speed and it is given by the equation below. Note that this is vector subtraction.
Maximum power of a drag based wind turbine
Equation will be the starting point in this derivation. Equation is used to define the force, and equation is used for the relative speed. These substitutions give the following formula for power.The formulas and are applied to express in nondimensional form:
It can be shown through calculus that equation achieves a maximum at
. By inspection one can see that equation will achieve larger values for . In these circumstances, the scalar product in equation makes the result negative. Thus, one can conclude that the maximum power is given by:
Experimentally it has been determined that a large
is 1.2, thus the maximum is approximately 0.1778.Maximum power of a lift based wind turbine
The derivation for a the maximum power of a lift based machine is similar, with some modifications. First we must recognize that drag is always present, thus cannot be ignored. It will be shown that neglecting drag leads to a final solution of infinite power. This result is clearly invalid, hence we will proceed with drag. As before, equations , and will be used along with to define the power below expression.Similarly, this is non-dimensionalized with equations and . However in this derivation the parameter
is also used:Solving the optimal speed ratio is complicated by the dependency on
and the fact that the optimal speed ratio is a solution to a cubic polynomial. Numerical methods can then be applied to determine this solution and the corresponding solution for a range of results. Some sample solutions are given in the table below.  | Optimal  | Optimal  |
|---|---|---|
| 0.5 | 1.23 | 0.75  |
| 0.2 | 3.29 | 3.87  |
| 0.1 | 6.64 | 14.98  |
| 0.05 | 13.32 | 59.43  |
| 0.04 | 16.66 | 92.76  |
| 0.03 | 22.2 | 164.78  |
| 0.02 | 33.3 | 370.54  |
| 0.01 | 66.7 | 1481.65  |
| 0.007 | 95.23 | 3023.6  |
Experiments have shown that it is not unreasonable to achieve a drag ratio (
) of approximately 0.01 at a lift coefficient of 0.6. This would give a of about 889. This is substantially better than the best drag based machine, hence why lift based machines are superior.In the analysis given here, there is an inconsistency compared to typical wind turbine non-dimensionalization. As stated in the preceding section the A in the
non-dimensionalization is not always the same as the A in the force equations and . Typically for the A is the area swept by the rotor blade in its motion. For and A is the area of the turbine wing section. For drag based machines, these two areas are almost identical so there is little difference. To make the lift based results comparable to the drag results, the area of the wing section was used to non-dimensionalize power. The results here could be interpreted as power per unit of material. Given that the material represents the cost (wind is free), this is a better variable for comparison.If one were to apply conventional non-dimensionalization, more information on the motion of the blade would be required. However the discussion on Horizontal Axis Wind Turbines will show that the maximum
there is 16/27. Thus, even by conventional non-dimensional analysis lift based machines are superior to drag based machines.There are several idealizations to the analysis. In any lift based machine (aircraft included) with finite wings, there is a wake that affects the incoming flow and creates induced drag. This phenomenon exists in wind turbines and was neglected in this analysis. Including induced drag requires information specific to the topology, In these cases it is expected that both the optimal speed ratio and the optimal
would be less. The analysis focused on the aerodynamic potential, but neglected structural aspects. In reality most optimal wind turbine design becomes a compromise between optimal aerodynamic design, and optimal structural design.Horizontal axis wind turbine
The aerodynamics of a horizontal-axis wind turbine (HAWT) are not straightforward. The air flow at the blades is not the same as the airflow further away from the turbine. The very nature of the way in which energy is extracted from the air also causes air to be deflected by the turbine. In addition the aerodynamicsAerodynamics
Aerodynamics is a branch of dynamics concerned with studying the motion of air, particularly when it interacts with a moving object. Aerodynamics is a subfield of fluid dynamics and gas dynamics, with much theory shared between them. Aerodynamics is often used synonymously with gas dynamics, with...
of a wind turbine
Wind turbine
A wind turbine is a device that converts kinetic energy from the wind into mechanical energy. If the mechanical energy is used to produce electricity, the device may be called a wind generator or wind charger. If the mechanical energy is used to drive machinery, such as for grinding grain or...
at the rotor surface exhibit phenomena that are rarely seen in other aerodynamic fields.
Axial momentum and the Lanchester-Betz-Joukowsky limit
Vapor pressure
Vapor pressure or equilibrium vapor pressure is the pressure of a vapor in thermodynamic equilibrium with its condensed phases in a closed system. All liquids have a tendency to evaporate, and some solids can sublimate into a gaseous form...
, kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
from the velocity and finally thermal energy
Thermal energy
Thermal energy is the part of the total internal energy of a thermodynamic system or sample of matter that results in the system's temperature....
. Gravitational and thermal energy have a negligible effect on the energy extraction process. From a macroscopic point of view, the air flow about the wind turbine is at atmospheric pressure. If pressure is constant then only kinetic energy is extracted. However up close near the rotor itself the air velocity is constant as it passes through the rotor plane. This is because of conservation of mass
Conservation of mass
The law of conservation of mass, also known as the principle of mass/matter conservation, states that the mass of an isolated system will remain constant over time...
. The air that passes through the rotor cannot slow down because it needs to stay out of the way of the air behind it. So at the rotor the energy is extracted by a pressure drop. The air directly behind the wind turbine is at sub-atmospheric pressure
Atmospheric pressure
Atmospheric pressure is the force per unit area exerted into a surface by the weight of air above that surface in the atmosphere of Earth . In most circumstances atmospheric pressure is closely approximated by the hydrostatic pressure caused by the weight of air above the measurement point...
; the air in front is under greater than atmospheric pressure. It is this high pressure in front of the wind turbine that deflects some of the upstream air around the turbine.
Frederick W. Lanchester was the first to study this phenomenon in application to ship propellers, five years later Nikolai Yegorovich Zhukovsky and Albert Betz
Albert Betz
Albert Betz was a German physicist and a pioneer of wind turbine technology.In 1910 he graduated as a naval engineer from Technische Hochschule Berlin...
independently arrived at the same results . It is believed that each researcher was not aware of the others work because of World War One and the Bolshevik Revolution. Thus formally, the proceeding limit should be referred to as the Lanchester-Betz-Joukowsky limit. In general Albert Betz
Albert Betz
Albert Betz was a German physicist and a pioneer of wind turbine technology.In 1910 he graduated as a naval engineer from Technische Hochschule Berlin...
is credited for this accomplishment because he published his work in a journal that had a wider circulation, while the other two published it in the publication associated with their respective institution, thus it is widely known as simply the Betz Limit.
This is derived by looking at the axial momentum of the air passing through the wind turbine. As stated above some of the air is deflected away from the turbine. This causes the air passing through the rotor plane to have a smaller velocity than the free stream velocity. The ratio of this reduction to that of the air velocity far away from the wind turbine is called the axial induction factor. It is defined as below:
- where: a is the axial induction factor, U1 is the wind speed far away upstream from the rotor, and U2 is the wind speed at the rotor.
The first step to deriving the Betz limit is applying conservation of axial momentum
Angular momentum
In physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
. As stated above the wind loses speed after the wind turbine compared to the speed far away from the turbine. This would violate the conservation of momentum if the wind turbine was not applying a thrust force on the flow. This thrust force manifests itself through the pressure drop across the rotor. The front operates at high pressure while the back operates at low pressure. The pressure difference from the front to back causes the thrust force. The momentum lost in the turbine is balanced by the thrust force.
Another equation is needed to relate the pressure difference to the velocity of the flow near the turbine. Here the Bernoulli equation
Bernoulli's principle
In fluid dynamics, Bernoulli's principle states that for an inviscid flow, an increase in the speed of the fluid occurs simultaneously with a decrease in pressure or a decrease in the fluid's potential energy...
is used between the field flow and the flow near the wind turbine. There is one limitation to the Bernoulli equation: the equation cannot be applied to fluid passing through the wind turbine. Instead conservation of mass is used to relate the incoming air to the outlet air. Betz used these equations and managed to solve the velocities of the flow in the far wake and near the wind turbine in terms of the far field flow and the axial induction factor. The velocities are given below as:
U4 is introduced here as the wind velocity in the far wake. This is important because the power extracted from the turbine is defined by the following equation. However the Betz limit is given in terms of the coefficient of power
. The coefficient of power is similar to efficiency but not the same. The formula for the coefficient of power is given beneath the formula for power:
Betz was able to develop an expression for
in terms of the induction factors. This is done by the velocity relations being substituted into power and power is substituted into the coefficient of power definition. The relationship Betz developed is given below:
The Betz limit is defined by the maximum value that can be given by the above formula. This is found by taking the derivative with respect to the axial induction factor, setting it to zero and solving for the axial induction factor. Betz was able to show that the optimum axial induction factor is one third. The optimum axial induction factor was then used to find the maximum coefficient of power. This maximum coefficient is the Betz limit. Betz was able to show that the maximum coefficient of power of a wind turbine is 16/27. Airflow operating at higher thrust will cause the axial induction factor to rise above the optimum value. Higher thrust cause more air to be deflected away from the turbine. When the axial induction factor falls below the optimum value the wind turbine is not extracting all the energy it can. This reduces pressure around the turbine and allows more air to pass through the turbine, but not enough to account for lack of energy being extracted.
The derivation of the Betz limit shows a simple analysis of wind turbine aerodynamics. In reality there is a lot more. A more rigorous analysis would include wake rotation, the effect of variable geometry. The effect of airfoils on the flow is a major component of wind turbine aerodynamics. Within airfoils alone, the wind turbine aerodynamicist has to consider the effect of surface roughness, dynamic stall tip losses, solidity, among other problems.
Angular momentum and wake rotation
The wind turbine described by Betz does not actually exist. It is merely an idealized wind turbine described as an actuator disk. It's a disk in space where fluid energy is simply extracted from the air. In the Betz turbine the energy extraction manifests itself through thrust. The equivalent turbine described by Betz would be a horizontal propeller type operating with infinite blades at infinite tip speed ratioTip speed ratio
The tip speed ratio λ or TSR for wind turbines is the ratio between the rotational speed of the tip of a blade and the actual velocity of the wind. If the velocity of the tip is exactly the same as the wind speed the tip speed ratio is 1. The tip speed ratio is related to efficiency, with the...
s and no losses. The tip speed ratio is ratio of the speed of the tip relative to the free stream flow. This turbine is not too far from actual wind turbines. Actual turbines are rotating blades. They typically operate at high tip speed ratios. At high tip speed ratios three blades are sufficient to interact with all the air passing through the rotor plane. Actual turbines still produce considerable thrust forces.
One key difference between actual turbines and the actuator disk, is that the energy is extracted through torque. The wind imparts a torque on the wind turbine, thrust is a necessary by-product of torque. Newtonian physics dictates that for every action there is an equal and opposite reaction. If the wind imparts a torque on the blades then the blades must be imparting a torque on the wind. This torque would then cause the flow to rotate. Thus the flow in the wake has two components, axial and tangential. This tangential flow is referred to as wake rotation.
Torque is necessary for energy extraction. However wake rotation is considered a loss. Accelerating the flow in the tangential direction increases the absolute velocity. This in turn increases the amount of kinetic energy in the near wake. This rotational energy is not dissipated in any form that would allow for a greater pressure drop (Energy extraction). Thus any rotational energy in the wake is energy that is lost and unavailable.
This loss is minimized by allowing the rotor to rotate very quickly. To the observer it may seem like the rotor is not moving fast; however, it is common for the tips to be moving through the air at 6 times the speed of the free stream. Newtonian mechanics defines power as torque multiplied by the rotational speed. The same amount of power can be extracted by allowing the rotor to rotate faster and produce less torque. Less torque means that there is less wake rotation. Less wake rotation means there is more energy available to extract.
Blade element and momentum theory
The simplest model for horizontal axis wind turbineHAWT
HAWT may refer to:* Health and welfare trust* Horizontal-axis wind turbine* Slang for Physical attractiveness or Sexual arousal...
(HAWT) aerodynamics is Blade Element
Blade element theory
Blade element theory is a mathematical process originally designed by William Froude , David W. Taylor and Stefan Drzewiecki to determine the behavior of propellers. It involves breaking a blade down into several small parts then determining the forces on each of these small blade elements...
Momentum (BEM) theory
Momentum theory
In fluid dynamics, the momentum theory or disk actuator theory is a theory describing a mathematical model of an ideal actuator disk, such as a propeller or helicopter rotor, by W.J.M. Rankine , Alfred George Greenhill and R.E. Froude ....
. The theory is based on the assumption that the flow at a given annulus does not affect the flow at adjacent annuli. This allows the rotor blade to be analyzed in sections, where the resulting forces are summed over all sections to get the overall forces of the rotor. The theory uses both axial and angular momentum balances to determine the flow and the resulting forces at the blade.
The momentum equations for the far field flow dictate that the thrust and torque will induce a secondary flow in the approaching wind. This in turn affects the flow geometry at the blade. The blade itself is the source of these thrust and torque forces. The force response of the blades is governed by the geometry of the flow, or better known as the angle of attack. Refer to the Airfoil
Airfoil
An airfoil or aerofoil is the shape of a wing or blade or sail as seen in cross-section....
article for more information on how airfoils create lift and drag forces at various angles of attack. This interplay between the far field momentum balances and the local blade forces requires one to solve the momentum equations and the airfoil equations simultaneously. Typically computers and numerical methods are employed to solve these models.
There is a lot of variation between different version of BEM theory. First, one can consider the effect of wake rotation or not. Second, one can go further and consider the pressure drop induced in wake rotation. Third, the tangential induction factors can be solved with a momentum equation, an energy balance or orthognal geometric constraint; the latter a result of Biot-Savart law
Biot-Savart law
The Biot–Savart law is an equation in electromagnetism that describes the magnetic field B generated by an electric current. The vector field B depends on the magnitude, direction, length, and proximity of the electric current, and also on a fundamental constant called the magnetic constant...
in vortex methods. These all lead to different set of equations that need to be solved. The simplest and most widely used equations are those that consider wake rotation with the momentum equation but ignore the pressure drop from wake rotation. Those equations are given below. a is the axial component of the induced flow, a' is the tangential component of the induced flow.
is the solidity of the rotor, is the local inflow angle. and are the coefficient of normal force and the coefficient of tangential force respectively. Both these coefficients are defined with the resulting lift and drag coefficients of the airfoil:
Corrections to blade element momentum theory
Blade Element Momentum (BEM) theory alone fails to accurately represent the true physics of real wind turbines. Two major shortcomings are the effect of discrete number of blades and far field effects when the turbine is heavily loaded. Secondary short-comings come from dealing with transient effects like dynamic stall, rotational effects like coriolis and centrifugal pumping, finally geometric effects that arise from coned and yawed rotors. The current state of the art in BEM uses corrections to deal with the major shortcoming. These corrections are discussed below. There is as yet no accepted treatment for the secondary shortcomings. These areas remain a highly active area of research in wind turbine aerodynamics.The effect of the discrete number of blades is dealt with by applying the Prandtl tip loss factor. The most common form of this factor is given below where B is the number of blades, R is the outer radius and r is the local radius. The definition of F is based on actuator disk models and not directly applicable to BEM. However the most common application multiplies induced velocity term by F in the momentum equations. As in the momentum equation there are many variations for applying F, some argue that the mass flow should be corrected in either the axial equation, or both axial and tangential equations. Others have suggested a second tip loss term to account for the reduced blade forces at the tip. Shown below are the above momentum equations with the most common application of 'F':
The typical momentum theory applied in BEM is only effective for axial induction factors up to 0.4 (thrust coefficient of 0.96). Beyond this point the wake collapses and turbulent mixing occurs. This state is highly transient and largely unpredictable by theoretical means. Accordingly, several empirical relations have been developed. As the usual case there are several version, however a simple one that is commonly used is a linear curve fit given below, with
. The turbulent wake function given excludes the tip loss function, however the tip loss is applied simply by multiplying the resulting axial induction by the tip loss function.
when
The terms
and represent different quantities. The first one is the thrust coefficient of the rotor, which is the one which should be corrected for high rotor loading (i.e. for high values of ), while the second one () is the tangential aerodynamic coefficient of an individual blade element, which is given by the aerodynamic lift and drag coefficients.Aerodynamic modelling
BEM is widely used due to its simplicity and overall accuracy, but its originating assumptions limit its use when the rotor disk is yawed, or when other non-axisymmetric effects (like the rotor wake) influence the flow. Limited success at improving predictive accuracy has been made using computational fluid dynamics (CFD)Computational fluid dynamics
Computational fluid dynamics, usually abbreviated as CFD, is a branch of fluid mechanics that uses numerical methods and algorithms to solve and analyze problems that involve fluid flows. Computers are used to perform the calculations required to simulate the interaction of liquids and gases with...
solvers based on Reynolds Averaged Navier Stokes
Reynolds-averaged Navier-Stokes equations
The Reynolds-averaged Navier–Stokes equations are time-averagedequations of motion for fluid flow. The idea behind the equations is Reynolds decomposition, whereby an instantaneous quantity is decomposed into its time-averaged and fluctuating quantities, an idea first proposed by Osborne Reynolds...
(RANS) and other similar three-dimensional models such as free vortex methods. These are very computationally intensive simulations to perform for several reasons. First, the solver must accurately model the far-field flow conditions, which can extend several rotor diameters up- and down-stream and include atmospheric boundary layer turbulence, while at the same time resolving the small-scale boundary-layer
Boundary layer
In physics and fluid mechanics, a boundary layer is that layer of fluid in the immediate vicinity of a bounding surface where effects of viscosity of the fluid are considered in detail. In the Earth's atmosphere, the planetary boundary layer is the air layer near the ground affected by diurnal...
flow conditions at the blades' surface (necessary to capture blade stall). In addition, many CFD solvers have difficulty meshing parts that move and deform, such as the rotor blades. Finally, there are many dynamic flow phenomena that are not easily modelled by RANS, such as dynamic stall and tower shadow. Due to the computational complexity, it is not currently practical to use these advanced methods for wind turbine design, though research continues in these and other areas related to helicopter and wind turbine aerodynamics.
Free vortex models (FVM) and Lagrangian particle vortex methods (LPVM) are both active areas of research that seek to increase modelling accuracy by accounting for more of the three-dimensional and unsteady flow effects than either BEM or RANS. FVM is similar to lifting line theory in that it assumes that the wind turbine rotor is shedding either a continuous vortex filament from the blade tips (and often the root), or a continuous vortex sheet from the blades' trailing edges. LPVM can use a variety of methods to introduce vorticity into the wake. Biot-Savart summation is used to determine the induced flow field of these wake vorticies' circulations, allowing for better approximations of the local flow over the rotor blades. These methods have largely confirmed much of the applicability of BEM and shed insight into the structure of wind turbine wakes. FVM has limitations due to its origin in potential flow theory, such as not explicitly modelling model viscous behavior, though LPVM is a fully viscous method. LPVM is more computationally intensive than either FVM or RANS, and FVM still relies on blade element theory for the blade forces.