
280 (number)
Encyclopedia
280 is the natural number
after 279 and before 281.
There are 280 plane tree
s with ten nodes.
As a consequence of this, 18 people around a round table can shake hands with each other in non-crossing ways, in 280 different ways (this includes rotations).
280 is a base 10 Harshad number
.
with 283, Sophie Germain prime
, sum of the first fourteen primes, sum of seven consecutive primes (29 + 31 + 37 + 41 + 43 + 47 + 53), Chen prime
, Eisenstein prime
with no imaginary part, centered decagonal number
amicable number
with 220
; nontotient
. 284 degrees Celsius is the flash point
of paper
.
, square pyramidal number
, Harshad number, also in Star Trek
, the total number of Rules of Acquisition
, repdigit
in base 7 (555).
, tetrahedral number
, nontotient
, also shorthand for the Intel 80286
microprocessor chip
, also shorthand for the Intel math coprocessor to the 80286
, 4 superfactorial, untouchable number
, Harshad number, self number
, and is two-dozen sets of a dozen, thus being two gross
, often told as a pun in maths classes. 288=32=11+22+33+44
, Friedman number
since (8 + 9)^2 = 289
Natural number
In mathematics, the natural numbers are the ordinary whole numbers used for counting and ordering . These purposes are related to the linguistic notions of cardinal and ordinal numbers, respectively...
after 279 and before 281.
| Ordinal Ordinal number In set theory, an ordinal number, or just ordinal, is the order type of a well-ordered set. They are usually identified with hereditarily transitive sets. Ordinals are an extension of the natural numbers different from integers and from cardinals... |
two hundred [and] eightieth |
| Cardinal Cardinal number In mathematics, cardinal numbers, or cardinals for short, are a generalization of the natural numbers used to measure the cardinality of sets. The cardinality of a finite set is a natural number – the number of elements in the set. The transfinite cardinal numbers describe the sizes of infinite... |
two hundred [and] eighty |
| Factorization Factorization In mathematics, factorization or factoring is the decomposition of an object into a product of other objects, or factors, which when multiplied together give the original... | 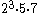 |
| Roman numeral | CCLXXX |
| Binary Binary numeral system The binary numeral system, or base-2 number system, represents numeric values using two symbols, 0 and 1. More specifically, the usual base-2 system is a positional notation with a radix of 2... |
100011000 |
| Hexadecimal Hexadecimal In mathematics and computer science, hexadecimal is a positional numeral system with a radix, or base, of 16. It uses sixteen distinct symbols, most often the symbols 0–9 to represent values zero to nine, and A, B, C, D, E, F to represent values ten to fifteen... |
118 |
In mathematics
The denominator of the eighth harmonic number, 280 is an octagonal number.There are 280 plane tree
Tree (graph theory)
In mathematics, more specifically graph theory, a tree is an undirected graph in which any two vertices are connected by exactly one simple path. In other words, any connected graph without cycles is a tree...
s with ten nodes.
As a consequence of this, 18 people around a round table can shake hands with each other in non-crossing ways, in 280 different ways (this includes rotations).
280 is a base 10 Harshad number
Harshad number
A Harshad number, or Niven number in a given number base, is an integer that is divisible by the sum of its digits when written in that base. Harshad numbers were defined by D. R. Kaprekar, a mathematician from India. The word "Harshad" comes from the Sanskrit + , meaning joy-giver. The Niven...
.
281
Two hundred [and] eighty-one 281 prime, twin primeTwin prime
A twin prime is a prime number that differs from another prime number by two. Except for the pair , this is the smallest possible difference between two primes. Some examples of twin prime pairs are , , , , and...
with 283, Sophie Germain prime
Sophie Germain prime
In number theory, a prime number p is a Sophie Germain prime if 2p + 1 is also prime. For example, 23 is a Sophie Germain prime because it is a prime and 2 × 23 + 1 = 47, and 47 is also a prime number...
, sum of the first fourteen primes, sum of seven consecutive primes (29 + 31 + 37 + 41 + 43 + 47 + 53), Chen prime
Chen prime
A prime number p is called a Chen prime if p + 2 is either a prime or a product of two primes. The even number 2p + 2 therefore satisfies Chen's theorem....
, Eisenstein prime
Eisenstein prime
In mathematics, an Eisenstein prime is an Eisenstein integerz = a + b\,\omega\qquadthat is irreducible in the ring-theoretic sense: its only Eisenstein divisors are the units , a + bω itself and its associates.The associates and the complex conjugate...
with no imaginary part, centered decagonal number
Centered decagonal number
A centered decagonal number is a centered figurate number that represents a decagon with a dot in the center and all other dots surrounding the center dot in successive decagonal layers...
283
Two hundred [and] eighty-three 283 prime, twin prime with 281, strictly non-palindromic numberStrictly non-palindromic number
A strictly non-palindromic number is an integer n that is not palindromic in any numeral system with a base b in the range 2 ≤ b ≤ n − 2...
284
Two hundred [and] eighty-four 284284 (number)
Two hundred eighty-four is the natural number following 283 and preceding 285.Its divisors are 1, 2, 4, 71, and 142, adding up to 220, in turn, the divisors of 220 add up to 284, making the two a pair of amicable numbers....
amicable number
Amicable number
Amicable numbers are two different numbers so related that the sum of the proper divisors of each is equal to the other number. A pair of amicable numbers constitutes an aliquot sequence of period 2...
with 220
220 (number)
220 is the natural number following 219 and preceding 221.-In mathematics:It is a composite number, with its divisors being 1, 2, 4, 5, 10, 11, 20, 22, 44, 55 and 110, making it an amicable number with 284...
; nontotient
Nontotient
In number theory, a nontotient is a positive integer n which is not in the range of Euler's totient function φ, that is, for which φ = n has no solution. In other words, n is a nontotient if there is no integer x that has exactly n coprimes below it. All odd numbers are nontotients, except 1,...
. 284 degrees Celsius is the flash point
Flash point
The flash point of a volatile material is the lowest temperature at which it can vaporize to form an ignitable mixture in air. Measuring a flash point requires an ignition source...
of paper
Paper
Paper is a thin material mainly used for writing upon, printing upon, drawing or for packaging. It is produced by pressing together moist fibers, typically cellulose pulp derived from wood, rags or grasses, and drying them into flexible sheets....
.
285
Two hundred [and] eighty-five 285 = 3·5·19, sphenic numberSphenic number
In number theory, a sphenic number is a positive integer which is the product of three distinct prime numbers.Note that this definition is more stringent than simply requiring the integer to have exactly three prime factors; e.g. 60 = 22 × 3 × 5 has exactly 3 prime factors, but is not sphenic.All...
, square pyramidal number
Square pyramidal number
In mathematics, a pyramid number, or square pyramidal number, is a figurate number that represents the number of stacked spheres in a pyramid with a square base...
, Harshad number, also in Star Trek
Star Trek
Star Trek is an American science fiction entertainment franchise created by Gene Roddenberry. The core of Star Trek is its six television series: The Original Series, The Animated Series, The Next Generation, Deep Space Nine, Voyager, and Enterprise...
, the total number of Rules of Acquisition
Rules of Acquisition
The Rules of Acquisition, in the fictional Star Trek universe, are a set of guidelines intended to ensure the profitability of businesses owned by the ultra-capitalist alien race known as Ferengi.-Background:...
, repdigit
Repdigit
In recreational mathematics, a repdigit is a natural number composed of repeated instances of the same digit, most often in the decimal numeral system....
in base 7 (555).
286
Two hundred [and] eighty-six 286 = 2·11·13, sphenic numberSphenic number
In number theory, a sphenic number is a positive integer which is the product of three distinct prime numbers.Note that this definition is more stringent than simply requiring the integer to have exactly three prime factors; e.g. 60 = 22 × 3 × 5 has exactly 3 prime factors, but is not sphenic.All...
, tetrahedral number
Tetrahedral number
A tetrahedral number, or triangular pyramidal number, is a figurate number that represents a pyramid with a triangular base and three sides, called a tetrahedron...
, nontotient
Nontotient
In number theory, a nontotient is a positive integer n which is not in the range of Euler's totient function φ, that is, for which φ = n has no solution. In other words, n is a nontotient if there is no integer x that has exactly n coprimes below it. All odd numbers are nontotients, except 1,...
, also shorthand for the Intel 80286
Intel 80286
The Intel 80286 , introduced on 1 February 1982, was a 16-bit x86 microprocessor with 134,000 transistors. Like its contemporary simpler cousin, the 80186, it could correctly execute most software written for the earlier Intel 8086 and 8088...
microprocessor chip
287
Two hundred [and] eighty-seven 287 = 7·41, sum of three consecutive primes (89 + 97 + 101), sum of five consecutive primes (47 + 53 + 59 + 61 + 67), sum of nine consecutive primes (17 + 19 + 23 + 29 + 31 + 37 + 41 + 43 + 47), Kynea number, pentagonal numberPentagonal number
A pentagonal number is a figurate number that extends the concept of triangular and square numbers to the pentagon, but, unlike the first two, the patterns involved in the construction of pentagonal numbers are not rotationally symmetrical...
, also shorthand for the Intel math coprocessor to the 80286
288
Two hundred [and] eighty-eight 288 = 25·32, pentagonal pyramidal numberPentagonal pyramidal number
A pentagonal pyramidal number is a figurate number that represents the number of objects in a pyramid with a pentagonal base. The nth pentagonal pyramidal number is equal to the sum of the first n pentagonal numbers....
, 4 superfactorial, untouchable number
Untouchable number
An untouchable number is a positive integer that cannot be expressed as the sum of all the proper divisors of any positive integer ....
, Harshad number, self number
Self number
A self number, Colombian number or Devlali number is an integer which, in a given base, cannot be generated by any other integer added to the sum of that other integer's digits. For example, 21 is not a self number, since it can be generated by the sum of 15 and the digits comprising 15, that is,...
, and is two-dozen sets of a dozen, thus being two gross
Gross (unit)
A gross is equal to a dozen dozen, i.e. 12 × 12 = 144.It can be used in duodecimal counting. The use of gross likely originated from the fact that 144 can be counted on the fingers using the fingertips and first two joints of each finger when marked by the thumb of one hand. The other hand...
, often told as a pun in maths classes. 288=32=11+22+33+44
289
Two hundred [and] eighty-nine 289 = 172, centered octagonal numberCentered octagonal number
A centered octagonal number is a centered figurate number that represents an octagon with a dot in the center and all other dots surrounding the center dot in successive octagonal layers...
, Friedman number
Friedman number
A Friedman number is an integer which, in a given base, is the result of an expression using all its own digits in combination with any of the four basic arithmetic operators and sometimes exponentiation. For example, 347 is a Friedman number since 347 = 73 + 4...
since (8 + 9)^2 = 289

