In mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, an Eisenstein prime is an Eisenstein integer

Eisenstein integer

In mathematics, Eisenstein integers , also known as Eulerian integers , are complex numbers of the formz = a + b\omega \,\!where a and b are integers and...

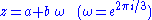
that is irreducible

Irreducible element

In abstract algebra, a non-zero non-unit element in an integral domain is said to be irreducible if it is not a product of two non-units.Irreducible elements should not be confused with prime elements...

 (or equivalently prime

Prime element

In abstract algebra, an element p of a commutative ring R is said to be prime if it is not zero, not a unit and whenever p divides ab for some a and b in R, then p divides a or p divides b...

) in the ring-theoretic sense: its only Eisenstein divisor

Divisor

In mathematics, a divisor of an integer n, also called a factor of n, is an integer which divides n without leaving a remainder.-Explanation:...

s are the unit

Unit (ring theory)

In mathematics, an invertible element or a unit in a ring R refers to any element u that has an inverse element in the multiplicative monoid of R, i.e. such element v that...

s (±1, ±ω, ±ω²), a + bω itself and its associates.

The associates (unit multiples) and the complex conjugate

Complex conjugate

In mathematics, complex conjugates are a pair of complex numbers, both having the same real part, but with imaginary parts of equal magnitude and opposite signs...

 of any Eisenstein prime are also prime.

An Eisenstein integer z = a + bω is an Eisenstein prime if and only if either of the following (mutually exclusive) conditions hold:

1. z is equal to the product of a unit and a natural prime
   Prime number
   A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
   
    of the form 3n − 1,
2. |z|² = a² − ab + b² is a natural prime (necessarily congruent to 0 or 1 modulo 3).

It follows that the absolute value squared of every Eisenstein prime is a natural prime or the square of a natural prime.

The first few Eisenstein primes that equal a natural prime 3n − 1 are:

2, 5, 11

11 (number)

11 is the natural number following 10 and preceding 12.Eleven is the first number which cannot be counted with a human's eight fingers and two thumbs additively. In English, it is the smallest positive integer requiring three syllables and the largest prime number with a single-morpheme name...

, 17

17 (number)

17 is the natural number following 16 and preceding 18. It is prime.In spoken English, the numbers 17 and 70 are sometimes confused because they sound similar. When carefully enunciated, they differ in which syllable is stressed: 17 vs 70...

, 23

23 (number)

23 is the natural number following 22 and preceding 24.- In mathematics :Twenty-three is the ninth prime number, the smallest odd prime that is not a twin prime. Twenty-three is also the fifth factorial prime, the third Woodall prime...

, 29

29 (number)

29 is the natural number following 28 and preceding 30.-In mathematics:It is the tenth prime number, and also the fourth primorial prime. It forms a twin prime pair with thirty-one, which is also a primorial prime. Twenty-nine is also the sixth Sophie Germain prime. It is also the sum of three...

, 41

41 (number)

41 is the natural number following 40 and preceding 42.-In mathematics:Forty-one is the 13th smallest prime number. The next is forty-three, with which it comprises a twin prime...

, 47

47 (number)

47 is the natural number following 46 and preceding 48.-In mathematics:Forty-seven is the fifteenth prime number, a safe prime, the thirteenth supersingular prime, and the sixth Lucas prime. Forty-seven is a highly cototient number...

, 53

53 (number)

53 is the natural number following 52 and preceding 54.-In mathematics:Fifty-three is the 16th prime number. It is also an Eisenstein prime....

, 59

59 (number)

59 is the natural number following 58 and preceding 60.-In mathematics:Fifty-nine is the 17th smallest prime number. The next is sixty-one, with which it comprises a twin prime. 59 is an irregular prime, a safe prime and the 14th supersingular prime. It is an Eisenstein prime with no imaginary...

, 71

71 (number)

71 is the natural number following 70 and preceding 72.-In mathematics:71 is the algebraic degree of Conway's constant, a remarkable number arising in the study of look-and-say sequences....

, 83

83 (number)

83 is the natural number following 82 and preceding 84.-In mathematics:Eighty-three is the sum of three consecutive primes as well as the sum of five consecutive primes ....

, 89, 101

101 (number)

101 is the natural number following 100 and preceding 102.It is variously pronounced "one hundred and one" / "a hundred and one", "one hundred one" / "a hundred one", and "one oh one"...

 

Natural primes that are congruent to 0 or 1 modulo 3 are not Eisenstein primes: they admit nontrivial factorizations in Z[ω]. For example:

3 = −(1 + 2ω)²

7 = (3 + ω)(2 − ω).

Some non-real Eisenstein primes are

2 + ω, 3 + ω, 4 + ω, 5 + 2ω, 6 + ω, 7 + ω, 7 + 3ω

Up to conjugacy and unit multiples, the primes listed above, together with 2 and 5, are all the Eisenstein primes of absolute value

Absolute value

In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...

 not exceeding 7.

, the largest known (real) Eisenstein prime is 19249 × 2^13018586 + 1, which is the tenth largest known prime

Largest known prime

The largest known prime number is the largest integer that is currently known to be a prime number.It was proven by Euclid that there are infinitely many prime numbers; thus, there is always a prime greater than the largest known prime. Many mathematicians and hobbyists search for large prime numbers...

, discovered by Konstantin Agafonov. All larger known primes are Mersenne prime

Mersenne prime

In mathematics, a Mersenne number, named after Marin Mersenne , is a positive integer that is one less than a power of two: M_p=2^p-1.\,...

s, discovered by GIMPS. Real Eisenstein primes are congruent to 2 mod 3, and Mersenne primes (except the smallest, 3) are congruent to 1 mod 3; thus no Mersenne prime is an Eisenstein prime.