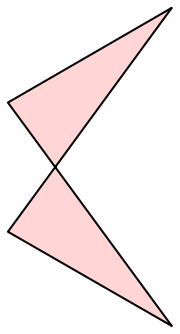
An antiparallelogram is a quadrilateral

Quadrilateral

In Euclidean plane geometry, a quadrilateral is a polygon with four sides and four vertices or corners. Sometimes, the term quadrangle is used, by analogy with triangle, and sometimes tetragon for consistency with pentagon , hexagon and so on...

 in which, like a parallelogram

Parallelogram

In Euclidean geometry, a parallelogram is a convex quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure...

, the pairs of nonadjacent sides

Adjacent

Adjacent is an adjective meaning contiguous, adjoining or abuttingIn geometry, adjacent is when sides meet to make an angle.In graph theory adjacent nodes in a graph are linked by an edge....

 are congruent

Congruence (geometry)

In geometry, two figures are congruent if they have the same shape and size. This means that either object can be repositioned so as to coincide precisely with the other object...

, but in which two opposite sides intersect (unlike in a parallelogram) and are therefore not parallel

Parallel (geometry)

Parallelism is a term in geometry and in everyday life that refers to a property in Euclidean space of two or more lines or planes, or a combination of these. The assumed existence and properties of parallel lines are the basis of Euclid's parallel postulate. Two lines in a plane that do not...

.

Properties

Every antiparallelogram has an axis of symmetry through its crossing point. Because of this symmetry, it has two pairs of equal angles as well as two pairs of equal sides. Together with the kites

Kite (geometry)

In Euclidean geometry a kite is a quadrilateral whose four sides can be grouped into two pairs of equal-length sides that are next to each other. In contrast, a parallelogram also has two pairs of equal-length sides, but they are opposite each other rather than next to each other...

 and the isosceles trapezoid

Isosceles trapezoid

In Euclidean geometry, an isosceles trapezoid is a convex quadrilateral with a line of symmetry bisecting one pair of opposite sides, making it automatically a trapezoid...

s, antiparallelograms form one of three basic classes of quadrilaterals with a symmetry axis. The convex hull

Convex hull

In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X....

 of an antiparallelogram is an isosceles trapezoid, and every antiparallelogram may be formed from the non-parallel sides and diagonals of an isosceles trapezoid.

Every antiparallelogram is a cyclic quadrilateral

Cyclic quadrilateral

In Euclidean geometry, a cyclic quadrilateral is a quadrilateral whose vertices all lie on a single circle. This circle is called the circumcircle or circumscribed circle, and the vertices are said to be concyclic. Other names for these quadrilaterals are chordal quadrilateral and inscribed...

, meaning that its four vertices all lie on a single circle

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

.

Uniform polyhedra and their duals

Several nonconvex uniform polyhedra

Nonconvex uniform polyhedron

In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting...

, including the tetrahemihexahedron

Tetrahemihexahedron

In geometry, the tetrahemihexahedron or hemicuboctahedron is a uniform star polyhedron, indexed as U4. It has 6 vertices and 12 edges, and 7 faces: 4 triangular and 3 square. Its vertex figure is a crossed quadrilateral. It has Coxeter-Dynkin diagram of ....

, cubohemioctahedron

Cubohemioctahedron

In geometry, the cubohemioctahedron is a nonconvex uniform polyhedron, indexed as U15. Its vertex figure is a crossed quadrilateral.A nonconvex polyhedron has intersecting faces which do not represent new edges or faces...

, octahemioctahedron

Octahemioctahedron

In geometry, the octahemioctahedron is a nonconvex uniform polyhedron, indexed as U3. Its vertex figure is a crossed quadrilateral.It is one of nine hemipolyhedra with 4 hexagonal faces passing through the model center.- Related polyhedra :...

, small rhombihexahedron

Small rhombihexahedron

In geometry, the small rhombihexahedron is a nonconvex uniform polyhedron, indexed as U18. It has 18 faces , 48 edges, and 24 vertices. Its vertex figure is an antiparallelogram.-Related polyhedra:...

, small icosihemidodecahedron

Small icosihemidodecahedron

In geometry, the small icosihemidodecahedron is a uniform star polyhedron, indexed as U49. Its vertex figure alternates two regular triangles and decagons as a crossed quadrilateral....

, and small dodecahemidodecahedron

Small dodecahemidodecahedron

In geometry, the small dodecahemidodecahedron is a nonconvex uniform polyhedron, indexed as U51. Its vertex figure alternates two regular pentagons and decagons as a crossed quadrilateral....

, have antiparallelograms as their vertex figure

Vertex figure

In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...

s. For uniform polyhedra of this type in which the faces do not pass through the center point of the polyhedron, the dual polyhedron

Dual polyhedron

In geometry, polyhedra are associated into pairs called duals, where the vertices of one correspond to the faces of the other. The dual of the dual is the original polyhedron. The dual of a polyhedron with equivalent vertices is one with equivalent faces, and of one with equivalent edges is another...

 has antiparallelograms as its faces; examples of dual uniform polyhedra with antiparallelogram faces include the small rhombihexacron

Small rhombihexacron

In geometry, the small rhombihexacron is the dual of the small rhombihexahedron. Its faces are antiparallelograms formed by pairs of coplanar triangles....

, the great rhombihexacron

Great rhombihexacron

In geometry, the great rhombihexacron is a nonconvex isohedral polyhedron. It is the dual of the uniform great rhombihexahedron . It has 24 identical bow-tie-shaped faces, 18 vertices, and 48 edges....

, the small rhombidodecacron

Small rhombidodecacron

In geometry, the small rhombidodecacron is a nonconvex isohedral polyhedron. It is the dual of the small rhombidodecahedron. It has 60 intersecting antiparallelogram faces.- External links :*...

, the great rhombidodecacron

Great rhombidodecacron

In geometry, the great rhombidodecacron is a nonconvex isohedral polyhedron. It is the dual of the great rhombidodecahedron. Its faces are antiparallelograms....

, the small dodecicosacron

Small dodecicosacron

In geometry, the small dodecicosacron is the dual of the small dodecicosahedron . It has 60 intersecting bow-tie-shaped faces.-External links:*...

, and the great dodecicosacron

Great dodecicosacron

In geometry, the great dodecicosacron is the dual of the great dodecicosahedron . It has 60 intersecting bow-tie-shaped faces.- External links :*...

.

Four-bar linkages

The antiparallelogram has been used as a form of four-bar linkage, in which four rigid beams of fixed length (the four sides of the antiparallelogram) may rotate with respect to each other at joints placed at the four vertices of the antiparallelogram. In this context it is also called a butterfly or bow-tie linkage. As a linkage, it has a point of instability in which it can be converted into a parallelogram and vice versa.

For both the parallelogram and antiparallelogram linkages, if one of the long edges of the linkage is fixed as a base, the free joints move on equal circles, but in a parallelogram they move in the same direction with equal velocities while in the antiparallelogram they move in opposite directions with unequal velocities. As James Watt

James Watt

James Watt, FRS, FRSE was a Scottish inventor and mechanical engineer whose improvements to the Newcomen steam engine were fundamental to the changes brought by the Industrial Revolution in both his native Great Britain and the rest of the world.While working as an instrument maker at the...

 discovered, if an antiparallelogram has its long side fixed in this way it forms a variant of Watt's linkage

Watt's linkage

Watt's linkage is a type of mechanical linkage invented by James Watt in which the central moving point of the linkage is constrained to travel on an approximation to a straight line...

, and the midpoint of the unfixed long edge will trace out a lemniscate

Lemniscate

In algebraic geometry, a lemniscate refers to any of several figure-eight or ∞ shaped curves. It may refer to:*The lemniscate of Bernoulli, often simply called the lemniscate, the locus of points whose product of distances from two foci equals the square of half the interfocal distance*The...

. For the antiparallelogram formed by the sides and diagonals of a square, it is the lemniscate of Bernoulli

Lemniscate of Bernoulli

In geometry, the lemniscate of Bernoulli is a plane curve defined from two given points F1 and F2, known as foci, at distance 2a from each other as the locus of points P so that PF1·PF2 = a2. The curve has a shape similar to the numeral 8 and to the ∞ symbol. Its name is from lemniscus, which is...

. If, instead, one of the short sides of the linkage is fixed, the crossing point moves in an ellipse

Ellipse

In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...

 with the fixed joints as its foci while, again, the other two joints move in circles.

The antiparallelogram is an important feature in the design of Hart's inversor, a linkage that (like the Peaucellier–Lipkin linkage) can convert rotary motion to straight-line motion. An antiparallelogram-shaped linkage can also be used to connect the two axle

Axle

An axle is a central shaft for a rotating wheel or gear. On wheeled vehicles, the axle may be fixed to the wheels, rotating with them, or fixed to its surroundings, with the wheels rotating around the axle. In the former case, bearings or bushings are provided at the mounting points where the axle...

s of a four-wheeled vehicle, decreasing the turning radius

Turning radius

The turning radius or turning circle of a vehicle is the size of the smallest circular turn that the vehicle is capable of making. The term turning radius is actually a misnomer, since the size of a circle is actually its diameter, not its radius. The less ambiguous term turning circle is preferred...

 of the vehicle relative to a suspension that only allows one axle to turn. A pair of nested antiparallelograms was used in a linkage defined by Alfred Kempe

Alfred Kempe

Sir Alfred Bray Kempe D.C.L. F.R.S. was a mathematician best known for his work on linkages and the four color theorem....

 as part of his universality theorem stating that any algebraic curve may be traced out by the joints of a suitably defined linkage. Kempe called the nested-antiparallelogram linkage a "multiplicator", as it could be used to multiply an angle by an integer.

Celestial mechanics

In the n-body problem

N-body problem

The n-body problem is the problem of predicting the motion of a group of celestial objects that interact with each other gravitationally. Solving this problem has been motivated by the need to understand the motion of the Sun, planets and the visible stars...

, the study of the motions of point masses under Newton's law of universal gravitation

Newton's law of universal gravitation

Newton's law of universal gravitation states that every point mass in the universe attracts every other point mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them...

, an important role is played by central configurations, solutions to the n-body problem in which all of the bodies rotate around some central point as if they were rigidly connected to each other. For instance, for three bodies, there are five solutions of this type, given by the five Lagrangian point

Lagrangian point

The Lagrangian points are the five positions in an orbital configuration where a small object affected only by gravity can theoretically be stationary relative to two larger objects...

s. For four bodies, with two pairs of the bodies having equal masses, numerical evidence indicates that there exists a continuous family of central configurations, related to each other by the motion of an antiparallelogram linkage.