Bohm interpretation
Encyclopedia
The de Broglie–Bohm theory, also called the pilot-wave
theory, Bohmian mechanics, and the causal interpretation, is an interpretation of quantum theory
. In addition to a wavefunction
on the space of all possible configurations, it also includes an actual configuration, even in situations where nobody observes it. The evolution over time of the configuration (that is, of the positions of all particles or the configuration of all fields) is defined by the wave function via a guiding equation. The evolution of the wavefunction over time is given by Schrödinger's equation
.
The de Broglie–Bohm theory expresses in an explicit manner the fundamental non-locality of quantum physics. The velocity of any one particle depends on the value of the wavefunction, which depends on the whole configuration of the universe.
This theory is deterministic. Most (but not all) relativistic variants require a preferred frame. Variants which include spin and curved spaces are known. It can be modified to include quantum field theory
. Bell's theorem
was inspired by Bell's discovery of the work of David Bohm
and his subsequent wondering if the obvious non-locality of the theory could be eliminated.
This theory results in a measurement formalism, analogous to thermodynamics for classical mechanics, which yields the standard quantum formalism generally associated with the Copenhagen interpretation
. The measurement problem is resolved by this theory since the outcome of an experiment is registered by the configuration of the particles of the experimental apparatus after the experiment is completed. The familiar wavefunction collapse of standard quantum mechanics emerges from an analysis of subsystems and the quantum equilibrium hypothesis.
The theory has a number of equivalent mathematical formulations and has been presented by a number of different names. The de Broglie wave has a macroscopical analogy termed Faraday wave
.
We have a configuration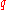
of the universe, described by coordinates 
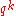, which is an element of the configuration space 
. The configuration space is different for different versions of pilot wave theory. For example, this may be the space of positions 
of 
particles, or, in case of field theory, the space of field configurations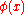 
. The configuration evolves according to the guiding equation
.
Here,
is the standard complex-valued wavefunction known from quantum theory, which evolves according to Schrödinger's equation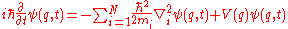
This already completes the specification of the theory for any quantum theory with Hamilton operator of type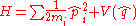
.
If the configuration is distributed according to
at some moment of time 
, this holds for all times. Such a state is named quantum equilibrium. In quantum equilibrium, this theory will agree with the results of standard quantum mechanics.
is an illustration of wave-particle duality. In it, a beam of particles (such as photons) travels through a barrier with two slits removed. If one puts a detector screen on the other side, the pattern of detected particles shows interference fringes characteristic of waves; however, the detector screen responds to particles. The system exhibits behaviour of both waves (interference patterns) and particles (dots on the screen).
If we modify this experiment so that one slit is closed, no interference pattern is observed. Thus, the state of both slits affects the final results. We can also arrange to have a minimally invasive detector at one of the slits to detect which slit the particle went through. When we do that, the interference pattern disappears.
The Copenhagen interpretation
states that the particles are not localised in space until they are detected, so that, if there is not any detector on the slits, there is no matter of fact about which slit the particle has passed through. If one slit has a detector on it, then the wavefunction collapses due to that detection.
In de Broglie–Bohm theory, the wavefunction travels through both slits, but each particle has a well-defined trajectory and passes through exactly one of the slits. The final position of the particle on the detector screen and the slit through which the particle passes by is determined by the initial position of the particle. Such initial position is not controllable by the experimenter, so there is an appearance of randomness in the pattern of detection. The wave function interferes with itself and guides the particles in such a way that the particles avoid the regions in which the interference is destructive and are attracted to the regions in which the interference is constructive, resulting in the interference pattern on the detector screen.
To explain the behavior when the particle is detected to go through one slit, one needs to appreciate the role of the conditional wavefunction and how it results in the collapse of the wavefunction; this is explained below. The basic idea is that the environment registering the detection effectively separates the two wave packets in configuration space.
of de Broglie-Bohm theory consists of a configuration
of the universe and a pilot wave 
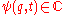. The configuration space 
can be chosen differently, as in classical mechanics and standard quantum mechanics.
Thus, the ontology of pilot wave theory contains as the trajectory
we know from classical mechanics, as the wave function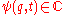 
of quantum theory. So, at every moment of time there exists not only a wave function, but also a well-defined configuration of the whole universe. The correspondence to our experiences is made by the identification of the configuration of our brain with some part of the configuration of the whole universe 
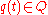, as in classical mechanics.
While the ontology of classical mechanics is part of the ontology of de Broglie–Bohm theory, the dynamics are very different. In classical mechanics, the accelerations of the particles are given by forces. In de Broglie–Bohm theory, the velocities of the particles are given by the wavefunction.
The wavefunction itself, and not the particles, determines the dynamical evolution of the system: the particles do not act back onto the wave function. As Bohm and Hiley worded it, “the Schrodinger equation for the quantum field does not have sources, nor does it have any other way by which the field could be directly affected by the condition of the particles [...] the quantum theory can be understood completely in terms of the assumption that the quantum field has no sources or other forms of dependence on the particles”. P. Holland considers this lack of reciprocal action of particles and wave function to be one “[a]mong the many nonclassical properties exhibited by this theory”. It should be noted however that Holland has later called this a merely apparent lack of back reaction, due to the incompleteness of the description.
In what follows below, we will give the setup for one particle moving in
followed by the setup for 
particles moving in 3 dimensions. In the first instance, configuration space and real space are the same while in the second, real space is still 
, but configuration space becomes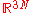 
. While the particle positions themselves are in real space, the velocity field and wavefunction are on configuration space which is how particles are entangled with each other in this theory.
Extensions to this theory include spin and more complicated configuration spaces.
We use variations of
for particle positions while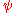 
represents the complex-valued wavefunction on configuration space.
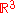, the particle's velocity is given
.
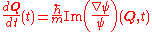For many particles, we label them as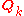
for the 
th particle and their velocities are given by
.
The main fact to notice is that this velocity field depends on the actual positions of all of the
particles in the universe. As explained below, in most experimental situations, the influence of all of those particles can be encapsulated into an effective wavefunction for a subsystem of the universe.
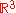. The equation represents a quantized version of the total energy of a classical system evolving under a real-valued potential function 
on 
:
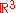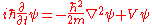
For many particles, the equation is the same except that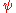
and 
are now on configuration space, 
.
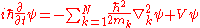
This is the same wavefunction of conventional quantum mechanics.
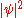. And that distribution is guaranteed to be true for all time by the guiding equation if the initial distribution of the particles satisfies 
.
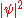For a given experiment, we can postulate this as being true and verify experimentally that it does indeed hold true, as it does. But, as argued in Dürr et al., one needs to argue that this distribution for subsystems is typical. They argue that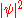
by virtue of its equivariance under the dynamical evolution of the system, is the appropriate measure of typicality for initial conditions of the positions of the particles. They then prove that the vast majority of possible initial configurations will give rise to statistics obeying the Born rule
(i.e.,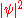
) for measurement outcomes. In summary, in a universe governed by the de Broglie–Bohm dynamics, Born rule behavior is typical.
The situation is thus analogous to the situation in classical statistical physics. A low entropy initial condition will, with overwhelmingly high probability, evolve into a higher entropy state: behavior consistent with the second law of thermodynamics is typical. There are, of course, anomalous initial conditions which would give rise to violations of the second law. However, absent some very detailed evidence supporting the actual realization of one of those special initial conditions, it would be quite unreasonable to expect anything but the actually observed uniform increase of entropy. Similarly, in the de Broglie–Bohm theory, there are anomalous initial conditions which would produce measurement statistics in violation of the Born rule (i.e., in conflict with the predictions of standard quantum theory). But the typicality theorem shows that, absent some particular reason to believe one of those special initial conditions was in fact realized, Born rule behavior is what one should expect.
It is in that qualified sense that Born rule is, for the de Broglie–Bohm theory, a theorem rather than (as in ordinary quantum theory) an additional postulate.
It can also be shown that a distribution of particles that is not distributed according to the Born rule (that is, a distribution 'out of quantum equilibrium') and evolving under the de Broglie-Bohm dynamics is overwhelmingly likely to evolve dynamically into a state distributed as
. See, for example Ref.
. A pretty video of the electron density in a 2D box evolving under this process is available here.
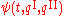, where 
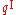denotes the configuration variables associated to some subsystem (I) of the universe and 
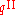denotes the remaining configuration variables. Denote, respectively, by 
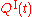and by 
the actual configuration of subsystem (I) and of the rest of the universe. For simplicity, we consider here only the spinless case. The conditional wave function of subsystem (I) is defined by:
It follows immediately from the fact that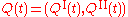
satisfies the guiding equation that also the configuration 
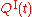satisfies a guiding equation identical to the one presented in the formulation of the theory, with the universal wave function 
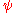replaced with the conditional wave function 
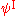. Also, the fact that 
is random with probability density
given by the square modulus of
implies that the conditional probability density of 
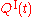given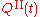 
is given by the square modulus of the (normalized) conditional wave function 
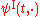(in the terminology of Dürr et al. this fact is called the fundamental conditional probability formula).
Unlike the universal wave function, the conditional wave function of a subsystem does not always evolve by the Schrödinger equation, but in many situations it does. For instance, if the universal wave function factors as:
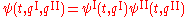
then the conditional wave function of subsystem (I) is (up to an irrelevant scalar factor) equal to
(this is what Standard Quantum Theory would regard as the wave function of subsystem (I)). If, in addition, the Hamiltonian does not contain an interaction term between subsystems (I) and (II) then 
does satisfy a Schrödinger equation. More generally, assume that the universal wave function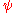 
can be written in the form:
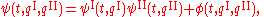
where
solves Schrödinger equation and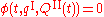 
for all 
and 
. Then, again, the conditional wave function of subsystem (I) is (up to an irrelevant scalar factor) equal to 
and if the Hamiltonian does not contain an interaction term between subsystems (I) and (II), 
satisfies a Schrödinger equation.
The fact that the conditional wave function of a subsystem does not always evolve by the Schrödinger equation is related to the fact that the usual collapse rule of Standard Quantum Theory emerges from the Bohmian formalism when one considers conditional wave functions of subsystems.
. The guiding equation is modified by taking inner products in spin space to reduce the complex vectors to complex numbers. The Schrödinger equation is modified by adding a Pauli spin term.
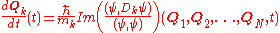
where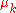
is the magnetic moment of the 
th particle,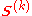 
is the appropriate spin operator acting on the 
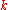th particle's spin space,
, 
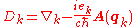and 
are, respectively, the magnetic field and the vector potential in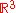 
(all other functions are fully on configuration space), 
is the charge of the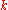 
th particle, and 
is the inner product in spin space 
,
For an example of a spin space, a system consisting of two spin 1/2 particle and one spin 1 particle has a wavefunctions of the form
.
That is, its spin space is a 12 dimensional space.
For a de Broglie–Bohm theory on curved space with spin, the spin space becomes a vector bundle
over configuration space and the potential in Schrödinger's equation becomes a local self-adjoint operator acting on that space.
Hrvoje Nikolić introduces a purely deterministic de Broglie–Bohm theory of particle creation and destruction, according to which particle trajectories are continuous, but particle detectors behave as if particles have been created or destroyed even when a true creation or destruction of particles does not take place.
has extended the de Broglie–Bohm theory to include signal nonlocality that would allow entanglement to be used as a stand-alone communication channel without a secondary classical "key" signal to "unlock" the message encoded in the entanglement. This violates orthodox quantum theory but it has the virtue that it makes the parallel universes of the chaotic inflation theory
observable in principle.
Unlike de Broglie–Bohm theory, Valentini's theory has the wavefunction evolution also depend on the ontological variables. This introduces an instability, a feedback loop that pushes the hidden variables out of "sub-quantal heat death". The resulting theory becomes nonlinear and non-unitary.
. While this is in conflict with the standard interpretation of relativity, the preferred foliation, if unobservable, does not lead to any empirical conflicts with relativity.
The relation between nonlocality and preferred foliation can be better understood as follows. In de Broglie–Bohm theory, nonlocality manifests as the fact that the velocity and acceleration of one particle depends on the instantaneous positions of all other particles. On the other hand, in the theory of relativity the concept of instantaneousness does not have an invariant meaning. Thus, to define particle trajectories, one needs an additional rule that defines which space-time points should be considered instantaneous. The simplest way to achieve this is to introduce a preferred foliation of space-time by hand, such that each hypersurface of the foliation defines a hypersurface of equal time. However, this way (which explicitly breaks the relativistic covariance) is not the only way. It is also possible that a rule which defines instantaneousness is contingent
, by emerging dynamically from relativistic covariant laws combined with particular initial conditions. In this way, the need for a preferred foliation can be avoided and relativistic covariance can be saved.
There has been work in developing relativistic versions of de Broglie–Bohm theory. See Bohm and Hiley: The Undivided Universe, and http://xxx.lanl.gov/abs/quant-ph/0208185, http://xxx.lanl.gov/abs/quant-ph/0302152, and references therein. Another approach is given in the work of Dürr et al. in which they use Bohm-Dirac models and a Lorentz-invariant foliation of space-time.
Initially, it been considered impossible to set out a description of photon trajectories in the de Broglie–Bohm theory in view of the difficulties of describing bosons relativistically. In 1996, Partha Ghose
had presented a relativistic quantum mechanical description of spin-0 and spin-1 bosons starting from the Duffin–Kemmer–Petiau equation, setting out Bohmian trajectories for massive bosons and for massless bosons, thus also for photon
s. In 2001, Jean-Pierre Vigier
emphasized the importance of deriving a well-defined description of light in terms of particle trajectories in the framework of either the Bohmian mechanics or the Nelson stochastic mechanics. The same year, Ghose worked out Bohmian photon trajectories for specific cases. Subsequent weak measurement
experiments yielded trajectories which coincide with the predicted trajectories.
Nikolić has proposed a Lorentz-covariant formulation of the Bohmian interpretation of many-particle wave functions. He has developed a generalized relativistic-invariant probabilistic interpretation of quantum theory, in which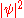
is no longer a probability density in space, but a probability density in space-time. He uses this generalized probabilistic interpretation to formulate a relativistic-covariant version of de Broglie–Bohm theory without introducing a preferred foliation of space-time. His work also covers the extension of the Bohmian interpretation to a quantization of fields and strings.
The basis for agreement with standard quantum mechanics is that the particles are distributed according to
. This is a statement of observer ignorance, but it can be proven that for a universe governed by this theory, this will typically be the case. There is apparent collapse of the wave function governing subsystems of the universe, but there is no collapse of the universal wavefunction.
or polarization of a particle directly; instead, the component in one direction is measured; the outcome from a single particle may be 1, meaning that the particle is aligned with the measuring apparatus, or -1, meaning that it is aligned the opposite way. For an ensemble of particles, if we expect the particles to be aligned, the results are all 1. If we expect them to be aligned oppositely, the results are all -1. For other alignments, we expect some results to be 1 and some to be -1 with a probability that depends on the expected alignment. For a full explanation of this, see the Stern-Gerlach Experiment.
In de Broglie–Bohm theory, the results of a spin experiment cannot be analyzed without some knowledge of the experimental setup. It is possible to modify the setup so that the trajectory of the particle is unaffected, but that the particle with one setup registers as spin up while in the other setup it registers as spin down. Thus, for the de Broglie–Bohm theory, the particle's spin is not an intrinsic property of the particle—instead spin is, so to speak, in the wave function of the particle in relation to the particular device being used to measure the spin. This is an illustration of what is sometimes referred to as contextuality, and is related to naive realism about operators.
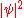distribution for the particles in the system (see the section on the conditional wave function of a subsystem for details).
It requires a special setup for the conditional wavefunction of a system to obey a quantum evolution. When a system interacts with its environment, such as through a measurement, the conditional wavefunction of the system evolves in a different way. The evolution of the universal wavefunction can become such that the wavefunction of the system appears to be in a superposition of distinct states. But if the environment has recorded the results of the experiment, then using the actual Bohmian configuration of the environment to condition on, the conditional wavefunction collapses to just one alternative, the one corresponding with the measurement results.
Collapse
of the universal wavefunction never occurs in de Broglie–Bohm theory. Its entire evolution is governed by Schrödinger's equation and the particles' evolutions are governed by the guiding equation. Collapse only occurs in a phenomenological way for systems that seem to follow their own Schrödinger's equation. As this is an effective description of the system, it is a matter of choice as to what to define the experimental system to include and this will affect when "collapse" occurs.
In the history of de Broglie–Bohm theory, the proponents have often had to deal with claims that this theory is impossible. Such arguments are generally based on inappropriate analysis of operators as observables. If one believes that spin measurements are indeed measuring the spin of a particle that existed prior to the measurement, then one does reach contradictions. De Broglie–Bohm theory deals with this by noting that spin is not a feature of the particle, but rather that of the wavefunction. As such, it only has a definite outcome once the experimental apparatus is chosen. Once that is taken into account, the impossibility theorems become irrelevant.
There have also been claims that experiments reject the Bohm trajectories
http://arxiv.org/abs/quant-ph/0206196 in favor of the standard QM lines. But as shown in http://arxiv.org/abs/quant-ph/0108038 and http://arxiv.org/abs/quant-ph/0305131, such experiments cited above only disprove a misinterpretation of the de Broglie–Bohm theory, not the theory itself.
There are also objections to this theory based on what it says about particular situations usually involving eigenstates of an operator. For example, the ground state of hydrogen is a real wavefunction. According to the guiding equation, this means that the electron is at rest when in this state. Nevertheless, it is distributed according to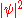
and no contradiction to experimental results is possible to detect.
Operators as observables leads many to believe that many operators are equivalent. De Broglie–Bohm theory, from this perspective, chooses the position observable as a favored observable rather than, say, the momentum observable. Again, the link to the position observable is a consequence of the dynamics. The motivation for de Broglie–Bohm theory is to describe a system of particles. This implies that the goal of the theory is to describe the positions of those particles at all times. Other observables do not have this compelling ontological status. Having definite positions explains having definite results such as flashes on a detector screen. Other observables would not lead to that conclusion, but there need not be any problem in defining a mathematical theory for other observables; see Hyman et al. for an exploration of the fact that a probability density and probability current can be defined for any set of commuting operators.
Bohm and Hiley have stated that they found their own choice of terms of an “interpretation in terms of hidden variables” to be too restrictive. In particular, a particle is not actually hidden but rather “is what is most directly manifested in an observation”, even if position and momentum of a particle cannot be observed with arbitrary precision. Put in simpler words, the particles postulated by the de Broglie–Bohm theory are anything but "hidden" variables: they are what the objects we see in everyday experience are made of; it is the wavefunction itself which is “hidden” in the sense of being invisible and not-directly-observable.
Even a whole particle trajectory can be measured by a weak measurement
. Such a measured trajectory coincides with the de Broglie–Bohm trajectory. In this sense, de Broglie–Bohm trajectories are not hidden variables. Or at least they are not more hidden than the wave function, in the sense that both can only be experimentally determined through a large number of measurements on an ensemble of equally prepared systems.
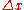, and the momentum with an accuracy of 
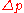, then 
If we make further measurements in order to get more information, we disturb the system and change the trajectory into a new one depending on the measurement setup; therefore, the measurement results are still subject to Heisenberg's uncertainty relation.
In de Broglie–Bohm theory, there is always a matter of fact about the position and momentum of a particle. Each particle has a well-defined trajectory. Observers have limited knowledge as to what this trajectory is (and thus of the position and momentum). It is the lack of knowledge of the particle's trajectory that accounts for the uncertainty relation. What one can know about a particle at any given time is described by the wavefunction. Since the uncertainty relation can be derived from the wavefunction in other interpretations of quantum mechanics, it can be likewise derived (in the epistemic sense mentioned above), on the de Broglie–Bohm theory.
To put the statement differently, the particles' positions are only known statistically. As in classical mechanics
, successive observations of the particles' positions refine the experimenter's knowledge of the particles' initial conditions. Thus, with succeeding observations, the initial conditions become more and more restricted. This formalism is consistent with the normal use of the Schrödinger equation.
For the derivation of the uncertainty relation, see Heisenberg uncertainty principle, noting that it describes it from the viewpoint of the Copenhagen interpretation
.
: it inspired John Stewart Bell
to prove his now-famous theorem
, which in turn led to the Bell test experiments
.
In the Einstein-Podolsky-Rosen paradox, the authors describe a thought-experiment one could perform on a pair of particles that have interacted, the results of which they interpreted as indicating that quantum mechanics is an incomplete theory.
Decades later John Bell
proved Bell's theorem
(see p. 14 in Bell), in which he showed that, if they are to agree with the empirical predictions of quantum mechanics, all such "hidden-variable" completions of quantum mechanics must either be nonlocal (as the Bohm interpretation is) or give up the assumption that experiments produce unique results (see counterfactual definiteness
and many-worlds interpretation
). In particular, Bell proved that any local theory with unique results must make empirical predictions satisfying a statistical constraint called "Bell's inequality".
Alain Aspect
performed a series of Bell test experiments
that test Bell's inequality using an EPR-type setup. Aspect's results show experimentally that Bell's inequality is in fact violated—meaning that the relevant quantum mechanical predictions are correct. In these Bell test experiments, entangled pairs of particles are created; the particles are separated, traveling to remote measuring apparatus. The orientation of the measuring apparatus can be changed while the particles are in flight, demonstrating the apparent non-locality of the effect.
The de Broglie–Bohm theory makes the same (empirically correct) predictions for the Bell test experiments as ordinary quantum mechanics. It is able to do this because it is manifestly nonlocal. It is often criticized or rejected based on this; Bell's attitude was: "It is a merit of the de Broglie–Bohm version to bring this [nonlocality] out so explicitly that it cannot be ignored."
The de Broglie–Bohm theory describes the physics in the Bell test experiments as follows: to understand the evolution of the particles, we need to set up a wave equation for both particles; the orientation of the apparatus affects the wavefunction. The particles in the experiment follow the guidance of the wavefunction. It is the wavefunction that carries the faster-than-light effect of changing the orientation of the apparatus. An analysis of exactly what kind of nonlocality is present and how it is compatible with relativity can be found in Maudlin. Note that in Bell's work, and in more detail in Maudlin's work, it is shown that the nonlocality does not allow for signaling at speeds faster than light.
in the early 2000s attempted to use the Bohm "particles" as an adaptive mesh that follows the actual trajectory of a quantum state in time and space. In the "quantum trajectory" method, one samples the quantum wavefunction with a mesh of quadrature points. One then evolves the quadrature points in time according to the Bohm equations of motion. At each time-step, one then re-synthesizes the wavefunction from the points, recomputes the quantum forces, and continues the calculation. (Quick-time movies of this for H+H2 reactive scattering can be found on the Wyatt group web-site at UT Austin.)
This approach has been adapted, extended, and used by a number of researchers in the Chemical Physics community as a way to compute semi-classical and quasi-classical molecular dynamics. A recent (2007) issue of the Journal of Physical Chemistry A was dedicated to Prof. Wyatt and his work on "Computational Bohmian Dynamics".
Eric R. Bittner
's group at the University of Houston
has advanced a statistical variant of this approach that uses Bayesian sampling technique to sample the quantum density and compute the quantum potential on a structureless mesh of points. This technique was recently used to estimate quantum effects in the heat-capacity of small clusters Nen for n~100.
There remain difficulties using the Bohmian approach, mostly associated with the formation of singularities in the quantum potential due to nodes in the
quantum wavefunction. In general, nodes forming due to interference effects lead to the case where
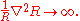
This results in an infinite force on the sample particles forcing them to move away from the node and often crossing the path of other sample points (which violates single-valuedness). Various schemes have been developed to overcome this; however, no general solution has yet emerged.
These methods, as does Bohm's Hamilton-Jacobi formulation, do not apply to situations in which the full dynamics of spin need to be taken into account.
field
. Everett's many-worlds interpretation
is an attempt to demonstrate that the wavefunction
alone is sufficient to account for all our observations. When we see the particle detectors flash or hear the click of a Geiger counter
then Everett's theory interprets this as our wavefunction responding to changes in the detector's wavefunction, which is responding in turn to the passage of another wavefunction (which we think of as a "particle", but is actually just another wave-packet). No particle (in the Bohm sense of having a defined position and velocity) exists, according to that theory. For this reason Everett sometimes referred to his own many-worlds approach as the "pure wave theory". Talking of Bohm's 1952 approach, Everett says:
In the Everettian view, then, the Bohm particles are superfluous entities, similar to, and equally as unnecessary as, for example, the luminiferous ether was found to be unnecessary in special relativity
. This argument of Everett's is sometimes called the "redundancy argument", since the superfluous particles are redundant in the sense of Occam's razor
.
Many authors have expressed critical views of the de Broglie-Bohm theory, by comparing it to Everett's many worlds approach. Many (but not all) proponents of the de Broglie-Bohm theory (such as Bohm and Bell) interpret the universal wave function as physically real. According to some supporters of Everett's theory, if the (never collapsing) wave function is taken to be physically real, then it is natural to interpret the theory as having the same many worlds as Everett's theory. In the Everettian view the role of the Bohm particle is to act as a "pointer", tagging, or selecting, just one branch of the universal wavefunction
(the assumption that this branch indicates which wave packet determines the observed result of a given experiment is called the "result assumption"); the other branches are designated "empty" and implicitly assumed by Bohm to be devoid of conscious observers. H. Dieter Zeh
comments on these "empty" branches:
David Deutsch
has expressed the same point more "acerbically":
According to Brown
& Wallace the de Broglie-Bohm particles play no role in the solution of the measurement problem. These authors claim that the "result assumption" (see above) is inconsistent with the view that there is no measurement problem in the predictable outcome (i.e. single-outcome) case. These authors also claim that a standard tacit assumption of the de Broglie-Bohm theory (that an observer becomes aware of configurations of particles of ordinary objects by means of correlations between such configurations and the configuration of the particles in the observer's brain) is unreasonable. This conclusion has been challenged by Valentini
who argues that the entirety of such objections arises from a failure to interpret de Broglie-Bohm theory on its own terms.
According to Peter R. Holland
, in a wider Hamiltonian framework, theories can be formulated in which particles do act back on the wave function.
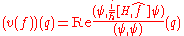where 
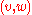is the local Hermitian inner product on the value space of the wavefunction.
pointed out that it was not compatible with a semi-classical technique Fermi had previously adopted in the case of inelastic scattering. Contrary to a popular legend, de Broglie actually gave the correct rebuttal that the particular technique could not be generalized for Pauli's purpose, although the audience might have been lost in the technical details and de Broglie's mild mannerism left the impression that Pauli's objection was valid. He was eventually persuaded to abandon this theory nonetheless in 1932 due to both the Copenhagen school's more successful P.R. efforts and his own inability to understand quantum decoherence
. Also in 1932, John von Neumann
published a paper, claiming to prove that all hidden-variable theories are impossible. This sealed the fate of de Broglie's theory for the next two decades. In truth, von Neumann's proof is based on invalid assumptions, such as quantum physics can be made local, and it does not really disprove the pilot-wave theory.
De Broglie's theory already applies to multiple spin-less particles, but lacks an adequate theory of measurement as no one understood quantum decoherence
at the time. An analysis of de Broglie's presentation is given in Bacciagaluppi et al.
Around this time Erwin Madelung
also developed a hydrodynamic version of Schrödinger's equation which is incorrectly considered as a basis for the density current derivation of the de Broglie–Bohm theory. The Madelung equations
, being quantum Euler equations (fluid dynamics), differ philosophically from the de Broglie–Bohm mechanics and are the basis of the hydrodynamic interpretation of quantum mechanics (quantum hydrodynamics
).
Peter R. Holland
has pointed out that, in 1927, Einstein
had submitted a preprint with a related proposal but, not convinced, had withdrawn it before publication. According to Holland, failure to appreciate key points of the de Broglie–Bohm theory has led to confusion, the key point being “that the trajectories of a many-body quantum system are correlated not because the particles exert a direct force on one another (à la Coulomb) but because all are acted upon by an entity – mathematically described by the wavefunction or functions of it – that lies beyond them.” This entity is the quantum potential
.
This stage applies to multiple particles, and is deterministic.
The de Broglie–Bohm theory is an example of a hidden variables theory. Bohm originally hoped that hidden variables could provide a local
, causal, objective
description that would resolve or eliminate many of the paradoxes of quantum mechanics, such as Schrödinger's cat
, the measurement problem
and the collapse of the wavefunction. However, Bell's theorem
complicates this hope, as it demonstrates that there can be no local hidden variable theory that is compatible with the predictions of quantum mechanics. The Bohmian interpretation is causal but not local
.
Bohm's paper was largely ignored by other physicists. Even Albert Einstein
did not consider it a satisfactory answer to the quantum non-locality question. The rest of the contemporary objections, however, were ad hominem
, focusing on Bohm's sympathy with liberals and supposed communists as exemplified by his refusal to give testimony to the House Un-American Activities Committee.
Eventually the cause was taken up by John Bell
. In "Speakable and Unspeakable in Quantum Mechanics" [Bell 1987], several of the papers refer to hidden variables theories (which include Bohm's). Bell showed that von Neumann's objection amounted to showing that hidden variables theories are nonlocal, and that nonlocality is a feature of all quantum mechanical systems.
. The term “Bohmian mechanics” is also often used to include most of the further extensions past the spin-less version of Bohm. While de Broglie–Bohm theory has Lagrangians and Hamilton-Jacobi equations as a primary focus and backdrop, with the icon of the quantum potential, Bohmian mechanics considers the continuity equation as primary and has the guiding equation as its icon. They are mathematically equivalent in so far as the Hamilton-Jacobi formulation applies, i.e., spin-less particles. The papers of Dürr et al. popularized the term.
All of non-relativistic quantum mechanics can be fully accounted for in this theory.
This stage covers work by Bohm and in collaboration with Jean-Pierre Vigier
and Basil Hiley
. Bohm is clear that this theory is non-deterministic (the work with Hiley includes a stochastic theory). As such, this theory is not, strictly speaking, a formulation of the de Broglie–Bohm theory. However, it deserves mention here because the term "Bohm Interpretation" is ambiguous between this theory and the de Broglie–Bohm theory.
Pilot wave
In theoretical physics, the Pilot Wave theory was the first known example of a hidden variable theory, presented by Louis de Broglie in 1927. Its more modern version, the Bohm interpretation,...
theory, Bohmian mechanics, and the causal interpretation, is an interpretation of quantum theory
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
. In addition to a wavefunction
Wavefunction
Not to be confused with the related concept of the Wave equationA wave function or wavefunction is a probability amplitude in quantum mechanics describing the quantum state of a particle and how it behaves. Typically, its values are complex numbers and, for a single particle, it is a function of...
on the space of all possible configurations, it also includes an actual configuration, even in situations where nobody observes it. The evolution over time of the configuration (that is, of the positions of all particles or the configuration of all fields) is defined by the wave function via a guiding equation. The evolution of the wavefunction over time is given by Schrödinger's equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
.
The de Broglie–Bohm theory expresses in an explicit manner the fundamental non-locality of quantum physics. The velocity of any one particle depends on the value of the wavefunction, which depends on the whole configuration of the universe.
This theory is deterministic. Most (but not all) relativistic variants require a preferred frame. Variants which include spin and curved spaces are known. It can be modified to include quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
. Bell's theorem
Bell's theorem
In theoretical physics, Bell's theorem is a no-go theorem, loosely stating that:The theorem has great importance for physics and the philosophy of science, as it implies that quantum physics must necessarily violate either the principle of locality or counterfactual definiteness...
was inspired by Bell's discovery of the work of David Bohm
David Bohm
David Joseph Bohm FRS was an American-born British quantum physicist who contributed to theoretical physics, philosophy, neuropsychology, and the Manhattan Project.-Youth and college:...
and his subsequent wondering if the obvious non-locality of the theory could be eliminated.
This theory results in a measurement formalism, analogous to thermodynamics for classical mechanics, which yields the standard quantum formalism generally associated with the Copenhagen interpretation
Copenhagen interpretation
The Copenhagen interpretation is one of the earliest and most commonly taught interpretations of quantum mechanics. It holds that quantum mechanics does not yield a description of an objective reality but deals only with probabilities of observing, or measuring, various aspects of energy quanta,...
. The measurement problem is resolved by this theory since the outcome of an experiment is registered by the configuration of the particles of the experimental apparatus after the experiment is completed. The familiar wavefunction collapse of standard quantum mechanics emerges from an analysis of subsystems and the quantum equilibrium hypothesis.
The theory has a number of equivalent mathematical formulations and has been presented by a number of different names. The de Broglie wave has a macroscopical analogy termed Faraday wave
Faraday wave
Faraday waves, also known as Faraday ripples, named after Michael Faraday, are nonlinear standing waves that appear on liquids enclosed by a vibrating receptacle. When the vibration frequency exceeds a critical value, the flat hydrostatic surface becomes unstable. This is known as the Faraday...
.
Overview
De Broglie–Bohm theory is based on the following:We have a configuration
of the universe, described by coordinates , which is an element of the configuration space . The configuration space is different for different versions of pilot wave theory. For example, this may be the space of positions of particles, or, in case of field theory, the space of field configurations 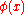. The configuration evolves according to the guiding equation.Here,
is the standard complex-valued wavefunction known from quantum theory, which evolves according to Schrödinger's equation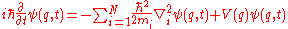This already completes the specification of the theory for any quantum theory with Hamilton operator of type
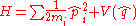.If the configuration is distributed according to
at some moment of time , this holds for all times. Such a state is named quantum equilibrium. In quantum equilibrium, this theory will agree with the results of standard quantum mechanics.Two-slit experiment
The double-slit experimentDouble-slit experiment
The double-slit experiment, sometimes called Young's experiment, is a demonstration that matter and energy can display characteristics of both waves and particles...
is an illustration of wave-particle duality. In it, a beam of particles (such as photons) travels through a barrier with two slits removed. If one puts a detector screen on the other side, the pattern of detected particles shows interference fringes characteristic of waves; however, the detector screen responds to particles. The system exhibits behaviour of both waves (interference patterns) and particles (dots on the screen).
If we modify this experiment so that one slit is closed, no interference pattern is observed. Thus, the state of both slits affects the final results. We can also arrange to have a minimally invasive detector at one of the slits to detect which slit the particle went through. When we do that, the interference pattern disappears.
The Copenhagen interpretation
Copenhagen interpretation
The Copenhagen interpretation is one of the earliest and most commonly taught interpretations of quantum mechanics. It holds that quantum mechanics does not yield a description of an objective reality but deals only with probabilities of observing, or measuring, various aspects of energy quanta,...
states that the particles are not localised in space until they are detected, so that, if there is not any detector on the slits, there is no matter of fact about which slit the particle has passed through. If one slit has a detector on it, then the wavefunction collapses due to that detection.
In de Broglie–Bohm theory, the wavefunction travels through both slits, but each particle has a well-defined trajectory and passes through exactly one of the slits. The final position of the particle on the detector screen and the slit through which the particle passes by is determined by the initial position of the particle. Such initial position is not controllable by the experimenter, so there is an appearance of randomness in the pattern of detection. The wave function interferes with itself and guides the particles in such a way that the particles avoid the regions in which the interference is destructive and are attracted to the regions in which the interference is constructive, resulting in the interference pattern on the detector screen.
To explain the behavior when the particle is detected to go through one slit, one needs to appreciate the role of the conditional wavefunction and how it results in the collapse of the wavefunction; this is explained below. The basic idea is that the environment registering the detection effectively separates the two wave packets in configuration space.
The ontology
The ontologyOntology
Ontology is the philosophical study of the nature of being, existence or reality as such, as well as the basic categories of being and their relations...
of de Broglie-Bohm theory consists of a configuration
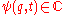of the universe and a pilot wave . The configuration space can be chosen differently, as in classical mechanics and standard quantum mechanics.Thus, the ontology of pilot wave theory contains as the trajectory
we know from classical mechanics, as the wave function 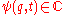of quantum theory. So, at every moment of time there exists not only a wave function, but also a well-defined configuration of the whole universe. The correspondence to our experiences is made by the identification of the configuration of our brain with some part of the configuration of the whole universe 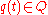, as in classical mechanics.While the ontology of classical mechanics is part of the ontology of de Broglie–Bohm theory, the dynamics are very different. In classical mechanics, the accelerations of the particles are given by forces. In de Broglie–Bohm theory, the velocities of the particles are given by the wavefunction.
The wavefunction itself, and not the particles, determines the dynamical evolution of the system: the particles do not act back onto the wave function. As Bohm and Hiley worded it, “the Schrodinger equation for the quantum field does not have sources, nor does it have any other way by which the field could be directly affected by the condition of the particles [...] the quantum theory can be understood completely in terms of the assumption that the quantum field has no sources or other forms of dependence on the particles”. P. Holland considers this lack of reciprocal action of particles and wave function to be one “[a]mong the many nonclassical properties exhibited by this theory”. It should be noted however that Holland has later called this a merely apparent lack of back reaction, due to the incompleteness of the description.
In what follows below, we will give the setup for one particle moving in
followed by the setup for particles moving in 3 dimensions. In the first instance, configuration space and real space are the same while in the second, real space is still , but configuration space becomes 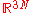. While the particle positions themselves are in real space, the velocity field and wavefunction are on configuration space which is how particles are entangled with each other in this theory.Extensions to this theory include spin and more complicated configuration spaces.
We use variations of
for particle positions while 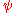represents the complex-valued wavefunction on configuration space.Guiding equation
For a single particle moving in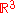, the particle's velocity is given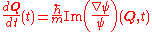.For many particles, we label them as
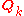for the th particle and their velocities are given by.The main fact to notice is that this velocity field depends on the actual positions of all of the
particles in the universe. As explained below, in most experimental situations, the influence of all of those particles can be encapsulated into an effective wavefunction for a subsystem of the universe.Schrödinger's equation
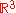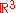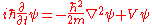
The one particle Schrödinger equation governs the time evolution of a complex-valued wavefunction on. The equation represents a quantized version of the total energy of a classical system evolving under a real-valued potential function on :For many particles, the equation is the same except that
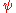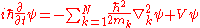
and are now on configuration space, .This is the same wavefunction of conventional quantum mechanics.
Relation to the Born Rule
In Bohm's original papers [Bohm 1952], he discusses how de Broglie–Bohm theory results in the usual measurement results of quantum mechanics. The main idea is that this is true if the positions of the particles satisfy the statistical distribution given by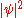. And that distribution is guaranteed to be true for all time by the guiding equation if the initial distribution of the particles satisfies 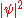.For a given experiment, we can postulate this as being true and verify experimentally that it does indeed hold true, as it does. But, as argued in Dürr et al., one needs to argue that this distribution for subsystems is typical. They argue that
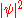by virtue of its equivariance under the dynamical evolution of the system, is the appropriate measure of typicality for initial conditions of the positions of the particles. They then prove that the vast majority of possible initial configurations will give rise to statistics obeying the Born ruleBorn rule
The Born rule is a law of quantum mechanics which gives the probability that a measurement on a quantum system will yield a given result. It is named after its originator, the physicist Max Born. The Born rule is one of the key principles of quantum mechanics...
(i.e.,
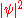) for measurement outcomes. In summary, in a universe governed by the de Broglie–Bohm dynamics, Born rule behavior is typical.The situation is thus analogous to the situation in classical statistical physics. A low entropy initial condition will, with overwhelmingly high probability, evolve into a higher entropy state: behavior consistent with the second law of thermodynamics is typical. There are, of course, anomalous initial conditions which would give rise to violations of the second law. However, absent some very detailed evidence supporting the actual realization of one of those special initial conditions, it would be quite unreasonable to expect anything but the actually observed uniform increase of entropy. Similarly, in the de Broglie–Bohm theory, there are anomalous initial conditions which would produce measurement statistics in violation of the Born rule (i.e., in conflict with the predictions of standard quantum theory). But the typicality theorem shows that, absent some particular reason to believe one of those special initial conditions was in fact realized, Born rule behavior is what one should expect.
It is in that qualified sense that Born rule is, for the de Broglie–Bohm theory, a theorem rather than (as in ordinary quantum theory) an additional postulate.
It can also be shown that a distribution of particles that is not distributed according to the Born rule (that is, a distribution 'out of quantum equilibrium') and evolving under the de Broglie-Bohm dynamics is overwhelmingly likely to evolve dynamically into a state distributed as
. See, for example Ref.. A pretty video of the electron density in a 2D box evolving under this process is available here.
The conditional wave function of a subsystem
In the formulation of the De Broglie–Bohm theory, there is only a wave function for the entire universe (which always evolves by the Schrödinger equation). However, once the theory is formulated, it is convenient to introduce a notion of wave function also for subsystems of the universe. Let us write the wave function of the universe as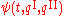, where 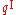denotes the configuration variables associated to some subsystem (I) of the universe and 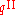denotes the remaining configuration variables. Denote, respectively, by 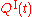and by the actual configuration of subsystem (I) and of the rest of the universe. For simplicity, we consider here only the spinless case. The conditional wave function of subsystem (I) is defined by:It follows immediately from the fact that
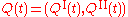satisfies the guiding equation that also the configuration 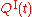satisfies a guiding equation identical to the one presented in the formulation of the theory, with the universal wave function 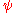replaced with the conditional wave function 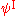. Also, the fact that is random with probability densityProbability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
given by the square modulus of
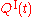implies that the conditional probability density of given 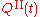is given by the square modulus of the (normalized) conditional wave function 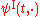(in the terminology of Dürr et al. this fact is called the fundamental conditional probability formula).Unlike the universal wave function, the conditional wave function of a subsystem does not always evolve by the Schrödinger equation, but in many situations it does. For instance, if the universal wave function factors as:
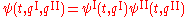
then the conditional wave function of subsystem (I) is (up to an irrelevant scalar factor) equal to
(this is what Standard Quantum Theory would regard as the wave function of subsystem (I)). If, in addition, the Hamiltonian does not contain an interaction term between subsystems (I) and (II) then does satisfy a Schrödinger equation. More generally, assume that the universal wave function 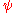can be written in the form: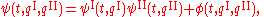where
solves Schrödinger equation and 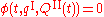for all and . Then, again, the conditional wave function of subsystem (I) is (up to an irrelevant scalar factor) equal to and if the Hamiltonian does not contain an interaction term between subsystems (I) and (II), 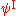satisfies a Schrödinger equation.The fact that the conditional wave function of a subsystem does not always evolve by the Schrödinger equation is related to the fact that the usual collapse rule of Standard Quantum Theory emerges from the Bohmian formalism when one considers conditional wave functions of subsystems.
Spin
To incorporate spin, the wavefunction becomes complex-vector valued. The value space is called spin space; for a spin-1/2 particle, spin space can be taken to be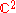. The guiding equation is modified by taking inner products in spin space to reduce the complex vectors to complex numbers. The Schrödinger equation is modified by adding a Pauli spin term.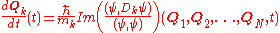where
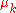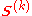is the magnetic moment of the th particle, is the appropriate spin operator acting on the 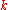th particle's spin space,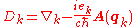, and are, respectively, the magnetic field and the vector potential in 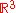(all other functions are fully on configuration space), is the charge of the 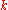th particle, and is the inner product in spin space ,For an example of a spin space, a system consisting of two spin 1/2 particle and one spin 1 particle has a wavefunctions of the form
.That is, its spin space is a 12 dimensional space.
Curved space
To extend de Broglie–Bohm theory to curved space (Riemannian manifolds in mathematical parlance), one simply notes that all of the elements of these equations make sense, such as gradients and Laplacians. Thus, we use equations that have the same form as above. Topological and boundary conditions may apply in supplementing the evolution of Schrödinger's equation.For a de Broglie–Bohm theory on curved space with spin, the spin space becomes a vector bundle
Vector bundle
In mathematics, a vector bundle is a topological construction that makes precise the idea of a family of vector spaces parameterized by another space X : to every point x of the space X we associate a vector space V in such a way that these vector spaces fit together...
over configuration space and the potential in Schrödinger's equation becomes a local self-adjoint operator acting on that space.
Quantum field theory
In Dürr et al., the authors describe an extension of de Broglie–Bohm theory for handling creation and annihilation operators, which they refer to as “Bell-type quantum field theories”. The basic idea is that configuration space becomes the (disjoint) space of all possible configurations of any number of particles. For part of the time, the system evolves deterministically under the guiding equation with a fixed number of particles. But under a stochastic process, particles may be created and annihilated. The distribution of creation events is dictated by the wavefunction. The wavefunction itself is evolving at all times over the full multi-particle configuration space.Hrvoje Nikolić introduces a purely deterministic de Broglie–Bohm theory of particle creation and destruction, according to which particle trajectories are continuous, but particle detectors behave as if particles have been created or destroyed even when a true creation or destruction of particles does not take place.
Exploiting nonlocality
Antony ValentiniAntony Valentini
Antony Valentini is a theoretical physicist and a professor at Clemson University. He is known for his work on the foundations of quantum physics.- Education and career :...
has extended the de Broglie–Bohm theory to include signal nonlocality that would allow entanglement to be used as a stand-alone communication channel without a secondary classical "key" signal to "unlock" the message encoded in the entanglement. This violates orthodox quantum theory but it has the virtue that it makes the parallel universes of the chaotic inflation theory
Chaotic inflation theory
The Chaotic Inflation theory is a variety of the inflationary universe model, which is itself an outgrowth of the Big Bang theory. Chaotic Inflation, proposed by physicist Andrei Linde, models our universe as one of many that grew as part of a multiverse owing to a vacuum that had not decayed to...
observable in principle.
Unlike de Broglie–Bohm theory, Valentini's theory has the wavefunction evolution also depend on the ontological variables. This introduces an instability, a feedback loop that pushes the hidden variables out of "sub-quantal heat death". The resulting theory becomes nonlinear and non-unitary.
Relativity
Pilot wave theory is explicitly nonlocal. As a consequence, most relativistic variants of pilot wave theory need a foliation of space-timeFrame of reference
A frame of reference in physics, may refer to a coordinate system or set of axes within which to measure the position, orientation, and other properties of objects in it, or it may refer to an observational reference frame tied to the state of motion of an observer.It may also refer to both an...
. While this is in conflict with the standard interpretation of relativity, the preferred foliation, if unobservable, does not lead to any empirical conflicts with relativity.
The relation between nonlocality and preferred foliation can be better understood as follows. In de Broglie–Bohm theory, nonlocality manifests as the fact that the velocity and acceleration of one particle depends on the instantaneous positions of all other particles. On the other hand, in the theory of relativity the concept of instantaneousness does not have an invariant meaning. Thus, to define particle trajectories, one needs an additional rule that defines which space-time points should be considered instantaneous. The simplest way to achieve this is to introduce a preferred foliation of space-time by hand, such that each hypersurface of the foliation defines a hypersurface of equal time. However, this way (which explicitly breaks the relativistic covariance) is not the only way. It is also possible that a rule which defines instantaneousness is contingent
Contingency (philosophy)
In philosophy and logic, contingency is the status of propositions that are neither true under every possible valuation nor false under every possible valuation . A contingent proposition is neither necessarily true nor necessarily false...
, by emerging dynamically from relativistic covariant laws combined with particular initial conditions. In this way, the need for a preferred foliation can be avoided and relativistic covariance can be saved.
There has been work in developing relativistic versions of de Broglie–Bohm theory. See Bohm and Hiley: The Undivided Universe, and http://xxx.lanl.gov/abs/quant-ph/0208185, http://xxx.lanl.gov/abs/quant-ph/0302152, and references therein. Another approach is given in the work of Dürr et al. in which they use Bohm-Dirac models and a Lorentz-invariant foliation of space-time.
Initially, it been considered impossible to set out a description of photon trajectories in the de Broglie–Bohm theory in view of the difficulties of describing bosons relativistically. In 1996, Partha Ghose
Partha Ghose
Partha Ghose, born 1939, is an Indian physicist, author, anchorperson and professor at the S.N. Bose National Centre for Basic Sciences in Calcutta.Partha Ghose is quoted as one of India's best known popularizers of modern science.Together with K...
had presented a relativistic quantum mechanical description of spin-0 and spin-1 bosons starting from the Duffin–Kemmer–Petiau equation, setting out Bohmian trajectories for massive bosons and for massless bosons, thus also for photon
Photon
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
s. In 2001, Jean-Pierre Vigier
Jean-Pierre Vigier
Jean-Pierre Vigier earned his Ph.D. in Mathematics from University of Geneva in 1946 and in 1948 was appointed assistant to Louis de Broglie, a position he held until the latter's retirement in 1962. Vigier was professor emeritus at in the Department of Gravitational Physics at Pierre et Marie...
emphasized the importance of deriving a well-defined description of light in terms of particle trajectories in the framework of either the Bohmian mechanics or the Nelson stochastic mechanics. The same year, Ghose worked out Bohmian photon trajectories for specific cases. Subsequent weak measurement
Weak measurement
Weak measurements are a type of quantum measurement, where the measured system is very weakly coupled to the measuring device. After the measurement the measuring device pointer is shifted by what is called the "weak value". So that a pointer initially pointing at zero before the measurement would...
experiments yielded trajectories which coincide with the predicted trajectories.
Nikolić has proposed a Lorentz-covariant formulation of the Bohmian interpretation of many-particle wave functions. He has developed a generalized relativistic-invariant probabilistic interpretation of quantum theory, in which
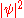is no longer a probability density in space, but a probability density in space-time. He uses this generalized probabilistic interpretation to formulate a relativistic-covariant version of de Broglie–Bohm theory without introducing a preferred foliation of space-time. His work also covers the extension of the Bohmian interpretation to a quantization of fields and strings.Results
Below are some highlights of the results that arise out of an analysis of de Broglie–Bohm theory. Experimental results agree with all of the standard predictions of quantum mechanics in so far as the latter has predictions. However, while standard quantum mechanics is limited to discussing the results of 'measurements', de Broglie–Bohm theory is a theory which governs the dynamics of a system without the intervention of outside observers (p. 117 in Bell).The basis for agreement with standard quantum mechanics is that the particles are distributed according to
. This is a statement of observer ignorance, but it can be proven that for a universe governed by this theory, this will typically be the case. There is apparent collapse of the wave function governing subsystems of the universe, but there is no collapse of the universal wavefunction.Measuring spin and polarization
According to ordinary quantum theory, it is not possible to measure the spinSpin (physics)
In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
or polarization of a particle directly; instead, the component in one direction is measured; the outcome from a single particle may be 1, meaning that the particle is aligned with the measuring apparatus, or -1, meaning that it is aligned the opposite way. For an ensemble of particles, if we expect the particles to be aligned, the results are all 1. If we expect them to be aligned oppositely, the results are all -1. For other alignments, we expect some results to be 1 and some to be -1 with a probability that depends on the expected alignment. For a full explanation of this, see the Stern-Gerlach Experiment.
In de Broglie–Bohm theory, the results of a spin experiment cannot be analyzed without some knowledge of the experimental setup. It is possible to modify the setup so that the trajectory of the particle is unaffected, but that the particle with one setup registers as spin up while in the other setup it registers as spin down. Thus, for the de Broglie–Bohm theory, the particle's spin is not an intrinsic property of the particle—instead spin is, so to speak, in the wave function of the particle in relation to the particular device being used to measure the spin. This is an illustration of what is sometimes referred to as contextuality, and is related to naive realism about operators.
Measurements, the quantum formalism, and observer independence
De Broglie–Bohm theory gives the same results as quantum mechanics. It treats the wavefunction as a fundamental object in the theory as the wavefunction describes how the particles move. This means that no experiment can distinguish between the two theories. This section outlines the ideas as to how the standard quantum formalism arises out of quantum mechanics. References include Bohm's original 1952 paper and Dürr et al.Collapse of the wavefunction
De Broglie–Bohm theory is a theory that applies primarily to the whole universe. That is, there is a single wavefunction governing the motion of all of the particles in the universe according to the guiding equation. Theoretically, the motion of one particle depends on the positions of all of the other particles in the universe. In some situations, such as in experimental systems, we can represent the system itself in terms of a de Broglie–Bohm theory in which the wavefunction of the system is obtained by conditioning on the environment of the system. Thus, the system can be analyzed with Schrödinger's equation and the guiding equation, with an initial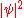 distribution for the particles in the system (see the section on the conditional wave function of a subsystem for details).It requires a special setup for the conditional wavefunction of a system to obey a quantum evolution. When a system interacts with its environment, such as through a measurement, the conditional wavefunction of the system evolves in a different way. The evolution of the universal wavefunction can become such that the wavefunction of the system appears to be in a superposition of distinct states. But if the environment has recorded the results of the experiment, then using the actual Bohmian configuration of the environment to condition on, the conditional wavefunction collapses to just one alternative, the one corresponding with the measurement results.
Collapse
Wavefunction collapse
In quantum mechanics, wave function collapse is the phenomenon in which a wave function—initially in a superposition of several different possible eigenstates—appears to reduce to a single one of those states after interaction with an observer...
of the universal wavefunction never occurs in de Broglie–Bohm theory. Its entire evolution is governed by Schrödinger's equation and the particles' evolutions are governed by the guiding equation. Collapse only occurs in a phenomenological way for systems that seem to follow their own Schrödinger's equation. As this is an effective description of the system, it is a matter of choice as to what to define the experimental system to include and this will affect when "collapse" occurs.
Operators as observables
In the standard quantum formalism, measuring observables is generally thought of as measuring operators on the Hilbert space. For example, measuring position is considered to be a measurement of the position operator. This relationship between physical measurements and Hilbert space operators is, for standard quantum mechanics, an additional axiom of the theory. The de Broglie–Bohm theory, by contrast, requires no such measurement axioms (and measurement as such is not a dynamically distinct or special sub-category of physical processes in the theory). In particular, the usual operators-as-observables formalism is, for de Broglie–Bohm theory, a theorem. A major point of the analysis is that many of the measurements of the observables do not correspond to properties of the particles; they are (as in the case of spin discussed above) measurements of the wavefunction.In the history of de Broglie–Bohm theory, the proponents have often had to deal with claims that this theory is impossible. Such arguments are generally based on inappropriate analysis of operators as observables. If one believes that spin measurements are indeed measuring the spin of a particle that existed prior to the measurement, then one does reach contradictions. De Broglie–Bohm theory deals with this by noting that spin is not a feature of the particle, but rather that of the wavefunction. As such, it only has a definite outcome once the experimental apparatus is chosen. Once that is taken into account, the impossibility theorems become irrelevant.
There have also been claims that experiments reject the Bohm trajectories
http://arxiv.org/abs/quant-ph/0206196 in favor of the standard QM lines. But as shown in http://arxiv.org/abs/quant-ph/0108038 and http://arxiv.org/abs/quant-ph/0305131, such experiments cited above only disprove a misinterpretation of the de Broglie–Bohm theory, not the theory itself.
There are also objections to this theory based on what it says about particular situations usually involving eigenstates of an operator. For example, the ground state of hydrogen is a real wavefunction. According to the guiding equation, this means that the electron is at rest when in this state. Nevertheless, it is distributed according to
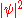and no contradiction to experimental results is possible to detect.Operators as observables leads many to believe that many operators are equivalent. De Broglie–Bohm theory, from this perspective, chooses the position observable as a favored observable rather than, say, the momentum observable. Again, the link to the position observable is a consequence of the dynamics. The motivation for de Broglie–Bohm theory is to describe a system of particles. This implies that the goal of the theory is to describe the positions of those particles at all times. Other observables do not have this compelling ontological status. Having definite positions explains having definite results such as flashes on a detector screen. Other observables would not lead to that conclusion, but there need not be any problem in defining a mathematical theory for other observables; see Hyman et al. for an exploration of the fact that a probability density and probability current can be defined for any set of commuting operators.
Hidden variables
De Broglie–Bohm theory is often referred to as a "hidden variable" theory. The alleged applicability of the term "hidden variable" comes from the fact that the particles postulated by Bohmian mechanics do not influence the evolution of the wavefunction. The argument is that, because adding particles does not have an effect on the wavefunction's evolution, such particles must not have effects at all and are, thus, unobservable, since they cannot have an effect on observers. There is no analogue of Newton's third law in this theory. The idea is supposed to be that, since particles cannot influence the wavefunction, and it is the wavefunction that determines measurement predictions through the Born rule, the particles are superfluous and unobservable.Bohm and Hiley have stated that they found their own choice of terms of an “interpretation in terms of hidden variables” to be too restrictive. In particular, a particle is not actually hidden but rather “is what is most directly manifested in an observation”, even if position and momentum of a particle cannot be observed with arbitrary precision. Put in simpler words, the particles postulated by the de Broglie–Bohm theory are anything but "hidden" variables: they are what the objects we see in everyday experience are made of; it is the wavefunction itself which is “hidden” in the sense of being invisible and not-directly-observable.
Even a whole particle trajectory can be measured by a weak measurement
Weak measurement
Weak measurements are a type of quantum measurement, where the measured system is very weakly coupled to the measuring device. After the measurement the measuring device pointer is shifted by what is called the "weak value". So that a pointer initially pointing at zero before the measurement would...
. Such a measured trajectory coincides with the de Broglie–Bohm trajectory. In this sense, de Broglie–Bohm trajectories are not hidden variables. Or at least they are not more hidden than the wave function, in the sense that both can only be experimentally determined through a large number of measurements on an ensemble of equally prepared systems.
Heisenberg's uncertainty principle
The Heisenberg uncertainty principle states that when two complementary measurements are made, there is a limit to the product of their accuracy. As an example, if one measures the position with an accuracy of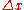, and the momentum with an accuracy of 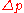, then If we make further measurements in order to get more information, we disturb the system and change the trajectory into a new one depending on the measurement setup; therefore, the measurement results are still subject to Heisenberg's uncertainty relation.In de Broglie–Bohm theory, there is always a matter of fact about the position and momentum of a particle. Each particle has a well-defined trajectory. Observers have limited knowledge as to what this trajectory is (and thus of the position and momentum). It is the lack of knowledge of the particle's trajectory that accounts for the uncertainty relation. What one can know about a particle at any given time is described by the wavefunction. Since the uncertainty relation can be derived from the wavefunction in other interpretations of quantum mechanics, it can be likewise derived (in the epistemic sense mentioned above), on the de Broglie–Bohm theory.
To put the statement differently, the particles' positions are only known statistically. As in classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
, successive observations of the particles' positions refine the experimenter's knowledge of the particles' initial conditions. Thus, with succeeding observations, the initial conditions become more and more restricted. This formalism is consistent with the normal use of the Schrödinger equation.
For the derivation of the uncertainty relation, see Heisenberg uncertainty principle, noting that it describes it from the viewpoint of the Copenhagen interpretation
Copenhagen interpretation
The Copenhagen interpretation is one of the earliest and most commonly taught interpretations of quantum mechanics. It holds that quantum mechanics does not yield a description of an objective reality but deals only with probabilities of observing, or measuring, various aspects of energy quanta,...
.
Quantum entanglement, Einstein-Podolsky-Rosen paradox, Bell's theorem, and nonlocality
De Broglie–Bohm theory highlighted the issue of nonlocalityNonlocality
In Classical physics, nonlocality is the direct influence of one object on another, distant object. In Quantum mechanics, nonlocality refers to the absence of a local, realist model in agreement with quantum mechanical predictions.Nonlocality may refer to:...
: it inspired John Stewart Bell
John Stewart Bell
John Stewart Bell FRS was a British physicist from Northern Ireland , and the originator of Bell's theorem, a significant theorem in quantum physics regarding hidden variable theories.- Early life and work :...
to prove his now-famous theorem
Bell's theorem
In theoretical physics, Bell's theorem is a no-go theorem, loosely stating that:The theorem has great importance for physics and the philosophy of science, as it implies that quantum physics must necessarily violate either the principle of locality or counterfactual definiteness...
, which in turn led to the Bell test experiments
Bell test experiments
The Bell test experiments serve to investigate the validity of the entanglement effect in quantum mechanics by using some kind of Bell inequality...
.
In the Einstein-Podolsky-Rosen paradox, the authors describe a thought-experiment one could perform on a pair of particles that have interacted, the results of which they interpreted as indicating that quantum mechanics is an incomplete theory.
Decades later John Bell
John Stewart Bell
John Stewart Bell FRS was a British physicist from Northern Ireland , and the originator of Bell's theorem, a significant theorem in quantum physics regarding hidden variable theories.- Early life and work :...
proved Bell's theorem
Bell's theorem
In theoretical physics, Bell's theorem is a no-go theorem, loosely stating that:The theorem has great importance for physics and the philosophy of science, as it implies that quantum physics must necessarily violate either the principle of locality or counterfactual definiteness...
(see p. 14 in Bell), in which he showed that, if they are to agree with the empirical predictions of quantum mechanics, all such "hidden-variable" completions of quantum mechanics must either be nonlocal (as the Bohm interpretation is) or give up the assumption that experiments produce unique results (see counterfactual definiteness
Counterfactual definiteness
In some interpretations of quantum mechanics, counterfactual definiteness is the ability to speak with meaning of the definiteness of the results of measurements that have not been performed...
and many-worlds interpretation
Many-worlds interpretation
The many-worlds interpretation is an interpretation of quantum mechanics that asserts the objective reality of the universal wavefunction, but denies the actuality of wavefunction collapse. Many-worlds implies that all possible alternative histories and futures are real, each representing an...
). In particular, Bell proved that any local theory with unique results must make empirical predictions satisfying a statistical constraint called "Bell's inequality".
Alain Aspect
Alain Aspect
Alain Aspect is a French physicist noted for his experimental work on quantum entanglement....
performed a series of Bell test experiments
Bell test experiments
The Bell test experiments serve to investigate the validity of the entanglement effect in quantum mechanics by using some kind of Bell inequality...
that test Bell's inequality using an EPR-type setup. Aspect's results show experimentally that Bell's inequality is in fact violated—meaning that the relevant quantum mechanical predictions are correct. In these Bell test experiments, entangled pairs of particles are created; the particles are separated, traveling to remote measuring apparatus. The orientation of the measuring apparatus can be changed while the particles are in flight, demonstrating the apparent non-locality of the effect.
The de Broglie–Bohm theory makes the same (empirically correct) predictions for the Bell test experiments as ordinary quantum mechanics. It is able to do this because it is manifestly nonlocal. It is often criticized or rejected based on this; Bell's attitude was: "It is a merit of the de Broglie–Bohm version to bring this [nonlocality] out so explicitly that it cannot be ignored."
The de Broglie–Bohm theory describes the physics in the Bell test experiments as follows: to understand the evolution of the particles, we need to set up a wave equation for both particles; the orientation of the apparatus affects the wavefunction. The particles in the experiment follow the guidance of the wavefunction. It is the wavefunction that carries the faster-than-light effect of changing the orientation of the apparatus. An analysis of exactly what kind of nonlocality is present and how it is compatible with relativity can be found in Maudlin. Note that in Bell's work, and in more detail in Maudlin's work, it is shown that the nonlocality does not allow for signaling at speeds faster than light.
Classical limit
Bohm's formulation of de Broglie–Bohm theory in terms of a classical-looking version has the merits that the emergence of classical behavior seems to follow immediately for any situation in which the quantum potential is negligible, as noted by Bohm in 1952. Modern methods of decoherence are relevant to an analysis of this limit. See Allori et al. for steps towards a rigorous analysis.Quantum trajectory method
Work by Robert E. WyattRobert E. Wyatt
Robert E. Wyatt is a professor of chemistry at University of Texas at Austin, Department Chemistry and Biochemistry.- Work :His work is focussed on theoretical chemistry, including the quantum theory of chemical reactions and the theory of intramolecular energy transfer...
in the early 2000s attempted to use the Bohm "particles" as an adaptive mesh that follows the actual trajectory of a quantum state in time and space. In the "quantum trajectory" method, one samples the quantum wavefunction with a mesh of quadrature points. One then evolves the quadrature points in time according to the Bohm equations of motion. At each time-step, one then re-synthesizes the wavefunction from the points, recomputes the quantum forces, and continues the calculation. (Quick-time movies of this for H+H2 reactive scattering can be found on the Wyatt group web-site at UT Austin.)
This approach has been adapted, extended, and used by a number of researchers in the Chemical Physics community as a way to compute semi-classical and quasi-classical molecular dynamics. A recent (2007) issue of the Journal of Physical Chemistry A was dedicated to Prof. Wyatt and his work on "Computational Bohmian Dynamics".
Eric R. Bittner
Eric R. Bittner
Eric R. Bittner is a theoretical chemist, physicist, and distinguished professor of chemical physics at the University of Houston.- Biography :...
's group at the University of Houston
University of Houston
The University of Houston is a state research university, and is the flagship institution of the University of Houston System. Founded in 1927, it is Texas's third-largest university with nearly 40,000 students. Its campus spans 667 acres in southeast Houston, and was known as University of...
has advanced a statistical variant of this approach that uses Bayesian sampling technique to sample the quantum density and compute the quantum potential on a structureless mesh of points. This technique was recently used to estimate quantum effects in the heat-capacity of small clusters Nen for n~100.
There remain difficulties using the Bohmian approach, mostly associated with the formation of singularities in the quantum potential due to nodes in the
quantum wavefunction. In general, nodes forming due to interference effects lead to the case where
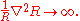
This results in an infinite force on the sample particles forcing them to move away from the node and often crossing the path of other sample points (which violates single-valuedness). Various schemes have been developed to overcome this; however, no general solution has yet emerged.
These methods, as does Bohm's Hamilton-Jacobi formulation, do not apply to situations in which the full dynamics of spin need to be taken into account.
Occam's razor criticism
Both Hugh Everett III and Bohm treated the wavefunction as a physically realScientific realism
Scientific realism is, at the most general level, the view that the world described by science is the real world, as it is, independent of what we might take it to be...
field
Field (physics)
In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...
. Everett's many-worlds interpretation
Many-worlds interpretation
The many-worlds interpretation is an interpretation of quantum mechanics that asserts the objective reality of the universal wavefunction, but denies the actuality of wavefunction collapse. Many-worlds implies that all possible alternative histories and futures are real, each representing an...
is an attempt to demonstrate that the wavefunction
Wavefunction
Not to be confused with the related concept of the Wave equationA wave function or wavefunction is a probability amplitude in quantum mechanics describing the quantum state of a particle and how it behaves. Typically, its values are complex numbers and, for a single particle, it is a function of...
alone is sufficient to account for all our observations. When we see the particle detectors flash or hear the click of a Geiger counter
Geiger counter
A Geiger counter, also called a Geiger–Müller counter, is a type of particle detector that measures ionizing radiation. They detect the emission of nuclear radiation: alpha particles, beta particles or gamma rays. A Geiger counter detects radiation by ionization produced in a low-pressure gas in a...
then Everett's theory interprets this as our wavefunction responding to changes in the detector's wavefunction, which is responding in turn to the passage of another wavefunction (which we think of as a "particle", but is actually just another wave-packet). No particle (in the Bohm sense of having a defined position and velocity) exists, according to that theory. For this reason Everett sometimes referred to his own many-worlds approach as the "pure wave theory". Talking of Bohm's 1952 approach, Everett says:
In the Everettian view, then, the Bohm particles are superfluous entities, similar to, and equally as unnecessary as, for example, the luminiferous ether was found to be unnecessary in special relativity
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
. This argument of Everett's is sometimes called the "redundancy argument", since the superfluous particles are redundant in the sense of Occam's razor
Occam's razor
Occam's razor, also known as Ockham's razor, and sometimes expressed in Latin as lex parsimoniae , is a principle that generally recommends from among competing hypotheses selecting the one that makes the fewest new assumptions.-Overview:The principle is often summarized as "simpler explanations...
.
Many authors have expressed critical views of the de Broglie-Bohm theory, by comparing it to Everett's many worlds approach. Many (but not all) proponents of the de Broglie-Bohm theory (such as Bohm and Bell) interpret the universal wave function as physically real. According to some supporters of Everett's theory, if the (never collapsing) wave function is taken to be physically real, then it is natural to interpret the theory as having the same many worlds as Everett's theory. In the Everettian view the role of the Bohm particle is to act as a "pointer", tagging, or selecting, just one branch of the universal wavefunction
Universal wavefunction
The Universal Wavefunction or Universal Wave Function is a term introduced by Hugh Everett in his Princeton PhD thesis The Theory of the Universal Wave Function, and forms a core concept in the relative state interpretation or many-worlds interpretation of quantum mechanics...
(the assumption that this branch indicates which wave packet determines the observed result of a given experiment is called the "result assumption"); the other branches are designated "empty" and implicitly assumed by Bohm to be devoid of conscious observers. H. Dieter Zeh
H. Dieter Zeh
Heinz-Dieter Zeh , is a Professor Emeritus of the University of Heidelberg and theoretical physicist...
comments on these "empty" branches:
David Deutsch
David Deutsch
David Elieser Deutsch, FRS is an Israeli-British physicist at the University of Oxford. He is a non-stipendiary Visiting Professor in the Department of Atomic and Laser Physics at the Centre for Quantum Computation in the Clarendon Laboratory of the University of Oxford...
has expressed the same point more "acerbically":
According to Brown
Harvey Brown (philosopher)
Harvey R. Brown, is a philosopher of physics. He is professor of philosophy at the University of Oxford and a Fellow of Wolfson College, Oxford, as well as a Fellow of the British Academy....
& Wallace the de Broglie-Bohm particles play no role in the solution of the measurement problem. These authors claim that the "result assumption" (see above) is inconsistent with the view that there is no measurement problem in the predictable outcome (i.e. single-outcome) case. These authors also claim that a standard tacit assumption of the de Broglie-Bohm theory (that an observer becomes aware of configurations of particles of ordinary objects by means of correlations between such configurations and the configuration of the particles in the observer's brain) is unreasonable. This conclusion has been challenged by Valentini
Antony Valentini
Antony Valentini is a theoretical physicist and a professor at Clemson University. He is known for his work on the foundations of quantum physics.- Education and career :...
who argues that the entirety of such objections arises from a failure to interpret de Broglie-Bohm theory on its own terms.
According to Peter R. Holland
Peter R. Holland
Peter R. Holland is a theoretical physicist, known for his book on the pilot wave theory and the de Broglie-Bohm causal interpretation of quantum mechanics and his work on foundational problems in quantum physics....
, in a wider Hamiltonian framework, theories can be formulated in which particles do act back on the wave function.
Derivations
De Broglie–Bohm theory has been derived many times and in many ways. Below are five derivations all of which are very different and lead to different ways of understanding and extending this theory.- Schrödinger's equation can be derived by using Einstein's light quanta hypothesisPhotoelectric effectIn the photoelectric effect, electrons are emitted from matter as a consequence of their absorption of energy from electromagnetic radiation of very short wavelength, such as visible or ultraviolet light. Electrons emitted in this manner may be referred to as photoelectrons...
:
and de Broglie's hypothesis: 
.
- The guiding equation can be derived in a similar fashion. We assume a plane wave:
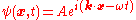. Notice that 
. Assuming that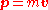 
for the particle's actual velocity, we have that 
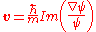. Thus, we have the guiding equation.
- Notice that this derivation does not use Schrödinger's equation.
- Preserving the density under the time evolution is another method of derivation. This is the method that Bell cites. It is this method which generalizes to many possible alternative theories. The starting point is the continuity equationContinuity equationA continuity equation in physics is a differential equation that describes the transport of a conserved quantity. Since mass, energy, momentum, electric charge and other natural quantities are conserved under their respective appropriate conditions, a variety of physical phenomena may be described...
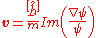for the density 
. This equation describes a probability flow along a current. We take the velocity field associated with this current as the velocity field whose integral curves yield the motion of the particle.
- A method applicable for particles without spin is to do a polar decomposition of the wavefunction and transform Schrödinger's equation into two coupled equations: the continuity equation from above and the Hamilton–Jacobi equation. This is the method used by Bohm in 1952. The decomposition and equations are as follows:
- Decomposition:
Note 
corresponds to the probability density 
.
- Continuity Equation:
- Hamilton–Jacobi Equation:
- The Hamilton–Jacobi equation is the equation derived from a Newtonian system with potential
and velocity field 
The potential 
is the classical potential that appears in Schrödinger's equation and the other term involving 
is the quantum potentialQuantum potentialThe quantum potential is a central concept of the de Broglie–Bohm formulation of quantum mechanics, introduced by David Bohm in 1952.Initially presented under the name quantum-mechanical potential, subsequently quantum potential, it was later elaborated upon by Bohm and Basil Hiley in its...
, terminology introduced by Bohm.
- This leads to viewing the quantum theory as particles moving under the classical force modified by a quantum force. However, unlike standard Newtonian mechanics, the initial velocity field is already specified by
which is a symptom of this being a first-order theory, not a second-order theory.
- A fourth derivation was given by Dürr et al. In their derivation, they derive the velocity field by demanding the appropriate transformation properties given by the various symmetries that Schrödinger's equation satisfies, once the wavefunction is suitably transformed. The guiding equation is what emerges from that analysis.
- A fifth derivation, given by Dürr et al. is appropriate for generalization to quantum field theory and the Dirac equation. The idea is that a velocity field can also be understood as a first order differential operator acting on functions. Thus, if we know how it acts on functions, we know what it is. Then given the Hamiltonian operator
, the equation to satisfy for all functions 
(with associated multiplication operator 
) is
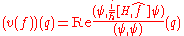where 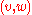is the local Hermitian inner product on the value space of the wavefunction.- This formulation allows for stochastic theories such as the creation and annihilation of particles.
- A further derivation has been given by Peter R. Holland, on which he bases the entire work presented in his quantum physics textbook The Quantum Theory of Motion, a main reference book on the de Broglie–Bohm theory. It is based on three basic postulates and an additional fourth postulate that links the wave function to measurement probabilities:
- 1. A physical system consists in a spatiotemporally propagating wave and a point particle guided by it;
- 2. The wave is described mathematically by a solution
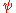to Schrödinger's wave equation; - 3. The particle motion is described by a solution to
in dependence on initial condition 
, with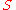 
the phase of 
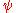. - The fourth postulate is subsidiary yet consistent with the first three:
- 4. The probability
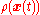to find the particle in the differential volume 
at time t equals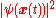 
.
History
De Broglie–Bohm theory has a history of different formulations and names. In this section, each stage is given a name and a main reference.Pilot-wave theory
Dr. de Broglie presented his pilot wave theory at the 1927 Solvay Conference, after close collaboration with Schrödinger, who developed his wave equation for de Broglie's theory. At the end of the presentation, Wolfgang PauliWolfgang Pauli
Wolfgang Ernst Pauli was an Austrian theoretical physicist and one of the pioneers of quantum physics. In 1945, after being nominated by Albert Einstein, he received the Nobel Prize in Physics for his "decisive contribution through his discovery of a new law of Nature, the exclusion principle or...
pointed out that it was not compatible with a semi-classical technique Fermi had previously adopted in the case of inelastic scattering. Contrary to a popular legend, de Broglie actually gave the correct rebuttal that the particular technique could not be generalized for Pauli's purpose, although the audience might have been lost in the technical details and de Broglie's mild mannerism left the impression that Pauli's objection was valid. He was eventually persuaded to abandon this theory nonetheless in 1932 due to both the Copenhagen school's more successful P.R. efforts and his own inability to understand quantum decoherence
Quantum decoherence
In quantum mechanics, quantum decoherence is the loss of coherence or ordering of the phase angles between the components of a system in a quantum superposition. A consequence of this dephasing leads to classical or probabilistically additive behavior...
. Also in 1932, John von Neumann
John von Neumann
John von Neumann was a Hungarian-American mathematician and polymath who made major contributions to a vast number of fields, including set theory, functional analysis, quantum mechanics, ergodic theory, geometry, fluid dynamics, economics and game theory, computer science, numerical analysis,...
published a paper, claiming to prove that all hidden-variable theories are impossible. This sealed the fate of de Broglie's theory for the next two decades. In truth, von Neumann's proof is based on invalid assumptions, such as quantum physics can be made local, and it does not really disprove the pilot-wave theory.
De Broglie's theory already applies to multiple spin-less particles, but lacks an adequate theory of measurement as no one understood quantum decoherence
Quantum decoherence
In quantum mechanics, quantum decoherence is the loss of coherence or ordering of the phase angles between the components of a system in a quantum superposition. A consequence of this dephasing leads to classical or probabilistically additive behavior...
at the time. An analysis of de Broglie's presentation is given in Bacciagaluppi et al.
Around this time Erwin Madelung
Erwin Madelung
Erwin Madelung was a German physicist.He was born in 1881 in Bonn. His father was the surgeon Otto Wilhelm Madelung. He earned a doctorate in 1905 from the University of Göttingen, specializing in crystal structure, and eventually became a professor...
also developed a hydrodynamic version of Schrödinger's equation which is incorrectly considered as a basis for the density current derivation of the de Broglie–Bohm theory. The Madelung equations
Madelung equations
The Madelung equations are Erwin Madelung's alternative formulation of the Schrödinger equation.-Equations:The Madelung equations are quantum Euler equations:\partial_t \rho+\nabla\cdot=0,...
, being quantum Euler equations (fluid dynamics), differ philosophically from the de Broglie–Bohm mechanics and are the basis of the hydrodynamic interpretation of quantum mechanics (quantum hydrodynamics
Quantum hydrodynamics
Quantum hydrodynamics is most generally the study of hydrodynamic systems which demonstrate behavior implicit in quantum subsystems . They arise in semiclassical mechanics in the study of semiconductor devices, in which case being derived from the Wigner-Boltzmann equation...
).
Peter R. Holland
Peter R. Holland
Peter R. Holland is a theoretical physicist, known for his book on the pilot wave theory and the de Broglie-Bohm causal interpretation of quantum mechanics and his work on foundational problems in quantum physics....
has pointed out that, in 1927, Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
had submitted a preprint with a related proposal but, not convinced, had withdrawn it before publication. According to Holland, failure to appreciate key points of the de Broglie–Bohm theory has led to confusion, the key point being “that the trajectories of a many-body quantum system are correlated not because the particles exert a direct force on one another (à la Coulomb) but because all are acted upon by an entity – mathematically described by the wavefunction or functions of it – that lies beyond them.” This entity is the quantum potential
Quantum potential
The quantum potential is a central concept of the de Broglie–Bohm formulation of quantum mechanics, introduced by David Bohm in 1952.Initially presented under the name quantum-mechanical potential, subsequently quantum potential, it was later elaborated upon by Bohm and Basil Hiley in its...
.
De Broglie–Bohm theory
After publishing a popular textbook on Quantum Mechanics which adhered entirely to the Copenhagen orthodoxy, Bohm was persuaded by Einstein to take a critical look at von Neumann's theorem. The result was 'A Suggested Interpretation of the Quantum Theory in Terms of "Hidden Variables" I and II' [Bohm 1952]. It extended the original Pilot Wave Theory to incorporate a consistent theory of measurement, and to address a criticism of Pauli that de Broglie did not properly respond to; it is taken to be deterministic (though Bohm hinted in the original papers that there should be disturbances to this, in the way Brownian motion disturbs Newtonian mechanics). This stage is known as the de Broglie–Bohm Theory in Bell's work [Bell 1987] and is the basis for 'The Quantum Theory of Motion' [Holland 1993].This stage applies to multiple particles, and is deterministic.
The de Broglie–Bohm theory is an example of a hidden variables theory. Bohm originally hoped that hidden variables could provide a local
Principle of locality
In physics, the principle of locality states that an object is influenced directly only by its immediate surroundings. Experiments have shown that quantum mechanically entangled particles must violate either the principle of locality or the form of philosophical realism known as counterfactual...
, causal, objective
Objectivity (philosophy)
Objectivity is a central philosophical concept which has been variously defined by sources. A proposition is generally considered to be objectively true when its truth conditions are met and are "mind-independent"—that is, not met by the judgment of a conscious entity or subject.- Objectivism...
description that would resolve or eliminate many of the paradoxes of quantum mechanics, such as Schrödinger's cat
Schrödinger's cat
Schrödinger's cat is a thought experiment, usually described as a paradox, devised by Austrian physicist Erwin Schrödinger in 1935. It illustrates what he saw as the problem of the Copenhagen interpretation of quantum mechanics applied to everyday objects. The scenario presents a cat that might be...
, the measurement problem
Measurement problem
The measurement problem in quantum mechanics is the unresolved problem of how wavefunction collapse occurs. The inability to observe this process directly has given rise to different interpretations of quantum mechanics, and poses a key set of questions that each interpretation must answer...
and the collapse of the wavefunction. However, Bell's theorem
Bell's theorem
In theoretical physics, Bell's theorem is a no-go theorem, loosely stating that:The theorem has great importance for physics and the philosophy of science, as it implies that quantum physics must necessarily violate either the principle of locality or counterfactual definiteness...
complicates this hope, as it demonstrates that there can be no local hidden variable theory that is compatible with the predictions of quantum mechanics. The Bohmian interpretation is causal but not local
Principle of locality
In physics, the principle of locality states that an object is influenced directly only by its immediate surroundings. Experiments have shown that quantum mechanically entangled particles must violate either the principle of locality or the form of philosophical realism known as counterfactual...
.
Bohm's paper was largely ignored by other physicists. Even Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
did not consider it a satisfactory answer to the quantum non-locality question. The rest of the contemporary objections, however, were ad hominem
Ad hominem
An ad hominem , short for argumentum ad hominem, is an attempt to negate the truth of a claim by pointing out a negative characteristic or belief of the person supporting it...
, focusing on Bohm's sympathy with liberals and supposed communists as exemplified by his refusal to give testimony to the House Un-American Activities Committee.
Eventually the cause was taken up by John Bell
John Stewart Bell
John Stewart Bell FRS was a British physicist from Northern Ireland , and the originator of Bell's theorem, a significant theorem in quantum physics regarding hidden variable theories.- Early life and work :...
. In "Speakable and Unspeakable in Quantum Mechanics" [Bell 1987], several of the papers refer to hidden variables theories (which include Bohm's). Bell showed that von Neumann's objection amounted to showing that hidden variables theories are nonlocal, and that nonlocality is a feature of all quantum mechanical systems.
Bohmian mechanics
This term is used to describe the same theory, but with an emphasis on the notion of current flow, which is determined on the basis of the quantum equilibrium hypothesis that the probability follows the Born ruleBorn rule
The Born rule is a law of quantum mechanics which gives the probability that a measurement on a quantum system will yield a given result. It is named after its originator, the physicist Max Born. The Born rule is one of the key principles of quantum mechanics...
. The term “Bohmian mechanics” is also often used to include most of the further extensions past the spin-less version of Bohm. While de Broglie–Bohm theory has Lagrangians and Hamilton-Jacobi equations as a primary focus and backdrop, with the icon of the quantum potential, Bohmian mechanics considers the continuity equation as primary and has the guiding equation as its icon. They are mathematically equivalent in so far as the Hamilton-Jacobi formulation applies, i.e., spin-less particles. The papers of Dürr et al. popularized the term.
All of non-relativistic quantum mechanics can be fully accounted for in this theory.
Causal interpretation and ontological interpretation
Bohm developed his original ideas, calling them the Causal Interpretation. Later he felt that causal sounded too much like deterministic and preferred to call his theory the Ontological Interpretation. The main reference is 'The Undivided Universe' [Bohm, Hiley 1993].This stage covers work by Bohm and in collaboration with Jean-Pierre Vigier
Jean-Pierre Vigier
Jean-Pierre Vigier earned his Ph.D. in Mathematics from University of Geneva in 1946 and in 1948 was appointed assistant to Louis de Broglie, a position he held until the latter's retirement in 1962. Vigier was professor emeritus at in the Department of Gravitational Physics at Pierre et Marie...
and Basil Hiley
Basil Hiley
Basil Hiley, born 1935, is a British quantum physicist and professor emeritus of the University of London.- Work :Hiley published a paper in 1961 on the random walk of a macromolecule, which was followed by further papers on the Ising model, and lattice constant systems defined in graph theoretical...
. Bohm is clear that this theory is non-deterministic (the work with Hiley includes a stochastic theory). As such, this theory is not, strictly speaking, a formulation of the de Broglie–Bohm theory. However, it deserves mention here because the term "Bohm Interpretation" is ambiguous between this theory and the de Broglie–Bohm theory.
See also
- David BohmDavid BohmDavid Joseph Bohm FRS was an American-born British quantum physicist who contributed to theoretical physics, philosophy, neuropsychology, and the Manhattan Project.-Youth and college:...
- Interpretation of quantum mechanicsInterpretation of quantum mechanicsAn interpretation of quantum mechanics is a set of statements which attempt to explain how quantum mechanics informs our understanding of nature. Although quantum mechanics has held up to rigorous and thorough experimental testing, many of these experiments are open to different interpretations...
- Madelung equationsMadelung equationsThe Madelung equations are Erwin Madelung's alternative formulation of the Schrödinger equation.-Equations:The Madelung equations are quantum Euler equations:\partial_t \rho+\nabla\cdot=0,...
- Local hidden variable theoryLocal hidden variable theoryIn quantum mechanics, a local hidden variable theory is one in which distant events are assumed to have no instantaneous effect on local ones....
- Quantum mechanicsQuantum mechanicsQuantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
- Pilot wavePilot waveIn theoretical physics, the Pilot Wave theory was the first known example of a hidden variable theory, presented by Louis de Broglie in 1927. Its more modern version, the Bohm interpretation,...
Literature
- Peter R. HollandPeter R. HollandPeter R. Holland is a theoretical physicist, known for his book on the pilot wave theory and the de Broglie-Bohm causal interpretation of quantum mechanics and his work on foundational problems in quantum physics....
: The quantum theory of motion, Cambridge University Press, 1993 (re-printed 2000, transferred to digital printing 2004), ISBN 0-521-48543-6
External links
- "Bohmian Mechanics" (Stanford Encyclopedia of Philosophy)
- "Pilot waves, Bohmian metaphysics, and the foundations of quantum mechanics", lecture course on de Broglie-Bohm theory by Mike Towler, Cambridge University.
- "21st-century directions in de Broglie-Bohm theory and beyond", August 2010 international conference on de Broglie-Bohm theory. Site contains slides for all the talks - the latest cutting-edge deBB research.
- "Observing the Trajectories of a Single Photon Using Weak Measurement"
- "Bohmian trajectories are no longer 'hidden variables'"