Classical field theory
Encyclopedia
A classical field theory is a physical theory that describes the study of how one or more physical fields
interact with matter. The word 'classical' is used in contrast to those field theories that incorporate quantum mechanics (quantum field theories
).
A physical field can be thought of as the assignment of a physical quantity
at each point of space
and time
. For example, in a weather forecast, the wind velocity during a day over a country is described by assigning a vector to each point in space. Each vector represents the direction of the movement of air at that point. As the day progresses, the directions in which the vectors point change as the directions of the wind change. From the mathematical viewpoint, classical fields are described by sections of fiber bundle
s (covariant classical field theory
). The term 'classical field theory' is commonly reserved for describing those physical theories that describe electromagnetism
and gravitation
, two of the fundamental forces of nature.
Descriptions of physical fields were given before the advent of relativity theory and then revised in light of this theory. Consequently, classical field theories are usually categorised as non-relativistic and relativistic.
lines of force when describing the electric field
. The gravitational field
was then similarly described.
es.
In a gravitational field, if a test particle
of gravitational mass m experiences a force F, then the gravitational field strength 'g' is defined by "g = F/m", where it is required that the test mass, m, be so small that its presence effectively does not disturb the gravitational field. Newton's law of gravitation says that two masses separated by a distance, r, experience a force
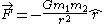
where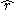
is a unit vector pointing away from the other object. Using Newton's 2nd law (for constant inertial mass), F=ma leads to a definition of the gravitational field strength due to a mass m as
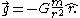
The experimental observation that inertial mass and gravitational mass are equal to unprecedented levels of accuracy leads to the identification of the gravitational field strength as identical to the acceleration experienced by a particle. This is the starting point of the equivalence principle
, which leads to general relativity
.
, E, so that F=qE. Using this and Coulomb's law
tells us that, we define the electric field due to a single charged particle as
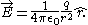
as this is now recognised as a fundamental aspect of nature. A field theory tends to be expressed mathematically by using Lagrangian
s. This is a function that, when subjected to an action principle, gives rise to the field equations and a conservation law
for the theory.
We use units where c=1 throughout.
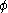, a scalar called the Lagrangian density
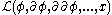can be constructed from 
and its derivatives.
From this density, the functional action can be constructed by integrating over spacetime
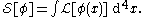
Then by enforcing the action principle
, the Euler-Lagrange equations are obtained
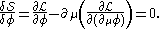
. Maxwell
's theory of electromagnetism
describes the interaction of charged matter with the electromagnetic field. The first formulation of this field theory used vector fields to describe the electric and magnetic fields. With the advent of special relativity, a better (and more consistent with mechanics) formulation using tensor
fields was found. Instead of using two vector fields describing the electric and magnetic fields, a tensor field representing these two fields together is used.
We have the electromagnetic potential,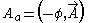
, and the electromagnetic four-current
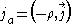. The electromagnetic field at any point in spacetime is described by the antisymmetric (0,2)-rank electromagnetic field tensor
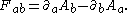
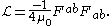We can use gauge field theory to get the interaction term, and this gives us
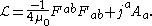
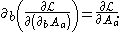
It is easy to see that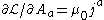
. The left hand side is trickier. Being careful with factors of 
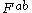, however, the calculation gives 
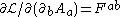. Together, then, the equations of motion are:
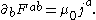
This gives us a vector equation, which are Maxwell's equations
in vacuum. The other two are obtained from the fact that F is the 4-curl of A:
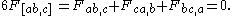
where the comma indicates a partial derivative
.
, Albert Einstein
formulated a new theory of gravitation called general relativity
. This treats gravitation
as a geometric phenomenon ('curved spacetime
') caused by masses and represents the gravitational field
mathematically by a tensor field
called the metric tensor
. The Einstein field equations
describe how this curvature is produced. The field equations may be derived by using the Einstein-Hilbert action
. Varying the Lagrangian
,
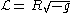where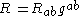
is the Ricci scalar written in terms of the Ricci tensor 
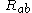and the metric tensor
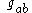, will yield the vacuum field equations:
,
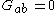where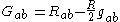
is the Einstein tensor
.
Field (physics)
In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...
interact with matter. The word 'classical' is used in contrast to those field theories that incorporate quantum mechanics (quantum field theories
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
).
A physical field can be thought of as the assignment of a physical quantity
Physical quantity
A physical quantity is a physical property of a phenomenon, body, or substance, that can be quantified by measurement.-Definition of a physical quantity:Formally, the International Vocabulary of Metrology, 3rd edition defines quantity as:...
at each point of space
Space
Space is the boundless, three-dimensional extent in which objects and events occur and have relative position and direction. Physical space is often conceived in three linear dimensions, although modern physicists usually consider it, with time, to be part of a boundless four-dimensional continuum...
and time
Time
Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
. For example, in a weather forecast, the wind velocity during a day over a country is described by assigning a vector to each point in space. Each vector represents the direction of the movement of air at that point. As the day progresses, the directions in which the vectors point change as the directions of the wind change. From the mathematical viewpoint, classical fields are described by sections of fiber bundle
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
s (covariant classical field theory
Covariant classical field theory
In recent years, there has been renewed interest in covariant classical field theory. Here, classical fields are represented by sections of fiber bundles and their dynamics is phrased in the context of a finite-dimensional space of fields. Nowadays, it is well known that jet bundles and the...
). The term 'classical field theory' is commonly reserved for describing those physical theories that describe electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
and gravitation
Gravitation
Gravitation, or gravity, is a natural phenomenon by which physical bodies attract with a force proportional to their mass. Gravitation is most familiar as the agent that gives weight to objects with mass and causes them to fall to the ground when dropped...
, two of the fundamental forces of nature.
Descriptions of physical fields were given before the advent of relativity theory and then revised in light of this theory. Consequently, classical field theories are usually categorised as non-relativistic and relativistic.
Non-relativistic field theories
Some of the simplest physical fields are vector force fields. Historically, the first time fields were taken seriously was with Faraday'sMichael Faraday
Michael Faraday, FRS was an English chemist and physicist who contributed to the fields of electromagnetism and electrochemistry....
lines of force when describing the electric field
Electric field
In physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
. The gravitational field
Gravitational field
The gravitational field is a model used in physics to explain the existence of gravity. In its original concept, gravity was a force between point masses...
was then similarly described.
Newtonian gravitation
A classical field theory describing gravity was Newtonian gravitation, which describes the gravitational force as a mutual interaction between two massMass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
es.
In a gravitational field, if a test particle
Test particle
In physical theories, a test particle is an idealized model of an object whose physical properties are assumed to be negligible except for the property being studied, which is considered to be insufficient to alter the behavior of the rest of the system...
of gravitational mass m experiences a force F, then the gravitational field strength 'g' is defined by "g = F/m", where it is required that the test mass, m, be so small that its presence effectively does not disturb the gravitational field. Newton's law of gravitation says that two masses separated by a distance, r, experience a force
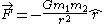
where
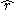is a unit vector pointing away from the other object. Using Newton's 2nd law (for constant inertial mass), F=ma leads to a definition of the gravitational field strength due to a mass m as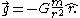The experimental observation that inertial mass and gravitational mass are equal to unprecedented levels of accuracy leads to the identification of the gravitational field strength as identical to the acceleration experienced by a particle. This is the starting point of the equivalence principle
Equivalence principle
In the physics of general relativity, the equivalence principle is any of several related concepts dealing with the equivalence of gravitational and inertial mass, and to Albert Einstein's assertion that the gravitational "force" as experienced locally while standing on a massive body is actually...
, which leads to general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
.
Electrostatics
A charged test particle, charge q, experiences a force, F, based solely on its charge. We can similarly describe the electric fieldElectric field
In physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
, E, so that F=qE. Using this and Coulomb's law
Coulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
tells us that, we define the electric field due to a single charged particle as
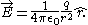
Relativistic field theory
Modern formulations of classical field theories generally require Lorentz covarianceLorentz covariance
In standard physics, Lorentz symmetry is "the feature of nature that says experimental results are independent of the orientation or the boost velocity of the laboratory through space"...
as this is now recognised as a fundamental aspect of nature. A field theory tends to be expressed mathematically by using Lagrangian
Lagrangian
The Lagrangian, L, of a dynamical system is a function that summarizes the dynamics of the system. It is named after Joseph Louis Lagrange. The concept of a Lagrangian was originally introduced in a reformulation of classical mechanics by Irish mathematician William Rowan Hamilton known as...
s. This is a function that, when subjected to an action principle, gives rise to the field equations and a conservation law
Conservation law
In physics, a conservation law states that a particular measurable property of an isolated physical system does not change as the system evolves....
for the theory.
We use units where c=1 throughout.
Lagrangian dynamics
Given a field tensor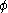, a scalar called the Lagrangian densityLagrangian
The Lagrangian, L, of a dynamical system is a function that summarizes the dynamics of the system. It is named after Joseph Louis Lagrange. The concept of a Lagrangian was originally introduced in a reformulation of classical mechanics by Irish mathematician William Rowan Hamilton known as...
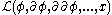can be constructed from and its derivatives.From this density, the functional action can be constructed by integrating over spacetime
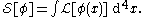
Then by enforcing the action principle
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
, the Euler-Lagrange equations are obtained
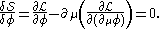
Relativistic fields
Two of the most well-known Lorentz covariant classical field theories are now described.Electromagnetism
Historically, the first (classical) field theories were those describing the electric and magnetic fields (separately). After numerous experiments, it was found that these two fields were related, or, in fact, two aspects of the same field: the electromagnetic fieldElectromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
. Maxwell
James Clerk Maxwell
James Clerk Maxwell of Glenlair was a Scottish physicist and mathematician. His most prominent achievement was formulating classical electromagnetic theory. This united all previously unrelated observations, experiments and equations of electricity, magnetism and optics into a consistent theory...
's theory of electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
describes the interaction of charged matter with the electromagnetic field. The first formulation of this field theory used vector fields to describe the electric and magnetic fields. With the advent of special relativity, a better (and more consistent with mechanics) formulation using tensor
Tensor
Tensors are geometric objects that describe linear relations between vectors, scalars, and other tensors. Elementary examples include the dot product, the cross product, and linear maps. Vectors and scalars themselves are also tensors. A tensor can be represented as a multi-dimensional array of...
fields was found. Instead of using two vector fields describing the electric and magnetic fields, a tensor field representing these two fields together is used.
We have the electromagnetic potential,
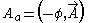, and the electromagnetic four-currentFour-current
In special and general relativity, the four-current is the Lorentz covariant four-vector that replaces the electromagnetic current density, or indeed any conventional charge current density...
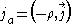. The electromagnetic field at any point in spacetime is described by the antisymmetric (0,2)-rank electromagnetic field tensor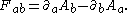The Lagrangian
To obtain the dynamics for this field, we try and construct a scalar from the field. In the vacuum, we have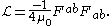 We can use gauge field theory to get the interaction term, and this gives us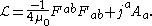The Equations
This, coupled with the Euler-Lagrange equations, gives us the desired result, since the E-L equations say that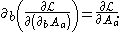It is easy to see that
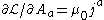. The left hand side is trickier. Being careful with factors of 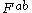, however, the calculation gives 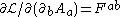. Together, then, the equations of motion are: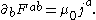This gives us a vector equation, which are Maxwell's equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
in vacuum. The other two are obtained from the fact that F is the 4-curl of A:
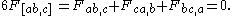
where the comma indicates a partial derivative
Partial derivative
In mathematics, a partial derivative of a function of several variables is its derivative with respect to one of those variables, with the others held constant...
.
Gravitation
After Newtonian gravitation was found to be inconsistent with special relativitySpecial relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
, Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
formulated a new theory of gravitation called general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
. This treats gravitation
Gravitation
Gravitation, or gravity, is a natural phenomenon by which physical bodies attract with a force proportional to their mass. Gravitation is most familiar as the agent that gives weight to objects with mass and causes them to fall to the ground when dropped...
as a geometric phenomenon ('curved spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
') caused by masses and represents the gravitational field
Gravitational field
The gravitational field is a model used in physics to explain the existence of gravity. In its original concept, gravity was a force between point masses...
mathematically by a tensor field
Tensor field
In mathematics, physics and engineering, a tensor field assigns a tensor to each point of a mathematical space . Tensor fields are used in differential geometry, algebraic geometry, general relativity, in the analysis of stress and strain in materials, and in numerous applications in the physical...
called the metric tensor
Metric tensor (general relativity)
In general relativity, the metric tensor is the fundamental object of study. It may loosely be thought of as a generalization of the gravitational field familiar from Newtonian gravitation...
. The Einstein field equations
Einstein field equations
The Einstein field equations or Einstein's equations are a set of ten equations in Albert Einstein's general theory of relativity which describe the fundamental interaction of gravitation as a result of spacetime being curved by matter and energy...
describe how this curvature is produced. The field equations may be derived by using the Einstein-Hilbert action
Einstein-Hilbert action
The Einstein–Hilbert action in general relativity is the action that yields the Einstein's field equations through the principle of least action...
. Varying the Lagrangian
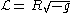,where
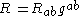is the Ricci scalar written in terms of the Ricci tensor 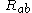and the metric tensorMetric tensor (general relativity)
In general relativity, the metric tensor is the fundamental object of study. It may loosely be thought of as a generalization of the gravitational field familiar from Newtonian gravitation...
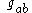, will yield the vacuum field equations: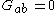,where
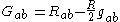is the Einstein tensorEinstein tensor
In differential geometry, the Einstein tensor , named after Albert Einstein, is used to express the curvature of a Riemannian manifold...
.
See also
- Covariant classical field theoryCovariant classical field theoryIn recent years, there has been renewed interest in covariant classical field theory. Here, classical fields are represented by sections of fiber bundles and their dynamics is phrased in the context of a finite-dimensional space of fields. Nowadays, it is well known that jet bundles and the...
- ElectromagnetismElectromagnetismElectromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
- Field (physics)Field (physics)In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...
- General relativityGeneral relativityGeneral relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
- Quantum field theoryQuantum field theoryQuantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
- Variational methods in general relativityVariational methods in general relativityVariational methods in general relativity refers to various mathematical techniques that employ the use of variational calculus in Einstein's theory of general relativity...
External links
- Giachetta, G., Mangiarotti, L., Sardanashvily, G.Gennadi SardanashvilyGennadi Sardanashvily is a theoretical physicist, a principal research scientist of Moscow State University.- Biography :...
, "Advanced Classical Field Theory", World Scientific, 2009, ISBN 9789812838957 )