Compact closed category
Encyclopedia
In category theory
, compact closed categories are a general context for treating dual object
s. The idea of a dual object generalizes the more familiar concept of the dual
of a finite-dimensional vector space
. So, the motivating example of a compact closed category is FdVect, the category with finite dimensional vector spaces as objects and linear maps as morphisms.
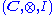is compact closed if every object 
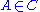has a
dual object
. If this holds, the dual object is unique up to canonical isomorphism, and it is denoted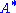
.
In a bit more detail, an object
is called the dual
of A if it is equipped with two morphisms called the unit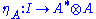
and the counit 
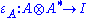, satisfying the equations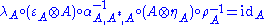
and
.
For clarity, we rewrite the above compositions diagramatically:
and
.
Compact closed categories are precisely the symmetric autonomous categories
. They are also *-autonomous
.
Every compact closed category C admits a trace. Namely, for every morphism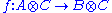
, one can define
which can be shown to be a proper trace.
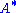is the usual dual of the vector space 
.
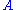The category of finite-dimensional representations of any group is also compact closed.
The category Vect, with all vector spaces as objects and linear maps as morphisms, is not compact closed.
Category theory
Category theory is an area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows , where these collections satisfy certain basic conditions...
, compact closed categories are a general context for treating dual object
Dual object
In category theory, a branch of mathematics, it is possible to define a concept of dual object generalizing the concept of dual space in linear algebra.A category in which each object has a dual is called autonomous or rigid.-Definition:...
s. The idea of a dual object generalizes the more familiar concept of the dual
Dual space
In mathematics, any vector space, V, has a corresponding dual vector space consisting of all linear functionals on V. Dual vector spaces defined on finite-dimensional vector spaces can be used for defining tensors which are studied in tensor algebra...
of a finite-dimensional vector space
Vector space
A vector space is a mathematical structure formed by a collection of vectors: objects that may be added together and multiplied by numbers, called scalars in this context. Scalars are often taken to be real numbers, but one may also consider vector spaces with scalar multiplication by complex...
. So, the motivating example of a compact closed category is FdVect, the category with finite dimensional vector spaces as objects and linear maps as morphisms.
Definition
A symmetric monoidal category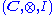 is compact closed if every object 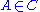has adual object
Dual object
In category theory, a branch of mathematics, it is possible to define a concept of dual object generalizing the concept of dual space in linear algebra.A category in which each object has a dual is called autonomous or rigid.-Definition:...
. If this holds, the dual object is unique up to canonical isomorphism, and it is denoted
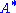.In a bit more detail, an object
is called the dualDual object
In category theory, a branch of mathematics, it is possible to define a concept of dual object generalizing the concept of dual space in linear algebra.A category in which each object has a dual is called autonomous or rigid.-Definition:...
of A if it is equipped with two morphisms called the unit
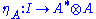and the counit 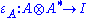, satisfying the equations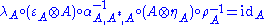and
.For clarity, we rewrite the above compositions diagramatically:
and
Properties
Compact closed categories are a special case of monoidal closed categories, which in turn are a special case of closed categoriesClosed category
In category theory, a branch of mathematics, a closed category is a special kind of category.In any category , the morphisms between any two given objects x and y comprise a set, the external hom...
.
Compact closed categories are precisely the symmetric autonomous categories
Autonomous category
In mathematics, an autonomous category is a monoidal category where dual objects exist.-Definition:A left autonomous category is a monoidal category where every object has a left dual. An autonomous category is a monoidal category where every object has both a left and a right dual...
. They are also *-autonomous
*-autonomous category
In mathematics, a *-autonomous category C is a symmetric monoidal closed category equipped with a dualizing object \bot.-Definition:Let C be a symmetric monoidal closed category...
.
Every compact closed category C admits a trace. Namely, for every morphism
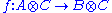, one can definewhich can be shown to be a proper trace.
Examples
The canonical example is the category FdVect with finite dimensional vector spaces as objects and linear maps as morphisms. Here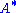 is the usual dual of the vector space 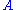.The category of finite-dimensional representations of any group is also compact closed.
The category Vect, with all vector spaces as objects and linear maps as morphisms, is not compact closed.