Compressible flow
Encyclopedia
Compressible flow is the area of fluid mechanics
that deals with fluids
in which the fluid density
varies significantly in response to a change in pressure
. Compressibility effects are typically considered significant if the Mach number
(the ratio of the flow velocity to the local speed of sound
) of the flow exceeds 0.3, or if the fluid undergoes very large pressure changes. The most distinct differences between the compressible and incompressible
flow models are that the compressible flow model allows for the existence of shock wave
s and choked flow
.
. However, in the many cases dealing with gas
es (especially at higher velocities) and those cases dealing with liquid
s with large pressure changes, the significant variations in density can occur, and the flow should be analysed as a compressible flow if accurate results are to be obtained.
Allowing for a change in density brings an additional variable into the analysis. In contrast to incompressible flows, which can usually be solved by considering only the equations from conservation of mass
and conservation of momentum. Usually, the principle of conservation of energy
is included. However, this introduces another variable (temperature
), and so a fourth equation (such as the ideal gas equation) is required to relate the temperature to the other thermodynamic properties in order to fully describe the flow.
When defining what is meant by a compressible flow, it is useful to compare the density to a reference value, such as the stagnation density,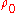
, which is the density of the fluid if it were to be slowed down isentropically
to stationary. As a general rule of thumb, if the change in density relative to the stagnation density is greater than 5%, then the fluid should be analysed as a compressible flow. For an ideal gas
with a ratio of specific heats
of 1.4, this occurs at a Mach number greater than approximately 0.3. Below this value, however, whether or not a specific case should be treated as compressible or incompressible depends largely on the level of accuracy that is required .
(see Internal Flows) and the presence of acoustic wave
s, which may also be referred to as either compression or expansion waves, depending on whether they lead to an increase or decrease in pressure.
are one of the most common examples of compressible flow phenomena. A shock is characterised by a discontinuous change in the thermodynamic properties. In one dimensional flows, shock waves can form when a series of compression waves coalesce, or when a membrane separating two regions of differing pressure is suddenly removed. This is the technique often used to produce shock waves in shock tubes (see Shock Tubes).
In two and three dimensional supersonic flows, oblique shock waves
occur as a result of a change in direction of the flow. A classic example of these shock waves are those shock waves that form off the nose of a supersonic aircraft.
is a subfield of fluid dynamics
and gas dynamics
, and is primarily concerned with obtaining the forces that air exerts on an object. For Mach numbers greater than about 0.3, density changes are significant, and the flow should be considered compressible for an accurate representation of reality.
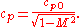
where
This correction factor works well for all Mach numbers between 0.3 and 0.7.
It should be noted that since this correction factor is derived from linearized equations, the pressures calculated is always less in magnitude than the actual pressures within the fluid.
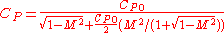
where
This correction factor is valid for M<0.8.
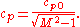
where
This is considered a valid approximation for Mach numbers greater than 1.3.
and the Whitcomb area rule
.
. This includes the flow of fluids through pipes and ducts, and often arise in industrial and manufacturing processes, and is vital in the analysis of propulsion systems.
One example is in die casting
or injection molding
processes. This involves injecting a liquid material (such as a thermosetting plastic for injection molding or molten metal for die casting) at very high pressures into a cavity. The air that is already in the cavity is displaced very rapidly, and compressibility needs to be considered in the design of the die if problems with air entrapment are to be avoided.
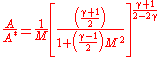
where
and 
are the area and Mach number at a point in the nozzle,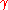 
is the ratio of specific heats, and 
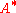is the area that would cause the flow velocity to reach a Mach number of 1 (i.e. the area at the throat, provided that the nozzle is choked).
Thus, for a subsonic flow to be accelerated to supersonic velocities, the nozzle needs to have a converging section in which the flow is subsonic, a throat, at which the flow velocity is the local speed of sound, and a diverging section with supersonic flow. Such an arrangement is called a de Laval nozzle
, and is commonly used in propulsion systems such as rocket
and supersonic jet engines
.
Note that Mach 1 can be a very high speed for a hot gas, since the speed of sound varies as the square root of absolute temperature. Thus the speed reached at a nozzle throat can be far higher than the speed of sound under standard atmospheric conditions. This fact is used extensively in rocketry where hypersonic flows are required, and where propellant mixtures are deliberately chosen to further increase the sonic speed.
has a similar effect as an area change on compressible flow. In a pipe of constant cross sectional area in which the walls exert a frictional force on the flow, the flow velocity will tend toward the speed of sound. In other words, subsonic flow through a pipe with friction will accelerate, and supersonic flow will decelerate. If the pipe length is long enough that the flow velocity would pass through unity, then the flow chokes such that the flow exiting the pipe is at Mach 1. As with the nozzle, this is achieved either through the flow rate at the inlet being limited, or the formation of shock waves in the pipe (for supersonic flows). For the adiabatic
flow of an ideal gas model, the effects of friction may be calculated using the Fanno flow
model. For a constant friction factor, the model is given by
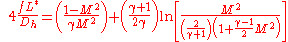
where
is the Fanning friction factor,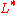 
is the required pipe length passed the point being considered that would result in the flow choking, and 
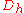is the hydraulic diameter of the pipe.
For an ideal gas in a constant area pipe, the effect of heat addition to the pipe may be calculated using the Rayleigh flow
model, which describes how the Mach number varies with changes in the stagnation temperature
. The stagnation temperature at a point is the temperature that the fluid would reach if it were to be slowed isentropically to stationary. As heat is added to the system, the stagnation temperature increases. The Rayleigh flow model is given by
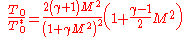
where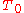
and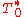 
represent the stagnation temperatures at the point under consideration, and at the point at which the Mach number is 1 respectively.
have been used to measure dissociation energies and molecular relaxation rates, investigate shock wave behaviour, and they have been used in aerodynamic tests. The fluid flow in the driven gas (the gas behind the shock wave) can be used much as a wind tunnel, allowing higher temperatures and pressures replicating the conditions in the turbine sections of jet engines. However, test times are limited to a few milliseconds, either by the arrival of the contact surface or the reflected shock wave.
They have been further developed into shock tunnels, with an added nozzle and dump tank. The resultant high temperature hypersonic flow can be used to simulate atmospheric re-entry of spacecraft or hypersonic craft, again with limited testing times.
Fluid mechanics
Fluid mechanics is the study of fluids and the forces on them. Fluid mechanics can be divided into fluid statics, the study of fluids at rest; fluid kinematics, the study of fluids in motion; and fluid dynamics, the study of the effect of forces on fluid motion...
that deals with fluids
Fluid
In physics, a fluid is a substance that continually deforms under an applied shear stress. Fluids are a subset of the phases of matter and include liquids, gases, plasmas and, to some extent, plastic solids....
in which the fluid density
Density
The mass density or density of a material is defined as its mass per unit volume. The symbol most often used for density is ρ . In some cases , density is also defined as its weight per unit volume; although, this quantity is more properly called specific weight...
varies significantly in response to a change in pressure
Pressure
Pressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
. Compressibility effects are typically considered significant if the Mach number
Mach number
Mach number is the speed of an object moving through air, or any other fluid substance, divided by the speed of sound as it is in that substance for its particular physical conditions, including those of temperature and pressure...
(the ratio of the flow velocity to the local speed of sound
Speed of sound
The speed of sound is the distance travelled during a unit of time by a sound wave propagating through an elastic medium. In dry air at , the speed of sound is . This is , or about one kilometer in three seconds or approximately one mile in five seconds....
) of the flow exceeds 0.3, or if the fluid undergoes very large pressure changes. The most distinct differences between the compressible and incompressible
Incompressible flow
In fluid mechanics or more generally continuum mechanics, incompressible flow refers to flow in which the material density is constant within an infinitesimal volume that moves with the velocity of the fluid...
flow models are that the compressible flow model allows for the existence of shock wave
Shock wave
A shock wave is a type of propagating disturbance. Like an ordinary wave, it carries energy and can propagate through a medium or in some cases in the absence of a material medium, through a field such as the electromagnetic field...
s and choked flow
Choked flow
Choked flow is a compressible flow effect. The parameter that becomes "choked" or "limited" is the velocity or the mass flow rate.Choked flow is a fluid dynamic condition associated with the Venturi effect...
.
Definition
Compressible flow describes the behaviour of fluids that experience significant variations in density. For flows in which the density does not vary significantly, the analysis of the behaviour of such flows may be simplified greatly by assuming a constant density. This is an idealization, which leads to the theory of incompressible flowIncompressible flow
In fluid mechanics or more generally continuum mechanics, incompressible flow refers to flow in which the material density is constant within an infinitesimal volume that moves with the velocity of the fluid...
. However, in the many cases dealing with gas
Gas
Gas is one of the three classical states of matter . Near absolute zero, a substance exists as a solid. As heat is added to this substance it melts into a liquid at its melting point , boils into a gas at its boiling point, and if heated high enough would enter a plasma state in which the electrons...
es (especially at higher velocities) and those cases dealing with liquid
Liquid
Liquid is one of the three classical states of matter . Like a gas, a liquid is able to flow and take the shape of a container. Some liquids resist compression, while others can be compressed. Unlike a gas, a liquid does not disperse to fill every space of a container, and maintains a fairly...
s with large pressure changes, the significant variations in density can occur, and the flow should be analysed as a compressible flow if accurate results are to be obtained.
Allowing for a change in density brings an additional variable into the analysis. In contrast to incompressible flows, which can usually be solved by considering only the equations from conservation of mass
Conservation of mass
The law of conservation of mass, also known as the principle of mass/matter conservation, states that the mass of an isolated system will remain constant over time...
and conservation of momentum. Usually, the principle of conservation of energy
Conservation of energy
The nineteenth century law of conservation of energy is a law of physics. It states that the total amount of energy in an isolated system remains constant over time. The total energy is said to be conserved over time...
is included. However, this introduces another variable (temperature
Temperature
Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
), and so a fourth equation (such as the ideal gas equation) is required to relate the temperature to the other thermodynamic properties in order to fully describe the flow.
When defining what is meant by a compressible flow, it is useful to compare the density to a reference value, such as the stagnation density,
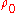, which is the density of the fluid if it were to be slowed down isentropicallyIsentropic process
In thermodynamics, an isentropic process or isoentropic process is one in which for purposes of engineering analysis and calculation, one may assume that the process takes place from initiation to completion without an increase or decrease in the entropy of the system, i.e., the entropy of the...
to stationary. As a general rule of thumb, if the change in density relative to the stagnation density is greater than 5%, then the fluid should be analysed as a compressible flow. For an ideal gas
Ideal gas
An ideal gas is a theoretical gas composed of a set of randomly-moving, non-interacting point particles. The ideal gas concept is useful because it obeys the ideal gas law, a simplified equation of state, and is amenable to analysis under statistical mechanics.At normal conditions such as...
with a ratio of specific heats
Heat capacity ratio
The heat capacity ratio or adiabatic index or ratio of specific heats, is the ratio of the heat capacity at constant pressure to heat capacity at constant volume . It is sometimes also known as the isentropic expansion factor and is denoted by \gamma or \kappa . The latter symbol kappa is...
of 1.4, this occurs at a Mach number greater than approximately 0.3. Below this value, however, whether or not a specific case should be treated as compressible or incompressible depends largely on the level of accuracy that is required .
Compressible Flow Phenomena
Two of the most distinctive phenomena which occur in compressible are the possibility of choked flowChoked flow
Choked flow is a compressible flow effect. The parameter that becomes "choked" or "limited" is the velocity or the mass flow rate.Choked flow is a fluid dynamic condition associated with the Venturi effect...
(see Internal Flows) and the presence of acoustic wave
Acoustic wave
Acoustic waves are a type of longitudinal waves that propagate by means of adiabatic compression and decompression. Longitudinal waves are waves that have the same direction of vibration as their direction of travel. Important quantities for describing acoustic waves are sound pressure, particle...
s, which may also be referred to as either compression or expansion waves, depending on whether they lead to an increase or decrease in pressure.
Shock Waves
Shock wavesShock wave
A shock wave is a type of propagating disturbance. Like an ordinary wave, it carries energy and can propagate through a medium or in some cases in the absence of a material medium, through a field such as the electromagnetic field...
are one of the most common examples of compressible flow phenomena. A shock is characterised by a discontinuous change in the thermodynamic properties. In one dimensional flows, shock waves can form when a series of compression waves coalesce, or when a membrane separating two regions of differing pressure is suddenly removed. This is the technique often used to produce shock waves in shock tubes (see Shock Tubes).
In two and three dimensional supersonic flows, oblique shock waves
Oblique shock
An oblique shock wave, unlike a normal shock, is inclined with respect to the incident upstream flow direction. It will occur when a supersonic flow encounters a corner that effectively turns the flow into itself and compresses. The upstream streamlines are uniformly deflected after the shock wave...
occur as a result of a change in direction of the flow. A classic example of these shock waves are those shock waves that form off the nose of a supersonic aircraft.
Aerodynamics
AerodynamicsAerodynamics
Aerodynamics is a branch of dynamics concerned with studying the motion of air, particularly when it interacts with a moving object. Aerodynamics is a subfield of fluid dynamics and gas dynamics, with much theory shared between them. Aerodynamics is often used synonymously with gas dynamics, with...
is a subfield of fluid dynamics
Fluid dynamics
In physics, fluid dynamics is a sub-discipline of fluid mechanics that deals with fluid flow—the natural science of fluids in motion. It has several subdisciplines itself, including aerodynamics and hydrodynamics...
and gas dynamics
Gas dynamics
Gas dynamics is a branch of fluid dynamics concerned with studying the motion of gases and its consequent effects. Gas dynamics combines the principles of fluid mechanics and thermodynamics...
, and is primarily concerned with obtaining the forces that air exerts on an object. For Mach numbers greater than about 0.3, density changes are significant, and the flow should be considered compressible for an accurate representation of reality.
Subsonic Aerodynamics
Due to the complexities of compressible flow theory, it is often easier to calculate the incompressible flow characteristics first, and then employ a correction factor to obtain the actual flow properties. Several correction factors exist with varying degrees of complexity and accuracy.Prandtl–Glauert transformation
The Prandtl-Glauert transformation is found by linearizing the potential equations associated with compressible, inviscid flow. The Prandtl–Glauert transformation or Prandtl–Glauert rule (also Prandtl–Glauert–Ackeret rule) is an approximation function which allows comparison of aerodynamical processes occurring at different Mach numbers. It was discovered that the linearized pressures in such a flow were equal to those found from incompressible flow theory multiplied by a correction factor. This correction factor is given below. :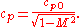where
- cp is the compressible pressure coefficient
- cp0 is the incompressible pressure coefficient
- M is the Mach number.
This correction factor works well for all Mach numbers between 0.3 and 0.7.
It should be noted that since this correction factor is derived from linearized equations, the pressures calculated is always less in magnitude than the actual pressures within the fluid.
Karman-Tsien correction factor
The Karman-Tsien transformation is a nonlinear correction factor to find the pressure coefficient of a compressible, inviscid flow. It is an empirically derived correction factor that tends to slightly overestimate the magnitude of the fluid's pressure. In order to employ this correction factor, the incompressible, inviscid fluid pressure must be known from previous investigation.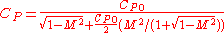where
- cp is the compressible pressure coefficient
- cp0 is the incompressible pressure coefficient
- M is the Mach number.
This correction factor is valid for M<0.8.
Supersonic Aerodynamics
As with subsonic aerodynamics, a compressibility correction factor can be derived by linearising the governing equations. The supersonic correction factor is similar to the Prandtl-Glauert transformation, but the terms under the square root sign are reversed.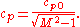where
- cp is the compressible pressure coefficient
- cp0 is the incompressible pressure coefficient
- M is the Mach number.
This is considered a valid approximation for Mach numbers greater than 1.3.
Transonic Aerodynamics
Transonic flow typically occurs in flows with Mach numbers between 0.8 and 1.2. Under these conditions, some of the flow is supersonic and some is subsonic. At these velocities, the correction factors derived using linearized theory breaks down due to a singularity that occurs at a Mach number of 1. In addition, severe instabilities caused by the formation of local shock waves and the existence of both subsonic and supersonic flow (which behave completely differently) makes the solution of the governing equations rather difficult. However, the analysis of compressible flows in the transonic regime has led to some developments which help reduce the increases in drag caused by compressibility effects, including the use of swept wingsSwept wing
A swept wing is a wing planform favored for high subsonic jet speeds first investigated by Germany during the Second World War. Since the introduction of the MiG-15 and North American F-86 which demonstrated a decisive superiority over the slower first generation of straight-wing jet fighters...
and the Whitcomb area rule
Area rule
The Whitcomb area rule, also called the transonic area rule, is a design technique used to reduce an aircraft's drag at transonic and supersonic speeds, particularly between Mach 0.75 and 1.2....
.
Internal Flows
If the flow of a fluid the fluid is confined by a surface, it is referred to as an internal flowInternal flow
In fluid mechanics, internal flow is a flow for which the fluid is confined by a surface. Hence the boundary layer is unable to develop without eventually being constrained...
. This includes the flow of fluids through pipes and ducts, and often arise in industrial and manufacturing processes, and is vital in the analysis of propulsion systems.
One example is in die casting
Die casting
Die casting is a metal casting process that is characterized by forcing molten metal under high pressure into a mold cavity. The mold cavity is created using two hardened tool steel dies which have been machined into shape and work similarly to an injection mold during the process...
or injection molding
Injection molding
Injection molding is a manufacturing process for producing parts from both thermoplastic and thermosetting plastic materials. Material is fed into a heated barrel, mixed, and forced into a mold cavity where it cools and hardens to the configuration of the cavity...
processes. This involves injecting a liquid material (such as a thermosetting plastic for injection molding or molten metal for die casting) at very high pressures into a cavity. The air that is already in the cavity is displaced very rapidly, and compressibility needs to be considered in the design of the die if problems with air entrapment are to be avoided.
Effect of area changes
Compressible flows play a big role in determining the behaviour of nozzles. Subsonic and supersonic flow react differently to changes in cross sectional area. While subsonic flow flowing through a converging duct (narrowing down from a wide diameter to a smaller diameter in the direction of the flow) will experience an increase in velocity, a supersonic flow through an identical duct will experience a decrease in velocity. In general, flow through a converging nozzle will always tend towards Mach 1. If the area convergence is great enough that the sound speed is reached, a phenomenon known as "choking" occurs. In this case, the flow is choked, and either the flow rate of the fluid entering the pipe is limited, or shock waves form in the nozzle such that the Mach number at the point of minimum area (called the throat) remains unity. Similarly, subsonic flow through a diverging nozzle will always be slowed, and supersonic flow will accelerate. The Mach number of the flow can be directly related to the area by the relation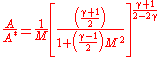where
and are the area and Mach number at a point in the nozzle, 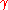is the ratio of specific heats, and 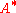is the area that would cause the flow velocity to reach a Mach number of 1 (i.e. the area at the throat, provided that the nozzle is choked).Thus, for a subsonic flow to be accelerated to supersonic velocities, the nozzle needs to have a converging section in which the flow is subsonic, a throat, at which the flow velocity is the local speed of sound, and a diverging section with supersonic flow. Such an arrangement is called a de Laval nozzle
De Laval nozzle
A de Laval nozzle is a tube that is pinched in the middle, making a carefully balanced, asymmetric hourglass-shape...
, and is commonly used in propulsion systems such as rocket
Rocket engine
A rocket engine, or simply "rocket", is a jet engineRocket Propulsion Elements; 7th edition- chapter 1 that uses only propellant mass for forming its high speed propulsive jet. Rocket engines are reaction engines and obtain thrust in accordance with Newton's third law...
and supersonic jet engines
Jet engine
A jet engine is a reaction engine that discharges a fast moving jet to generate thrust by jet propulsion and in accordance with Newton's laws of motion. This broad definition of jet engines includes turbojets, turbofans, rockets, ramjets, pulse jets...
.
Note that Mach 1 can be a very high speed for a hot gas, since the speed of sound varies as the square root of absolute temperature. Thus the speed reached at a nozzle throat can be far higher than the speed of sound under standard atmospheric conditions. This fact is used extensively in rocketry where hypersonic flows are required, and where propellant mixtures are deliberately chosen to further increase the sonic speed.
Effect of friction
FrictionFriction
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and/or material elements sliding against each other. There are several types of friction:...
has a similar effect as an area change on compressible flow. In a pipe of constant cross sectional area in which the walls exert a frictional force on the flow, the flow velocity will tend toward the speed of sound. In other words, subsonic flow through a pipe with friction will accelerate, and supersonic flow will decelerate. If the pipe length is long enough that the flow velocity would pass through unity, then the flow chokes such that the flow exiting the pipe is at Mach 1. As with the nozzle, this is achieved either through the flow rate at the inlet being limited, or the formation of shock waves in the pipe (for supersonic flows). For the adiabatic
Adiabatic process
In thermodynamics, an adiabatic process or an isocaloric process is a thermodynamic process in which the net heat transfer to or from the working fluid is zero. Such a process can occur if the container of the system has thermally-insulated walls or the process happens in an extremely short time,...
flow of an ideal gas model, the effects of friction may be calculated using the Fanno flow
Fanno flow
Fanno flow refers to adiabatic flow through a constant area duct where the effect of friction is considered. Compressibility effects often come into consideration, although the Fanno flow model certainly also applies to incompressible flow. For this model, the duct area remains constant, the flow...
model. For a constant friction factor, the model is given by
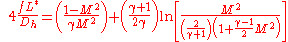
where
is the Fanning friction factor, 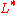is the required pipe length passed the point being considered that would result in the flow choking, and 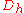is the hydraulic diameter of the pipe.Effect of heat transfer
Adding heat to a fluid flowing at subsonic velocities in a pipe will cause the flow to accelerate, and adding heat to supersonic flow in a pipe will cause the flow to decelerate. As with the cases of friction and area change discussed above, adding more heat than that required to reach a Mach number of 1 will result in the flow choking.For an ideal gas in a constant area pipe, the effect of heat addition to the pipe may be calculated using the Rayleigh flow
Rayleigh flow
Rayleigh flow refers to diabatic flow through a constant area duct where the effect of heat addition or rejection is considered. Compressibility effects often come into consideration, although the Rayleigh flow model certainly also applies to incompressible flow. For this model, the duct area...
model, which describes how the Mach number varies with changes in the stagnation temperature
Stagnation temperature
In thermodynamics and fluid mechanics, stagnation temperature is the temperature at a stagnation point in a fluid flow. At a stagnation point the speed of the fluid is zero and all of the kinetic energy has been converted to internal energy and is added to the local static enthalpy...
. The stagnation temperature at a point is the temperature that the fluid would reach if it were to be slowed isentropically to stationary. As heat is added to the system, the stagnation temperature increases. The Rayleigh flow model is given by
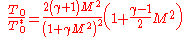
where
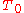and 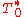represent the stagnation temperatures at the point under consideration, and at the point at which the Mach number is 1 respectively.Shock Tubes
In addition to measurements of rates of chemical kinetics, shock tubesShock tube
For the pyrotechnic initiator, see Shock tube detonatorThe shock tube is an instrument used to replicate and direct blast waves at a sensor or a model in order to simulate actual explosions and their effects, usually on a smaller scale...
have been used to measure dissociation energies and molecular relaxation rates, investigate shock wave behaviour, and they have been used in aerodynamic tests. The fluid flow in the driven gas (the gas behind the shock wave) can be used much as a wind tunnel, allowing higher temperatures and pressures replicating the conditions in the turbine sections of jet engines. However, test times are limited to a few milliseconds, either by the arrival of the contact surface or the reflected shock wave.
They have been further developed into shock tunnels, with an added nozzle and dump tank. The resultant high temperature hypersonic flow can be used to simulate atmospheric re-entry of spacecraft or hypersonic craft, again with limited testing times.
See also
- Gas dynamicsGas dynamicsGas dynamics is a branch of fluid dynamics concerned with studying the motion of gases and its consequent effects. Gas dynamics combines the principles of fluid mechanics and thermodynamics...
- Transonic flow
- Supersonic flow
- Hypersonic flow
- Fanno flowFanno flowFanno flow refers to adiabatic flow through a constant area duct where the effect of friction is considered. Compressibility effects often come into consideration, although the Fanno flow model certainly also applies to incompressible flow. For this model, the duct area remains constant, the flow...
- Rayleigh flowRayleigh flowRayleigh flow refers to diabatic flow through a constant area duct where the effect of heat addition or rejection is considered. Compressibility effects often come into consideration, although the Rayleigh flow model certainly also applies to incompressible flow. For this model, the duct area...
- Isothermal flowIsothermal flowIsothermal flow is a model of compressible fluid flow whereby the flow remains at the same temperature while flowing in a conduit. In the model, heat transferred through the walls of the conduit is offset by frictional heating back into the flow. Although the flow temperature remains constant, a...
- Mach numberMach numberMach number is the speed of an object moving through air, or any other fluid substance, divided by the speed of sound as it is in that substance for its particular physical conditions, including those of temperature and pressure...
- AerodynamicsAerodynamicsAerodynamics is a branch of dynamics concerned with studying the motion of air, particularly when it interacts with a moving object. Aerodynamics is a subfield of fluid dynamics and gas dynamics, with much theory shared between them. Aerodynamics is often used synonymously with gas dynamics, with...
- Nozzles