In mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, the homotopy principle (or h-principle) is a very general way to solve partial differential equation

Partial differential equation

In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...

s (PDEs), and more generally partial differential relations (PDRs). The h-principle is good for underdetermined PDEs or PDRs, such as occur in the immersion problem, isometric immersion problem, and other areas.

The theory was started by works of Yakov Eliashberg

Yakov Eliashberg

Yakov Eliashberg is a Russian mathematician. He received his Ph.D. from Leningrad University in 1972 under the direction of Vladimir Rokhlin. From 1972 to 1979 he taught at the Syktyvkar State University of Komi Republic of Russia and from 1980 to 1987 worked in industry as the head of a computer...

, Mikhail Gromov and Anthony V. Phillips. It was based on earlier results that reduced partial differential relations to homotopy, particularly for immersions.
These started with the Whitney–Graustein theorem, and followed the work of Stephen Smale

Stephen Smale

Steven Smale a.k.a. Steve Smale, Stephen Smale is an American mathematician from Flint, Michigan. He was awarded the Fields Medal in 1966, and spent more than three decades on the mathematics faculty of the University of California, Berkeley .-Education and career:He entered the University of...

 and Morris W. Hirsch on immersions; also work by Nicolaas Kuiper

Nicolaas Kuiper

Nicolaas Hendrik "Nico" Kuiper was a Dutch mathematician, known for Kuiper's test and proving Kuiper's theorem. He also contributed to the Nash embedding theorem.Kuiper completed his Ph.D...

 and John Forbes Nash

John Forbes Nash

John Forbes Nash, Jr. is an American mathematician whose works in game theory, differential geometry, and partial differential equations have provided insight into the forces that govern chance and events inside complex systems in daily life...

.

Rough idea

Assume we want to find a function ƒ on R^m which satisfies a partial differential equation of degree k, in co-ordinates . One can rewrite it as


where stands for all partial derivatives of ƒ up to order k. Let us exchange every variable in for new independent variables
Then our original equation can be thought as a system of


and some number of equations of the following type

A solution of


is called a non-holonomic solution, and a solution of the system (which is a solution of our original PDE) is called a holonomic solution.
In order to check if a solution exists, first check if there is a non-holonomic solution (usually it is quite easy and if not then our original equation did not have any solutions).

A PDE satisfies the h-principle if any non-holonomic solution can be deformed

Homotopy

In topology, two continuous functions from one topological space to another are called homotopic if one can be "continuously deformed" into the other, such a deformation being called a homotopy between the two functions...

 into a holonomic one in the class of non-holonomic solutions.

Therefore, once you prove that an equation satisfies the h-principle, it is really easy to check whether it has solutions. Many underdetermined partial differential equations satisfy the h-principle. However, the falsity of an h-principle is also an interesting statement, intuitively this means the objects being studied have non-trivial geometry that cannot be reduced to topology. As an example, embedded Lagrangians in a symplectic manifold do not satisfy an h-principle, to prove this one needs to find invariants coming from pseudo-holomorphic curves

Pseudoholomorphic curve

In mathematics, specifically in topology and geometry, a pseudoholomorphic curve is a smooth map from a Riemann surface into an almost complex manifold that satisfies the Cauchy–Riemann equation. Introduced in 1985 by Mikhail Gromov, pseudoholomorphic curves have since revolutionized the study of...

.

Monotone functions

Perhaps the simplest partial differential relation is for the derivative to not vanish: Properly, this is an ordinary differential relation, as this is a function in one variable. These are the strictly monotone differentiable functions, either increasing or decreasing, and one may ask the homotopy type of this space, as compared with spaces without this restriction. The space of (differentiable, strictly) monotone functions on the real line consists of two disjoint convex set

Convex set

In Euclidean space, an object is convex if for every pair of points within the object, every point on the straight line segment that joins them is also within the object...

s: the increasing ones and the decreasing ones, and has the homotopy type of two points. The space of all functions on the real line is a convex set, and has the homotopy type of one point. This does not appear promising – they have not even the same components – but closer examination reveals that this is the only problem: all of the higher homotopy groups agree. If instead one restricts to all maps with given endpoint values: such that and , then for the inclusion of functions with non-vanishing derivative in all continuous functions is a homotopy equivalence – both the spaces are convex, and in fact the monotone functions are a convex subset. Further, there is a natural base point, namely the linear function – this is the function with shortest path length in this space.

While this is a very simple example, it illustrates some of the general aspects of h-principles:

• The lowest homotopy groups – showing that the inclusion is 0-connected or 1-connected – is hardest;
• h-principles are largely about showing that higher homotopy groups agree (rather, that the inclusion is an isomorphism on these groups) – showing that, once an inclusion has been shown to be 1-connected, it is in fact n-connected
  N-connected
  In the mathematical branch of algebraic topology, specifically homotopy theory, n-connectedness is a way to say that a space vanishes or that a map is an isomorphism "up to dimension n, in homotopy".-n-connected space:...
  
  , possibly for all n;
• h-principles can sometimes be shown by variational methods
  Calculus of variations
  Calculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
  
  , as in the above length example.

This example also extends to significant results:
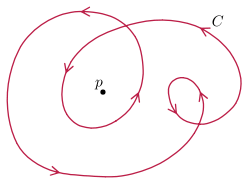
extending this to immersions of a circle into itself classifies them by order (or winding number

Winding number

In mathematics, the winding number of a closed curve in the plane around a given point is an integer representing the total number of times that curve travels counterclockwise around the point...

), by lifting the map to the universal covering space and applying the above analysis to the resulting monotone map – the linear map corresponds to multiplying angle: ( in complex numbers). Note that here there are no immersions of order 0, as those would need to turn back on themselves. Extending this to circles immersed in the plane – the immersion condition is precisely the condition that the derivative does not vanish – the Whitney–Graustein theorem classified these by turning number by considering the homotopy class of the Gauss map

Gauss map

In differential geometry, the Gauss map maps a surface in Euclidean space R3 to the unit sphere S2. Namely, given a surface X lying in R3, the Gauss map is a continuous map N: X → S2 such that N is a unit vector orthogonal to X at p, namely the normal vector to X at p.The Gauss map can be defined...

 and showing that this satisfies an h-principle; here again order 0 is more complicated.

Smale's classification of immersions of spheres as the homotopy groups of Stiefel manifold

Stiefel manifold

In mathematics, the Stiefel manifold Vk is the set of all orthonormal k-frames in Rn. That is, it is the set of ordered k-tuples of orthonormal vectors in Rn. It is named after Swiss mathematician Eduard Stiefel...

s, and Hirsch's generalization of this to immersions of manifolds being classified as homotopy classes of maps of frame bundle

Frame bundle

In mathematics, a frame bundle is a principal fiber bundle F associated to any vector bundle E. The fiber of F over a point x is the set of all ordered bases, or frames, for Ex...

s are much further-reaching generalizations, and much more involved, but similar in principle – immersion requires the derivative to have rank k, which requires the partial derivatives in each direction to not vanish and to be linearly independent, and the resulting analog of the Gauss map is a map to the Stiefel manifold, or more generally between frame bundles.

A car in the plane

As another simple example, consider a car moving in the plane. The position of a car in the plane is determined by three parameters: two coordinates and for the location (a good choice is the location of the midpoint between the back wheels) and an angle which describes the orientation of the car. The motion of the car satisfies the equation

since a non-skidding car must move in the direction of its wheels. In robotics

Robotics

Robotics is the branch of technology that deals with the design, construction, operation, structural disposition, manufacture and application of robots...

 terms, not all paths in the task space are holonomic.

A non-holonomic solution in this case, roughly speaking, corresponds to a motion of the car by sliding in the plane. In this case the non-holonomic solutions are not only homotopic to holonomic ones but also can be arbitrarily well approximated by the holonomic ones (by going back and forth, like parallel parking in a limited space) – note that this approximates both the position and the angle of the car arbitrarily closely. This implies that, theoretically, it is possible to parallel park in any space longer than the length of your car. It also implies that, in a contact 3 manifold, any curve is -close to a Legendrian

Legendrian knot

In mathematics, a Legendrian knot often refers to a smooth embedding of the circle into \mathbb R^3, which is tangent to the standard contact structure on \mathbb R^3...

 curve.
This last property is stronger than the general h-principle; it is called the -dense h-principle.

While this example is simple, compare to the Nash embedding theorem

Nash embedding theorem

The Nash embedding theorems , named after John Forbes Nash, state that every Riemannian manifold can be isometrically embedded into some Euclidean space. Isometric means preserving the length of every path...

, specifically the Nash–Kuiper theorem, which says that any short

Short map

In the mathematical theory of metric spaces, a metric map is a function between metric spaces that does not increase any distance .These maps are the morphisms in the category of metric spaces, Met ....

 smooth () embedding or immersion of in or larger can be arbitrarily well approximated by an isometric -embedding (respectively, immersion). This is also a dense h-principle, and can be proven by an essentially similar "wrinkling" – or rather, circling – technique to the car in the plane, though it is much more involved.

Some paradoxes

Here we list a few counter-intuitive results which can be proved by applying the
h-principle:

• Cone Eversion. Let us consider functions f on R² without origin f(x) = |x|. Then there is a continuous one-parameter family of functions such that , and for any , is not zero at any point.

• Any open manifold admits a (non-complete) Riemannian metric of positive (or negative) curvature.

• Smale's paradox
  Smale's paradox
  In differential topology, Smale's paradox states that it is possible to turn a sphere inside out in a three-dimensional space with possible self-intersections but without creating any crease, a process often called sphere eversion...
  
   can be done using isometric embedding of .

• Nash embedding theorem
  Nash embedding theorem
  The Nash embedding theorems , named after John Forbes Nash, state that every Riemannian manifold can be isometrically embedded into some Euclidean space. Isometric means preserving the length of every path...