Kinetic isotope effect
Encyclopedia
The kinetic isotope effect (KIE) is the ratio of reaction rates of two different isotopically labeled molecules in a chemical reaction. It is also called "isotope fractionation," although this term is somewhat broader in meaning. A KIE involving hydrogen
and deuterium
is represented as:
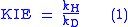
with kH and kD are reaction rate constants.
An isotopic substitution can greatly modify the reaction rate
when the isotopic replacement is in a chemical bond
that is broken or formed in the rate limiting step. In such a case, the change is termed a primary isotope effect. When the substitution is not involved in the bond that is breaking or forming, a smaller rate change, termed a secondary isotope effect is observed. Thus, the magnitude of the kinetic isotope effect can be used to elucidate the reaction mechanism
. If other steps are partially rate-determining, the effect of isotopic substitution will be masked. This masking of the intrinsic isotope effect has been referred to as 'kinetic complexity'.
Isotopic rate changes are most pronounced when the relative mass
change is greatest, since the effect is related to vibrational frequencies of the affected bonds. For instance, changing a hydrogen
atom to deuterium
represents a 100% increase in mass, whereas in replacing carbon
-12 with carbon-13, the mass increases by only 8%. The rate of a reaction involving a C-H bond is typically 6 to 10 times faster than the corresponding C-D bond, whereas a 12C reaction is only ~1.04 times faster than the corresponding 13C reaction (even though, in both cases, the isotope is one atomic mass unit
heavier).
Isotopic substitution can modify the rate of reaction in a variety of ways. In many cases, the rate difference can be rationalized by noting that the mass of an atom affects the vibrational frequency
of the chemical bond
that it forms, even if the electron configuration
is nearly identical. Heavier atoms will (classically
) lead to lower vibration frequencies, or, viewed quantum mechanically
, will have lower zero-point energy
. With a lower zero-point energy, more energy must be supplied to break the bond, resulting in a higher activation energy
for bond cleavage, which in turn lowers the measured rate (see, for example, the Arrhenius equation
).
The Swain equation (also cited as the Swain-Schaad-Stivers equations) relate the intrinsic KIEs for the hydrogen isotopes to each other - i.e. using these relationships, one can extract the protium/tritium (H/T) KIE or the deuterium/tritium (D/T) KIE from the protium/deuterium KIE (H/D); similarly, if one of these KIEs is known, the other two can be extracted using the appropriate form of the Swain-Schaad equation.
The kinetic isotope effect leads to a specific distribution of deuterium isotopes in natural products, depending on the route they were synthesized in nature. By NMR spectroscopy, it is therefore easy to detect whether the alcohol in wine was fermented from glucose
, or from illicitly added saccharose.
Isotopic effect expressed with Eq. (1) only refer to reactions that can be described with first-order kinetics. In all instances in which this is not possible, transient kinetic isotope effects
should be taken into account using the GEBIK and GEBIF equations.
:
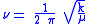
where k is the spring constant for the bond, and μ is the reduced mass
of the A-B system:
(
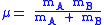
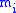is the mass of atom 
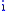). Quantum mechanically, the energy of the 
-th level of a harmonic oscillator is given by:
Thus, the zero-point energy (
= 0) will decrease as the reduced mass increases. With a lower zero-point energy, more energy is required to overcome the activation energy for bond cleavage.
In changing a carbon-hydrogen bond to a carbon-deuterium bond, k remains unchanged, but the reduced mass µ is different. As a good approximation, on going from C-H to C-D, the reduced mass increases by a factor of approximately 2. Thus, the frequency for a C-D bond should be approximately 1/√2 or 0.71 times that of the corresponding C-H bond. This effect is much larger than for changing the carbon-12 to carbon-13.
In physical organic chemistry, SKIE is discussed in terms of electronic effect
s such as induction, bond hybridization, or hyperconjugation
. These properties are determined by electron distribution, and depend upon vibrationally averaged bond length and angles that are not greatly affected by isotopic substitution. Thus, the use of the term "electronic isotope effect" while legitimate is discouraged from use as it can be misinterpreted to suggest that the isotope effect is electronic in nature rather than vibrational.
SKIE's can be explained in terms of changes in orbital hybridization. When the hybridization of a carbon atom changes from sp3 to sp2, a number of vibrational modes (stretches, in-plane and out-of-plane bending) are affected. The in-plane and out-of-plane bending in an sp3 hybridized carbon are similar in frequency due to the symmetry of an sp3 hybridized carbon. In an sp2 hybridized carbon the in-plane bend is much stiffer than the out-of-plane bending resulting in a large difference in the frequency, the ZPE
and thus the SKIE (which exists when there is a difference in the ZPE of the reactant and transition state). The theoretical maximum change caused by the bending frequency difference has been calculated as 1.4.
When carbon undergoes a reaction that changes its hybridization from sp3 to sp2, the out of plane bending force constant at the transition state is weaker as it is developing sp2 character and a "normal" SKIE is observed with typical values of 1.1 to 1.2. Conversely, when carbon's hybridization changes from sp2 to sp3, the out of plane bending force constants at the transition state increase and an inverse SKIE is observed with typical values of 0.8 to 0.9.
More generally the SKIE for reversible reactions can be "normal" one way and "inverse" the other if bonding in the transition state is midway in stiffness between substrate and product, or they can be "normal" both ways if bonding is weaker in the transition state, or "inverse" both ways if bonding is stronger in the transition state than in either reactant.
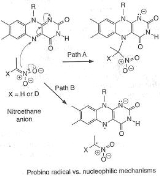
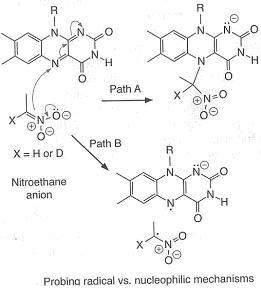
An example of an "inverse" α secondary kinetic isotope effect can be seen in the work of Fitzpatrick and Kurtz who used such an effect to distinguish between two proposed pathways for the reaction of d-amino acid oxidase
with nitroalkane anions. Path A involved a nucleophilic attack on the coenzyme FAD
, while path B involves a free-radical intermediate. As path A results in the intermediate carbon changing hybridization from sp2 to sp3 an "inverse" a SKIE is expected. If path B occurs then no SKIE should be observed as the free radical intermediate does not change hybridization. An SKIE of 0.84 was observed and Path A verified as shown in the scheme below.
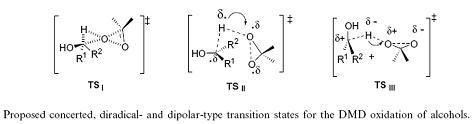
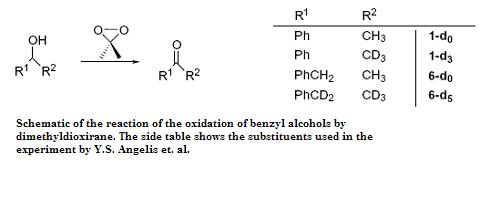
Another example of an SKIE is the oxidation of benzyl alcohols by dimethyldioxirane where three transition states for different mechanisms were proposed. Angelis et al. used SKIE to show that TS| was the transition state as TS|| and TS||| should show a large SKIE due to the hyperconjugative effect of the five hydrogen atoms in 6-d0 versus the five deuterium atoms in 6-d5 (kH/kD = 1.03–1.1 per deuterium atom). This did not match the observed SKIE of approximately 1 which eliminated TS|| and TS|||.
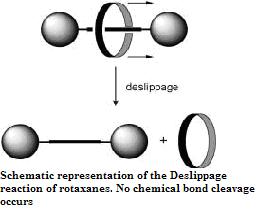
Another example of the steric isotope effect has been reported by Schalley and Felder in the deslipping reaction of rotaxanes where the deuterium isotope due to its smaller effective size allows faster passage through the stoppers resulting in faster rates of deslipping for the deuterated rotaxanes. A depiction of the deslippage reaction of rotaxanes where the mechanical bond is broken and the components freed without cleavage of chemical bonds.
s are routinely elucidated using the kinetic isotope effect, e.g. the halogenation
of toluene
:
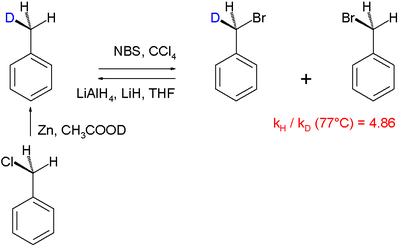
In this particular "intramolecular KIE" study the radical substitution
of hydrogen by bromine is examined in the reaction involving N-bromosuccinimide
as the brominating agent. With mono-deuterated toluene (obtained by organic reduction of benzyl chloride
with zinc
and deuterated acetic acid
), it was found that hydrogen is replaced by bromine faster than deuterium, the reaction product gets enriched in deuterium. A KIE of 4.86 was determined. This finding is in accordance with the general accepted view of a radical substitution in which a hydrogen atom is removed by a bromine free radical species in the rate-determining step
.
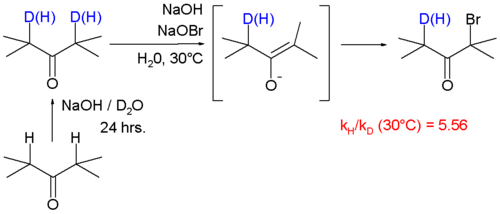
A large KIE of 5.56 is also reported for reaction of ketone
s with bromine
and sodium hydroxide forming a haloketone
with the α-carbonyl positions deuterated.
In this reaction the rate-limiting step is enolate formation by proton (deuterium) abstraction from the ketone by base. In this study the KIE is calculated from the reaction rate constants for regular 2,4-dimethyl-3-pentanone and its deuterated isomer by optical density measurements.
. This is typically only observed for reactions involving bonds to hydrogen atoms.
Tunneling occurs when a molecule penetrates through a potential energy barrier rather than over it. Although not allowed by the laws of classical mechanics
, particles can pass through classically forbidden regions of space in quantum mechanics based on wave-particle duality.
Analysis of tunneling can be made using Bell’s modification of the Arrhenius equation
which includes the addition of a tunneling factor, Q:
where A is the Arrhenius parameter, E is the barrier height
and
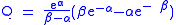
where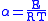
and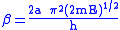
Examination of the β term shows exponential dependency on the mass of the particle. As a result, tunneling is much more likely for a lighter particle such as hydrogen. Simply doubling the mass of a tunneling proton by replacing it with its deuterium
isotope drastically reduces the rate of such reactions. As a result, very large kinetic isotope effects are observed that can not be accounted for by differences in zero point energies.
In addition, the β term depends linearly with barrier width, 2a. As with mass, tunneling is greatest for small barrier widths. Optimal tunneling distances of protons between donor and acceptor atom is 0.4 Å.
. Therefore, tunneling tends to become more important at low temperatures, where even the smallest kinetic energy barriers may not be overcome but can be tunneled through.
Peter S. Zuev et al. reported rate constants for the ring expansion of 1-methylcyclobutylfluorocarbene to be 4.0 x 10−6/s in nitrogen and 4.0 x 10−5/s in argon at 8 kelvin. They calculated that at 8 kelvin, the reaction would proceed via a single quantum state of the reactant so that the reported rate constant is temperature independent and the tunneling contribution to the rate was 152 orders of magnitude greater than the contribution of passage over the transition state energy barrier.
So despite the fact that conventional chemical reactions tend to slow down dramatically as the temperature is lowered, tunneling reactions rarely change at all. Particles that tunnel through an activation barrier are a direct result of the fact that the wave function of an intermediate species, reactant or product is not confined to the energy well of a particular trough along the energy surface of a reaction but can “leak out” into the next energy minimum. In light of this, tunneling should be temperature independent.
Noboru Fujisaki et al. reported the gas phase H/D kinetic isotope effect for the hydrogen abstraction from some n-alkanes and cycloalkanes by hydrogen atoms over the temperature range 363-463 K. The data they obtained was characterized by small preexponential factor ratios AH/AD ranging from 0.43 to 0.54 and large activation energy differences from 9.0 to 9.7 kJ/mol. Basing their arguments on transition state theory
, the small A factor ratios associated with the large activation energy differences (usually about 4.5 kJ/mol for C-H(D) bonds) provided strong evidence for tunneling. For the purpose of this discussion, what is important to note is that the A factor ratio for the various paraffins they used was approximately constant throughout the temperature range.
The observed fact that tunneling isn't entirely temperature independent can be explained by the fact that not all molecules of a certain species occupy their vibrational ground state at varying temperatures. Adding thermal energy to a potential energy well could cause higher vibrational levels other than the ground state to become populated. For a conventional kinetically driven reaction, this excitation would only have a small influence on the rate. However, for a tunneling reaction, the difference between the zero point energy and the first vibrational energy level could be huge. The tunneling correction term Q is linearly dependent on barrier width and this width is significantly diminished as the number vibrational modes on the Morse potential
increase. The decrease of the barrier width can have such a huge impact on the tunneling rate that even a small population of excited vibrational states would dominate this process.
1.Δ(EaH-EaD) > Δ(ZPEH-ZPED) (Ea=activation energy; ZPE=zero point energy)
2.Reaction still proceeds at lower temperatures.
3.The Arrhenius
pre-exponential factors AD/AH is not equal to 1.
4.There is a large negative entropy
of activation.
5.The geometries of the reactants and products are usually very similar.
Also for reactions where isotopes include H, D and T, a criterion of tunneling is the Swain-Schaad relations which compare the rate constants(k) of the reactions when H,D or T are the protons:
kH/kT=(kD/kT)X and kH/kT=(kH/kD)Y
Experimental values of X exceeding 3.26 and Y lower than 1.44 are evidence of a certain amount of contribution from tunneling.
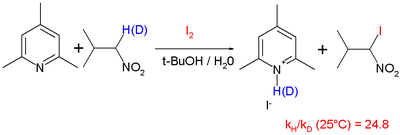
and iodination of nitropropane
with hindered pyridine
base with a reported KIE of 25 at 25 °C:
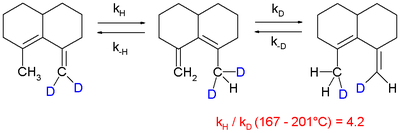
and in a 1,5-sigmatropic hydrogen shift
although it is observed that it is difficult to extrapolate experimental values obtained at elevated temperatures to lower temperatures:
There has long been speculation that high efficiency of enzyme catalysis in proton or hydride ion transfer reactions could be due partly to the quantum mechanical tunneling effect. Environment at the active site of an enzyme positions the donor and acceptor atom close to the optimal tunneling distance, where the amino acid side chains can "force" the donor and acceptor atom closer together by electrostatic and noncovalent interactions. It is also possible that the enzyme and its unusual hydrophobic environment inside a reaction site provides tunneling-promoting vibration.
Studies on ketosteroid isomerase have provided experimental evidence that the enzyme actually enhances the coupled motion/hydrogen tunneling by comparing primary and secondary kinetic isotope effects of the reaction under enzyme catalyzed and non-enzyme catalyzed conditions.
There are many examples of proton tunneling in enzyme catalyzed reactions that were discovered by KIE. A well studied example is methylamine dehydrogenase, where large primary KIEs of 5-55 have been observed for the proton transfer step.
Another example of tunneling contribution to proton transfer in enzymatic reactions is the reaction carried out by alcohol dehydrogenase
. Competitive KIEs for the hydrogen transfer step at 25 °C resulted in 3.6 and 10.2 for primary and secondary KIEs, respectively.
Hydrogen
Hydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly...
and deuterium
Deuterium
Deuterium, also called heavy hydrogen, is one of two stable isotopes of hydrogen. It has a natural abundance in Earth's oceans of about one atom in of hydrogen . Deuterium accounts for approximately 0.0156% of all naturally occurring hydrogen in Earth's oceans, while the most common isotope ...
is represented as:
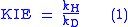
with kH and kD are reaction rate constants.
An isotopic substitution can greatly modify the reaction rate
Reaction rate
The reaction rate or speed of reaction for a reactant or product in a particular reaction is intuitively defined as how fast or slow a reaction takes place...
when the isotopic replacement is in a chemical bond
Chemical bond
A chemical bond is an attraction between atoms that allows the formation of chemical substances that contain two or more atoms. The bond is caused by the electromagnetic force attraction between opposite charges, either between electrons and nuclei, or as the result of a dipole attraction...
that is broken or formed in the rate limiting step. In such a case, the change is termed a primary isotope effect. When the substitution is not involved in the bond that is breaking or forming, a smaller rate change, termed a secondary isotope effect is observed. Thus, the magnitude of the kinetic isotope effect can be used to elucidate the reaction mechanism
Reaction mechanism
In chemistry, a reaction mechanism is the step by step sequence of elementary reactions by which overall chemical change occurs.Although only the net chemical change is directly observable for most chemical reactions, experiments can often be designed that suggest the possible sequence of steps in...
. If other steps are partially rate-determining, the effect of isotopic substitution will be masked. This masking of the intrinsic isotope effect has been referred to as 'kinetic complexity'.
Isotopic rate changes are most pronounced when the relative mass
Mass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
change is greatest, since the effect is related to vibrational frequencies of the affected bonds. For instance, changing a hydrogen
Hydrogen
Hydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly...
atom to deuterium
Deuterium
Deuterium, also called heavy hydrogen, is one of two stable isotopes of hydrogen. It has a natural abundance in Earth's oceans of about one atom in of hydrogen . Deuterium accounts for approximately 0.0156% of all naturally occurring hydrogen in Earth's oceans, while the most common isotope ...
represents a 100% increase in mass, whereas in replacing carbon
Carbon
Carbon is the chemical element with symbol C and atomic number 6. As a member of group 14 on the periodic table, it is nonmetallic and tetravalent—making four electrons available to form covalent chemical bonds...
-12 with carbon-13, the mass increases by only 8%. The rate of a reaction involving a C-H bond is typically 6 to 10 times faster than the corresponding C-D bond, whereas a 12C reaction is only ~1.04 times faster than the corresponding 13C reaction (even though, in both cases, the isotope is one atomic mass unit
Atomic mass unit
The unified atomic mass unit or dalton is a unit that is used for indicating mass on an atomic or molecular scale. It is defined as one twelfth of the rest mass of an unbound neutral atom of carbon-12 in its nuclear and electronic ground state, and has a value of...
heavier).
Isotopic substitution can modify the rate of reaction in a variety of ways. In many cases, the rate difference can be rationalized by noting that the mass of an atom affects the vibrational frequency
Molecular vibration
A molecular vibration occurs when atoms in a molecule are in periodic motion while the molecule as a whole has constant translational and rotational motion...
of the chemical bond
Chemical bond
A chemical bond is an attraction between atoms that allows the formation of chemical substances that contain two or more atoms. The bond is caused by the electromagnetic force attraction between opposite charges, either between electrons and nuclei, or as the result of a dipole attraction...
that it forms, even if the electron configuration
Electron configuration
In atomic physics and quantum chemistry, electron configuration is the arrangement of electrons of an atom, a molecule, or other physical structure...
is nearly identical. Heavier atoms will (classically
Classical physics
What "classical physics" refers to depends on the context. When discussing special relativity, it refers to the Newtonian physics which preceded relativity, i.e. the branches of physics based on principles developed before the rise of relativity and quantum mechanics...
) lead to lower vibration frequencies, or, viewed quantum mechanically
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
, will have lower zero-point energy
Zero-point energy
Zero-point energy is the lowest possible energy that a quantum mechanical physical system may have; it is the energy of its ground state. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave-like nature...
. With a lower zero-point energy, more energy must be supplied to break the bond, resulting in a higher activation energy
Activation energy
In chemistry, activation energy is a term introduced in 1889 by the Swedish scientist Svante Arrhenius that is defined as the energy that must be overcome in order for a chemical reaction to occur. Activation energy may also be defined as the minimum energy required to start a chemical reaction...
for bond cleavage, which in turn lowers the measured rate (see, for example, the Arrhenius equation
Arrhenius equation
The Arrhenius equation is a simple, but remarkably accurate, formula for the temperature dependence of the reaction rate constant, and therefore, rate of a chemical reaction. The equation was first proposed by the Dutch chemist J. H. van 't Hoff in 1884; five years later in 1889, the Swedish...
).
The Swain equation (also cited as the Swain-Schaad-Stivers equations) relate the intrinsic KIEs for the hydrogen isotopes to each other - i.e. using these relationships, one can extract the protium/tritium (H/T) KIE or the deuterium/tritium (D/T) KIE from the protium/deuterium KIE (H/D); similarly, if one of these KIEs is known, the other two can be extracted using the appropriate form of the Swain-Schaad equation.
The kinetic isotope effect leads to a specific distribution of deuterium isotopes in natural products, depending on the route they were synthesized in nature. By NMR spectroscopy, it is therefore easy to detect whether the alcohol in wine was fermented from glucose
Glucose
Glucose is a simple sugar and an important carbohydrate in biology. Cells use it as the primary source of energy and a metabolic intermediate...
, or from illicitly added saccharose.
Isotopic effect expressed with Eq. (1) only refer to reactions that can be described with first-order kinetics. In all instances in which this is not possible, transient kinetic isotope effects
Transient kinetic isotope fractionation
Transient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
should be taken into account using the GEBIK and GEBIF equations.
Inverse KIE's
Reactions are known where the deuterated species reacts faster than the undeuterated analogue, and these cases are said to exhibit inverse kinetic isotope effects (IKIE). IKIE's are often observed in the reductive elimination of alkyl metal hydrides, e.g. (Me2NCH2CH2NMe2)PtMe(H). In such cases the C-D bond in the transition state, an agostic species, is highly stabilized relative to the C-H bond.Mathematical details in a diatomic molecule
One approach to studying the effect is for that of a diatomic molecule. The fundamental vibrational frequency (ν) of a chemical bond between atom A and B is, when approximated by a harmonic oscillatorHarmonic oscillator
In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force, F, proportional to the displacement, x: \vec F = -k \vec x \, where k is a positive constant....
:
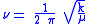
where k is the spring constant for the bond, and μ is the reduced mass
Reduced mass
Reduced mass is the "effective" inertial mass appearing in the two-body problem of Newtonian mechanics. This is a quantity with the unit of mass, which allows the two-body problem to be solved as if it were a one-body problem. Note however that the mass determining the gravitational force is not...
of the A-B system:
(
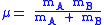
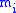is the mass of atom 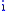). Quantum mechanically, the energy of the -th level of a harmonic oscillator is given by:Thus, the zero-point energy (
= 0) will decrease as the reduced mass increases. With a lower zero-point energy, more energy is required to overcome the activation energy for bond cleavage.In changing a carbon-hydrogen bond to a carbon-deuterium bond, k remains unchanged, but the reduced mass µ is different. As a good approximation, on going from C-H to C-D, the reduced mass increases by a factor of approximately 2. Thus, the frequency for a C-D bond should be approximately 1/√2 or 0.71 times that of the corresponding C-H bond. This effect is much larger than for changing the carbon-12 to carbon-13.
Secondary isotope effect
The secondary kinetic isotope effect (SKIE) arises in cases where the isotopic substitution is remote from the bond being broken. The remote atom, nonetheless, influences the internal vibrations of the system that via changes in the zero point energy (ZPE) affect the rates of chemical reactions. Such effects are expressed as ratios of rate for the light isotope to that of the heavy isotope and can be "normal" (ratio is greater than or equal to 1) or "inverse" (ratio is less than 1) effects. SKIE are defined as α,β (etc.) secondary isotope effects where such prefixes refer to the position of the isotopic substitution relative to the reaction center (see alpha and beta carbon). The prefix α refers to the isotope associated with the reaction center while the prefix β refers to the isotope associated with an atom neighboring the reaction center and so on.In physical organic chemistry, SKIE is discussed in terms of electronic effect
Electronic effect
An electronic effect influences the structure, reactivity, or properties of molecule but is neither a traditional bond nor a steric effect. In organic chemistry, the term stereoelectronic effect is also used to emphasize the relation between the electronic structure and the geometry of a...
s such as induction, bond hybridization, or hyperconjugation
Hyperconjugation
In organic chemistry, hyperconjugation is the interaction of the electrons in a sigma bond with an adjacent empty non-bonding p-orbital or antibonding π orbital or filled π orbital, to give an extended molecular orbital that increases the stability of the system...
. These properties are determined by electron distribution, and depend upon vibrationally averaged bond length and angles that are not greatly affected by isotopic substitution. Thus, the use of the term "electronic isotope effect" while legitimate is discouraged from use as it can be misinterpreted to suggest that the isotope effect is electronic in nature rather than vibrational.
SKIE's can be explained in terms of changes in orbital hybridization. When the hybridization of a carbon atom changes from sp3 to sp2, a number of vibrational modes (stretches, in-plane and out-of-plane bending) are affected. The in-plane and out-of-plane bending in an sp3 hybridized carbon are similar in frequency due to the symmetry of an sp3 hybridized carbon. In an sp2 hybridized carbon the in-plane bend is much stiffer than the out-of-plane bending resulting in a large difference in the frequency, the ZPE
Zero-point energy
Zero-point energy is the lowest possible energy that a quantum mechanical physical system may have; it is the energy of its ground state. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave-like nature...
and thus the SKIE (which exists when there is a difference in the ZPE of the reactant and transition state). The theoretical maximum change caused by the bending frequency difference has been calculated as 1.4.
When carbon undergoes a reaction that changes its hybridization from sp3 to sp2, the out of plane bending force constant at the transition state is weaker as it is developing sp2 character and a "normal" SKIE is observed with typical values of 1.1 to 1.2. Conversely, when carbon's hybridization changes from sp2 to sp3, the out of plane bending force constants at the transition state increase and an inverse SKIE is observed with typical values of 0.8 to 0.9.
More generally the SKIE for reversible reactions can be "normal" one way and "inverse" the other if bonding in the transition state is midway in stiffness between substrate and product, or they can be "normal" both ways if bonding is weaker in the transition state, or "inverse" both ways if bonding is stronger in the transition state than in either reactant.
An example of an "inverse" α secondary kinetic isotope effect can be seen in the work of Fitzpatrick and Kurtz who used such an effect to distinguish between two proposed pathways for the reaction of d-amino acid oxidase
D-amino acid oxidase
D-amino acid oxidase is a peroxisomal enzyme containing FAD as cofactor that is expressed in a wide range of species from yeasts to human. It is not present in bacteria or in plants...
with nitroalkane anions. Path A involved a nucleophilic attack on the coenzyme FAD
FAD
In biochemistry, flavin adenine dinucleotide is a redox cofactor involved in several important reactions in metabolism. FAD can exist in two different redox states, which it converts between by accepting or donating electrons. The molecule consists of a riboflavin moiety bound to the phosphate...
, while path B involves a free-radical intermediate. As path A results in the intermediate carbon changing hybridization from sp2 to sp3 an "inverse" a SKIE is expected. If path B occurs then no SKIE should be observed as the free radical intermediate does not change hybridization. An SKIE of 0.84 was observed and Path A verified as shown in the scheme below.
Another example of an SKIE is the oxidation of benzyl alcohols by dimethyldioxirane where three transition states for different mechanisms were proposed. Angelis et al. used SKIE to show that TS| was the transition state as TS|| and TS||| should show a large SKIE due to the hyperconjugative effect of the five hydrogen atoms in 6-d0 versus the five deuterium atoms in 6-d5 (kH/kD = 1.03–1.1 per deuterium atom). This did not match the observed SKIE of approximately 1 which eliminated TS|| and TS|||.
Steric isotope effect
The steric isotope effect is an SKIE that does not involve bond breaking or formation. This effect is attributed to the different vibrational amplitudes of isotopologues (molecular entities differing in isotopic composition only.) An example of such an effect can be seen in the work of Kurt Mislow et al. who demonstrated through the racemization of 9,10-dihydro-4,5-dimethylphenanthrene that the smaller amplitude of vibration for deuterium as compared to hydrogen in C-H (Carbon -Hydrogen), C-D (Carbon -Deuterium) bonds results in a smaller van der Waals radius or effective size in addition to a difference in the ZPE between the two. When there is a greater effective bulk of molecules containing one over the other this may be manifested by a steric effect on the rate constant. For the example above deuterium racemizes faster than the hydrogen isotopologue resulting in a steric isotope effect.Another example of the steric isotope effect has been reported by Schalley and Felder in the deslipping reaction of rotaxanes where the deuterium isotope due to its smaller effective size allows faster passage through the stoppers resulting in faster rates of deslipping for the deuterated rotaxanes. A depiction of the deslippage reaction of rotaxanes where the mechanical bond is broken and the components freed without cleavage of chemical bonds.
Applications
Reaction mechanismReaction mechanism
In chemistry, a reaction mechanism is the step by step sequence of elementary reactions by which overall chemical change occurs.Although only the net chemical change is directly observable for most chemical reactions, experiments can often be designed that suggest the possible sequence of steps in...
s are routinely elucidated using the kinetic isotope effect, e.g. the halogenation
Halogenation
Halogenation is a chemical reaction that incorporates a halogen atom into a molecule in substitution of hydrogen atom. Halogenation takes place in the gas phase. There are four types of halogenation: fluorination, chlorination, bromination, and iodination...
of toluene
Toluene
Toluene, formerly known as toluol, is a clear, water-insoluble liquid with the typical smell of paint thinners. It is a mono-substituted benzene derivative, i.e., one in which a single hydrogen atom from the benzene molecule has been replaced by a univalent group, in this case CH3.It is an aromatic...
:
In this particular "intramolecular KIE" study the radical substitution
Radical substitution
In organic chemistry, a radical substitution reaction is a substitution reaction involving free radicals as a reactive intermediate.The reaction always involves at least two steps, and possibly a third....
of hydrogen by bromine is examined in the reaction involving N-bromosuccinimide
N-Bromosuccinimide
N-Bromosuccinimide or NBS is a chemical reagent which is used in radical substitution and electrophilic addition reactions in organic chemistry. NBS can be considered a convenient source of cationic bromine.-Preparation:...
as the brominating agent. With mono-deuterated toluene (obtained by organic reduction of benzyl chloride
Benzyl chloride
Benzyl chloride, or α-chlorotoluene, is an organic compound with the formula C6H5CH2Cl. This colourless liquid is a reactive organochlorine compound that is a widely used chemical building block.-Preparation:...
with zinc
Zinc
Zinc , or spelter , is a metallic chemical element; it has the symbol Zn and atomic number 30. It is the first element in group 12 of the periodic table. Zinc is, in some respects, chemically similar to magnesium, because its ion is of similar size and its only common oxidation state is +2...
and deuterated acetic acid
Acetic acid
Acetic acid is an organic compound with the chemical formula CH3CO2H . It is a colourless liquid that when undiluted is also called glacial acetic acid. Acetic acid is the main component of vinegar , and has a distinctive sour taste and pungent smell...
), it was found that hydrogen is replaced by bromine faster than deuterium, the reaction product gets enriched in deuterium. A KIE of 4.86 was determined. This finding is in accordance with the general accepted view of a radical substitution in which a hydrogen atom is removed by a bromine free radical species in the rate-determining step
Rate-determining step
The rate-determining step is a chemistry term for the slowest step in a chemical reaction. The rate-determining step is often compared to the neck of a funnel; the rate at which water flows through the funnel is determined by the width of the neck, not by the speed at which water is poured in. In...
.
A large KIE of 5.56 is also reported for reaction of ketone
Ketone
In organic chemistry, a ketone is an organic compound with the structure RCR', where R and R' can be a variety of atoms and groups of atoms. It features a carbonyl group bonded to two other carbon atoms. Many ketones are known and many are of great importance in industry and in biology...
s with bromine
Bromine
Bromine ") is a chemical element with the symbol Br, an atomic number of 35, and an atomic mass of 79.904. It is in the halogen element group. The element was isolated independently by two chemists, Carl Jacob Löwig and Antoine Jerome Balard, in 1825–1826...
and sodium hydroxide forming a haloketone
Haloketone
A haloketone in organic chemistry is a functional group consisting of a ketone group or more general a carbonyl group with a α-halogen substituent. The general structure is RR'CCR where R is an alkyl or aryl residue and X any one of the halogens...
with the α-carbonyl positions deuterated.
In this reaction the rate-limiting step is enolate formation by proton (deuterium) abstraction from the ketone by base. In this study the KIE is calculated from the reaction rate constants for regular 2,4-dimethyl-3-pentanone and its deuterated isomer by optical density measurements.
Tunneling
In some cases, an additional rate enhancement is seen for the lighter isotope, possibly due to quantum mechanical tunnellingQuantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
. This is typically only observed for reactions involving bonds to hydrogen atoms.
Tunneling occurs when a molecule penetrates through a potential energy barrier rather than over it. Although not allowed by the laws of classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
, particles can pass through classically forbidden regions of space in quantum mechanics based on wave-particle duality.
Analysis of tunneling can be made using Bell’s modification of the Arrhenius equation
Arrhenius equation
The Arrhenius equation is a simple, but remarkably accurate, formula for the temperature dependence of the reaction rate constant, and therefore, rate of a chemical reaction. The equation was first proposed by the Dutch chemist J. H. van 't Hoff in 1884; five years later in 1889, the Swedish...
which includes the addition of a tunneling factor, Q:
where A is the Arrhenius parameter, E is the barrier height
and
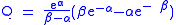
where
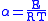
and
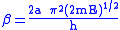
Examination of the β term shows exponential dependency on the mass of the particle. As a result, tunneling is much more likely for a lighter particle such as hydrogen. Simply doubling the mass of a tunneling proton by replacing it with its deuterium
Deuterium
Deuterium, also called heavy hydrogen, is one of two stable isotopes of hydrogen. It has a natural abundance in Earth's oceans of about one atom in of hydrogen . Deuterium accounts for approximately 0.0156% of all naturally occurring hydrogen in Earth's oceans, while the most common isotope ...
isotope drastically reduces the rate of such reactions. As a result, very large kinetic isotope effects are observed that can not be accounted for by differences in zero point energies.
In addition, the β term depends linearly with barrier width, 2a. As with mass, tunneling is greatest for small barrier widths. Optimal tunneling distances of protons between donor and acceptor atom is 0.4 Å.
Temperature Dependence in Tunneling
Tunneling is a quantum mechanical effect tied to the laws of wave mechanics, not kineticsKinetics (physics)
In physics and engineering, kinetics is a term for the branch of classical mechanics that is concerned with the relationship between the motion of bodies and its causes, namely forces and torques...
. Therefore, tunneling tends to become more important at low temperatures, where even the smallest kinetic energy barriers may not be overcome but can be tunneled through.
Peter S. Zuev et al. reported rate constants for the ring expansion of 1-methylcyclobutylfluorocarbene to be 4.0 x 10−6/s in nitrogen and 4.0 x 10−5/s in argon at 8 kelvin. They calculated that at 8 kelvin, the reaction would proceed via a single quantum state of the reactant so that the reported rate constant is temperature independent and the tunneling contribution to the rate was 152 orders of magnitude greater than the contribution of passage over the transition state energy barrier.
So despite the fact that conventional chemical reactions tend to slow down dramatically as the temperature is lowered, tunneling reactions rarely change at all. Particles that tunnel through an activation barrier are a direct result of the fact that the wave function of an intermediate species, reactant or product is not confined to the energy well of a particular trough along the energy surface of a reaction but can “leak out” into the next energy minimum. In light of this, tunneling should be temperature independent.
Noboru Fujisaki et al. reported the gas phase H/D kinetic isotope effect for the hydrogen abstraction from some n-alkanes and cycloalkanes by hydrogen atoms over the temperature range 363-463 K. The data they obtained was characterized by small preexponential factor ratios AH/AD ranging from 0.43 to 0.54 and large activation energy differences from 9.0 to 9.7 kJ/mol. Basing their arguments on transition state theory
Transition state theory
Transition state theory explains the reaction rates of elementary chemical reactions. The theory assumes a special type of chemical equilibrium between reactants and activated transition state complexes....
, the small A factor ratios associated with the large activation energy differences (usually about 4.5 kJ/mol for C-H(D) bonds) provided strong evidence for tunneling. For the purpose of this discussion, what is important to note is that the A factor ratio for the various paraffins they used was approximately constant throughout the temperature range.
The observed fact that tunneling isn't entirely temperature independent can be explained by the fact that not all molecules of a certain species occupy their vibrational ground state at varying temperatures. Adding thermal energy to a potential energy well could cause higher vibrational levels other than the ground state to become populated. For a conventional kinetically driven reaction, this excitation would only have a small influence on the rate. However, for a tunneling reaction, the difference between the zero point energy and the first vibrational energy level could be huge. The tunneling correction term Q is linearly dependent on barrier width and this width is significantly diminished as the number vibrational modes on the Morse potential
Morse potential
The Morse potential, named after physicist Philip M. Morse, is a convenient model for the potential energy of a diatomic molecule. It is a better approximation for the vibrational structure of the molecule than the quantum harmonic oscillator because it explicitly includes the effects of bond...
increase. The decrease of the barrier width can have such a huge impact on the tunneling rate that even a small population of excited vibrational states would dominate this process.
Criteria for KIE tunneling
To determine if tunneling is involved in KIE of a reaction with H or D, there are a few criteria:1.Δ(EaH-EaD) > Δ(ZPEH-ZPED) (Ea=activation energy; ZPE=zero point energy)
2.Reaction still proceeds at lower temperatures.
3.The Arrhenius
Arrhenius
Arrhenius may refer to* Carl Axel Arrhenius , Swedish chemist and discoverer of the element yttrium* Niklas Arrhenius, Swedish discus thrower* Svante Arrhenius , Swedish physical chemist and 1903 Nobel laureate...
pre-exponential factors AD/AH is not equal to 1.
4.There is a large negative entropy
Entropy
Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...
of activation.
5.The geometries of the reactants and products are usually very similar.
Also for reactions where isotopes include H, D and T, a criterion of tunneling is the Swain-Schaad relations which compare the rate constants(k) of the reactions when H,D or T are the protons:
kH/kT=(kD/kT)X and kH/kT=(kH/kD)Y
Experimental values of X exceeding 3.26 and Y lower than 1.44 are evidence of a certain amount of contribution from tunneling.
Examples for tunneling in KIE
In organic reactions, this proton tunneling effect has been observed in such reactions as the deprotonationDeprotonation
Deprotonation is the removal of a proton from a molecule, forming the conjugate base.The relative ability of a molecule to give up a proton is measured by its pKa value. A low pKa value indicates that the compound is acidic and will easily give up its proton to a base...
and iodination of nitropropane
Nitro compound
Nitro compounds are organic compounds that contain one or more nitro functional groups . They are often highly explosive, especially when the compound contains more than one nitro group and is impure. The nitro group is one of the most common explosophores used globally...
with hindered pyridine
Pyridine
Pyridine is a basic heterocyclic organic compound with the chemical formula C5H5N. It is structurally related to benzene, with one C-H group replaced by a nitrogen atom...
base with a reported KIE of 25 at 25 °C:
and in a 1,5-sigmatropic hydrogen shift
Sigmatropic reaction
A sigmatropic reaction in organic chemistry is a pericyclic reaction wherein the net result is one σ-bond is changed to another σ-bond in an uncatalyzed intramolecular process. The name sigmatropic is the result of a compounding of the long-established sigma designation from single carbon-carbon...
although it is observed that it is difficult to extrapolate experimental values obtained at elevated temperatures to lower temperatures:
There has long been speculation that high efficiency of enzyme catalysis in proton or hydride ion transfer reactions could be due partly to the quantum mechanical tunneling effect. Environment at the active site of an enzyme positions the donor and acceptor atom close to the optimal tunneling distance, where the amino acid side chains can "force" the donor and acceptor atom closer together by electrostatic and noncovalent interactions. It is also possible that the enzyme and its unusual hydrophobic environment inside a reaction site provides tunneling-promoting vibration.
Studies on ketosteroid isomerase have provided experimental evidence that the enzyme actually enhances the coupled motion/hydrogen tunneling by comparing primary and secondary kinetic isotope effects of the reaction under enzyme catalyzed and non-enzyme catalyzed conditions.
There are many examples of proton tunneling in enzyme catalyzed reactions that were discovered by KIE. A well studied example is methylamine dehydrogenase, where large primary KIEs of 5-55 have been observed for the proton transfer step.
Another example of tunneling contribution to proton transfer in enzymatic reactions is the reaction carried out by alcohol dehydrogenase
Alcohol dehydrogenase
Alcohol dehydrogenases are a group of dehydrogenase enzymes that occur in many organisms and facilitate the interconversion between alcohols and aldehydes or ketones with the reduction of nicotinamide adenine dinucleotide...
. Competitive KIEs for the hydrogen transfer step at 25 °C resulted in 3.6 and 10.2 for primary and secondary KIEs, respectively.
See also
- Transient kinetic isotope effectTransient kinetic isotope fractionationTransient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
- Isotopic ratioTransient kinetic isotope fractionationTransient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
- Isotopic compositionTransient kinetic isotope fractionationTransient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
- Fractionation factorTransient kinetic isotope fractionationTransient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
- Isotopic enrichmentTransient kinetic isotope fractionationTransient kinetic isotope effects occur when the reaction leading to isotope fractionation does not follow pure first-order kinetics and therefore isotopic effects cannot be described with the classical equilibrium fractionation equations or with steady-state kinetic fractionation equations...
- Magnetic isotope effectMagnetic Isotope EffectMagnetic isotope effects arise when a chemical reaction involves spin-selective processes, such as the radical pair mechanism. The result is that some isotopes react preferentially, depending on their nuclear spin quantum number I. This is in contrast to more familiar mass-dependent isotope effects....