In differential geometry of curves

Differential geometry of curves

Differential geometry of curves is the branch of geometry that dealswith smooth curves in the plane and in the Euclidean space by methods of differential and integral calculus....

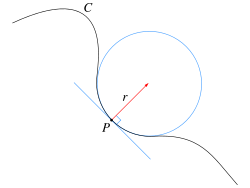
, the osculating circle of a sufficiently smooth plane curve

Curve

In mathematics, a curve is, generally speaking, an object similar to a line but which is not required to be straight...

 at a given point p on the curve has been traditionally defined as the circle

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

 passing through p and a pair of additional points on the curve infinitesimal

Infinitesimal

Infinitesimals have been used to express the idea of objects so small that there is no way to see them or to measure them. The word infinitesimal comes from a 17th century Modern Latin coinage infinitesimus, which originally referred to the "infinite-th" item in a series.In common speech, an...

ly close to p. Its center lies on the inner normal line, and its curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

 is the same as that of the given curve at that point. This circle, which is the one among all tangent circles

Tangent circles

In geometry, tangent circles are circles in a common plane that intersect in a single point. There are two types of tangency: internal and external...

at the given point that approaches the curve most tightly, was named circulus osculans (Latin

Latin

Latin is an Italic language originally spoken in Latium and Ancient Rome. It, along with most European languages, is a descendant of the ancient Proto-Indo-European language. Although it is considered a dead language, a number of scholars and members of the Christian clergy speak it fluently, and...

 for "kissing circle") by Leibniz.

The center and radius of the osculating circle at a given point are called center of curvature and radius of curvature of the curve at that point. A geometric construction was described by Isaac Newton

Isaac Newton

Sir Isaac Newton PRS was an English physicist, mathematician, astronomer, natural philosopher, alchemist, and theologian, who has been "considered by many to be the greatest and most influential scientist who ever lived."...

 in his Principia:

Description in lay terms

Imagine a car moving along a curved road on a vast flat plane. Suddenly, at one point along the road, the steering wheel locks in its present position. Thereafter, the car moves in a circle that "kisses" the road at the point of locking. The curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

 of the circle is equal to that of the road at that point. That circle is the osculating circle of the road curve at that point.

Mathematical description

Let γ(s) be a regular parametric curve, where s is the arc length

Arc length

Determining the length of an irregular arc segment is also called rectification of a curve. Historically, many methods were used for specific curves...

, or natural parameter. This determines the unit tangent vector T, the unit normal vector N, the signed curvature k(s) and the radius of curvature at each point:

Suppose that P is a point on C where k ≠ 0. The corresponding center of curvature is the point Q at distance R along N, in the same direction if k is positive and in the opposite direction if k is negative. The circle with center at Q and with radius R is called the osculating circle to the curve C at the point P.

If C is a regular space curve then the osculating circle is defined in a similar way, using the principal normal vector N. It lies in the osculating plane

Osculating plane

In mathematics, particularly in differential geometry, an osculating plane is a plane in a Euclidean space or affine space which meets a submanifold at a point in such a way as to have a second order of contact at the point. The word osculate is from the Latin osculatus which is a past participle...

, the plane spanned by the tangent and principal normal vectors T and N at the point P.

Properties

For a curve C given by a sufficiently smooth parametric equations (twice continuously differentiable), the osculating circle may be obtained by a limiting procedure: it is the limit of the circles passing through three distinct points on C as these points approach P. This is entirely analogous to the construction of the tangent

Tangent

In geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...

 to a curve as a limit of the secant lines through pairs of distinct points on C approaching P.

The osculating circle S to a plane curve C at a regular point P can be characterized by the following properties:

• The circle S passes through P.
• The circle S and the curve C have the common tangent
  Tangent lines to circles
  In Euclidean plane geometry, tangent lines to circles form the subject of several theorems, and play an important role in many geometrical constructions and proofs...
  
   line at P, and therefore the common normal line.
• Close to P, the distance between the points of the curve C and the circle S in the normal direction decays as the cube or a higher power of the distance to P in the tangential direction.

This is usually expressed as "the curve and its osculating circle have the third or higher order contact" at P. Loosely speaking, the vector functions representing C and S agree together with their first and second derivatives at P.
If the derivative of the curvature with respect to s is nonzero at P then the osculating circle crosses the curve C at P. Points P at which the derivative of the curvature is zero are called vertices

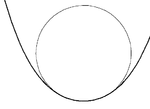
Vertex (curve)

In the geometry of curves, a vertex is a point of where the first derivative of curvature is zero. This is typically a local maximum or minimum of curvature. Other special cases may occur, for instance when the second derivative is also zero, or when the curvature is constant...

. If P is a vertex then C and its osculating circle have contact of order at least four. If, moreover, the curvature has a non-zero local maximum or minimum at P then the osculating circle touches the curve C at P but does not cross it.

The curve C may be obtained as the envelope

Envelope (mathematics)

In geometry, an envelope of a family of curves in the plane is a curve that is tangent to each member of the family at some point. Classically, a point on the envelope can be thought of as the intersection of two "adjacent" curves, meaning the limit of intersections of nearby curves...

 of the one-parameter family of its osculating circles. Their centers, i.e. the centers of curvature, form another curve, called the evolute

Evolute

In the differential geometry of curves, the evolute of a curve is the locus of all its centers of curvature. Equivalently, it is the envelope of the normals to a curve....

of C. Vertices of C correspond to singular points on its evolute.

Further reading

For some historical notes on the study of curvature, see
For application to maneuvering vehicles see

• JC Alexander and JH Maddocks: On the maneuvering of vehicles

External links

• Module for Curvature