
Positive-real function
Encyclopedia
Positive-real functions, often abbreviated to PR function, are a kind of mathematical function that first arose in electrical network analysis
. They are complex functions, Z(s), of a complex variable, s. A rational function
is defined to have the PR property if it has a positive real part and is analytic in the right halfplane of the complex plane and takes on real values on the real axis.
In symbols the definition is,
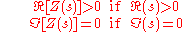
In electrical network analysis, Z(s) represents an impedance
expression and s is the complex frequency variable, often expressed as its real and imaginary parts;

in which terms the PR condition can be stated;
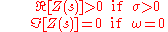
The importance to network analysis of the PR condition lies in the realisability condition. Z(s) is realisable as a one-port rational impedance if and only if it meets the PR condition. Realisable in this sense means that the impedance can be constructed from a finite (hence rational) number of discrete ideal passive
linear elements (resistor
s, inductor
s and capacitor
s in electrical terminology).
Many authors strictly adhere to this definition by explicitly requiring rationality, or by restricting attention to rational functions, at least in the first instance. However, a similar more general condition, not restricted to rational functions had earlier been considered by Cauer, and some authors ascribe the term positive-real to this type of condition, while other consider it to be a generalization of the basic definition.
(1926) who determined that it was a necessary condition. Otto Brune (1931) coined the term positive-real for the condition and proved that it was both necessary and sufficient for realisability.
functions of a wider class of passive linear electrical networks.
Some authors start from this more general definition, and then particularize it to the rational case.
admittance matrices
. So by extending the definition of PR to matrix-valued functions, linear multi-port networks which are passive may be distinguished from those that are not. A possibly irrational matrix-valued function Z(s) is PR if and only if
Network analysis (electrical circuits)
A network, in the context of electronics, is a collection of interconnected components. Network analysis is the process of finding the voltages across, and the currents through, every component in the network. There are a number of different techniques for achieving this...
. They are complex functions, Z(s), of a complex variable, s. A rational function
Rational function
In mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
is defined to have the PR property if it has a positive real part and is analytic in the right halfplane of the complex plane and takes on real values on the real axis.
In symbols the definition is,
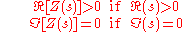
In electrical network analysis, Z(s) represents an impedance
Electrical impedance
Electrical impedance, or simply impedance, is the measure of the opposition that an electrical circuit presents to the passage of a current when a voltage is applied. In quantitative terms, it is the complex ratio of the voltage to the current in an alternating current circuit...
expression and s is the complex frequency variable, often expressed as its real and imaginary parts;

in which terms the PR condition can be stated;
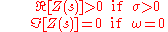
The importance to network analysis of the PR condition lies in the realisability condition. Z(s) is realisable as a one-port rational impedance if and only if it meets the PR condition. Realisable in this sense means that the impedance can be constructed from a finite (hence rational) number of discrete ideal passive
Passivity (engineering)
Passivity is a property of engineering systems, used in a variety of engineering disciplines, but most commonly found in analog electronics and control systems...
linear elements (resistor
Resistor
A linear resistor is a linear, passive two-terminal electrical component that implements electrical resistance as a circuit element.The current through a resistor is in direct proportion to the voltage across the resistor's terminals. Thus, the ratio of the voltage applied across a resistor's...
s, inductor
Inductor
An inductor is a passive two-terminal electrical component used to store energy in a magnetic field. An inductor's ability to store magnetic energy is measured by its inductance, in units of henries...
s and capacitor
Capacitor
A capacitor is a passive two-terminal electrical component used to store energy in an electric field. The forms of practical capacitors vary widely, but all contain at least two electrical conductors separated by a dielectric ; for example, one common construction consists of metal foils separated...
s in electrical terminology).
Definition
The term positive-real function was originally defined by Otto Brune to describe any function Z(s) which- is rationalRational functionIn mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
(the quotient of two polynomials), - is real when s is real
- has positive real part when s has a positive real part
Many authors strictly adhere to this definition by explicitly requiring rationality, or by restricting attention to rational functions, at least in the first instance. However, a similar more general condition, not restricted to rational functions had earlier been considered by Cauer, and some authors ascribe the term positive-real to this type of condition, while other consider it to be a generalization of the basic definition.
History
The condition was first proposed by Wilhelm CauerWilhelm Cauer
Wilhelm Cauer was a German mathematician and scientist. He is most noted for his work on the analysis and synthesis of electrical filters and his work marked the beginning of the field of network synthesis...
(1926) who determined that it was a necessary condition. Otto Brune (1931) coined the term positive-real for the condition and proved that it was both necessary and sufficient for realisability.
Properties
- The sum of two PR functions is PR.
- The compositionFunction compositionIn mathematics, function composition is the application of one function to the results of another. For instance, the functions and can be composed by computing the output of g when it has an argument of f instead of x...
of two PR functions is PR. In particular, if Z(s) is PR, then so are 1/Z(s) and Z(1/s). - All the poles and zerosZero (complex analysis)In complex analysis, a zero of a holomorphic function f is a complex number a such that f = 0.-Multiplicity of a zero:A complex number a is a simple zero of f, or a zero of multiplicity 1 of f, if f can be written asf=g\,where g is a holomorphic function g such that g is not zero.Generally, the...
of a PR function are in the left half plane or on its boundary the imaginary axis. - Any poles and zeroes on the imaginary axis are simple (have a multiplicityMultiplicity (mathematics)In mathematics, the multiplicity of a member of a multiset is the number of times it appears in the multiset. For example, the number of times a given polynomial equation has a root at a given point....
of one). - Any poles on the imaginary axis have real strictly positive residuesResidue (complex analysis)In mathematics, more specifically complex analysis, the residue is a complex number proportional to the contour integral of a meromorphic function along a path enclosing one of its singularities...
, and similarly at any zeroes on the imaginary axis, the function has a real strictly positive derivative. - Over the right half plane, the minimum value of the real part of a PR function occurs on the imaginary axis (because the real part of an analytic function constitutes a harmonic functionHarmonic functionIn mathematics, mathematical physics and the theory of stochastic processes, a harmonic function is a twice continuously differentiable function f : U → R which satisfies Laplace's equation, i.e....
over the plane, and therefore satisfies the maximum principleMaximum principleIn mathematics, the maximum principle is a property of solutions to certain partial differential equations, of the elliptic and parabolic types. Roughly speaking, it says that the maximum of a function in a domain is to be found on the boundary of that domain...
). - For a rationalRational functionIn mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
PR function, the number of poles and number of zeroes differ by at most one.
Generalizations
A couple of generalizations are sometimes made, with intention of characterizing the immittanceImmittance
Immittance in electrical and acoustical terminology is a concept combining the impedance and admittance of a system or circuit. The term was invented by Bode....
functions of a wider class of passive linear electrical networks.
Irrational functions
The impedance Z(s) of a network consisting of an infinite number of components (such as a semi-infinite ladder), need not be a rational function of s, and in particular may have branch points on the negative real s-axis. To accommodate such functions in the definition of PR, it is therefore necessary to relax the condition that the function be real for all real s, and only require this when s is positive. Thus, a possibly irrational function Z(s) is PR if and only if- Z(s) is analytic in the open right half s-plane (Re[s] > 0)
- Z(s) is real when s is positive and real
- Re[Z(s)] ≥ 0 when Re[s] ≥ 0
Some authors start from this more general definition, and then particularize it to the rational case.
Matrix-valued functions
Linear electrical networks with more than one port may be described by impedance orImpedance parameters
Impedance parameters or Z-parameters are properties used in electrical engineering, electronic engineering, and communication systems engineering to describe the electrical behavior of linear electrical networks. They are also used to describe the small-signal response of non-linear networks...
admittance matrices
Admittance parameters
Admittance parameters or Y-parameters are properties used in electrical engineering, electronic engineering, and communication systems engineering describe the electrical behavior of linear electrical networks. They are also used to describe the small-signal response of non-linear networks...
. So by extending the definition of PR to matrix-valued functions, linear multi-port networks which are passive may be distinguished from those that are not. A possibly irrational matrix-valued function Z(s) is PR if and only if
- Each element of Z(s) is analytic in the open right half s-plane (Re[s] > 0)
- Each element of Z(s) is real when s is positive and real
- The Hermitian part (Z(s) + Z†(s))/2 of Z(s) is positive semi-definitePositive-definite matrixIn linear algebra, a positive-definite matrix is a matrix that in many ways is analogous to a positive real number. The notion is closely related to a positive-definite symmetric bilinear form ....
when Re[s] ≥ 0

