Quartz crystal microbalance
Encyclopedia
A quartz crystal microbalance (QCM) measures a mass per unit area by measuring the change in frequency
of a quartz crystal resonator. The resonance
is disturbed by the addition or removal of a small mass due to oxide growth/decay or film deposition at the surface of the acoustic resonator. The QCM can be used under vacuum, in gas phase
("gas sensor", first use described by King) and more recently in liquid environments. It is useful for monitoring the rate of deposition in thin film deposition systems under vacuum. In liquid, it is highly effective at determining the affinity
of molecules (protein
s, in particular) to surfaces functionalized with recognition sites. Larger entities such as virus
es or polymer
s are investigated, as well. QCM has also been used to investigate interactions between biomolecules. Frequency measurements are easily made to high precision (discussed below); hence, it is easy to measure mass densities down to a level of below 1 μg/cm2. In addition to measuring the frequency, the dissipation
is often measured to help analysis. The dissipation is a parameter quantifying the damping
in the system, and is related to the sample's viscoelastic
properties. The dissipation is equal to the ratio of bandwidth, w, (see below) and frequency, f.
is one member of a family of crystals that experience the piezoelectric effect
. The piezoelectric effect has found applications in high power sources, sensors, actuators, frequency standards, motors, etc., and the relationship between applied voltage
and mechanical deformation is well known; this allows probing an acoustic resonance by electrical means. Applying alternating current to the quartz crystal will induce oscillations. With an alternating current between the electrodes of a properly cut crystal, a standing shear wave is generated. The Q factor
, which is the ratio of frequency and bandwidth, can be as high as 106. Such a narrow resonance leads to highly stable oscillators and a high accuracy in the determination of the resonance frequency. The QCM exploits this ease and precision for sensing. Common equipment allows resolution down to 1 Hz on crystals with a fundamental resonant frequency in the 4 – 6 MHz range. A typical setup for the QCM contains water cooling tubes, the retaining unit, frequency sensing equipment through a microdot feed-through, an oscillation source, and a measurement and recording device.
The frequency of oscillation of the quartz crystal is partially dependent on the thickness of the crystal. During normal operation, all the other influencing variables remain constant; thus a change in thickness correlates directly to a change in frequency. As mass is deposited on the surface of the crystal, the thickness increases; consequently the frequency of oscillation decreases from the initial value. With some simplifying assumptions, this frequency change can be quantified and correlated precisely to the mass change using Sauerbrey's equation
.
Other techniques for measuring the properties of thin films include Ellipsometry
, Surface Plasmon Resonance
(SPR) Spectroscopy
, and Dual Polarisation Interferometry
.
, are available. These devices exploit the Sauerbrey relation. For thin films, the resonance frequency is – by-and-large – inversely proportional to the total thickness of the plate. The latter increases when a film is deposited onto the crystal surface. Monolayer
sensitivity is easily reached. However, when the film thickness increases, viscoelastic effects come into play. In the late 80’s, it was recognized that the QCM can also be operated in liquids, if proper measures are taken to overcome the consequences of the large damping. Again, viscoelastic effects contribute strongly to the resonance properties.
Today, microweighing is one of several uses of the QCM.
Measurements of viscosity
and more general, viscoelastic properties, are of much importance as well. The “non-gravimetric” QCM is by no means an alternative to the conventional QCM. Many researchers, who use quartz resonators for purposes other than gravimetry, have continued to call the quartz crystal resonator “QCM”. Actually, the term "balance" makes sense even for non-gravimetric applications if it is understood in the sense of a force
balance. At resonance, the force exerted upon the crystal by the sample is balanced by a force originating from the shear gradient inside the crystal. This is the essence of the small-load approximation.
Crystalline α–quartz is by far the most important material for thickness-shear resonators. Langasite
(La3Ga5SiO14, “LGS”) and
gallium-orthophosphate
(GaPO4) are investigated as alternatives to quartz, mainly (but not only) for use at high temperatures. Such devices are also called “QCM”, even though they are not made out of quartz (and may or may not be used for gravimetry).
(SH-SAW) devices, Love-wave
devices and torsional
resonators. Surface acoustic wave-based devices make use of the fact that the reflectivity of an acoustic wave at the crystal surface depends on the impedance
(the stress-to-speed ratio) of the adjacent medium. (Some acoustic sensors for temperature or pressure make use of the fact that the speed of sound inside the crystal depends on temperature, pressure, or bending. These sensors do not exploit surface effects.) In the context of surface-acoustic wave based sensing, the QCM is also termed “bulk acoustic wave resonator (BAW-resonator)” or “thickness-shear resonator”. The displacement pattern of an unloaded BAW resonator is a standing shear wave with anti-nodes
at the crystal surface. This makes the analysis particularly easy and transparent.
When the QCM was first developed, natural quartz was harvested, selected for its quality and then cut in the lab
. However, most of today’s crystals are grown using seed crystal
s. A seed crystal serves as an anchoring point and template for crystal growth. Grown crystals are subsequently cut and polished into hair-thin discs which support thickness shear resonance in the 1-30 MHz range. The "AT" or "SC" oriented cuts (discussed below) are widely used in applications.
boundary conditions well under control. Grounding the front electrode (the electrode in contact with the sample) is one option. A π-network sometimes is employed for the same reason. A π-network is an arrangement of resistor
s, which almost short-circuit
the two electrodes. This makes the device less susceptible to electrical perturbations.
would be radiated into the bulk and potentially be reflected back to the crystal from the opposing cell wall. Such reflections are avoided with transverse waves. The range of penetration of a 5 MHz-shear wave in water is 250 nm. This finite penetration depth renders the QCM surface-specific. Also, liquids and gases have a rather small shear-acoustic impedance and therefore only weakly damp the oscillation. The exceptionally high Q-factors of acoustic resonators are linked to their weak coupling to the environment.
. By fitting a resonance curve to the conductance curve, one obtains the frequency and bandwidth of the resonance as fit parameters. In ring-down, one measures the voltage between the electrodes after the exciting voltage has suddenly been turned off. The resonator emits a decaying sine wave
, where the resonance parameters are extracted from the period of oscillation and the decay rate.
s, typically indexed by the number of nodal planes parallel to the crystal surfaces. Only odd harmonic
s can be excited electrically because only these induce charges of opposite sign at the two crystal surfaces. Overtones are to be distinguished from anharmonic side bands (spurious modes), which have nodal planes perpendicular to the plane of the resonator. The best agreement between theory and experiment is reached with planar, optically polished crystals for overtone orders between n = 5 and n = 13. On low harmonics, energy trapping is insufficient, while on high harmonics, anharmonic side bands interfere with the main resonance.
of lateral displacement rarely exceeds a nanometer. More specifically one has
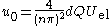
with u0 the amplitude of lateral displacement, n the overtone order, d the piezoelectric strain coefficient, Q the quality factor, and Uel the amplitude of electrical driving. The piezoelectric strain coefficient is given as d = 3.1·10‑12 m/V for AT-cut quartz crystals. Due to the small amplitude, stress
and strain
usually are proportional to each other. The QCM operates in the range of linear acoustics.
AT-cut crystals are singularly rotated Y-axis cuts in which the top and bottom half of the crystal move in opposite directions (thickness shear vibration) during oscillation.
The AT-cut crystal is easily manufactured. However, it has limitations at high and low temperature, as it is easily disrupted by internal stresses caused by temperature gradients in these temperature extremes (relative to room temperature, ~25 °C). These internal stress points produce undesirable frequency shifts in the crystal, decreasing its accuracy. The relationship between temperature and frequency is cubic
. The cubic relationship has an inflection point
near room temperature. As a consequence the AT-cut quartz crystal is most effective when operating at or near room temperature. For applications which are above room temperature, water cooling is often helpful.
Stress-compensated (SC) crystals are available with a doubly rotated cut that minimizes the frequency changes due to temperature gradients when the system is operating at high temperatures, and reduces the reliance on water cooling. SC-cut crystals have an inflection point of ~92 °C. In addition to their high temperature inflection point, they also have a smoother cubic relationship and are less affected by temperature deviations from the inflection point. However, due to the more difficult manufacturing process, they are more expensive and are not widely commercially available.
QCM (EQCM) is particularly advanced. Using the EQCM, one determines the ratio of mass deposited at the electrode surface during an electrochemical reaction to the total charge passed through the electrode. This ratio is called the current efficiency.
losses inside the electrode or the crystal. In the literature some parameters other than w itself are used to quantify bandwidth. The Q-factor (quality factor) is given by Q = fr/w. The “dissipation”, D, is the inverse of the Q-factor: D = Q−1 = w/fr. The half-band-half-width, Γ, is Γ = w/2. The use of Γ is motivated by a complex formulation of the equations governing the motion of the crystal. A complex
resonance frequency is defined as fr* = fr + iΓ, where the imaginary part, Γ, is half the bandwidth at half maximum. Using a complex notation, one can treat shifts of frequency, Δf, and bandwidth, ΔΓ, within the same set of (complex) equations.
The motional resistance of the resonator, R1, is also used as a measure of dissipation. R1 is an output parameter of some instruments based on advanced oscillator circuits. R1 usually is not strictly proportional to the bandwidth (although it should be according to the BvD circuit; see below). Also, in absolute terms, R1 – being an electrical quantity and not a frequency – is more severely affected by calibration
problems than the bandwidth.
ically equivalent to the continuum mechanics
description and to a description in terms of acoustic reflectivities. They provide for a graphical representation of the resonator’s properties and their shifts upon loading. These representations are not just cartoons. They are tools to predict the shift of the resonance parameters in response to the addition of the load.
Equivalent circuits build on the electromechanical analogy
. In the same way as the current through a network of resistors can predicted from their arrangement and the applied voltage, the displacement of a network of mechanical elements can predicted from the topology
of the network and the applied force. The electro-mechanical analogy maps forces onto voltages and speeds onto currents. The ratio of force and speed is termed “mechanical impedance
”. Note: Here, speed means the time derivative of a displacement, not the speed of sound. There also is an electro-acoustic analogy, within which stresses (rather than forces) are mapped onto voltages. In acoustics, forces are normalized to area. The ratio of stress and speed should not be called "acoustic impedance
" (in analogy to the mechanical impedance) because this term is already in use for the material property Zac = ρc with ρ the density
and c the speed of sound). The ratio of stress and speed at the crystal surface is called load impedance, ZL. Synonymous terms are "surface impedance" and "acoustic load." The load impedance is in general not equal to the material constant Zac = ρc = (Gρ)1/2. Only for propagating plain waves are the values of ZL and Zac the same.
The electro-mechanical analogy provides for mechanical equivalents of a resistor, an inductance
, and a capacitance
, which are the dashpot
(quantified by the drag coefficient
, ξp), the point mass (quantified by the mass, mp), and the spring
(quantified by the spring constant
, κp). For a dashpot, the impedance by definition is Zm=F / (du/dt)=ξm with F the force and (du/dt) the speed). For a point mass undergoing oscillatory motion u(t) = u0 exp(iωt) we have Zm = iωmp. The spring obeys Zm =κp/(iω). Piezoelectric coupling is depicted as a transformer
. It is characterized by a parameter φ. While φ is dimensionless for usual transformers (the ratio of the number of loops on both sides), it has the dimension charge/length in the case electromechanical coupling. The transformer acts as an impedance converter in the sense that a mechanical impedance, Zm, appears as an electrical impedance, Zel, across the electrical ports. Zel is given by Zel = φ2 Zm. For planar piezoelectric crystals, φ takes the value φ = Ae/dq, where A is the effective area, e is the piezoelectric stress coefficient (e = 9.65·102 C/m2 for AT-cut quartz) and dq is the thickness of the plate. The transformer often is not explicitly depicted. Rather, the mechanical elements are directly depicted as electrical elements (capacitor replaces a spring, etc.).
There is a pitfall with the application of the electro-mechanical analogy, which has to do with how networks are drawn. When a spring pulls onto a dashpot, one would usually draw the two elements in series. However, when applying the electro-mechanical analogy, the two elements have to be placed in parallel. For two parallel electrical elements the currents are additive. Since the speeds (= currents) add when placing a spring behind a dashpot, this assembly has to be represented by a parallel network.
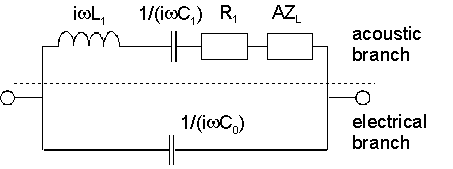The figure on the right shows the Butterworth-van Dyke (BvD) equivalent circuit. The acoustic properties of the crystal are represented by the motional inductance, L1, the motional capacitance, C1, and the motional resistance R1. ZL is the load impedance. Note that the load, ZL, cannot be determined from a single measurement. It is inferred from the comparison of the loaded and the unloaded state. Some authors use the BvD circuit without the load ZL. This circuit is also called “four element network”. The values of L1, C1, and R1 then change their value in the presence of the load (they do not if the element ZL is explicitly included).
ff is the frequency of the fundamental
. Zq is the acoustic impedance of material. For AT-cut quartz, its value is Zq = 8.8·106 kg m−2 s−1.
The small-load approximation is central to the interpretation of QCM-data. It holds for arbitrary samples and can be applied in an average sense. Assume that the sample is a complex material, such as a cell culture
, a sand pile, a froth, an assembly of spheres or vesicles
, or a droplet. If the average stress-to-speed ratio of the sample at the crystal surface (the load impedance, ZL) can be calculated in one way or another, a quantitative analysis of the QCM experiment is in reach. Otherwise, the interpretation will have to remain qualitative.
The limits of the small-load approximation are noticed either when the frequency shift is large or when the overtone-dependence of Δf and Δ(w/2) is analyzed in detail in order to derive the viscoelastic properties of the sample. A more general relation is
This equation is implicit
in Δf*, and must be solved numerically. Approximate solutions also exist, which go beyond the small-load approximation. The small-load approximation is the first order solution of a perturbation analysis
.
The definition of the load impedance implicitly assumes that stress and speed are proportional and that the ratio therefore is independent of speed. This assumption is justified when the crystal is operated in liquids and in air. The laws of linear acoustics then hold. However, when the crystal is in contact with a rough surface, stress can easily become a nonlinear function of strain (and speed) because the stress is transmitted across a finite number of rather small load-bearing asperities. The stress at the points of contact is high, and phenomena like slip, partial slip, yield, etc. set in. These are part of non-linear acoustics. There is a generalization of the small-load equation dealing with this problem. If the stress, σ(t), is periodic in time and synchronous with the crystal oscillation one has
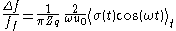
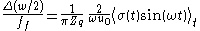
Angular brackets denote a time average and σ(t) is the (small) stress exerted by the external surface. The function σ(t) may or may not be harmonic. One can always test for nonlinear behavior by checking for a dependence of the resonance parameters on the driving voltage. If linear acoustics hold, there is no drive level-dependence. Note, however, that quartz crystals have an intrinsic drive level-dependence, which must not be confused with nonlinear interactions between the crystal and the sample.
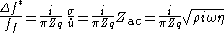
η’ and η’’ are the real and the imaginary part of the viscosity, respectively. Zac = ρc =(G ρ)1/2 is the acoustic impedance of the medium. ρ is the density, c, the speed of sound, and G = i ωη is the shear modulus.
For Newtonian liquids
(η’ = const, η’’ = 0), Δf and Δ(w/2) are equal and opposite. They scale as the square root of the overtone order, n1/2. For viscoelastic liquids (η’ = η(ω), η’’≠ 0), the complex viscosity can be obtained as
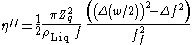
Importantly, the QCM only probes the region close to the crystal surface. The shear wave evanescently decays into the liquid. In water the penetration depth is about 250 nm at 5 MHz. Surface roughness, nano-bubbles at the surface, slip, and compressional waves can interfere with the measurement of viscosity. Also, the viscosity determined at MHz frequencies sometimes differs from the low-frequency viscosity. In this respect, torsional resonators (with a frequency around 100 kHz) are closer to application than thickness-shear resonators.
, which implies σ = -ω2u0mF, where u0 is the amplitude of oscillation and mF is the (average) mass per unit area. Inserting this result into the small-load-approximation one finds
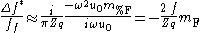
If the density of the film is known, one can convert from mass per unit area, mF, to thickness, dF. The thickness thus derived is also called the Sauerbrey thickness to show that it was derived by applying the Sauerbrey equation to the frequency shift.
The shift in bandwidth is zero if the Sauerbrey equation holds. Checking for the bandwidth therefore amounts to checking the applicability of the Sauerbrey equation.
The Sauerbrey equation was first derived by G. Sauerbrey in 1959 and correlates changes in the oscillation frequency of a piezoelectric crystal with mass deposited on it. He simultaneously developed a method for measuring the resonance frequency and its changes by using the crystal as the frequency-determining component of an oscillator circuit. His method continues to be used as the primary tool in quartz crystal microbalance experiments for conversion of frequency to mass.
Because the film is treated as an extension of thickness, Sauerbrey’s equation only applies to systems in which (a) the deposited mass has the same acoustic properties as the crystal and (b) the frequency change is small (Δf / f < 0.05).
If the change in frequency is greater than 5%, that is, Δf / f > 0.05, the Z-match method must be used to determine the change in mass. The formula for the Z-match method is:
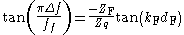
kF is the wave vector inside the film and dF its thickness. Inserting kF = 2·π·f /cF = 2·π·f·ρF / ZF as well as dF = mF / ρF yields
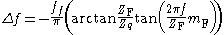
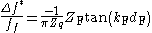
Here ZF is the acoustic impedance of the film (ZF = ρFcF = (ρFGf)1/2)= (ρF/Jf)1/2), kF is the wave vector and dF is the film thickness. Jf is the film's viscoelastic compliance, ρF is the density.
The poles of the tangent (kF dF = π/2) define the film resonances. At the film resonance, one has dF = λ/4. The agreement between experiment and theory is often poor close to the film resonance. Typically, the QCM only works well for film thicknesses much less than a quarter of the wavelength of sound (corresponding to a few micrometres, depending on the softness of the film and the overtone order).
Note that the properties of a film as determined with the QCM are fully specified by two parameters, which are its acoustic impedance, ZF = ρFcF and its mass per unit area, mF = dF/ρF. The wave number kF = ω/cF is not algebraically independent from ZF and mF. Unless the density of the film is known independently, the QCM can only measure mass per unit area, never the geometric thickness itself.
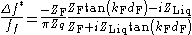
The indices F and Liq denote the film and the liquid. Here, the reference state is the crystal immersed in liquid (but not covered with a film). For thin films, one can Taylor-expand
the above equation to first order in dF, yielding
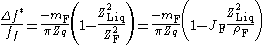
Apart from the term in brackets, this equation is equivalent to the Sauerbrey equation. The term in brackets is a viscoelastic correction, dealing with the fact that in liquids, soft layers lead to a smaller Sauerbrey thickness than rigid layers.
For thin films in liquids, there is an approximate analytical result, relating the elastic compliance of the film, JF’ to the ratio of Δ(w/2); and Δf. The shear compliance is the inverse of the shear modulus, G. In the thin-film limit, the ratio of Δ(w/2) and –Δf is independent of film thickness. It is an intrinsic property of the film. One has
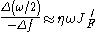
For thin films in air an analogous analytical result is
Here J’’ is the viscous shear compliance.
a) The QCM always measures an areal mass density, never a geometric thickness. The conversion from areal mass density to thickness usually requires the physical density as an independent input.
b) It is difficult to infer the viscoelastic correction factor from QCM data. However, if the correction factor differs significantly from unity, it may be expected that it affects the bandwidth Δ(w/2) and also that it depends on overtone order. If, conversely, such effects are absent (Δ(w/2) « Δf, Sauerbrey thickness same on all overtone orders) one may assume that (1-ZLiq2/ZF2)≈1.
c) Complex samples are often laterally heterogeneous.
d) Complex samples often have fuzzy interfaces. A "fluffy" interface will often lead to a viscoelastic correction and, as a consequence, to a non-zero Δ(w/2) as well as an overtone-dependent Sauerbrey mass. In the absence of such effects, one may conclude that the outer interface of film is sharp.
e) When the viscoelastic correction, as discussed in (b), is insignificant, this does by no means imply that the film is not swollen by the solvent
. It only means that the (swollen) film is much more rigid than the ambient liquid. QCM data taken on the wet sample alone do not allow inference of the degree of swelling. The amount of swelling can be inferred from the comparison of the wet and the dry thickness. The degree of swelling is also accessible by comparing the acoustic thickness (in the Sauerbrey sense) to the optical thickness as determined by, for example, surface plasmon resonance (SPR) spectroscopy or ellipsometry. Solvent contained in the film usually does contribute to the acoustic thickness (because it takes part in the movement), whereas it does not contribute to the optic thickness (because the electronic polarizability
of a solvent molecule does not change when it is located inside a film).
Often, the external object is so heavy that it does not take part in the MHz oscillation of the crystal due to inertia. It then rests in place in the laboratory frame. When the crystal surface is laterally displaced, the contact exerts a restoring force upon the crystal surface. The stress is proportional to the number density of the contacts, NS, and their average spring constant, κS. The spring constant may be complex (κS* = κS’ + iκS’’), where the imaginary part quantifies a withdrawal of energy from the crystal oscillation (for instance due to viscoelastic effects). For such a situation, the small-load approximation predicts
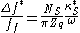
The QCM allows for non-destructive testing of the shear stiffness of multi-asperity contacts.
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
of a quartz crystal resonator. The resonance
Resonance
In physics, resonance is the tendency of a system to oscillate at a greater amplitude at some frequencies than at others. These are known as the system's resonant frequencies...
is disturbed by the addition or removal of a small mass due to oxide growth/decay or film deposition at the surface of the acoustic resonator. The QCM can be used under vacuum, in gas phase
Phase (matter)
In the physical sciences, a phase is a region of space , throughout which all physical properties of a material are essentially uniform. Examples of physical properties include density, index of refraction, and chemical composition...
("gas sensor", first use described by King) and more recently in liquid environments. It is useful for monitoring the rate of deposition in thin film deposition systems under vacuum. In liquid, it is highly effective at determining the affinity
Chemical affinity
In chemical physics and physical chemistry, chemical affinity is the electronic property by which dissimilar chemical species are capable of forming chemical compounds...
of molecules (protein
Protein
Proteins are biochemical compounds consisting of one or more polypeptides typically folded into a globular or fibrous form, facilitating a biological function. A polypeptide is a single linear polymer chain of amino acids bonded together by peptide bonds between the carboxyl and amino groups of...
s, in particular) to surfaces functionalized with recognition sites. Larger entities such as virus
Virus
A virus is a small infectious agent that can replicate only inside the living cells of organisms. Viruses infect all types of organisms, from animals and plants to bacteria and archaea...
es or polymer
Polymer
A polymer is a large molecule composed of repeating structural units. These subunits are typically connected by covalent chemical bonds...
s are investigated, as well. QCM has also been used to investigate interactions between biomolecules. Frequency measurements are easily made to high precision (discussed below); hence, it is easy to measure mass densities down to a level of below 1 μg/cm2. In addition to measuring the frequency, the dissipation
Dissipation
In physics, dissipation embodies the concept of a dynamical system where important mechanical models, such as waves or oscillations, lose energy over time, typically from friction or turbulence. The lost energy converts into heat, which raises the temperature of the system. Such systems are called...
is often measured to help analysis. The dissipation is a parameter quantifying the damping
Damping
In physics, damping is any effect that tends to reduce the amplitude of oscillations in an oscillatory system, particularly the harmonic oscillator.In mechanics, friction is one such damping effect...
in the system, and is related to the sample's viscoelastic
Viscoelasticity
Viscoelasticity is the property of materials that exhibit both viscous and elastic characteristics when undergoing deformation. Viscous materials, like honey, resist shear flow and strain linearly with time when a stress is applied. Elastic materials strain instantaneously when stretched and just...
properties. The dissipation is equal to the ratio of bandwidth, w, (see below) and frequency, f.
General
QuartzQuartz
Quartz is the second-most-abundant mineral in the Earth's continental crust, after feldspar. It is made up of a continuous framework of SiO4 silicon–oxygen tetrahedra, with each oxygen being shared between two tetrahedra, giving an overall formula SiO2. There are many different varieties of quartz,...
is one member of a family of crystals that experience the piezoelectric effect
Piezoelectricity
Piezoelectricity is the charge which accumulates in certain solid materials in response to applied mechanical stress. The word piezoelectricity means electricity resulting from pressure...
. The piezoelectric effect has found applications in high power sources, sensors, actuators, frequency standards, motors, etc., and the relationship between applied voltage
Voltage
Voltage, otherwise known as electrical potential difference or electric tension is the difference in electric potential between two points — or the difference in electric potential energy per unit charge between two points...
and mechanical deformation is well known; this allows probing an acoustic resonance by electrical means. Applying alternating current to the quartz crystal will induce oscillations. With an alternating current between the electrodes of a properly cut crystal, a standing shear wave is generated. The Q factor
Q factor
In physics and engineering the quality factor or Q factor is a dimensionless parameter that describes how under-damped an oscillator or resonator is, or equivalently, characterizes a resonator's bandwidth relative to its center frequency....
, which is the ratio of frequency and bandwidth, can be as high as 106. Such a narrow resonance leads to highly stable oscillators and a high accuracy in the determination of the resonance frequency. The QCM exploits this ease and precision for sensing. Common equipment allows resolution down to 1 Hz on crystals with a fundamental resonant frequency in the 4 – 6 MHz range. A typical setup for the QCM contains water cooling tubes, the retaining unit, frequency sensing equipment through a microdot feed-through, an oscillation source, and a measurement and recording device.
The frequency of oscillation of the quartz crystal is partially dependent on the thickness of the crystal. During normal operation, all the other influencing variables remain constant; thus a change in thickness correlates directly to a change in frequency. As mass is deposited on the surface of the crystal, the thickness increases; consequently the frequency of oscillation decreases from the initial value. With some simplifying assumptions, this frequency change can be quantified and correlated precisely to the mass change using Sauerbrey's equation
Sauerbrey equation
The Sauerbrey equation was developed by G. Sauerbrey in 1959 as a method for correlating changes in the oscillation frequency of a piezoelectric crystal with the mass deposited on it. He simultaneously developed a method for measuring the characteristic frequency and its changes by using the...
.
Other techniques for measuring the properties of thin films include Ellipsometry
Ellipsometry
Ellipsometry is an optical technique for the investigation of the dielectric properties of thin films....
, Surface Plasmon Resonance
Surface plasmon resonance
The excitation of surface plasmons by light is denoted as a surface plasmon resonance for planar surfaces or localized surface plasmon resonance for nanometer-sized metallic structures....
(SPR) Spectroscopy
Spectroscopy
Spectroscopy is the study of the interaction between matter and radiated energy. Historically, spectroscopy originated through the study of visible light dispersed according to its wavelength, e.g., by a prism. Later the concept was expanded greatly to comprise any interaction with radiative...
, and Dual Polarisation Interferometry
Dual Polarisation Interferometry
Dual polarization interferometry is an analytical technique that can probe molecular scale layers adsorbed to the surface of a waveguide by using the evanescent wave of a laser beam confined to the waveguide...
.
Gravimetric and non-gravimetric QCM
The classical sensing application of quartz crystal resonators is microgravimetry. Many commercial instruments, some of which are called thickness monitorsThin-film thickness monitor
Thin-film thickness monitors, deposition rate controllers, and so on, are a family of instruments used in high and ultra-high vacuum systems. They can measure the thickness of a thin film, not only after it has been made, but while it is still being deposited, and some can control either the final...
, are available. These devices exploit the Sauerbrey relation. For thin films, the resonance frequency is – by-and-large – inversely proportional to the total thickness of the plate. The latter increases when a film is deposited onto the crystal surface. Monolayer
Monolayer
- Chemistry :A Langmuir monolayer or insoluble monolayer is a one-molecule thick layer of an insoluble organic material spread onto an aqueous subphase. Traditional compounds used to prepare Langmuir monolayers are amphiphilic materials that possess a hydrophilic headgroup and a hydrophobic tail...
sensitivity is easily reached. However, when the film thickness increases, viscoelastic effects come into play. In the late 80’s, it was recognized that the QCM can also be operated in liquids, if proper measures are taken to overcome the consequences of the large damping. Again, viscoelastic effects contribute strongly to the resonance properties.
Today, microweighing is one of several uses of the QCM.
Measurements of viscosity
Viscosity
Viscosity is a measure of the resistance of a fluid which is being deformed by either shear or tensile stress. In everyday terms , viscosity is "thickness" or "internal friction". Thus, water is "thin", having a lower viscosity, while honey is "thick", having a higher viscosity...
and more general, viscoelastic properties, are of much importance as well. The “non-gravimetric” QCM is by no means an alternative to the conventional QCM. Many researchers, who use quartz resonators for purposes other than gravimetry, have continued to call the quartz crystal resonator “QCM”. Actually, the term "balance" makes sense even for non-gravimetric applications if it is understood in the sense of a force
Force
In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...
balance. At resonance, the force exerted upon the crystal by the sample is balanced by a force originating from the shear gradient inside the crystal. This is the essence of the small-load approximation.
Crystalline α–quartz is by far the most important material for thickness-shear resonators. Langasite
Lanthanum gallium silicate
Lanthanum gallium silicate , also known as langasite, has a chemical formula of the form A3BC3D2O14, where A, B, C and D indicate particular cation sites. A is a decahedral site coordinated by 8 oxygen atoms...
(La3Ga5SiO14, “LGS”) and
gallium-orthophosphate
Gallium phosphate
Gallium phosphate is a colorless trigonal crystal with a hardness of 5.5 on the Mohs scale. GaPO4 is isotypic with quartz, possessing very similar properties, but the silicon atoms are alternately substituted with gallium and phosphorus, thereby doubling the piezoelectric effect...
(GaPO4) are investigated as alternatives to quartz, mainly (but not only) for use at high temperatures. Such devices are also called “QCM”, even though they are not made out of quartz (and may or may not be used for gravimetry).
Surface acoustic wave-based sensors
The QCM is a member of a wider class of sensing instruments based on acoustic waves at surfaces. Instruments sharing similar principles of operation are shear horizontal surface acoustic waveSurface acoustic wave
]A surface acoustic wave is an acoustic wave traveling along the surface of a material exhibiting elasticity, with an amplitude that typically decays exponentially with depth into the substrate.-Discovery:...
(SH-SAW) devices, Love-wave
Love wave
In elastodynamics, Love waves are horizontally polarized shear waves guided by an elastic layer, which is "welded" to an elastic half space on one side while bordering a vacuum on the other side...
devices and torsional
Torsion (mechanics)
In solid mechanics, torsion is the twisting of an object due to an applied torque. In sections perpendicular to the torque axis, the resultant shear stress in this section is perpendicular to the radius....
resonators. Surface acoustic wave-based devices make use of the fact that the reflectivity of an acoustic wave at the crystal surface depends on the impedance
Mechanical impedance
Mechanical impedance is a measure of how much a structure resists motion when subjected to a given force. It relates forces with velocities acting on a mechanical system. The mechanical impedance of a point on a structure is the ratio of the force applied at a point to the resulting velocity at...
(the stress-to-speed ratio) of the adjacent medium. (Some acoustic sensors for temperature or pressure make use of the fact that the speed of sound inside the crystal depends on temperature, pressure, or bending. These sensors do not exploit surface effects.) In the context of surface-acoustic wave based sensing, the QCM is also termed “bulk acoustic wave resonator (BAW-resonator)” or “thickness-shear resonator”. The displacement pattern of an unloaded BAW resonator is a standing shear wave with anti-nodes
Node (physics)
A node is a point along a standing wave where the wave has minimal amplitude. For instance, in a vibrating guitar string, the ends of the string are nodes. By changing the position of the end node through frets, the guitarist changes the effective length of the vibrating string and thereby the...
at the crystal surface. This makes the analysis particularly easy and transparent.
Resonator crystals
Laboratory
A laboratory is a facility that provides controlled conditions in which scientific research, experiments, and measurement may be performed. The title of laboratory is also used for certain other facilities where the processes or equipment used are similar to those in scientific laboratories...
. However, most of today’s crystals are grown using seed crystal
Seed crystal
A seed crystal is a small piece of single crystal/polycrystal material from which a large crystal of the same material typically is to be grown...
s. A seed crystal serves as an anchoring point and template for crystal growth. Grown crystals are subsequently cut and polished into hair-thin discs which support thickness shear resonance in the 1-30 MHz range. The "AT" or "SC" oriented cuts (discussed below) are widely used in applications.
Electromechanical coupling
The QCM consists of a thin piezoelectric plate with electrodes evaporated onto both sides. Due to the piezo-effect, an AC voltage across the electrodes induces a shear deformation and vice versa. The electromechanical coupling provides a simple way to detect an acoustic resonance by electrical means. Otherwise, it is of minor importance. However, electromechanical coupling can have a slight influence on the resonance frequency via piezoelectric stiffening. This effect can be used for sensing, but is usually avoided. It is essential to have the electric and dielectricDielectric
A dielectric is an electrical insulator that can be polarized by an applied electric field. When a dielectric is placed in an electric field, electric charges do not flow through the material, as in a conductor, but only slightly shift from their average equilibrium positions causing dielectric...
boundary conditions well under control. Grounding the front electrode (the electrode in contact with the sample) is one option. A π-network sometimes is employed for the same reason. A π-network is an arrangement of resistor
Resistor
A linear resistor is a linear, passive two-terminal electrical component that implements electrical resistance as a circuit element.The current through a resistor is in direct proportion to the voltage across the resistor's terminals. Thus, the ratio of the voltage applied across a resistor's...
s, which almost short-circuit
Short circuit
A short circuit in an electrical circuit that allows a current to travel along an unintended path, often where essentially no electrical impedance is encountered....
the two electrodes. This makes the device less susceptible to electrical perturbations.
Shear waves decay in liquids and gases
Most acoustic-wave-based sensors employ shear (transverse) waves. Shear waves decay rapidly in liquid and gaseous environments. Compressional (longitudinal) wavesLongitudinal wave
Longitudinal waves, as known as "l-waves", are waves that have the same direction of vibration as their direction of travel, which means that the movement of the medium is in the same direction as or the opposite direction to the motion of the wave. Mechanical longitudinal waves have been also...
would be radiated into the bulk and potentially be reflected back to the crystal from the opposing cell wall. Such reflections are avoided with transverse waves. The range of penetration of a 5 MHz-shear wave in water is 250 nm. This finite penetration depth renders the QCM surface-specific. Also, liquids and gases have a rather small shear-acoustic impedance and therefore only weakly damp the oscillation. The exceptionally high Q-factors of acoustic resonators are linked to their weak coupling to the environment.
Modes of operation
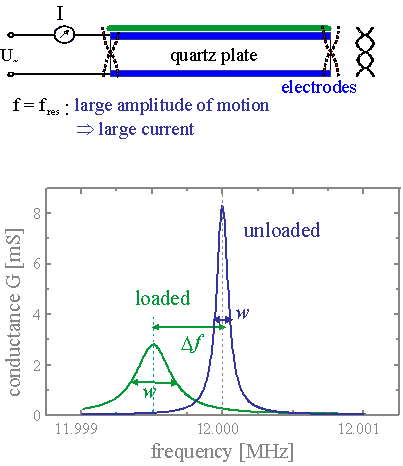
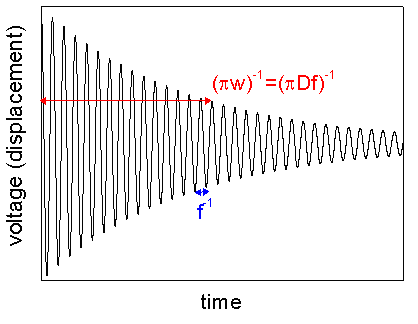
Economic ways of driving a QCM make use of oscillator circuits. Oscillator circuits are also widely employed in time and frequency control applications, where the oscillator serves as a clock. Other modes of operation are impedance analysis and ring-down. In impedance analysis, the electric conductance as a function of driving frequency is determined by means of a network analyzerNetwork analyzer (electrical)
A network analyzer is an instrument that measures the network parameters of electrical networks. Today, network analyzers commonly measure s–parameters because reflection and transmission of electrical networks are easy to measure at high frequencies, but there are other network parameter...
. By fitting a resonance curve to the conductance curve, one obtains the frequency and bandwidth of the resonance as fit parameters. In ring-down, one measures the voltage between the electrodes after the exciting voltage has suddenly been turned off. The resonator emits a decaying sine wave
Sine wave
The sine wave or sinusoid is a mathematical function that describes a smooth repetitive oscillation. It occurs often in pure mathematics, as well as physics, signal processing, electrical engineering and many other fields...
, where the resonance parameters are extracted from the period of oscillation and the decay rate.
Energy trapping
The electrodes at the front and the back of the crystal usually are key-hole shaped, thereby making the resonator thicker in the center than at the rim. This confines the displacement field to the center of the crystal by a mechanism called energy trapping. The crystal turns into an acoustic lens and the wave is focused to the center of the crystal. Energy trapping is necessary in order to be able to mount the crystal at the edge without excessive damping. Energy trapping slightly distorts the otherwise planar wave fronts. The deviation from the plane thickness-shear mode entails flexural contribution to the displacement pattern. Flexural waves emit compressional waves into the adjacent medium, which is a problem when operating the crystal in a liquid environment.Overtones
Planar resonators can be operated at a number of overtoneOvertone
An overtone is any frequency higher than the fundamental frequency of a sound. The fundamental and the overtones together are called partials. Harmonics are partials whose frequencies are whole number multiples of the fundamental These overlapping terms are variously used when discussing the...
s, typically indexed by the number of nodal planes parallel to the crystal surfaces. Only odd harmonic
Harmonic
A harmonic of a wave is a component frequency of the signal that is an integer multiple of the fundamental frequency, i.e. if the fundamental frequency is f, the harmonics have frequencies 2f, 3f, 4f, . . . etc. The harmonics have the property that they are all periodic at the fundamental...
s can be excited electrically because only these induce charges of opposite sign at the two crystal surfaces. Overtones are to be distinguished from anharmonic side bands (spurious modes), which have nodal planes perpendicular to the plane of the resonator. The best agreement between theory and experiment is reached with planar, optically polished crystals for overtone orders between n = 5 and n = 13. On low harmonics, energy trapping is insufficient, while on high harmonics, anharmonic side bands interfere with the main resonance.
Amplitude of motion
The amplitudeAmplitude
Amplitude is the magnitude of change in the oscillating variable with each oscillation within an oscillating system. For example, sound waves in air are oscillations in atmospheric pressure and their amplitudes are proportional to the change in pressure during one oscillation...
of lateral displacement rarely exceeds a nanometer. More specifically one has
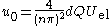
with u0 the amplitude of lateral displacement, n the overtone order, d the piezoelectric strain coefficient, Q the quality factor, and Uel the amplitude of electrical driving. The piezoelectric strain coefficient is given as d = 3.1·10‑12 m/V for AT-cut quartz crystals. Due to the small amplitude, stress
Stress (physics)
In continuum mechanics, stress is a measure of the internal forces acting within a deformable body. Quantitatively, it is a measure of the average force per unit area of a surface within the body on which internal forces act. These internal forces are a reaction to external forces applied on the body...
and strain
Strain (materials science)
In continuum mechanics, the infinitesimal strain theory, sometimes called small deformation theory, small displacement theory, or small displacement-gradient theory, deals with infinitesimal deformations of a continuum body...
usually are proportional to each other. The QCM operates in the range of linear acoustics.
Effects of temperature and stress
The resonance frequency of acoustic resonators depends on temperature, pressure, and bending stress. Temperature-frequency coupling is minimized by employing special crystal cuts. A widely used temperature-compensated cut of quartz is the AT-cut. Careful control of temperature and stress is essential in the operation of the QCM.AT-cut crystals are singularly rotated Y-axis cuts in which the top and bottom half of the crystal move in opposite directions (thickness shear vibration) during oscillation.
The AT-cut crystal is easily manufactured. However, it has limitations at high and low temperature, as it is easily disrupted by internal stresses caused by temperature gradients in these temperature extremes (relative to room temperature, ~25 °C). These internal stress points produce undesirable frequency shifts in the crystal, decreasing its accuracy. The relationship between temperature and frequency is cubic
Cubic function
In mathematics, a cubic function is a function of the formf=ax^3+bx^2+cx+d,\,where a is nonzero; or in other words, a polynomial of degree three. The derivative of a cubic function is a quadratic function...
. The cubic relationship has an inflection point
Inflection point
In differential calculus, an inflection point, point of inflection, or inflection is a point on a curve at which the curvature or concavity changes sign. The curve changes from being concave upwards to concave downwards , or vice versa...
near room temperature. As a consequence the AT-cut quartz crystal is most effective when operating at or near room temperature. For applications which are above room temperature, water cooling is often helpful.
Stress-compensated (SC) crystals are available with a doubly rotated cut that minimizes the frequency changes due to temperature gradients when the system is operating at high temperatures, and reduces the reliance on water cooling. SC-cut crystals have an inflection point of ~92 °C. In addition to their high temperature inflection point, they also have a smoother cubic relationship and are less affected by temperature deviations from the inflection point. However, due to the more difficult manufacturing process, they are more expensive and are not widely commercially available.
Electrochemical QCM
The QCM can be combined with other surface-analytical instruments. The electrochemicalElectrochemistry
Electrochemistry is a branch of chemistry that studies chemical reactions which take place in a solution at the interface of an electron conductor and an ionic conductor , and which involve electron transfer between the electrode and the electrolyte or species in solution.If a chemical reaction is...
QCM (EQCM) is particularly advanced. Using the EQCM, one determines the ratio of mass deposited at the electrode surface during an electrochemical reaction to the total charge passed through the electrode. This ratio is called the current efficiency.
Quantification of dissipative processes
For advanced QCMs, both the resonance frequency, fr, and the bandwidth, w, are available for analysis. The latter quantifies processes which withdraw energy from the oscillation. These may include damping by the holder and ohmicOhm's law
Ohm's law states that the current through a conductor between two points is directly proportional to the potential difference across the two points...
losses inside the electrode or the crystal. In the literature some parameters other than w itself are used to quantify bandwidth. The Q-factor (quality factor) is given by Q = fr/w. The “dissipation”, D, is the inverse of the Q-factor: D = Q−1 = w/fr. The half-band-half-width, Γ, is Γ = w/2. The use of Γ is motivated by a complex formulation of the equations governing the motion of the crystal. A complex
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
resonance frequency is defined as fr* = fr + iΓ, where the imaginary part, Γ, is half the bandwidth at half maximum. Using a complex notation, one can treat shifts of frequency, Δf, and bandwidth, ΔΓ, within the same set of (complex) equations.
The motional resistance of the resonator, R1, is also used as a measure of dissipation. R1 is an output parameter of some instruments based on advanced oscillator circuits. R1 usually is not strictly proportional to the bandwidth (although it should be according to the BvD circuit; see below). Also, in absolute terms, R1 – being an electrical quantity and not a frequency – is more severely affected by calibration
Calibration
Calibration is a comparison between measurements – one of known magnitude or correctness made or set with one device and another measurement made in as similar a way as possible with a second device....
problems than the bandwidth.
Equivalent circuits
Modeling of acoustic resonators often occurs with equivalent electrical circuits. Equivalent circuits are algebraAlgebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
ically equivalent to the continuum mechanics
Continuum mechanics
Continuum mechanics is a branch of mechanics that deals with the analysis of the kinematics and the mechanical behavior of materials modelled as a continuous mass rather than as discrete particles...
description and to a description in terms of acoustic reflectivities. They provide for a graphical representation of the resonator’s properties and their shifts upon loading. These representations are not just cartoons. They are tools to predict the shift of the resonance parameters in response to the addition of the load.
Equivalent circuits build on the electromechanical analogy
Analogy
Analogy is a cognitive process of transferring information or meaning from a particular subject to another particular subject , and a linguistic expression corresponding to such a process...
. In the same way as the current through a network of resistors can predicted from their arrangement and the applied voltage, the displacement of a network of mechanical elements can predicted from the topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
of the network and the applied force. The electro-mechanical analogy maps forces onto voltages and speeds onto currents. The ratio of force and speed is termed “mechanical impedance
Mechanical impedance
Mechanical impedance is a measure of how much a structure resists motion when subjected to a given force. It relates forces with velocities acting on a mechanical system. The mechanical impedance of a point on a structure is the ratio of the force applied at a point to the resulting velocity at...
”. Note: Here, speed means the time derivative of a displacement, not the speed of sound. There also is an electro-acoustic analogy, within which stresses (rather than forces) are mapped onto voltages. In acoustics, forces are normalized to area. The ratio of stress and speed should not be called "acoustic impedance
Acoustic impedance
The acoustic impedance at a particular frequency indicates how much sound pressure is generated by a given air vibration at that frequency. The acoustic impedance Z is frequency dependent and is very useful, for example, for describing the behaviour of musical wind instruments...
" (in analogy to the mechanical impedance) because this term is already in use for the material property Zac = ρc with ρ the density
Density
The mass density or density of a material is defined as its mass per unit volume. The symbol most often used for density is ρ . In some cases , density is also defined as its weight per unit volume; although, this quantity is more properly called specific weight...
and c the speed of sound). The ratio of stress and speed at the crystal surface is called load impedance, ZL. Synonymous terms are "surface impedance" and "acoustic load." The load impedance is in general not equal to the material constant Zac = ρc = (Gρ)1/2. Only for propagating plain waves are the values of ZL and Zac the same.
The electro-mechanical analogy provides for mechanical equivalents of a resistor, an inductance
Inductance
In electromagnetism and electronics, inductance is the ability of an inductor to store energy in a magnetic field. Inductors generate an opposing voltage proportional to the rate of change in current in a circuit...
, and a capacitance
Capacitance
In electromagnetism and electronics, capacitance is the ability of a capacitor to store energy in an electric field. Capacitance is also a measure of the amount of electric potential energy stored for a given electric potential. A common form of energy storage device is a parallel-plate capacitor...
, which are the dashpot
Dashpot
A dashpot is a mechanical device, a damper which resists motion via viscous friction. The resulting force is proportional to the velocity, but acts in the opposite direction, slowing the motion and absorbing energy. It is commonly used in conjunction with a spring...
(quantified by the drag coefficient
Drag coefficient
In fluid dynamics, the drag coefficient is a dimensionless quantity that is used to quantify the drag or resistance of an object in a fluid environment such as air or water. It is used in the drag equation, where a lower drag coefficient indicates the object will have less aerodynamic or...
, ξp), the point mass (quantified by the mass, mp), and the spring
Spring (device)
A spring is an elastic object used to store mechanical energy. Springs are usually made out of spring steel. Small springs can be wound from pre-hardened stock, while larger ones are made from annealed steel and hardened after fabrication...
(quantified by the spring constant
Hooke's law
In mechanics, and physics, Hooke's law of elasticity is an approximation that states that the extension of a spring is in direct proportion with the load applied to it. Many materials obey this law as long as the load does not exceed the material's elastic limit. Materials for which Hooke's law...
, κp). For a dashpot, the impedance by definition is Zm=F / (du/dt)=ξm with F the force and (du/dt) the speed). For a point mass undergoing oscillatory motion u(t) = u0 exp(iωt) we have Zm = iωmp. The spring obeys Zm =κp/(iω). Piezoelectric coupling is depicted as a transformer
Transformer
A transformer is a device that transfers electrical energy from one circuit to another through inductively coupled conductors—the transformer's coils. A varying current in the first or primary winding creates a varying magnetic flux in the transformer's core and thus a varying magnetic field...
. It is characterized by a parameter φ. While φ is dimensionless for usual transformers (the ratio of the number of loops on both sides), it has the dimension charge/length in the case electromechanical coupling. The transformer acts as an impedance converter in the sense that a mechanical impedance, Zm, appears as an electrical impedance, Zel, across the electrical ports. Zel is given by Zel = φ2 Zm. For planar piezoelectric crystals, φ takes the value φ = Ae/dq, where A is the effective area, e is the piezoelectric stress coefficient (e = 9.65·102 C/m2 for AT-cut quartz) and dq is the thickness of the plate. The transformer often is not explicitly depicted. Rather, the mechanical elements are directly depicted as electrical elements (capacitor replaces a spring, etc.).
There is a pitfall with the application of the electro-mechanical analogy, which has to do with how networks are drawn. When a spring pulls onto a dashpot, one would usually draw the two elements in series. However, when applying the electro-mechanical analogy, the two elements have to be placed in parallel. For two parallel electrical elements the currents are additive. Since the speeds (= currents) add when placing a spring behind a dashpot, this assembly has to be represented by a parallel network.
Small-load approximation
The BvD circuit predicts the resonance parameters. One can show that the following simple relation holds as long as the frequency shift is much smaller than the frequency itself:ff is the frequency of the fundamental
Fundamental frequency
The fundamental frequency, often referred to simply as the fundamental and abbreviated f0, is defined as the lowest frequency of a periodic waveform. In terms of a superposition of sinusoids The fundamental frequency, often referred to simply as the fundamental and abbreviated f0, is defined as the...
. Zq is the acoustic impedance of material. For AT-cut quartz, its value is Zq = 8.8·106 kg m−2 s−1.
The small-load approximation is central to the interpretation of QCM-data. It holds for arbitrary samples and can be applied in an average sense. Assume that the sample is a complex material, such as a cell culture
Cell culture
Cell culture is the complex process by which cells are grown under controlled conditions. In practice, the term "cell culture" has come to refer to the culturing of cells derived from singlecellular eukaryotes, especially animal cells. However, there are also cultures of plants, fungi and microbes,...
, a sand pile, a froth, an assembly of spheres or vesicles
Vesicle (biology)
A vesicle is a bubble of liquid within another liquid, a supramolecular assembly made up of many different molecules. More technically, a vesicle is a small membrane-enclosed sack that can store or transport substances. Vesicles can form naturally because of the properties of lipid membranes , or...
, or a droplet. If the average stress-to-speed ratio of the sample at the crystal surface (the load impedance, ZL) can be calculated in one way or another, a quantitative analysis of the QCM experiment is in reach. Otherwise, the interpretation will have to remain qualitative.
The limits of the small-load approximation are noticed either when the frequency shift is large or when the overtone-dependence of Δf and Δ(w/2) is analyzed in detail in order to derive the viscoelastic properties of the sample. A more general relation is
This equation is implicit
Implicit function
The implicit function theorem provides a link between implicit and explicit functions. It states that if the equation R = 0 satisfies some mild conditions on its partial derivatives, then one can in principle solve this equation for y, at least over some small interval...
in Δf*, and must be solved numerically. Approximate solutions also exist, which go beyond the small-load approximation. The small-load approximation is the first order solution of a perturbation analysis
Perturbation theory
Perturbation theory comprises mathematical methods that are used to find an approximate solution to a problem which cannot be solved exactly, by starting from the exact solution of a related problem...
.
The definition of the load impedance implicitly assumes that stress and speed are proportional and that the ratio therefore is independent of speed. This assumption is justified when the crystal is operated in liquids and in air. The laws of linear acoustics then hold. However, when the crystal is in contact with a rough surface, stress can easily become a nonlinear function of strain (and speed) because the stress is transmitted across a finite number of rather small load-bearing asperities. The stress at the points of contact is high, and phenomena like slip, partial slip, yield, etc. set in. These are part of non-linear acoustics. There is a generalization of the small-load equation dealing with this problem. If the stress, σ(t), is periodic in time and synchronous with the crystal oscillation one has
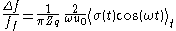
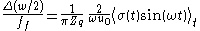
Angular brackets denote a time average and σ(t) is the (small) stress exerted by the external surface. The function σ(t) may or may not be harmonic. One can always test for nonlinear behavior by checking for a dependence of the resonance parameters on the driving voltage. If linear acoustics hold, there is no drive level-dependence. Note, however, that quartz crystals have an intrinsic drive level-dependence, which must not be confused with nonlinear interactions between the crystal and the sample.
Assumptions
For a number of experimental configurations, there are explicit expressions relating the shifts of frequency and bandwidth to the sample properties. The assumptions underlying the equations are the following:- The resonator and all cover layers are laterally homogeneous and infinite.
- The distortion of the crystal is given by a transverse plane wave with the wave-vectorWave vectorIn physics, a wave vector is a vector which helps describe a wave. Like any vector, it has a magnitude and direction, both of which are important: Its magnitude is either the wavenumber or angular wavenumber of the wave , and its direction is ordinarily the direction of wave propagation In...
perpendicular to the surface normal (thickness-shear mode). There are neither compressional waves nor flexural contributions to the displacement pattern. There are no nodal lines in the plane of the resonator. - All stresses are proportional to strain. Linear viscoelasticity holds.
- Piezoelectric stiffening may be ignored.
Semi-infinite viscoelastic medium
For a semi-infinite medium, one has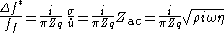η’ and η’’ are the real and the imaginary part of the viscosity, respectively. Zac = ρc =(G ρ)1/2 is the acoustic impedance of the medium. ρ is the density, c, the speed of sound, and G = i ωη is the shear modulus.
For Newtonian liquids
Newtonian fluid
A Newtonian fluid is a fluid whose stress versus strain rate curve is linear and passes through the origin. The constant of proportionality is known as the viscosity.-Definition:...
(η’ = const, η’’ = 0), Δf and Δ(w/2) are equal and opposite. They scale as the square root of the overtone order, n1/2. For viscoelastic liquids (η’ = η(ω), η’’≠ 0), the complex viscosity can be obtained as
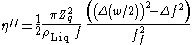
Importantly, the QCM only probes the region close to the crystal surface. The shear wave evanescently decays into the liquid. In water the penetration depth is about 250 nm at 5 MHz. Surface roughness, nano-bubbles at the surface, slip, and compressional waves can interfere with the measurement of viscosity. Also, the viscosity determined at MHz frequencies sometimes differs from the low-frequency viscosity. In this respect, torsional resonators (with a frequency around 100 kHz) are closer to application than thickness-shear resonators.
Inertial loading (Sauerbrey equation)
The frequency shift induced by a thin sample which is rigidly coupled to the crystal (such as a thin film), is described by the Sauerbrey equation. The stress is governed by inertiaInertia
Inertia is the resistance of any physical object to a change in its state of motion or rest, or the tendency of an object to resist any change in its motion. It is proportional to an object's mass. The principle of inertia is one of the fundamental principles of classical physics which are used to...
, which implies σ = -ω2u0mF, where u0 is the amplitude of oscillation and mF is the (average) mass per unit area. Inserting this result into the small-load-approximation one finds
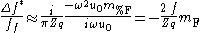
If the density of the film is known, one can convert from mass per unit area, mF, to thickness, dF. The thickness thus derived is also called the Sauerbrey thickness to show that it was derived by applying the Sauerbrey equation to the frequency shift.
The shift in bandwidth is zero if the Sauerbrey equation holds. Checking for the bandwidth therefore amounts to checking the applicability of the Sauerbrey equation.
The Sauerbrey equation was first derived by G. Sauerbrey in 1959 and correlates changes in the oscillation frequency of a piezoelectric crystal with mass deposited on it. He simultaneously developed a method for measuring the resonance frequency and its changes by using the crystal as the frequency-determining component of an oscillator circuit. His method continues to be used as the primary tool in quartz crystal microbalance experiments for conversion of frequency to mass.
Because the film is treated as an extension of thickness, Sauerbrey’s equation only applies to systems in which (a) the deposited mass has the same acoustic properties as the crystal and (b) the frequency change is small (Δf / f < 0.05).
If the change in frequency is greater than 5%, that is, Δf / f > 0.05, the Z-match method must be used to determine the change in mass. The formula for the Z-match method is:
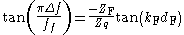
kF is the wave vector inside the film and dF its thickness. Inserting kF = 2·π·f /cF = 2·π·f·ρF / ZF as well as dF = mF / ρF yields
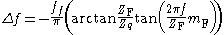
Viscoelastic film
For a viscoelastic film, the frequency shift is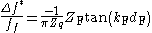Here ZF is the acoustic impedance of the film (ZF = ρFcF = (ρFGf)1/2)= (ρF/Jf)1/2), kF is the wave vector and dF is the film thickness. Jf is the film's viscoelastic compliance, ρF is the density.
The poles of the tangent (kF dF = π/2) define the film resonances. At the film resonance, one has dF = λ/4. The agreement between experiment and theory is often poor close to the film resonance. Typically, the QCM only works well for film thicknesses much less than a quarter of the wavelength of sound (corresponding to a few micrometres, depending on the softness of the film and the overtone order).
Note that the properties of a film as determined with the QCM are fully specified by two parameters, which are its acoustic impedance, ZF = ρFcF and its mass per unit area, mF = dF/ρF. The wave number kF = ω/cF is not algebraically independent from ZF and mF. Unless the density of the film is known independently, the QCM can only measure mass per unit area, never the geometric thickness itself.
Viscoelastic film in liquid
For a film immersed in a liquid environment, the frequency shift is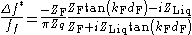The indices F and Liq denote the film and the liquid. Here, the reference state is the crystal immersed in liquid (but not covered with a film). For thin films, one can Taylor-expand
Taylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
the above equation to first order in dF, yielding
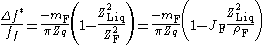
Apart from the term in brackets, this equation is equivalent to the Sauerbrey equation. The term in brackets is a viscoelastic correction, dealing with the fact that in liquids, soft layers lead to a smaller Sauerbrey thickness than rigid layers.
Derivation of viscoelastic constants
The frequency shift depends on the acoustic impedance of the material; the latter in turn depends on the viscoelastic properties of the material. Therefore, in principle, one can derive the complex shear modulus (or equivalently, the complex viscosity). However, there are certain caveats to be kept in mind:- The viscoelastic parameters themselves usually depend on frequency (and therefore on the overtone order).
- It is often difficult to disentangle effects of inertia and viscoelasticity. Unless the film thickness is known independently, it is difficult to obtain unique fitting results.
- Electrode effects can be of importance.
- For films in air, the small-load approximation must be replaced by the corresponding results from perturbation theory unless the films are very soft.
For thin films in liquids, there is an approximate analytical result, relating the elastic compliance of the film, JF’ to the ratio of Δ(w/2); and Δf. The shear compliance is the inverse of the shear modulus, G. In the thin-film limit, the ratio of Δ(w/2) and –Δf is independent of film thickness. It is an intrinsic property of the film. One has
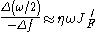
For thin films in air an analogous analytical result is
Here J’’ is the viscous shear compliance.
Interpretation of the Sauerbrey thickness
The correct interpretation of the frequency shift from QCM experiments in liquids is a challenge. Practitioners often just apply the Sauerbrey equation to their data and term the resulting areal mass (mass per unit area) the "Sauerbrey mass" and the corresponding thickness "Sauerbrey thickness". Even though the Sauerbrey thickness can certainly serve to compare different experiments, it must not be naively identified with the geometric thickness. Worthwhile considerations are the following:a) The QCM always measures an areal mass density, never a geometric thickness. The conversion from areal mass density to thickness usually requires the physical density as an independent input.
b) It is difficult to infer the viscoelastic correction factor from QCM data. However, if the correction factor differs significantly from unity, it may be expected that it affects the bandwidth Δ(w/2) and also that it depends on overtone order. If, conversely, such effects are absent (Δ(w/2) « Δf, Sauerbrey thickness same on all overtone orders) one may assume that (1-ZLiq2/ZF2)≈1.
c) Complex samples are often laterally heterogeneous.
d) Complex samples often have fuzzy interfaces. A "fluffy" interface will often lead to a viscoelastic correction and, as a consequence, to a non-zero Δ(w/2) as well as an overtone-dependent Sauerbrey mass. In the absence of such effects, one may conclude that the outer interface of film is sharp.
e) When the viscoelastic correction, as discussed in (b), is insignificant, this does by no means imply that the film is not swollen by the solvent
Solvent
A solvent is a liquid, solid, or gas that dissolves another solid, liquid, or gaseous solute, resulting in a solution that is soluble in a certain volume of solvent at a specified temperature...
. It only means that the (swollen) film is much more rigid than the ambient liquid. QCM data taken on the wet sample alone do not allow inference of the degree of swelling. The amount of swelling can be inferred from the comparison of the wet and the dry thickness. The degree of swelling is also accessible by comparing the acoustic thickness (in the Sauerbrey sense) to the optical thickness as determined by, for example, surface plasmon resonance (SPR) spectroscopy or ellipsometry. Solvent contained in the film usually does contribute to the acoustic thickness (because it takes part in the movement), whereas it does not contribute to the optic thickness (because the electronic polarizability
Polarizability
Polarizability is the measure of the change in a molecule's electron distribution in response to an applied electric field, which can also be induced by electric interactions with solvents or ionic reagents. It is a property of matter...
of a solvent molecule does not change when it is located inside a film).
Point contacts
The equations concerning viscoelastic properties assume planar layer systems. A frequency shift is also induced when the crystal makes contact with discrete objects across small, load-bearing asperities. Such contacts are often encountered with rough surfaces. It is assumed that the stress–speed ratio may be replaced by an average stress–speed ratio, where the average stress just is the lateral force divided by the active area of the crystal.Often, the external object is so heavy that it does not take part in the MHz oscillation of the crystal due to inertia. It then rests in place in the laboratory frame. When the crystal surface is laterally displaced, the contact exerts a restoring force upon the crystal surface. The stress is proportional to the number density of the contacts, NS, and their average spring constant, κS. The spring constant may be complex (κS* = κS’ + iκS’’), where the imaginary part quantifies a withdrawal of energy from the crystal oscillation (for instance due to viscoelastic effects). For such a situation, the small-load approximation predicts
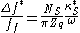
The QCM allows for non-destructive testing of the shear stiffness of multi-asperity contacts.
See also
- Sauerbrey equationSauerbrey equationThe Sauerbrey equation was developed by G. Sauerbrey in 1959 as a method for correlating changes in the oscillation frequency of a piezoelectric crystal with the mass deposited on it. He simultaneously developed a method for measuring the characteristic frequency and its changes by using the...
- Weighing scaleWeighing scaleA weighing scale is a measuring instrument for determining the weight or mass of an object. A spring scale measures weight by the distance a spring deflects under its load...
- PiezoelectricityPiezoelectricityPiezoelectricity is the charge which accumulates in certain solid materials in response to applied mechanical stress. The word piezoelectricity means electricity resulting from pressure...
- Thin-film thickness monitorThin-film thickness monitorThin-film thickness monitors, deposition rate controllers, and so on, are a family of instruments used in high and ultra-high vacuum systems. They can measure the thickness of a thin film, not only after it has been made, but while it is still being deposited, and some can control either the final...
- QCM-DQCM-DThe Quartz Crystal Microbalance with Dissipation Monitoring is a special type of QCM based on the ring-down technique,. The term QCM-D is a trademark owned by Q-sense AB, Gothenburg, Sweden. The active component of a QCM is a thin quartz crystal disk sandwiched between a pair of electrodes...