Rankine-Hugoniot equation
Encyclopedia
The Rankine–Hugoniot conditions, also referred to as Rankine–Hugoniot jump conditions or Rankine–Hugoniot relations, relate to the behaviour of shock wave
s traveling normal to the prevailing flow. They are named in recognition of the work carried out by Scottish engineer and physicist William John Macquorn Rankine
and French engineer Pierre Henri Hugoniot
. See also Salas (2006) for some historical background.
. In addition, assume that the fluid is inviscid
(negligible viscosity effects). Such a system can be described by the following system of conservation law
s, known as the 1D Euler equations
where,
fluid mass density, [kg/m3]
fluid velocity
, [m/s]
fluid-specific internal energy
, [J/kg]
fluid pressure
, [Pa]
time, [s]
distance, [m], and
fluid-specific total energy, [J/kg].
For adiabatic conditions a polytropic process can be represented by the equation-of-state
,
where
represents the polytropic exponent (equal to the ratio of specific heats 
) of the polytropic process
For an extensive list of compressible flow equations, etc., refer to NACA
Report 1135 (1953).
Note: For a calorically ideal gas
is a constant and for a thermally ideal gas 
is a function of temperature.
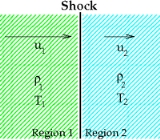
Consider a 1D situation where there is a jump in the scalar conserved physical quantity
, which is governed by the hyperbolic conservation law
Let the solution exhibit a jump (or shock) at
and integrate over the partial domain, 
, where 
and 
,
The subscripts 1 and 2 indicate conditions just upstream and just downstream of the jump respectively. Note, to arrive at equation (8) we have used the fact that
and 
.
Now, let
and 
, when we have 
and 
, and in the limit
where we have defined
(the system characteristic or shock speed), which by simple division is given by
Equation (9) represents the jump condition for conservation equation (6). A shock situation arises in a system where its characteristics intersect, and under these conditions a requirement for a unique single-valued solution is that the solution should satisfy the admissibility condition or entropy condition. For physically real applications this means that the solution should satisfy the Lax entropy condition
where
and 
represent characteristic speeds at upstream and downstream conditions respectively.
and the jump conditions become
Equations (12), (13) and (14) are known as the Rankine–Hugoniot conditions for the Euler equations and are derived by enforcing the conservation laws in integral form over a control volume that includes the shock. For this situation 
cannot be obtained by simple division. However, it can be shown by transforming the problem to a moving co-ordinate system, i.e. 
, and some algebraic manipulation, that the shock speed is given by
where
is the speed of sound in the fluid at upstream conditions.
See Laney (1998),
LeVeque (2002), Toro (1999), Wesseling (2001), and Whitham (1999) for further discussion.
, and for the 1D Euler equations we have
In view of equation (12) we can simplify equation (14) to
which is a statement of Bernoulli's principle
, under conditions where gravitational effects can be neglected.
Substituting
and 
from equations (12) and (13) into equation (15) yields the following relationship:
where
represents specific enthalpy
of the fluid. Eliminating internal energy
in equation (15) by use of the equation-of-state, equation ( 4), yields
From physical considerations it is clear that both the upstream and downstream pressures must be positive, and this imposes an upper limit on the density ratio in equations (17) and (18) such that
. As the strength of the shock increases, there is a corresponding increase in downstream gas temperature, but the density ratio 
approaches a finite limit: 4 for an ideal monatomic gas 
and 6 for an ideal diatomic
gas
. Note: air is comprised predominately of diatomic molecules and therefore at standard conditions 
.
Shock wave
A shock wave is a type of propagating disturbance. Like an ordinary wave, it carries energy and can propagate through a medium or in some cases in the absence of a material medium, through a field such as the electromagnetic field...
s traveling normal to the prevailing flow. They are named in recognition of the work carried out by Scottish engineer and physicist William John Macquorn Rankine
William John Macquorn Rankine
William John Macquorn Rankine was a Scottish civil engineer, physicist and mathematician. He was a founding contributor, with Rudolf Clausius and William Thomson , to the science of thermodynamics....
and French engineer Pierre Henri Hugoniot
Pierre Henri Hugoniot
Pierre-Henri Hugoniot who mostly lived in Montbéliard, . He was an inventor, mathematician, and physicist who worked on fluid mechanics, especially on issues related to material shock.After going into the marine artillery upon his graduation from the École Polytechnique in 1872, Hugoniot became...
. See also Salas (2006) for some historical background.
Introduction
Consider a one-dimensional, calorically ideal gas described by a polytropic equation-of-state and operating under adiabatic conditionsAdiabatic process
In thermodynamics, an adiabatic process or an isocaloric process is a thermodynamic process in which the net heat transfer to or from the working fluid is zero. Such a process can occur if the container of the system has thermally-insulated walls or the process happens in an extremely short time,...
. In addition, assume that the fluid is inviscid
Inviscid flow
In fluid dynamics there are problems that are easily solved by using the simplifying assumption of an ideal fluid that has no viscosity. The flow of a fluid that is assumed to have no viscosity is called inviscid flow....
(negligible viscosity effects). Such a system can be described by the following system of conservation law
Conservation law
In physics, a conservation law states that a particular measurable property of an isolated physical system does not change as the system evolves....
s, known as the 1D Euler equations
where,
fluid mass density, [kg/m3] fluid velocityVelocity
In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...
, [m/s]
fluid-specific internal energyInternal energy
In thermodynamics, the internal energy is the total energy contained by a thermodynamic system. It is the energy needed to create the system, but excludes the energy to displace the system's surroundings, any energy associated with a move as a whole, or due to external force fields. Internal...
, [J/kg]
fluid pressurePressure
Pressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
, [Pa]
time, [s] distance, [m], and fluid-specific total energy, [J/kg].For adiabatic conditions a polytropic process can be represented by the equation-of-state
Equation of state
In physics and thermodynamics, an equation of state is a relation between state variables. More specifically, an equation of state is a thermodynamic equation describing the state of matter under a given set of physical conditions...
,
where
represents the polytropic exponent (equal to the ratio of specific heats ) of the polytropic process
For an extensive list of compressible flow equations, etc., refer to NACA
National Advisory Committee for Aeronautics
The National Advisory Committee for Aeronautics was a U.S. federal agency founded on March 3, 1915 to undertake, promote, and institutionalize aeronautical research. On October 1, 1958 the agency was dissolved, and its assets and personnel transferred to the newly created National Aeronautics and...
Report 1135 (1953).
Note: For a calorically ideal gas
is a constant and for a thermally ideal gas is a function of temperature.The jump condition
Before proceeding further it is necessary to introduce the concept of a jump condition – a condition that holds at a discontinuity or abrupt change.Consider a 1D situation where there is a jump in the scalar conserved physical quantity
, which is governed by the hyperbolic conservation law
Let the solution exhibit a jump (or shock) at
and integrate over the partial domain, , where and ,
The subscripts 1 and 2 indicate conditions just upstream and just downstream of the jump respectively. Note, to arrive at equation (8) we have used the fact that
and .Now, let
and , when we have and , and in the limit
where we have defined
(the system characteristic or shock speed), which by simple division is given by
Equation (9) represents the jump condition for conservation equation (6). A shock situation arises in a system where its characteristics intersect, and under these conditions a requirement for a unique single-valued solution is that the solution should satisfy the admissibility condition or entropy condition. For physically real applications this means that the solution should satisfy the Lax entropy condition
where
and represent characteristic speeds at upstream and downstream conditions respectively.Euler equations shock condition
In the case of the hyperbolic conservation equation (6), we have seen that the shock speed can be obtained by simple division. However, for the 1D Euler equations ( 1), ( 2) and ( 3), we have the vector state variable and the jump conditions become
cannot be obtained by simple division. However, it can be shown by transforming the problem to a moving co-ordinate system, i.e. , and some algebraic manipulation, that the shock speed is given by
where
is the speed of sound in the fluid at upstream conditions.See Laney (1998),
LeVeque (2002), Toro (1999), Wesseling (2001), and Whitham (1999) for further discussion.
Stationary shock
For a stationary shock, and for the 1D Euler equations we have
In view of equation (12) we can simplify equation (14) to
which is a statement of Bernoulli's principle
Bernoulli's principle
In fluid dynamics, Bernoulli's principle states that for an inviscid flow, an increase in the speed of the fluid occurs simultaneously with a decrease in pressure or a decrease in the fluid's potential energy...
, under conditions where gravitational effects can be neglected.
Substituting
and from equations (12) and (13) into equation (15) yields the following relationship:
where
represents specific enthalpyEnthalpy
Enthalpy is a measure of the total energy of a thermodynamic system. It includes the internal energy, which is the energy required to create a system, and the amount of energy required to make room for it by displacing its environment and establishing its volume and pressure.Enthalpy is a...
of the fluid. Eliminating internal energy
in equation (15) by use of the equation-of-state, equation ( 4), yields
From physical considerations it is clear that both the upstream and downstream pressures must be positive, and this imposes an upper limit on the density ratio in equations (17) and (18) such that
. As the strength of the shock increases, there is a corresponding increase in downstream gas temperature, but the density ratio approaches a finite limit: 4 for an ideal monatomic gas and 6 for an ideal diatomicDiatomic
Diatomic molecules are molecules composed only of two atoms, of either the same or different chemical elements. The prefix di- means two in Greek. Common diatomic molecules are hydrogen , nitrogen , oxygen , and carbon monoxide . Seven elements exist in the diatomic state in the liquid and solid...
gas
. Note: air is comprised predominately of diatomic molecules and therefore at standard conditions .See also
- Calculate normal shock wave parameters for mixtures of imperfect gases. Gas Dynamics Toolbox
- Shock polarShock polarThe term shock polar is generally used with the graphical representation of the Rankine-Hugoniot equations in either the hodograph plane or the pressure ratio-flow deflection angle plane...