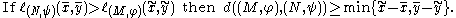Size theory
Encyclopedia
In mathematics
, size theory studies the properties of topological space
s endowed with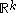 -valued functions
-valued functions
, with respect to the change of these functions. More formally, the subject of size theory is the study of the natural pseudodistance
between size pairs.
A survey of size theory can be found in
.
, introduced by Frosini. Size functions have been initially used as a mathematical tool for shape comparison in computer vision
and pattern recognition
.
An extension of the concept of size function to algebraic topology
was made in
,
where size homotopy groups were introduced, together with the natural pseudodistance
for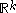 -valued functions.
-valued functions.
An extension to homology theory
(the size functor
) was introduced in
.
The size homotopy group and the size functor
are strictly related to the concept of persistent homology group
,
studied in persistent homology. It is worth to point out that the size function is the rank of the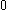 -th persistent homology group, while the relation between the persistent homology group
-th persistent homology group, while the relation between the persistent homology group
and the size homotopy group is analogous to the one existing between homology groups and homotopy group
s.
In size theory, size function
s and size homotopy groups are seen as tools to compute lower bounds for the natural pseudodistance
.
Actually, the following link exists between the values taken by the size functions ,
, 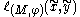 and the natural pseudodistance
and the natural pseudodistance
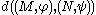 between the size pairs
between the size pairs 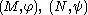
,
An analogous result holds for Size homotopy group.
The attempt to generalize size theory and the concept of natural pseudodistance
to norms that are different from the supremum norm has led to the study of other reparametrization invariant norms .
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, size theory studies the properties of topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
s endowed with
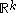 -valued functions
-valued functionsFunction (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
, with respect to the change of these functions. More formally, the subject of size theory is the study of the natural pseudodistance
Natural pseudodistance
In size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
between size pairs.
A survey of size theory can be found in
.
History and applications
The beginning of size theory is rooted in the concept of size functionSize function
Size functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane xSize functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane...
, introduced by Frosini. Size functions have been initially used as a mathematical tool for shape comparison in computer vision
Computer vision
Computer vision is a field that includes methods for acquiring, processing, analysing, and understanding images and, in general, high-dimensional data from the real world in order to produce numerical or symbolic information, e.g., in the forms of decisions...
and pattern recognition
Pattern recognition
In machine learning, pattern recognition is the assignment of some sort of output value to a given input value , according to some specific algorithm. An example of pattern recognition is classification, which attempts to assign each input value to one of a given set of classes...
.
An extension of the concept of size function to algebraic topology
Algebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
was made in
,
where size homotopy groups were introduced, together with the natural pseudodistance
Natural pseudodistance
In size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
for
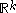 -valued functions.
-valued functions.An extension to homology theory
Homology theory
In mathematics, homology theory is the axiomatic study of the intuitive geometric idea of homology of cycles on topological spaces. It can be broadly defined as the study of homology theories on topological spaces.-The general idea:...
(the size functor
Size functor
Given a size pair \ where M\ is a manifold of dimensionn\ and f\ is an arbitrary real continuous function definedon it, the i\ -th size functor, with i=0,\ldots,n\ , denoted...
) was introduced in
.
The size homotopy group and the size functor
Size functor
Given a size pair \ where M\ is a manifold of dimensionn\ and f\ is an arbitrary real continuous function definedon it, the i\ -th size functor, with i=0,\ldots,n\ , denoted...
are strictly related to the concept of persistent homology group
,
studied in persistent homology. It is worth to point out that the size function is the rank of the
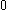 -th persistent homology group, while the relation between the persistent homology group
-th persistent homology group, while the relation between the persistent homology groupand the size homotopy group is analogous to the one existing between homology groups and homotopy group
Homotopy group
In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, which records information about loops in a space...
s.
In size theory, size function
Size function
Size functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane xSize functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane...
s and size homotopy groups are seen as tools to compute lower bounds for the natural pseudodistance
Natural pseudodistance
In size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
.
Actually, the following link exists between the values taken by the size functions
 ,
, 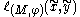 and the natural pseudodistance
and the natural pseudodistanceNatural pseudodistance
In size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
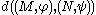 between the size pairs
between the size pairs 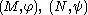
,
An analogous result holds for Size homotopy group.
The attempt to generalize size theory and the concept of natural pseudodistance
Natural pseudodistance
In size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
to norms that are different from the supremum norm has led to the study of other reparametrization invariant norms .
See also
- Size functionSize functionSize functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane xSize functions are shape descriptors, in a geometrical/topological sense. They are functions from the half-plane...
- Natural pseudodistanceNatural pseudodistanceIn size theory, the natural pseudodistance between two size pairs \ , \ is the value \inf_h \|\varphi-\psi\circ h\|_\infty\ , where h\ varies in the set of all homeomorphisms from the manifold M\ to the manifold N\ and \|\cdot\|_\infty\ is the supremum norm...
- Size functorSize functorGiven a size pair \ where M\ is a manifold of dimensionn\ and f\ is an arbitrary real continuous function definedon it, the i\ -th size functor, with i=0,\ldots,n\ , denoted...
- Size homotopy group
- Size pair
- Matching distanceMatching distanceIn mathematics, the matching distance is a metric on the space of size functions.The core of the definition of matching distance is the observation that the...