Toric code
Encyclopedia
The Toric Code is a topological
quantum error correcting code
, and an example of a stabilizer code
, defined on a two dimensional spin
lattice
It is the simplest and most well studied of the quantum double models.
, with a spin-½
particle located on each edge. The periodic boundary conditions of the lattice are chosen such that it is wrapped around a torus
, giving the code its name. Stabilizer
operators are defined on the spins around each vertex
and plaquette (or face) 
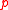of the lattice as follows,
Where here we use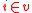
to denote the edges touching the vertex 
, and 
to denote the edges surrounding the plaquette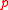 
. The stabilizer space of the code is that for which all stabilizers act trivially, hence,
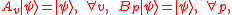
for any state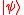
. For the toric code, this space is four dimensional, and so can be used to store two qubits of quantum information
. This can be proven by considering the number of independent stabilizer operators. The occurrence of errors will move the state out of the stabilizer space, resulting in vertices and plaquettes for which the above condition does not hold. The positions of these violations is the syndrome of the code, which can be used for error correction.
The unique nature of the topological codes, such as the toric code, is that stabilizer violations can be interpreted as quasiparticles. Specifically, if the code is in a state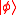
such that,
,
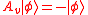a quasiparticle known as an
anyon
can be said to exist on the vertex
. Similarly violations of the 
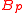are associated with so called 
anyons on the plaquettes. The stabilizer space therefore corresponds to the anyonic vacuum. Single spin errors cause pairs of anyons to be created and transported around the lattice.
When errors create an anyon pair and move the anyons, one can imagine a path connecting the two composed of all links acted upon. If the anyons then meet and are annihilated, this path describes a loop. If the loop is topologically trivial, it has no effect on the stored information. The annihilation of the anyons in this case corrects all of the errors involved in their creation and transport. However, if the loop is topologically non-trivial, though reannihilation of the anyons returns the state to the stabilizer space it also implements a logical operation on the stored information. The errors in this case are therefore not corrected, but consolidated.
Let us use
to denote the probability of a 
error on each spin. When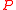 
is low, this will create sparsely distributed pairs of 
anyons which have not moved far from their point of creation. In this case minimum weight perfect matching
can be used to identify the pairs and reannihilate them, correcting the errors . As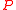
increases, however, it becomes more ambiguous as to how the anyons may be matched without risking the formation of topologically non-trivial loops. This gives a threshold probability, under which the error correction will almost certainly succeed. Through a mapping to the random bond Ising model, this critical probability has been found to be 
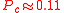. The threshold holds also for 
errors.
The means to perform quantum computation on logical information stored within the toric code has been considered, with the properties of the code providing fault-tolerance. It has been shown that extending the stabilizer space using 'holes', vertices or plaquettes on which stabilizers are not enforced, allows a universal set of gates
to be performed. A measurement based
scheme for quantum computation based upon this principle has been found, whose error threshold is the highest known for a two dimensional architecture.
The ground state of this Hamiltonian is the stabilizer space of the code. Excited states correspond to those of anyons, with the energy proportional to their number. Local errors are therefore energetically suppressed by the gap, which has been shown to be stable against local perturbations. However, the dynamic effects of such perturbations can still cause problems for the code.
The gap also gives the code a certain resilience against thermal errors, allowing it to be correctable almost surely for a certain critical time. This time increases with
, but since arbitrary increases of this coupling are unrealistic, the code still has its limits. Accordingly, many attempts are being made to make the toric code fully thermally stable with a lifetime which increases with the system size .
and 
quasiparticles are associated with the vertices and plaquettes of the model, respectively. These quasiparticles can be described as anyons, due to the non-trivial effect of their braiding. Specifically, though both species of anyons are bosonic with respect to themselves, the braiding of two 
's or 
's having no effect, a full monodromy of an 
and an 
will yield a phase of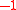 
. Such a result is not consistent with either bosonic or fermionic statistics
, and hence is anyonic.
The anyonic mutual statistics of the quasiparticles demonstrate the logical operations performed by topologically non-trivial loops. Consider the creation of a pair of
anyons followed by the transport of one around a topologically nontrivial loop, such as that shown on the torus in blue on the figure above, before the pair are reannhilated. The state is returned to the stabilizer space, but the loop implements a logical operation on one of the stored qubits. If 
anyons are similarly moved through the red loop above a logical operation will also result. The phase of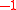 
resulting when braiding the anyons shows that these operations do not commute, but rather anticommute. They may therefore be interpreted as logical 
and 
Pauli operators on one of the stored qubits. The corresponding logical Pauli's on the other qubit correspond to an 
anyon following the blue loop and an 
anyon following the red. No braiding occurs when 
and 
pass through parallel paths, the phase of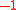 
therefore does not arise and the corresponding logical operations commute. This is as should be expected since these form operations acting on different qubits.
Due to the fact that both
and 
anyons can be created in pairs, it is clear to see that both these quasiparticles are their own antiparticles. A composite particle composed of two 
anyons is therefore equivalent to the vacuum, since the vacuum can yield such a pair and such a pair will annihilate to the vacuum. Accordingly, these composites have bosonic statistics, since their braiding is always completely trivial. A composite of two 
anyons is similarly equivalent to the vacuum. The creation of such composites is known as the fusion of anyons, and the results can be written in terms of fusion rules. In this case, these take the form,
Where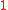
denotes the vacuum. A composite of an 
and an 
is not trivial. This therefore constitutes another quasiparticle in the model, sometimes denoted 
, with fusion rule,
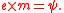
From the braiding statistics of the anyons we see that, since any single exchange of two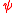
's will involve a full monodromy of a constituent 
and 
, a phase of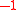 
will result. This implies fermionic self-statistics for the 
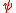's.
systems. For example, it has been used to study the effects of thermalization on the topological entropy
of states. It has also been shown that, using superposition states of the Abelian toric code anyons, some properties of the more complex non-Abelian anyons can be realized.
It is also possible to define similar codes using higher dimensional spins. These are the quantum double models, which allow a greater richness in the behaviour of anyons, and so may be used for more advanced quantum computation and error correction proposals. These not only include models with Abelian anyons, but also those with non-Abelian statistics.
Hamiltonian, these simply prepare the code in the stabilizer space and demonstrate the creation, transport and statistics of the anyons.
For realizations of the toric code and its generalizations with a Hamiltonian, much progress has been made using Josephson junctions. The theory of how the Hamiltonians may be implemented has been developed for a wide class of topological codes. An experiment has also been performed, realizing the toric code Hamiltonian for a small lattice, and demonstrating the quantum memory provided by its degenerate ground state.
Other theoretical work towards experimental realizations is based on cold atoms. A toolkit of methods that may be used to realize topological codes with optical lattices has been explored,
as have experiments concerning minimal instances of topological order. Progress is also being made into simulations of the toric model with Rydberg atoms, in which the Hamiltonian and the effects of dissipative noise can be demonstrated.
Topological order
In physics, topological order is a new kind of order in a quantum state that is beyond the Landau symmetry-breaking description. It cannot be described by local order parameters and long range correlations...
quantum error correcting code
Quantum error correction
Quantum error correction is used in quantum computing to protect quantum information from errors due to decoherence and other quantum noise. Quantum error correction is essential if one is to achieve fault-tolerant quantum computation that can deal not only with noise on stored quantum...
, and an example of a stabilizer code
Stabilizer code
The theory of quantum error correction plays a prominent role in the practical realization and engineering ofquantum computing and quantum communication devices. The first quantumerror-correcting codes are strikingly similar to classical block codes in their...
, defined on a two dimensional spin
Spin (physics)
In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
lattice
Planar graph
In graph theory, a planar graph is a graph that can be embedded in the plane, i.e., it can be drawn on the plane in such a way that its edges intersect only at their endpoints...
It is the simplest and most well studied of the quantum double models.
Error correction and computation
The toric code is defined on a two dimensional lattice, usually chosen to be the square latticeSquare lattice
In mathematics, the square lattice is a type of lattice in a two-dimensional Euclidean space. It is the two-dimensional version of the integer lattice. It is one of the five types of two-dimensional lattices as classified by their symmetry groups; its symmetry group is known symbolically as p4m.Two...
, with a spin-½
Spin-½
In quantum mechanics, spin is an intrinsic property of all elementary particles. Fermions, the particles that constitute ordinary matter, have half-integer spin. Spin-½ particles constitute an important subset of such fermions. All known elementary fermions have a spin of ½.- Overview :Particles...
particle located on each edge. The periodic boundary conditions of the lattice are chosen such that it is wrapped around a torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
, giving the code its name. Stabilizer
Stabilizer code
The theory of quantum error correction plays a prominent role in the practical realization and engineering ofquantum computing and quantum communication devices. The first quantumerror-correcting codes are strikingly similar to classical block codes in their...
operators are defined on the spins around each vertex
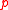and plaquette (or face) of the lattice as follows,Where here we use
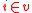to denote the edges touching the vertex , and to denote the edges surrounding the plaquette 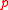. The stabilizer space of the code is that for which all stabilizers act trivially, hence,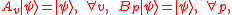for any state
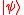. For the toric code, this space is four dimensional, and so can be used to store two qubits of quantum informationQuantum information
In quantum mechanics, quantum information is physical information that is held in the "state" of a quantum system. The most popular unit of quantum information is the qubit, a two-level quantum system...
. This can be proven by considering the number of independent stabilizer operators. The occurrence of errors will move the state out of the stabilizer space, resulting in vertices and plaquettes for which the above condition does not hold. The positions of these violations is the syndrome of the code, which can be used for error correction.
The unique nature of the topological codes, such as the toric code, is that stabilizer violations can be interpreted as quasiparticles. Specifically, if the code is in a state
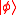such that,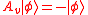,a quasiparticle known as an
anyonAnyon
In physics, an anyon is a type of particle that occurs only in two-dimensional systems. It is a generalization of the fermion and boson concept.-From theory to reality:...
can be said to exist on the vertex
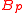. Similarly violations of the are associated with so called anyons on the plaquettes. The stabilizer space therefore corresponds to the anyonic vacuum. Single spin errors cause pairs of anyons to be created and transported around the lattice.When errors create an anyon pair and move the anyons, one can imagine a path connecting the two composed of all links acted upon. If the anyons then meet and are annihilated, this path describes a loop. If the loop is topologically trivial, it has no effect on the stored information. The annihilation of the anyons in this case corrects all of the errors involved in their creation and transport. However, if the loop is topologically non-trivial, though reannihilation of the anyons returns the state to the stabilizer space it also implements a logical operation on the stored information. The errors in this case are therefore not corrected, but consolidated.
Let us use
to denote the probability of a error on each spin. When 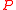is low, this will create sparsely distributed pairs of anyons which have not moved far from their point of creation. In this case minimum weight perfect matchingEdmonds's matching algorithm
Edmonds's matching algorithmis an algorithm in graph theory for constructing maximum matchings on graphs. The algorithm was discovered by Jack Edmonds in 1965. Given a general graph G = , the algorithm finds a matching M such that each vertex in V is incident with at most one edge in M and |M| is...
can be used to identify the pairs and reannihilate them, correcting the errors . As
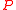increases, however, it becomes more ambiguous as to how the anyons may be matched without risking the formation of topologically non-trivial loops. This gives a threshold probability, under which the error correction will almost certainly succeed. Through a mapping to the random bond Ising model, this critical probability has been found to be 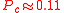. The threshold holds also for errors.The means to perform quantum computation on logical information stored within the toric code has been considered, with the properties of the code providing fault-tolerance. It has been shown that extending the stabilizer space using 'holes', vertices or plaquettes on which stabilizers are not enforced, allows a universal set of gates
Quantum gate
In quantum computing and specifically the quantum circuit model of computation, a quantum gate is a basic quantum circuit operating on a small number of qubits. They are the building blocks of quantum circuits, like classical logic gates are for conventional digital circuits.Unlike many classical...
to be performed. A measurement based
One-way quantum computer
The one-way or measurement based quantum computer is a method of quantum computing that first prepares an entangled resource state, usually a cluster state or graph state, then performs single qubit measurements on it...
scheme for quantum computation based upon this principle has been found, whose error threshold is the highest known for a two dimensional architecture.
Hamiltonian
Since the stabilizer operators of the toric code are quasilocal, acting only on spins located near each other on a two dimensional lattice, it is not unrealistic to define the following Hamiltonian,The ground state of this Hamiltonian is the stabilizer space of the code. Excited states correspond to those of anyons, with the energy proportional to their number. Local errors are therefore energetically suppressed by the gap, which has been shown to be stable against local perturbations. However, the dynamic effects of such perturbations can still cause problems for the code.
The gap also gives the code a certain resilience against thermal errors, allowing it to be correctable almost surely for a certain critical time. This time increases with
, but since arbitrary increases of this coupling are unrealistic, the code still has its limits. Accordingly, many attempts are being made to make the toric code fully thermally stable with a lifetime which increases with the system size .Anyon model
As mention above, so called and quasiparticles are associated with the vertices and plaquettes of the model, respectively. These quasiparticles can be described as anyons, due to the non-trivial effect of their braiding. Specifically, though both species of anyons are bosonic with respect to themselves, the braiding of two 's or 's having no effect, a full monodromy of an and an will yield a phase of 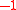. Such a result is not consistent with either bosonic or fermionic statisticsSpin-statistics theorem
In quantum mechanics, the spin-statistics theorem relates the spin of a particle to the particle statistics it obeys. The spin of a particle is its intrinsic angular momentum...
, and hence is anyonic.
The anyonic mutual statistics of the quasiparticles demonstrate the logical operations performed by topologically non-trivial loops. Consider the creation of a pair of
anyons followed by the transport of one around a topologically nontrivial loop, such as that shown on the torus in blue on the figure above, before the pair are reannhilated. The state is returned to the stabilizer space, but the loop implements a logical operation on one of the stored qubits. If anyons are similarly moved through the red loop above a logical operation will also result. The phase of 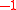resulting when braiding the anyons shows that these operations do not commute, but rather anticommute. They may therefore be interpreted as logical and Pauli operators on one of the stored qubits. The corresponding logical Pauli's on the other qubit correspond to an anyon following the blue loop and an anyon following the red. No braiding occurs when and pass through parallel paths, the phase of 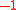therefore does not arise and the corresponding logical operations commute. This is as should be expected since these form operations acting on different qubits.Due to the fact that both
and anyons can be created in pairs, it is clear to see that both these quasiparticles are their own antiparticles. A composite particle composed of two anyons is therefore equivalent to the vacuum, since the vacuum can yield such a pair and such a pair will annihilate to the vacuum. Accordingly, these composites have bosonic statistics, since their braiding is always completely trivial. A composite of two anyons is similarly equivalent to the vacuum. The creation of such composites is known as the fusion of anyons, and the results can be written in terms of fusion rules. In this case, these take the form,Where
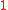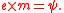
denotes the vacuum. A composite of an and an is not trivial. This therefore constitutes another quasiparticle in the model, sometimes denoted , with fusion rule,From the braiding statistics of the anyons we see that, since any single exchange of two
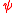's will involve a full monodromy of a constituent and , a phase of 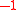will result. This implies fermionic self-statistics for the 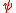's.Other uses
As a simple model of anyons, the toric code can be used in studies of anyonic behaviour and topologically orderedTopological order
In physics, topological order is a new kind of order in a quantum state that is beyond the Landau symmetry-breaking description. It cannot be described by local order parameters and long range correlations...
systems. For example, it has been used to study the effects of thermalization on the topological entropy
Topological entropy
In mathematics, the topological entropy of a topological dynamical system is a nonnegative real number that measures the complexity of the system. Topological entropy was first introduced in 1965 by Adler, Konheim and McAndrew. Their definition was modelled after the definition of the...
of states. It has also been shown that, using superposition states of the Abelian toric code anyons, some properties of the more complex non-Abelian anyons can be realized.
Generalizations
The use of a torus is not required to form an error correcting code. Other surfaces may also be used, with their topological properties determining the degeneracy of the stabilizer space. In general, quantum error correcting codes defined on two dimensional spin lattices according to the principles above are known as surface codes.It is also possible to define similar codes using higher dimensional spins. These are the quantum double models, which allow a greater richness in the behaviour of anyons, and so may be used for more advanced quantum computation and error correction proposals. These not only include models with Abelian anyons, but also those with non-Abelian statistics.
Experimental Progress
The most explicit demonstration of the properties of the toric code has been in state based approaches. Rather than attempting to realize theHamiltonian, these simply prepare the code in the stabilizer space and demonstrate the creation, transport and statistics of the anyons.
For realizations of the toric code and its generalizations with a Hamiltonian, much progress has been made using Josephson junctions. The theory of how the Hamiltonians may be implemented has been developed for a wide class of topological codes. An experiment has also been performed, realizing the toric code Hamiltonian for a small lattice, and demonstrating the quantum memory provided by its degenerate ground state.
Other theoretical work towards experimental realizations is based on cold atoms. A toolkit of methods that may be used to realize topological codes with optical lattices has been explored,
as have experiments concerning minimal instances of topological order. Progress is also being made into simulations of the toric model with Rydberg atoms, in which the Hamiltonian and the effects of dissipative noise can be demonstrated.