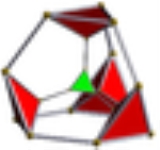
Truncated 5-cell

5-cell	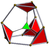Truncated 5-cell	Bitruncated 5-cell
Schlegel diagrams centered on [3,3] (cells at opposite at [3,3])

In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, a truncated 5-cell is a uniform polychoron

Uniform polychoron

In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....

(4-dimensional uniform polytope

Polytope

In elementary geometry, a polytope is a geometric object with flat sides, which exists in any general number of dimensions. A polygon is a polytope in two dimensions, a polyhedron in three dimensions, and so on in higher dimensions...

) formed as the truncation

Truncation (geometry)

In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex.- Uniform truncation :...

of the regular 5-cell.

There are two degrees of trunctions, including a bitruncation

Bitruncation

In geometry, a bitruncation is an operation on regular polytopes. It represents a truncation beyond rectification. The original edges are lost completely and the original faces remain as smaller copies of themselves....

Truncated 5-cell
Schlegel diagram (tetrahedron Tetrahedron In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids... cells visible)
Type	Uniform polychoron Uniform polychoron In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....
Schläfli symbol	t_0,1{3,3,3}
Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors...
Cells	10	5 (3.3.3) Tetrahedron In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids... 5 (3.6.6) Truncated tetrahedron In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 regular triangular faces, 12 vertices and 18 edges.- Area and volume :...
Faces	30	20 {3} 10 {6}
Edges	40
Vertices	20
Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...	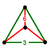Irr. tetrahedron
Symmetry group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...	A₄, [3,3,3], order 120
Properties	convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... , isogonal
Uniform index	2 Rectified 5-cell In four dimensional geometry, the rectified 5-cell is a uniform polychoron composed of 5 regular tetrahedral and 5 regular octahedral cells. Each edge has one tetrahedron and two octahedra. Each vertex has two tetrahedra and three octahedra. In total it has 30 triangle faces, 30 edges, and 10... 3 4 Cantellated 5-cell In four-dimensional geometry, a cantellated 5-cell is a convex uniform polychoron, being a cantellation of the regular 5-cell.There are 2 unique degrees of runcinations of the 5-cell including with permutations truncations....

The truncated 5-cell, truncated pentatope or truncated 4-simplex is bounded by 10 cells

Cell (geometry)

In geometry, a cell is a three-dimensional element that is part of a higher-dimensional object.- In polytopes :A cell is a three-dimensional polyhedron element that is part of the boundary of a higher-dimensional polytope, such as a polychoron or honeycomb For example, a cubic honeycomb is made...

: 5 tetrahedra

Tetrahedron

In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids...

, and 5 truncated tetrahedra

Truncated tetrahedron

In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 regular triangular faces, 12 vertices and 18 edges.- Area and volume :...

. Each vertex is surrounded by 3 truncated tetrahedra and one tetrahedron; the vertex figure

Vertex figure

In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...

is an elongated tetrahedron.

Construction

The truncated 5-cell may be constructed from the 5-cell by truncating

Truncation (geometry)

In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex.- Uniform truncation :...

its vertices at 1/3 the edge length. This truncates the 5 tetrahedral cells into truncated tetrahedra, and introduces 5 new tetrahedral cells positioned on the original vertices.

Structure

The truncated tetrahedra are joined to each other at their hexagonal faces, and to the tetrahedra at their triangular faces.

Projections

The tetrahedron-first parallel projection of the truncated 5-cell into 3-dimensional space has the following structure:

The projection envelope is a truncated tetrahedron
Truncated tetrahedron
In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 regular triangular faces, 12 vertices and 18 edges.- Area and volume :...

.
One of the truncated tetrahedral cells project onto the entire envelope.
One of the tetrahedral cells project onto a tetrahedron lying at the center of the envelope.
Four flattened tetrahedra are joined to the triangular faces of the envelope, and connected to the central tetrahedron via 4 radial edges. These are the images of the remaining 4 tetrahedral cells.
Between the central tetrahedron and the 4 hexagonal faces of the envelope are 4 irregular truncated tetrahedral volumes, which are the images of the 4 remaining trucated tetrahedral cells.

This layout of cells in projection is analogous to the layout of faces in the face-first projection of the truncated tetrahedron into 2-dimensional space. The truncated 5-cell is the 4-dimensional analogue of the truncated tetrahedron.

Alternate names

Truncated pentatope
Truncated 4-simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
Truncated pentachoron (Acronym: tip) (Jonathan Bowers)

Coordinates

The Cartesian coordinates for the vertices of an origin-centered truncated 5-cell having edge length 2 are: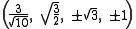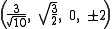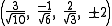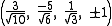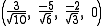

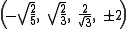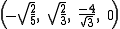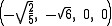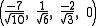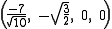More simply, the vertices of the truncated 5-cell can be constructed on a hyperplane

Hyperplane

A hyperplane is a concept in geometry. It is a generalization of the plane into a different number of dimensions.A hyperplane of an n-dimensional space is a flat subset with dimension n − 1...

in 5-space as permutations of (0,0,0,1,2) or of (0,1,2,2,2). These coordinates come from positive orthant

Orthant

In geometry, an orthant or hyperoctant is the analogue in n-dimensional Euclidean space of a quadrant in the plane or an octant in three dimensions....

facets of the truncated pentacross and bitruncated penteract respectively.

Bitruncated 5-cell

Schlegel diagram with alternate cells hidden.
Bitruncated 5-cell
Type	Uniform polychoron Uniform polychoron In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....
Schläfli symbol	t_1,2{3,3,3}
Coxeter-Dynkin diagram Coxeter-Dynkin diagram In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors...
Cells	10 (3.6.6 Truncated tetrahedron In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 regular triangular faces, 12 vertices and 18 edges.- Area and volume :... )
Faces	40	20 {3} 20 {6}
Edges	60
Vertices	30
Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...	(Tetragonal disphenoid)
Symmetry group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...	A₄, 3,3,3, order 240
Properties	convex Convex polytope A convex polytope is a special case of a polytope, having the additional property that it is also a convex set of points in the n-dimensional space Rn... , isogonal isotoxal, isochoric
Uniform index	5 6 7

The bitruncated 5-cell (also called a bitruncated pentachoron, decachoron and 10-cell) is a 4-dimensional polytope

Polytope

, or polychoron

Polychoron

In geometry, a polychoron or 4-polytope is a four-dimensional polytope. It is a connected and closed figure, composed of lower dimensional polytopal elements: vertices, edges, faces , and cells...

, composed of 10 cells in the shape of truncated tetrahedra

Truncated tetrahedron

In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 regular triangular faces, 12 vertices and 18 edges.- Area and volume :...

. Each hexagonal face of the truncated tetrahedra is joined in complementary orientation to the neighboring truncated tetrahedron. Each edge is shared by two hexagons and one triangle. Each vertex is surrounded by 4 truncated tetrahedral cells in a tetragonal disphenoid vertex figure

Vertex figure

In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...

.

The bitruncated 5-cell is the intersection

Intersection (set theory)

In mathematics, the intersection of two sets A and B is the set that contains all elements of A that also belong to B , but no other elements....

of two pentachora

Pentachoron

In geometry, the 5-cell is a four-dimensional object bounded by 5 tetrahedral cells. It is also known as the pentachoron, pentatope, or hyperpyramid...

in dual configuration. As such, it is also the intersection of a penteract

Penteract

In five dimensional geometry, a 5-cube is a name for a five dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract hypercells....

with the hyperplane that bisects the penteract's long diagonal orthogonally. In this sense it is the 4-dimensional analog of the regular octahedron

Octahedron

In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....

(intersection of regular tetrahedra in dual configuration / tesseract

Tesseract

In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...

bisection on long diagonal) and the regular hexagon (equilateral triangles / cube). The 5-dimensional analog is the birectified 5-simplex, and the

-dimensional analog is the polytope whose Coxeter–Dynkin diagram

Coxeter-Dynkin diagram

In geometry, a Coxeter–Dynkin diagram is a graph with numerically labeled edges representing the spatial relations between a collection of mirrors...

is linear with rings on the middle one or two nodes.

The bitruncated 5-cell is one of the two non-regular uniform polychora

Uniform polychoron

In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....

which are cell-transitive. The other is the bitruncated 24-cell, which is composed of 48 truncated cubes.

Symmetry

This polychoron has a higher extended pentachoric symmetry (A₄, 3,3,3), doubled to order 240, because the element corresponding to any element of the underlying 5-cell can be exchanged with one of those corresponding to an element of its dual.

Alternative names

Bitruncated 5-cell (Norman W. Johnson)
10-cell as a cell-transitive 4-polytope
Bitruncated pentachoron
Bitruncated pentatope
Bitruncated 4-simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
Decachoron (Acronym: deca) (Jonathan Bowers)

Images

stereographic projection of spherical polychoron (centred on a hexagon face)	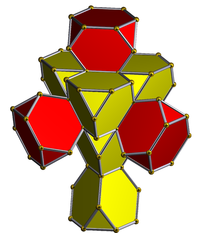Net (polytope)

Coordinates

The Cartesian coordinates of an origin-centered bitruncated 5-cell having edge length 2 are: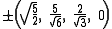

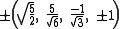
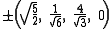
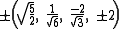
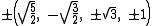
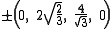
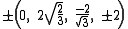
More simply, the vertices of the bitruncated 5-cell can be constructed on a hyperplane

Hyperplane

A hyperplane is a concept in geometry. It is a generalization of the plane into a different number of dimensions.A hyperplane of an n-dimensional space is a flat subset with dimension n − 1...

in 5-space as permutations of (0,0,1,2,2). These represent positive orthant

Orthant

In geometry, an orthant or hyperoctant is the analogue in n-dimensional Euclidean space of a quadrant in the plane or an octant in three dimensions....

facets of the bitruncated pentacross.

Another 5-space construction are all 20 permutations of (1,0,0,0,-1).

Related regular skew polyhedron

The regular skew polyhedron

Regular skew polyhedron

In geometry, the regular skew polyhedra are generalizations to the set of regular polyhedron which include the possibility of nonplanar faces or vertex figures....

, {6,4|3}, exists in 4-space with 4 hexagonal around each vertex, in a zig-zagging nonplanar vertex figure. These hexagonal faces can be seen on the bitruncated 5-cell, using all 60 edges and 20 vertices. The 20 triangular faces of the bitruncated 5-cell can be seen as removed. The dual regular skew polyhedron, {4,6|3}, is similarly related to the square faces of the runcinated 5-cell.

Related polytopes

These polytope are from a set of 9 uniform polychora

Uniform polychoron

In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....

constructed from the [3,3,3] Coxeter group

Coxeter group

In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.