Field emission
Encyclopedia
Field emission (also known as field electron emission and electron field emission) is emission of electron
s induced by an electrostatic field. The most common context is FE from a solid surface into vacuum. However, FE can take place from solid or liquid surfaces, into vacuum, air, a fluid, or any non-conducting or weakly-conducting dielectric. The field-induced promotion of electrons from the valence to conduction band of semiconductors (the Zener effect
) can also be regarded as a form of FE. The terminology is historical because related phenomena of surface photoeffect, thermionic emission or Richardson-Dushman effect and "cold electronic emission", i.e. the emission of electrons in strong static (or quasi-static) electric fields, were discovered and studied independently from 1880s to 1930s. When field emission is used without qualifiers it typically means the "cold emission."
Field emission in pure metals occurs in high electric fields: the gradients are typically higher than 1 gigavolt per metre and strongly dependent upon the work function
. Electron sources based on field emission have a number of applications, but it is most commonly an undesirable primary source of vacuum breakdown and electrical discharge
phenomena, which engineers work to prevent. Examples of applications for surface field emission include construction of bright electron sources for high-resolution electron microscope
s or to discharge spacecraft
from induced charges. Devices which eliminate induced charges are termed charge-neutralizers.
Field emission was explained by quantum tunneling of electrons in the late 1920s. This was one of the triumphs of the nascent quantum mechanics
. The theory of field emission from bulk metals was proposed by Fowler
and Nordheim
.
A family of approximate equations, "Fowler–Nordheim equations", is named after them. Strictly, Fowler-Nordheim equations apply only to field emission from bulk metals and (with suitable modification) to other bulk crystalline solids, but they are often used – as a rough approximation – to describe field emission from other materials.
In some respects, field electron emission is a paradigm example of what physicists mean by tunneling
. Unfortunately, it is also a paradigm example of the intense mathematical difficulties that can arise. Simple solvable models of the tunneling barrier lead to equations (including the original 1928 Fowler-Nordheim-type equation) that get predictions of emission current density too low by a factor of 100 or more. If one inserts a more realistic barrier model into the simplest form of the Schrödinger equation
, then an awkward mathematical problem arises over the resulting differential equation: it is known to be mathematically impossible in principle to solve this equation exactly in terms of the usual functions of mathematical physics, or in any simple way. To get even an approximate solution, it is necessary to use special approximate methods known in physics as "semi-classical" or "quasi-classical" methods. Worse, a mathematical error was made in the original application of these methods to field emission, and even the corrected theory that was put in place in the 1950s has been formally incomplete until very recently. A consequence of these (and other) difficulties has been a heritage of misunderstanding and disinformation that still persists in some current field emission research literature. This article tries to present a basic account of field emission "for the 21st century and beyond" that is free from these confusions.
Fowler–Nordheim tunneling is the wave-mechanical tunneling of electrons through a rounded triangular barrier created at the surface of an electron conductor by applying a very high electric field. Individual electrons can escape by Fowler-Nordheim tunneling from many materials in various different circumstances.
Cold field electron emission (CFE) is the name given to a particular statistical emission regime, in which the electrons in the emitter are initially in internal thermodynamic equilibrium
, and in which most emitted electrons escape by Fowler-Nordheim tunneling from electron states close to the emitter Fermi level. [By contrast, in the Schottky emission
regime, most electrons escape over the top of a field-reduced barrier, from states well above the Fermi level.] Many solid and liquid materials can emit electrons in a CFE regime if an electric field of an appropriate size is applied.
Fowler–Nordheim-type equations are a family of approximate equations derived to describe CFE from the internal electron states in bulk metals. The different members of the family represent different degrees of approximation to reality. Approximate equations are necessary because, for physically realistic models of the tunneling barrier, it is mathematically impossible in principle to solve the Schrödinger equation
exactly in any simple way. There is no theoretical reason to believe that Fowler-Nordheim-type equations validly describe field emission from materials other than bulk crystalline solids.
For metals, the CFE regime extends to well above room temperature. There are other electron emission regimes (such as "thermal electron emission
" and "Schottky emission
") that require significant external heating of the emitter. There are also emission regimes where the internal electrons are not in thermodynamic equilibrium and the emission current is, partly or completely, determined by the supply of electrons to the emitting region. A non-equilibrium emission process of this kind may be called field (electron) emission if most of the electrons escape by tunneling, but strictly it is not CFE, and is not accurately described by a Fowler-Nordheim-type equation.
Care is necessary because in some contexts (e.g. spacecraft engineering), the name "field emission" is applied to the field-induced emission of ions (field ion emission), rather than electrons, and because in some theoretical contexts "field emission" is used as a general name covering both field electron emission and field ion emission.
Historically, the phenomenon of field electron emission has been known by a variety of names, including "the aeona effect", "autoelectronic emission", "cold emission", "cold cathode emission", "field emission", "field electron emission" and "electron field emission".
Equations in this article are written using the International System of Quantities (ISQ). This is the modern (post-1970s) international system, based around the rationalized-meter-kilogram-second (rmks) system of equations, which is used to define SI units. Older field emission literature (and papers that directly copy equations from old literature) often write some equations using an older equation system that does not use the quantity ε0. In this article, all such equations have been converted to modern international form. For clarity, this should always be done.
Since work function is normally given in electronvolts (eV), and it is often convenient to measure fields in volts per nanometer (V/nm), values of most universal constants are given here in units involving the eV, V and nm. Increasingly, this is normal practice in field emission research. However, all equations here are ISQ-compatible equations and remain dimensionally consistent, as is required by the modern international system. To indicate their status, numerical values of universal constants are given to seven significant figures. Values are derived using the 2006 values of the fundamental constants.
In retrospect, it seems likely that the electrical discharges reported by Winkler in 1744 were started by CFE from his wire electrode. However, meaningful investigations had to wait until after J.J. Thomson's identification of the electron in 1897, and until after it was understood – from thermal emission
and photo-emission work – that electrons could be emitted from inside metals (rather than from surface-adsorbed gas molecules
), and that – in the absence of applied fields – electrons escaping from metals had to overcome a work function barrier.
It was suspected at least as early as 1913 that field-induced emission was a separate physical effect. However, only after vacuum and specimen cleaning techniques had significantly improved, did this become well established. Lilienfeld
(who was primarily interested in electron sources for medical X-ray
applications) published in 1922 the first clear account in English of the experimental phenomenology of the effect he had called "autoelectronic emission". He had worked on this topic, in Leipzig, since about 1910. Kleint describes this and other early work.
After 1922, experimental interest increased, particularly in the groups led by Millikan at the California Institute in Pasadena, and by Gossling at the General Electric Company in London. Attempts to understand autoelectronic emission included plotting experimental current-voltage (i - V) data in different ways, to look for a straight-line relationship. Current increased with voltage more rapidly than linearly, but plots of type (log(i) vs. V) were not straight. Schottky
suggested in 1923 that the effect might be due to thermally-induced emission over a field-reduced barrier. If so, then plots of type (log(i) vs. V1/2) should be straight; but they were not. Nor is Schottky's explanation compatible with the experimental observation of only very weak temperature dependence in CFE – a point initially overlooked.
A breakthrough came when Lauritsen
(and Oppenheimer independently) found that plots of type (log(i) vs. 1/V) yielded good straight lines. This result, published by Millikan and Lauritsen in early 1928, was known to Fowler
and Nordheim
.
Oppenheimer had predicted that the field-induced tunneling of electrons from atoms (the effect now called field ionization) would have this i(V) dependence, had found this dependence in the published experimental field emission results of Millikan and Eyring, and proposed that CFE was due to field-induced tunneling
of electrons from atomic-like orbitals
in surface metal atoms. An alternative Fowler-Nordheim theory explained both the Millikan-Lauritsen finding and the very weak dependence of current on temperature. Fowler-Nordheim theory predicted both to be consequences if CFE were due to field-induced tunneling from free-electron-type states
in what we would now call a metal conduction band
, with the electron states occupied in accordance with Fermi–Dirac statistics.
In fact, Oppenheimer (although right in principle about the theory of field ionization) had mathematical details of his theory seriously incorrect. There was also a small numerical error in the final equation given by Fowler-Nordheim theory for CFE current density
: this was corrected in the 1929 paper of .
Strictly, if the barrier field in Fowler-Nordheim 1928 theory is exactly proportional to the applied voltage, and if the emission area is independent of voltage, then the Fowler-Nordheim 1928 theory predicts that plots of the form (log(i/V2) vs. 1/V) should be exact straight lines. However, contemporary experimental techniques were not good enough to distinguish between the Fowler-Nordheim theoretical result and the Millikan-Lauritsen experimental result.
Thus, by 1928 basic physical understanding of the origin of CFE from bulk metals had been achieved, and the original Fowler-Nordheim-type equation had been derived.
The literature often presents Fowler-Nordheim work as a proof of the existence of electron tunneling
, as predicted by wave-mechanics. Whilst this is correct, the validity of wave-mechanics was largely accepted by 1928. The more important role of the Fowler-Nordheim paper was that it was a convincing argument from experiment that Fermi–Dirac statistics applied to the behavior of electrons in metals, as suggested by Sommerfeld
in 1927. The success of Fowler-Nordheim theory did much to support the correctness of Sommerfeld's ideas, and greatly helped to establish modern electron band theory
. In particular, the original Fowler-Nordheim-type equation was one of the first to incorporate the statistical-mechanical
consequences of the existence of electron spin into the theory of an experimental condensed-matter effect. The Fowler-Nordheim paper also established the physical basis for a unified treatment of field-induced and thermally-induced electron emission
. Prior to 1928 it had been hypothesized that two types of electrons, "thermions" and "conduction electrons", existed in metals, and that thermally emitted electron currents were due to the emission of thermions, but that field-emitted currents were due to the emission of conduction electrons. The Fowler-Nordheim 1928 work suggested that thermions did not need to exist as a separate class of internal electrons: electrons could come from a single band
occupied in accordance with Fermi–Dirac statistics, but would be emitted in statistically different ways under different conditions of temperature and applied field.
The ideas of Oppenheimer, Fowler
and Nordheim
were also an important stimulus to the development, by Gurney
and Condon
, later in 1928, of the theory of the radioactive decay
of nuclei (by alpha particle
tunneling).
desire to develop miniaturized X-ray
tubes for medical applications. However, it was too early for this technology to succeed.
After Fowler-Nordheim theoretical work in 1928, a major advance came with the development in 1937 by Erwin W. Mueller of the spherical-geometry field electron microscope (FEM) (also called the "field emission microscope"). In this instrument, the electron emitter is a sharply pointed wire, of apex radius r. This is placed, in a vacuum enclosure, opposite an image detector (originally a phosphor screen), at a distance R from it. The microscope screen shows a projection image of the distribution of current-density J across the emitter apex, with magnification approximately (R/r), typically 105 to 106. In FEM studies the apex radius is typically 100 nm to 1 μm. The tip of the pointed wire, when referred to as a physical object, has been called a "field emitter", a "tip", or (recently) a "Mueller emitter".
When the emitter surface is clean, this FEM image is characteristic of: (a) the material from which the emitter is made: (b) the orientation of the material relative to the needle/wire axis; and (c) to some extent, the shape of the emitter endform. In the FEM image, dark areas correspond to regions where the local work function φ is relatively high and/or the local barrier field F is relatively low, so J is relatively low; the light areas correspond to regions where φ is relatively low and/or F is relatively high, so J is relatively high. This is as predicted by the exponent of Fowler-Nordheim-type equations [see eq. (30) below].
The adsorption
of layers of gas atoms (such as oxygen) onto the emitter surface, or part of it, can create surface electric dipoles that change the local work function of this part of the surface. This affects the FEM image; also, the change of work-function can be measured using a Fowler-Nordheim plot (see below). Thus, the FEM became an early observational tool of surface science
. For example, in the 1960s, FEM results contributed significantly to discussions on heterogeneous catalysis
. FEM has also been used for studies of surface-atom diffusion
. However, FEM has now been almost completely superseded by newer surface-science techniques.
A consequence of FEM development, and subsequent experimentation, was that it became possible to identify (from FEM image inspection) when an emitter was "clean", and hence exhibiting its clean-surface work-function as established by other techniques. This was important in experiments designed to test the validity of the standard Fowler-Nordheim-type equation.
These experiments deduced a value of voltage-to-barrier-field conversion factor β from a Fowler-Nordheim plot (see below), assuming the clean-surface φ–value for tungsten, and compared this with values derived from electron-microscope observations of emitter shape and electrostatic modeling. Agreement to within about 10% was achieved. Only very recently has it been possible to do the comparison the other way round, by bringing a well-prepared probe so close to a well-prepared surface that approximate parallel-plate geometry can be assumed and the conversion factor can be taken as 1/W, where W is the measured probe-to emitter separation. Analysis of the resulting Fowler-Nordheim plot yields a work-function value close to the independently known work-function of the emitter.
about a point in the emitter is very nearly conserved. Hence any kinetic energy
that, at emission, is in a direction parallel to the emitter surface gets converted into energy associated with the radial direction of motion. So what gets measured in an energy analyzer is the total energy at emission.
With the development of sensitive electron energy analyzers in the 1960s, it became possible to measure fine details of the total energy distribution. These reflect fine details of the surface physics, and the technique of Field Electron Spectroscopy flourished for a while, before being superseded by newer surface-science techniques.
), it is helpful to start with an electron source that is small, optically bright and stable. Sources based on the geometry of a Mueller emitter qualify well on the first two criteria. The first electron microscope
(EM) observation of an individual atom was made by Crewe, Wall and Langmore in 1970, using a scanning electron microscope
equipped with an early field emission gun.
From the 1950s onwards, extensive effort has been devoted to the development of field emission sources for use in electron gun
s. [e.g., DD53] Methods have been developed for generating on-axis beams, either by field-induced emitter build-up, or by selective deposition of a low-work-function adsorbate
(usually Zirconium oxide - ZrO) into the flat apex of a (100) oriented
Tungsten
emitter.
Sources that operate at room temperature have the disadvantage that they rapidly become covered with adsorbate molecule
s that arrive from the vacuum
system walls, and the emitter has to be cleaned from time to time by "flashing" to high temperature. Nowadays, it is more common to use Mueller-emitter-based sources that are operated at elevated temperatures, either in the Schottky emission
regime or in the so-called temperature-field intermediate regime. Many modern high-resolution electron microscopes and electron beam instruments use some form of Mueller-emitter-based electron source. Currently, attempts are being made to develop carbon nanotubes (CNTs) as electron-gun field emission sources.
The use of field emission sources in electron optical instruments has involved the development of appropriate theories of charged particle optics, and the development of related modeling. Various shape models have been tried for Mueller emitters; the best seems to be the "Sphere on Orthogonal Cone" (SOC) model introduced by Dyke, Trolan. Dolan and Barnes in 1953. Important simulations, involving trajectory tracing using the SOC emitter model, were made by Wiesener and Everhart. Nowadays, the facility to simulate field emission from Mueller emitters is often incorporated into the commercial electron-optics programmes used to design electron beam instruments. The design of efficient modern field-emission electron guns requires highly specialized expertise.
(FIM) images of the emitter. Single-atom-apex Mueller emitters also have relevance to the scanning probe microscopy
and helium scanning ion microscopy
(He SIM). Techniques for preparing them have been under investigation for many years. A related important recent advance has been the development (for use in the He SIM) of an automated technique for restoring a three-atom ("trimer") apex to its original state, if the trimer breaks up.
One of the original two device types, the "Spindt array", used silicon-integrated-circuit (IC)
fabrication techniques to make regular arrays in which molybdenum
cones were deposited in small cylindrical voids in an oxide film, with the void covered by a counterelectrode with a central circular aperture. This overall geometry has also been used with carbon nanotubes grown in the void.
The other original device type was the "Latham emitter". These were MIMIV (metal-insulator-metal-insulator-vacuum) – or, more generally, CDCDV (conductor-dielectric-conductor-dielectric-vacuum) – devices that contained conducting particulates in a dielectric film. The device field-emits because its microstructure/nanostructure has field-enhancing properties. This material had a potential production advantage, in that it could be deposited as an "ink", so IC fabrication techniques were not needed. However in practice, uniformly reliable devices proved difficult to fabricate.
Research advanced to look for other materials that could be deposited/grown as thin films with suitable field-enhancing properties. In a parallel-plate arrangement, the "macroscopic" field FM between the plates is given by FM = V/W, where W is the plate separation and V is the applied voltage. If a sharp object is created on one plate, then the local field F at its apex is greater than FM and can be related to FM by
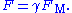
The parameter γ is called the "field enhancement factor" and is basically determined by the object's shape. Since field emission characteristics are determined by the local field F, then the higher the γ-value of the object, then the lower the value of FM at which significant emission occurs. Hence, for a given value of W, the lower the applied voltage V at which significant emission occurs.
For a roughly ten year-period from the mid-1990s, there was great interest in field emission from plasma-deposited films of amorphous and "diamond-like" carbon. However, interest subsequently lessened, partly due to the arrival of CNT
emitters, and partly because evidence emerged that the emission sites might be associated with particulate carbon objects created in an unknown way during the deposition process
: this suggested that quality control
of an industrial-scale production process might be problematic.
The introduction of CNT field emitters, both in "mat" form and in "grown array" forms, was a significant step forwards. Extensive research has been undertaken into both their physical characteristics and possible technological applications. For field emission, an advantage of CNTs is that, due to their shape, with its high aspect ratio
, they are "natural field-enhancing objects".
In recent years there has also been massive growth in interest in the development of other forms of thin-film emitter, both those based on other carbon forms (such as "carbon nanowalls") and on various forms of wide-band-gap semiconductor. A particular aim is to develop "high-γ" nanostructures with a sufficiently high density of individual emission sites.
Common problems with all field emission devices, particularly those that operate in "industrial vacuum conditions" is that the emission performance can be degraded by the adsorption of gas atoms arriving from elsewhere in the system, and the emitter shape can be in principle be modified deleteriously by a variety of unwanted subsidiary processes, such as bombardment by ions created by the impact of emitted electrons onto gas-phase atoms and/or onto the surface of counter-electrodes. Thus, an important industrial requirement is "robustness in poor vacuum conditions"; this needs to be taken into account in research on new emitter materials.
At the time of writing, the most promising forms of large-area field emission source (certainly in terms of achieved average emission current density) seem to be Spindt arrays and the various forms of source based on CNTs.
. These are known as "field emission display
s" or "nano-emissive displays". Although several prototypes have been demonstrated, the development of such displays into reliable commercial products has been hindered by a variety of industrial production problems not directly related to the source characteristics [En08].
Other proposed applications of large-area field emission sources include microwave
generation, space-vehicle neutralization, X-ray generation, and (for array sources) multiple e-beam lithography
. There are also recent attempts to develop large-area emitters on flexible substrates, in line with wider trends towards "plastic electronics".
The development of such applications is the mission of vacuum nanoelectronics. However, field emitters work best in conditions of good ultrahigh vacuum. Their most successful applications to date (FEM, FES and EM guns) have occurred in these conditions. The sad fact remains that field emitters and industrial vacuum conditions do not go well together, and the related problems of reliably ensuring good "vacuum robustness" of field emission sources used in such conditions still await better solutions (probably cleverer materials solutions) than we currently have.
"), takes place by a field-induced tunneling process that can be regarded as a form of Fowler-Nordheim tunneling. For example, Rhoderick's
book discusses the theory relevant to metal-semiconductor contacts
.
", by considering electron tunneling
through a rounded triangular barrier; (2) an integration over internal electron states to obtain the "total energy distribution"; (3) a second integration, to obtain the emission current density as a function of local barrier field and local work function; (4) conversion of this to a formula for current as a function of applied voltage. The modified equations needed for large-area emitters, and issues of experimental data analysis, are dealt with separately.
Fowler–Nordheim tunneling is the wave-mechanical tunneling
of an electron through an exact or rounded triangular barrier. Two basic situations are recognized: (1) when the electron is initially in a localized state
; (2) when the electron is initially not strongly localized, and is best represented by a travelling wave. Emission from a bulk metal
conduction band
is a situation of the second type, and discussion here relates to this case. It is also assumed that the barrier is one-dimensional (i.e., has no lateral structure), and has no fine-scale structure that causes "scattering
" or "resonance" effects. To keep this explanation of Fowler-Nordheim tunneling relatively simple, these assumptions are needed; but the atomic structure of matter is in effect being disregarded.
can be written in the form
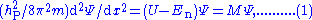
where Ψ(x) is the electron wave-function, expressed as a function of distance x measured from the emitter's electrical surface, hP is Planck's constant, m is the electron mass, U(x) is the electron potential energy
, En is the total electron energy associated with motion in the x-direction, and M(x) [=U(x)–En] is called the electron motive energy. M(x) can be interpreted as the negative of the electron kinetic energy associated with the motion of a hypothetical classical point electron in the x-direction, and is positive in the barrier.
The shape of a tunneling barrier is determined by how M(x) varies with position, in the region where M(x)>0. Two models have special status in field emission theory. (1) The exact triangular (ET) barrier is described by
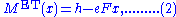
where h is the zero-field height (or unreduced height) of the barrier, e is the elementary positive charge
, and F is the barrier field. By convention, F is taken as positive, even though the classical electrostatic field
would be negative. (2) The Schottky-Nordheim (SN) barrier is described by
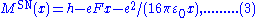
where ε0 is the electric constant (formerly called the "permittivity of free space"). This uses the classical image potential energy to represent the physical effect "correlation and exchange".
" or "penetration coefficient") is a function of h and F, and is denoted by D(h,F). The primary aim of tunneling theory is to calculate D(h,F). For physically realistic barrier models, such as the Schottky-Nordheim barrier, the Schrödinger equation
cannot be solved exactly in any simple way. The following so-called "semi-classical" approach can be used. A parameter G(h,F) can be defined by the JWKB (Jeffreys-Wentzel-Kramers-Brillouin)
integral:
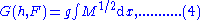
where the integral is taken across the barrier (i.e., across the region where M>0), and the parameter g is a universal constant given by
Forbes has re-arranged a result proved by Fröman and Fröman, to show that – formally – in a one-dimensional treatment – the exact solution for D can be written
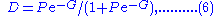
where the tunneling pre-factor P can in principle be evaluated by complicated iterative integrations along a path in complex space
. In the CFE regime we have (by definition) G>>1. Also, for simple models P~1. So eq. (6) reduces to the so-called simple JWKB
formula:
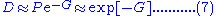
For the exact triangular barrier, putting eq. (2) into eq. (4) yields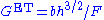
, where
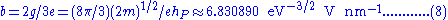
This parameter b is a universal constant sometimes called the Second Fowler–Nordheim Constant. For barriers of other shapes, we write:
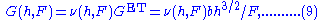
where
("nu") is a correction factor that in general has to be determined by numerical integration
, using eq. (4).
is a function of a single variable fh, defined by 
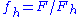, where 
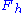is the field necessary to reduce the height of a Schottky-Nordheim barrier from h to 0. This field is given by:
.
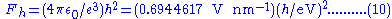The parameter fh runs from 0 to 1, and may be called the scaled barrier field, for a Schottky-Nordheim barrier of zero-field height h.
For the Schottky-Nordheim barrier,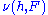
is given by the particular value v(fh) of a function v(
). The latter is a function of mathematical physics in its own right and has been called the Principal Schottky-Nordheim Barrier Function. An explicit series expansion for v(
) is derived in. The following good simple approximation for v(fh) has been found:
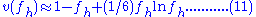
D decreases as the barrier height h increases; dh is defined by:
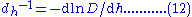
When h increases by dh then the escape probability D decreases by a factor close to e [≈2.718282], where e is the base of natural logarithms. For an elementary model, based on the exact triangular barrier, where we put
and P ≈ 1, we get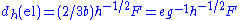 
. The decay width dh derived from the more general expression (12) differs from this by a "decay-width correction factor" λd, so:
Usually, the correction factor can be approximated as unity.
The decay-width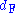
for a barrier with h equal to the local work-function φ is of special interest. Numerically this is given by:
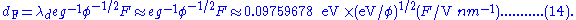
For metals, the value of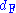
is typically of order 0.2 eV, but varies with barrier-field F.
and Houston in 1953, and its mathematics was developed further by Murphy and Good in 1956. This corrected function, sometimes known as a "special field emission elliptic function", was expressed as a function of a mathematical variable y known as the "Nordheim parameter". Only recently (2006 to 2008) has it been realized that, mathematically, it is much better to use the variable
[
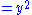]. And only recently has it been possible to complete the definition of v(
) by developing and proving the validity of an exact series expansion for this function (by starting from known special-case solutions of the Gauss hypergeometric differential equation
). Also, approximation (11) has been found only recently. Approximation (11) outperforms, and will presumably eventually displace, all older approximations of equivalent complexity. These recent developments, and their implications, will probably have a significant impact on field emission research in due course.
The following summary brings these results together. For tunneling well below the top of a well-behaved barrier of reasonable height, the escape probability D(h,F) is given formally by:
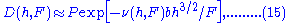
where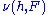
is a correction factor that in general has to be found by numerical integration. For the special case of a Schottky-Nordheim barrier, an analytical result exists and 
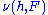is given by v(fh), as discussed above; approximation (11) for v(fh) is more than sufficient for all technological purposes. The pre-factor P is also in principle a function of h and (maybe) F, but for the simple physical models discussed here it is usually satisfactory to make the approximation P=1. The exact triangular barrier is a special case where the Schrödinger equation
can be solved exactly, as was done by Fowler and Nordheim; for this physically unrealistic case,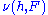
=1, and an analytical approximation for P exists.
The approach described here was originally developed to describe Fowler-Nordheim tunneling from smooth, classically flat, planar emitting surfaces. It is adequate for smooth, classical curved surfaces of radii down to about 10 to 20 nm. It can be adapted to surfaces of sharper radius, but quantities such as
and D then become significant functions of the parameter(s) used to describe the surface curvature. When the emitter is so sharp that atomic-level detail cannot be neglected, and/or the tunneling barrier is thicker than the emitter-apex dimensions, then a more sophisticated approach is desirable.
As noted at the beginning, the effects of the atomic structure of materials are disregarded in the relatively simple treatments of field electron emission discussed here. Taking atomic structure properly into account is a very difficult problem, and only limited progress has been made. However, it seems probable that the main influences on the theory of Fowler-Nordheim tunneling will (in effect) be to change the values of P and
in eq. (15), by amounts that cannot easily be estimated at present.
All these remarks apply in principle to Fowler-Nordheim tunneling from any conductor where (before tunneling) the electrons may be treated as in travelling-wave states. The approach may be adapted to apply (approximately) to situations where the electrons are initially in localized states
at or very close inside the emitting surface, but this is beyond the scope of this article.
The theoretical explanation here follows the approach of Forbes. If ε denotes the total electron energy relative to the emitter Fermi level, and Kp denotes the kinetic energy
of the electron parallel to the emitter surface, then the electron's normal energy εn (sometimes called its "forwards energy") is defined by
.
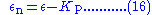Two types of theoretical energy distribution are recognized: the normal-energy distribution (NED), which shows how the energy εn is distributed immediately after emission (i.e., immediately outside the tunneling barrier); and the total-energy distribution, which shows how the total energy ε is distributed. When the emitter Fermi level is used as the reference zero level, both ε and εn can be either positive or negative.
Energy analysis experiments have been made on field emitters since the 1930s. However, only in the late 1950s was it realized (by Young and Mueller[,YM58]) that these experiments always measured the total energy distribution, which is now usually denoted by j(ε). This is also true (or nearly true) when the emission comes from a small field enhancing protrusion on an otherwise flat surface.
To see how the total energy distribution can be calculated within the framework of a Sommerfeld free-electron-type model
, look at the P-T energy-space diagram (P-T="parallel-total").
This shows the "parallel kinetic energy
" Kp on the horizontal axis and the total energy ε on the vertical axis. An electron inside the bulk metal
usually has values of Kp and ε that lie within the lightly shaded area. It can be shown that each element dεdKp of this energy space makes a contribution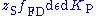
to the electron current density incident on the inside of the emitter boundary. Here, zS is the universal constant (called here the Sommerfeld
supply density):
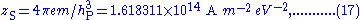
and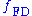
is the Fermi-Dirac distribution function
:
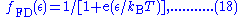
where T is thermodynamic temperature
and kB is Boltzmann's constant.
This element of incident current density sees a barrier of height h given by:
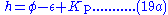
The corresponding escape probability
is D(h,F): this may be expanded (approximately) in the form
where DF is the escape probability for a barrier of unreduced height equal to the local work-function φ
. Hence, the element dεdKp makes a contribution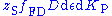
to the emission current density, and the total contribution made by incident electrons with energies in the elementary range dε is thus
,
where the integral is in principle taken along the strip shown in the diagram, but can in practice be extended to ∞ when the decay-width dF is very much less than the Fermi energy
KF (which is always the case for a metal
). The outcome of the integration can be written:
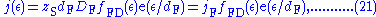
where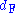
and 
are values appropriate to a barrier of unreduced height h equal to the local work function φ, and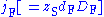 
is defined by this equation.
For a given emitter, with a given field applied to it,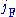
is independent of F, so eq. (21) shows that the shape of the distribution (as ε increases from a negative value well below the Fermi level) is a rising exponential, multiplied by the FD distribution function
. This generates the familiar distribution shape first predicted by Young. At low temperatures,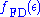
goes sharply from 1 to 0 in the vicinity of the Fermi level, and the FWHM
of the distribution is given by:
The fact that experimental CFE total energy distributions have this basic shape is a good experimental confirmation that electrons in metal
s obey Fermi-Dirac statistics
.
of a bulk metal
. The emission current density (ECD) J for some small uniform region of an emitting surface is usually expressed as a function J(φ,F) of the local work-function φ
and the local barrier field F that characterize the small region. For sharply curved surfaces, J may also depend on the parameter(s) used to describe the surface curvature.
Owing to the physical assumptions made in the original derivation, the term Fowler-Nordheim-type equation has long been used only for equations that describe the ECD at zero temperature. However, it is better to allow this name to include the slightly modified equations (discussed below) that are valid for finite temperatures within the CFE emission regime.
fFD = 1 for ε<0, and fFD = 0 for ε>0. So the ECD at 0 K, J0, is given from eq. (18) by
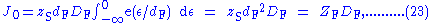
where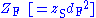
is the effective supply for state F, and is defined by this equation. Strictly, the lower limit of the integral should be –KF, where KF is the Fermi Energy
; but if dF is very much less than KF (which is always the case for a metal) then no significant contribution to the integral comes from energies below KF, and it can formally be extended to –∞.
Result (23) can be given a simple and useful physical interpretation by referring to Fig. 1. The electron state at point "F" on the diagram ("state F") is the "forwards moving state at the Fermi level" (i.e., it describes a Fermi-level electron moving normal to and towards the emitter surface). At 0 K, an electron in this state sees a barrier of unreduced height φ, and has an escape probability DF that is higher than that for any other occupied electron state. So it is convenient to write J0 as ZFDF, where the "effective supply" ZF is the current density that would have to be carried by state F inside the metal if all of the emission came out of state F.
In practice, the current density mainly comes out of a group of states close in energy to state F, most of which lie within the heavily shaded area in the energy-space diagram. Since, for a free-electron model
, the contribution to the current density is directly proportional to the area in energy space (with the Sommerfeld
supply density zS as the constant of proportionality), it is useful to think of the ECD as drawn from electron states in an area of size dF2 (measured in eV2) in the energy-space diagram. That is, it is useful to think of the ECD as drawn from states in the heavily-shaded area in Fig. 1. (This approximation gets slowly worse as temperature increases.)
ZF can also be written in the form:
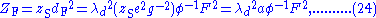
where the universal constant a, sometimes called the First Fowler–Nordheim Constant, is given by
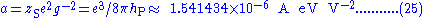
This shows clearly that the pre-exponential factor a φ−1F2, that appears in Fowler-Nordheim-type equations, relates to the effective supply of electrons to the emitter surface, in a free-electron model.
– the ECD J can be written in the form:
where λT is a temperature correction factor given by the integral. The integral can be transformed, by writing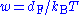
and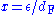 
, and then 
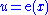, into the standard result::
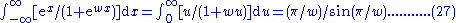
This is valid for w>1 (i.e., dF/kBT > 1). Hence – for temperatures such that kBT<dF:
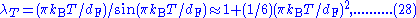
where the expansion is valid only if (πkBT /dF) << 1. An example value (for φ= 4.5 eV, F= 5 V/nm, T= 300 K) is λT= 1.024. Normal thinking has been that, in the CFE regime, λT is always small in comparison with other uncertainties, and that it is usually unnecessary to explicitly include it in formulae for the current density at room temperature.
The emission regimes for metals are, in practice, defined, by the ranges of barrier field F and temperature T for which a given family of emission equations is mathematically adequate. When the barrier field F is high enough for the CFE regime to be operating for metal emission at 0 K, then the condition kBT<dF provides a formal upper bound (in temperature) to the CFE emission regime. However, it has been argued that (due to approximations made elsewhere in the derivation) the condition kBT<0.7dF is a better working limit: this corresponds to a λT-value of around 1.09, and (for the example case) an upper temperature limit on the CFE regime of around 1770 K. This limit is a function of barrier field.
Note that result (28) here applies for a barrier of any shape (though dF will be different for different barriers).
, the prefactor P, and (to a limited extent) the correction factor λd. These changes will, in turn, affect the values of the parameter DF and (to a limited extent) the parameter dF. For a real metal
, the supply density will vary with position in energy space, and the value at point "F" may be different from the Sommerfeld supply density. We can take account of this effect by introducing an electronic-band-structure correction factor λB into eq. (23). Modinos has discussed how this factor might be calculated: he estimates that it is most likely to be between 0.1 and 1; it might lie outside these limits but is most unlikely to lie outside the range 0.01<λB<10.
By defining an overall supply correction factor λZ equal to λT λB λd2, and combining equations above, we reach the so-called physically complete Fowler-Nordheim-type equation:
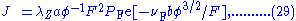
where
[=
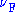(φ,F)] is the exponent correction factor for a barrier of unreduced height φ. This is the most general equation of the Fowler–Nordheim type. Other equations in the family are obtained by substituting specific expressions for the three correction factors 
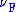, PF and λZ it contains. The so-called elementary Fowler-Nordheim-type equation, that appears in undergraduate textbook discussions of field emission, is obtained by putting λZ→1, PF→1, 
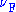→1; this does not yield good quantitative predictions because it makes the barrier stronger than it is in physical reality. The so-called standard Fowler-Nordheim-type equation, originally developed by Murphy and Good, and much used in past literature, is obtained by putting λZ→tF−2, PF→1, 
→vF, where vF is v(f), where f is the value of fh obtained by putting h=φ, and tF is a related parameter (of value close to unity).
Within the more complete theory described here, the factor tF−2 is a component part of the correction factor λd2 [see, and note that λd2 is denoted by λD there]. There is no significant value in continuing the separate identification of tF−2. Probably, in the present state of knowledge, the best approximation for simple Fowler-Nordheim-type equation based modeling of CFE from metals is obtained by putting λZ→1, PF → 1,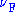
→ v(f). This re-generates the Fowler-Nordheim-type equation used by Dyke and Dolan in 1956, and can be called the "simplified standard Fowler-Nordheim-type equation".
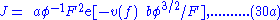
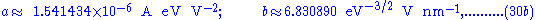
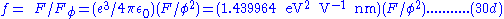
where Fφ here is the field needed to reduce to zero a Schottky-Nordheim barrier of unreduced height equal to the local work-function φ
, and f is the scaled barrier field for a Schottky-Nordheim barrier of unreduced height φ. [This quantity f could have been written more exactly as fφSN, but it makes this Fowler-Nordheim-type equation look less cluttered if the convention is adopted that simple f means the quantity denoted by fφSN in, eq. (2.16).] For the example case (φ= 4.5 eV, F= 5 V/nm), f≈ 0.36 and v(f) ≈ 0.58; practical ranges for these parameters are discussed further in.
Note that the variable f (the scaled barrier field) is not the same as the variable y (the Nordheim parameter) extensively used in past field emission literature, and that " v(f) " does NOT have the same mathematical meaning and values as the quantity " v(y) " that appears in field emission literature. In the context of the revised theory described here, formulae for v(y), and tables of values for v(y) should be disregarded, or treated as values of v(f1/2). If more exact values for v(f) are required, then provides formulae that give values for v(f) to an absolute mathematical accuracy of better than 8×10−10. However, approximation formula (30c) above, which yields values correct to within an absolute mathematical accuracy of better 0.0025, should gives values sufficiently accurate for all technological purposes.
. The approach used here was introduced by Forbes in 2004 and may be described as "integrating via the total energy distribution, using the parallel kinetic energy Kp as the first variable of integration". Basically, it is a free-electron equivalent of the Modinos procedure (in a more advanced quantum-mechanical treatment) of "integrating over the surface Brillouin zone". By contrast, the free-electron treatments of CFE by Young in 1959, Gadzuk and Plummer in 1973 and Modinos in 1984, also integrate via the total energy distribution, but use the normal energy εn (or a related quantity) as the first variable of integration.
There is also an older approach, based on a seminal paper by Nordheim in 1928, that formulates the problem differently and then uses first Kp and then εn (or a related quantity) as the variables of integration: this is known as "integrating via the normal-energy distribution". This approach continues to be used by some authors. Although it has some advantages, particularly when discussing resonance phenomena, it requires integration of the Fermi-Dirac distribution function in the first stage of integration: for non-free-electron-like electronic band-structures this can lead to very complex and error-prone mathematics (as in the work of Stratton on semiconductors). Further, integrating via the normal-energy distribution does not generate experimentally measured electron energy distributions.
In general, the approach used here seems easier to understand, and leads to simpler mathematics.
It is also closer in principle to the more sophisticated approaches used when dealing with real bulk crystalline solids, where the first step is either to integrate contributions to the ECD over constant energy surfaces in a wave-vector
space ( k -space), or to integrate contributions over the relevant surface Brillouin zone. The Forbes approach is equivalent either to integrating over a spherical surface in k -space, using the variable Kp to define a ring-like integration element that has cylindrical symmetry about an axis in a direction normal to the emitting surface, or to integrating over an (extended) surface Brillouin zone using circular-ring elements.
For CFE, basic theoretical treatments provide a relationship between the local emission current density J and the local barrier field F, at a local position on the emitting surface. Experiments measure the emission current i from some defined part of the emission surface, as a function of the voltage V applied to some counter-electrode. To relate these variables to J and F, auxiliary equations are used.
The voltage-to-barrier-field conversion factor β is defined by:
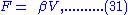
The value of F varies from position to position on an emitter surface, and the value of β varies correspondingly.
For a metal emitter, the β−value for a given position will be constant (independent of voltage) under the following conditions: (1) the apparatus is a "diode" arrangement, where the only electrodes present are the emitter and a set of "surroundings", all parts of which are at the same voltage; (2) no significant field-emitted vacuum space-charge
(FEVSC) is present (this will be true except at very high emission current densities, around 109 A/m2 or higher ); (3) no significant "patch fields" exist, as a result of non-uniformities in local work-function
(this is normally assumed to be true, but may not be in some circumstances). For non-metals, the physical effects called "field penetration" and "band bending
" [M084] can make β a function of applied voltage, although – surprisingly – there are few studies of this effect.
The emission current density J varies from position to position across the emitter surface. The total emission current i from a defined part of the emitter is obtained by integrating J across this part. To obtain a simple equation for i(V), the following procedure is used. A reference point "r" is selected within this part of the emitter surface (often the point at which the current density is highest), and the current density at this reference point is denoted by Jr. A parameter Ar, called the notional emission area (with respect to point "r"), is then defined by:
where the integral is taken across the part of the emitter of interest.
This parameter Ar was introduced into CFE theory by Stern, Gossling and Fowler in 1929 (who called it a "weighted mean area"). For practical emitters, the emission current density used in Fowler-Nordheim-type equations is always the current density at some reference point (though this is usually not stated). Long-established convention denotes this reference current density by the simple symbol J, and the corresponding local field and conversion factor by the simple symbols F and β, without the subscript "r" used above; in what follows, this convention is used.
The notional emission area Ar will often be a function of the reference local field (and hence voltage), and in some circumstances might be a significant function of temperature.
Because Ar has a mathematical definition, it does not necessarily correspond to the area from which emission is observed to occur from a single-point emitter in a field electron (emission) microscope. With a large-area emitter, which contains many individual emission sites, Ar will nearly always be very very much less than the "macroscopic" geometrical area (AM) of the emitter as observed visually (see below).
Incorporating these auxiliary equations into eq. (30a) yields
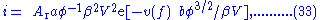
This is the simplified standard Fowler-Nordheim-type equation, in i-V form. The corresponding "physically complete" equation is obtained by multiplying by λZPF.
For such emitters, the notional emission area will nearly always be very very much less than the apparent "macroscopic" geometrical area (AM) of the physical emitter as observed visually. A dimensionless parameter αr, the area efficiency of emission, can be defined by
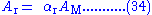
Also, a "macroscopic" (or "mean") emission current density JM (averaged over the geometrical area AM of the emitter) can be defined, and related to the reference current density Jr used above, by
This leads to the following "large-area versions" of the simplified standard Fowler-Nordheim-type equation:
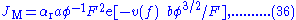
Both these equations contain the area efficiency of emission αr. For any given emitter this parameter has a value that is usually not well known. In general, αr varies greatly as between different emitter materials, and as between different specimens of the same material prepared and processed in different ways. Values in the range 10−10 to 10−6 appear to be likely, and values outside this range may be possible.
The presence of αr in eq. (36) accounts for the difference between the macroscopic current densities often cited in the literature (typically 10 A/m2 for many forms of large-area emitter other than Spindt arrays) and the local current densities at the actual emission sites, which can vary widely but which are thought to be generally of the order of 109 A/m2, or possibly slightly less.
A significant part of the technological literature on large-area emitters fails to make clear distinctions between local and macroscopic current densities, or between notional emission area Ar and macroscopic area AM, and/or omits the parameter αr from cited equations. Care is necessary in order to avoid errors of interpretation.
It is also sometimes convenient to split the conversion factor βr into a "macroscopic part" that relates to the overall geometry of the emitter and its surroundings, and a "local part" that relates to the ability of the very-local structure of the emitter surface to enhance the electric field. This is usually done by defining a "macroscopic field" FM that is the field that would be present at the emitting site in the absence of the local structure that causes enhancement. This field FM is related to the applied voltage by a "voltage-to-macroscopic-field conversion factor" βM defined by:
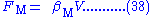
In the common case of a system comprising two parallel plates, separated by a distance W, with emitting nanostructures created on one of them, βM = 1/W.
A "field enhancement factor" γ is then defined and related to the values of βr and βM by
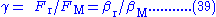
With eq. (31), this generates the following formulae:
where, in accordance with the usual convention, the suffix "r" has now been dropped from parameters relating to the reference point. Formulae exist for the estimation of γ, using classical electrostatics
, for a variety of emitter shapes, in particular the "hemisphere on a post".
Equation (40) implies that versions of Fowler-Nordheim-type equations can be written where either F or βV is everywhere replaced by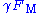
. This is often done in technological applications where the primary interest is in the field enhancing properties of the local emitter nanostructure. However in some past work, failure to make a clear distinction between barrier field F and macroscopic field FM has caused confusion or error.
More generally, the aims in technological development of large-area field emitters are to enhance the uniformity of emission by increasing the value of the area efficiency of emission αr, and to reduce the "onset" voltage at which significant emission occurs, by increasing the value of β. Eq. (41) shows that this can be done in two ways: either by trying to develop "high-γ" nanostructures, or by changing the overall geometry of the system so that βM is increased. Various trade-offs and constraints exist.
In practice, although the definition of macroscopic field used above is the commonest one, other (differently defined) types of macroscopic field and field enhancement factor are used in the literature, particularly in connection with the use of probes to investigate the i-V characteristics of individual emitters.
In technological contexts field emission data are often plotted using (a particular definition of) FM or 1/FM as the x-coordinate. However, for scientific analysis it usually better not to pre-manipulate the experimental data, but to plot the raw measured i-V data directly. Values of technological parameters such as (the various forms of) γ can then be obtained from the fitted parameters of the i-V data plot (see below), using the relevant definitions.
In the 1920s, empirical equations were used to find the power of V that appeared in the exponent of a semi-logarithmic equation assumed to describe experimental CFE results. In 1928, theory and experiment were brought together to show that (except, possibly, for very sharp emitters) this power is V−1. It has recently been suggested that CFE experiments should now be carried out to try to find the power (κ) of V in the pre-exponential of the following empirical CFE equation:
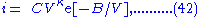
where B, C and κ are treated as constants.
From eq. (42) it is readily shown that
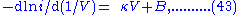
In the 1920s, experimental techniques could not distinguish between the results κ =0 (assumed by Millikan and Laurtisen) and κ=2 (predicted by the original Fowler-Nordheim-type equation). However, it should now be possible to make reasonably accurate measurements of dlni/d(1/V) (if necessary by using lock-in amplifier
/phase-sensitive detection techniques and computer-controlled equipment), and to derive κ from the slope of an appropriate data plot.
Following the discovery of approximation (30b), it is now very clear that – even for CFE from bulk metals – the value κ=2 is not expected. This can be shown as follows. Using eq. (30c) above, a dimensionless parameter η may be defined by
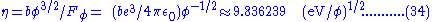
For φ = 4.50 eV, this parameter has the value η = 4.64. Since f = F/Fφ and v(f) is given by eq (30b), the exponent in the simplified standard Fowler-Nordheim-type equation (30) can be written in an alternative form and then expanded as follows:
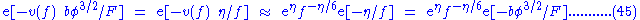
Provided that the conversion factor β is independent of voltage, the parameter f has the alternative definition f = V/Vφ, where Vφ is the voltage needed, in a particular experimental system, to reduce the height of a Schottky-Nordheim barrier from φ to zero. Thus, it is clear that the factor v(f) in the exponent of the theoretical equation (30) gives rise to additional V-dependence in the pre-exponential of the empirical equation. Thus, (for effects due to the Schottky-Nordheim barrier, and for an emitter with φ=4.5 eV) we obtain the prediction:
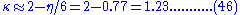
Since there may also be voltage dependence in other factors in a Fowler-Nordheim-type equation, in particular in the notional emission area Ar and in the local work-function, it is not necessarily expected that κ for CFE from a metal of local work-function 4.5 eV should have the value κ = 1.23, but there is certainly no reason to expect that it will have the original Fowler-Nordheim value κ = 2.
A first experimental test of this proposal has been carried out by Kirk, who used a slightly more complex form of data analysis to find a value 1.36 for his parameter κ. His parameter κ is very similar to, but not quite the same as, the parameter κ used here, but nevertheless his results do appear to confirm the potential usefulness of this form of analysis.
Use of the empirical CFE equation (42), and the measurement of κ, may be of particular use for non-metals. Strictly, Fowler-Nordheim-type equations apply only to emission from the conduction band
of bulk crystalline solids. However, empirical equations of form (42) should apply to all materials (though, conceivably, modification might be needed for very sharp emitters). It seems very likely that one way in which CFE equations for newer materials may differ from Fowler-Nordheim-type equations is that these CFE equations may have a different power of F (or V) in their pre-exponentials. Measurements of κ might provide some experimental indication of this.
Hence, knowledge of φ would allow β to be determined, or vice-versa.
[In principle, in system geometries where there is local field-enhancing nanostructure present, and the macroscopic conversion factor βM can be determined, knowledge of β then allows the value of the emitter's effective field enhancement factor γ to be determined from the formula γ = β/βM. In the common case of a film emitter generated on one plate of a two-plate arrangement with plate-separation W (so βM = 1/W) then
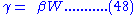
Nowadays, this is one of the most likely applications of Fowler-Nordheim plots.]
It subsequently became clear that the original thinking above is strictly correct only for the physically unrealistic situation of a flat emitter and an exact triangular barrier. For real emitters and real barriers a "slope correction factor" σFN has to be introduced, yielding the revised formula
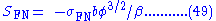
The value of σFN will, in principle, be influenced by any parameter in the physically complete Fowler-Nordheim-type equation for i(V) that has a voltage dependence.
At present, the only parameter that is considered important is the correction factor
relating to the barrier shape, and the only barrier for which there is any well-established detailed theory is the Schottky-Nordheim barrier. In this case, σFN is given by a mathematical function called s. This function s was first tabulated correctly (as a function of the Nordheim parameter y) by Burgess, Kroemer
and Houston in 1953; and a modern treatment that gives s as function of the scaled barrier field f for a Schottky-Nordheim barrier is given in. However, it has long been clear that, for practical emitter operation, the value of s lies in the range 0.9 to 1.
In practice, due to the extra complexity involved in taking the slope correction factor into detailed account, many authors (in effect) put σFN = 1 in eq. (49), thereby generating a systematic error in their estimated values of β and/or γ, thought usually to be around 5%.
However, empirical equation (42) – which in principle is more general than Fowler-Nordheim-type equations - brings with it possible new ways of analyzing field emission i-V data. In general, it may be assumed that the parameter B in the empirical equation is related to the unreduced height H of some characteristic barrier seen by tunneling electrons by
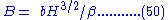
(In most cases, but not necessarily all, H would be equal to the local work-function; certainly this is true for metals.) The issue is how to determine the value of B by experiment. There are two obvious ways. (1) Suppose that eq. (43) can be used to determine a reasonably accurate experimental value of κ, from the slope of a plot of form [–dln{i}/d(1/V) vs. V]. In this case, a second plot, of ln(i)/Vκ vs. 1/V, should be an exact straight line of slope –B. This approach should be the most accurate way of determining B.
(2) Alternatively, if the value of κ is not exactly known, and cannot be accurately measured, but can be estimated or guessed, then a value for B can be derived from a plot of the form [ln{i} vs. 1/V]. This is the form of plot used by Millikan and Lauritsen in 1928. Re-arranging eq. (43) gives
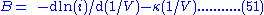
Thus, B can be determined, to a good degree of approximation, by determining the mean slope of a Millikan-Lauritsen plot over some range of values of 1/V, and by applying a correction, using the value of 1/V at the mid-point of the range and an assumed value of κ.
The main advantages of using a Millikan-Lauritsen plot, and this form of correction procedure, rather than a Fowler-Nordheim plot and a slope correction factor, are seen to be the following. (1) The plotting procedure is marginally more straightforward. (2) The correction involves a physical parameter (V) that is a measured quantity, rather than a physical parameter (f) that has to be calculated [in order to then calculate a value of s(f) or, more generally σFN(f)]. (3) Both the parameter κ itself, and the correction procedure, are more transparent (and more readily understood) than the Fowler-Nordheim-plot equivalents. (4) This procedure takes into account all physical effects that influence the value of κ, whereas the Fowler-Nordheim-plot correction procedure (in the form in which it has been carried out for the last 50 years) takes into account only those effects associated with barrier shape – assuming, furthermore, that this shape is that of a Schottky-Nordheim barrier. (5) There is a cleaner separation of theoretical and technological concerns: theoreticians will be interested in establishing what information any measured values of κ provide about CFE theory; but experimentalists can simply use measured values of κ to make more accurate estimates (if needed) of field enhancement factors.
This correction procedure for Millikan-Lauritsen plots will become easier to apply when a sufficient number of measurements of κ have been made, and a better idea is available of what typical values actually are. At present, it seems probable that for most materials κ will lie in the range -1<κ<3.
, with its particular assumptions about the distribution of internal electron states in energy, applies adequately to many metal
s as a first approximation. (2) Most of the time, metals have no surface states
and (in many cases) metal wave-functions
have no significant "surface resonances
". (3) Metals have a high density of states
at the Fermi level, so the charge that generates/screens external electric fields lies mainly on the outside of the top atomic layer, and no meaningful "field penetration" occurs. (4) Metals have high electrical conductivity: no significant voltage drops occur inside metal
emitters: this means that there are no factors obstructing the supply of electrons to the emitting surface, and that the electrons in this region can be both in effective local thermodynamic equilibrium
and in effective thermodynamic equilibrium with the electrons in the metal support structure on which the emitter is mounted. (5) Atomic-level effects are disregarded.
The development of "simple" theories of field electron emission, and in particular the development of Fowler-Nordheim-type equations, relies on all five of the above factors being true. For materials other than metals (and for atomically sharp metal emitters) one or more of the above factors will be untrue. For example, crystalline semiconductors do not have a free-electron-like band-structure, do have surface states, are subject to field penetration and band bending
, and may exhibit both internal voltage drops and statistical decoupling of the surface-state electron distribution from the electron distribution in the surface region of the bulk band-structure (this decoupling is known as "the Modinos effect").
This, basically, is why this article is confined to the theory of CFE from bulk metals. The complications involved in presenting CFE theory for non-metals are too great to be dealt with satisfactorily via Wikipedia. In any case, the basic theory of CFE from bulk metals needs to be understood first.
In practice, the theory of the actual Fowler-Nordheim tunneling process is much the same for all materials (though details of barrier shape may vary, and modified theory has to be developed for initial states that are localized rather than are travelling-wave-like). However, notwithstanding such differences, one expects (for thermodynamic equilibrium
situations) that all CFE equations will have exponents that behave in a generally similar manner. This is why applying Fowler-Nordheim-type equations to materials outside the scope of the derivations given here often works. If interest is only in parameters (such as field enhancement factor) that relate to the slope of Fowler-Nordheim or Millikan-Lauritsen plots and to the exponent of the CFE equation, then Fowler-Nordheim-type theory will often give sensible estimates. However, attempts to derive meaningful current density values will usually or always fail.
Note that a straight line in a Fowler-Nordheim or Millikan-Lauritsen plot does not indicate that emission from the corresponding material obeys a Fowler-Nordheim-type equation: it indicates only that the emission mechanism for individual electrons is probably Fowler-Nordheim tunneling.
Different materials may have radically different distributions in energy of their internal electron states, so the process of integrating current-density contributions over the internal electron states may give rise to significantly different expressions for the current-density pre-exponentials, for different classes of material. In particular, the power of barrier field appearing in the pre-exponential may be different from the original Fowler-Nordheim value "2". Investigation of effects of this kind is an active research topic. Atomic-level "resonance" and "scattering
" effects, if they occur, will also modify the theory.
Where materials are subject to field penetration and band bending, a necessary preliminary is to have good theories of such effects (for each different class of material) before detailed theories of CFE can be developed. Where voltage-drop effects occur, then the theory of the emission current may, to a greater or lesser extent, become theory that involves internal transport effects, and may become very complex.
Field penetration and band bending (semiconductors)
Field emitted vacuum space-charge
Field emission at high temperatures, and photo-field emission
Field-induced explosive electron emission
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
s induced by an electrostatic field. The most common context is FE from a solid surface into vacuum. However, FE can take place from solid or liquid surfaces, into vacuum, air, a fluid, or any non-conducting or weakly-conducting dielectric. The field-induced promotion of electrons from the valence to conduction band of semiconductors (the Zener effect
Zener effect
The Zener effect is a type of electrical breakdown in a reverse biased P-N junction diode in which the electric field across the diode breaks some of the covalent bonds of the semiconductor atoms leading to a large number of free minority carriers, which suddenly increase the reverse current...
) can also be regarded as a form of FE. The terminology is historical because related phenomena of surface photoeffect, thermionic emission or Richardson-Dushman effect and "cold electronic emission", i.e. the emission of electrons in strong static (or quasi-static) electric fields, were discovered and studied independently from 1880s to 1930s. When field emission is used without qualifiers it typically means the "cold emission."
Field emission in pure metals occurs in high electric fields: the gradients are typically higher than 1 gigavolt per metre and strongly dependent upon the work function
Work function
In solid-state physics, the work function is the minimum energy needed to remove an electron from a solid to a point immediately outside the solid surface...
. Electron sources based on field emission have a number of applications, but it is most commonly an undesirable primary source of vacuum breakdown and electrical discharge
Electrical breakdown
The term electrical breakdown or electric breakdown has several similar but distinctly different meanings. For example, the term can apply to the failure of an electric circuit....
phenomena, which engineers work to prevent. Examples of applications for surface field emission include construction of bright electron sources for high-resolution electron microscope
Electron microscope
An electron microscope is a type of microscope that uses a beam of electrons to illuminate the specimen and produce a magnified image. Electron microscopes have a greater resolving power than a light-powered optical microscope, because electrons have wavelengths about 100,000 times shorter than...
s or to discharge spacecraft
Spacecraft
A spacecraft or spaceship is a craft or machine designed for spaceflight. Spacecraft are used for a variety of purposes, including communications, earth observation, meteorology, navigation, planetary exploration and transportation of humans and cargo....
from induced charges. Devices which eliminate induced charges are termed charge-neutralizers.
Field emission was explained by quantum tunneling of electrons in the late 1920s. This was one of the triumphs of the nascent quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
. The theory of field emission from bulk metals was proposed by Fowler
Ralph H. Fowler
Sir Ralph Howard Fowler OBE FRS was a British physicist and astronomer.-Education:Fowler was initially educated at home but then attended Evans' preparatory school at Horris Hill and Winchester College...
and Nordheim
Lothar Wolfgang Nordheim
Lothar Wolfgang Nordheim was a German-born Jewish theoretical physicist...
.
A family of approximate equations, "Fowler–Nordheim equations", is named after them. Strictly, Fowler-Nordheim equations apply only to field emission from bulk metals and (with suitable modification) to other bulk crystalline solids, but they are often used – as a rough approximation – to describe field emission from other materials.
In some respects, field electron emission is a paradigm example of what physicists mean by tunneling
Quantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
. Unfortunately, it is also a paradigm example of the intense mathematical difficulties that can arise. Simple solvable models of the tunneling barrier lead to equations (including the original 1928 Fowler-Nordheim-type equation) that get predictions of emission current density too low by a factor of 100 or more. If one inserts a more realistic barrier model into the simplest form of the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
, then an awkward mathematical problem arises over the resulting differential equation: it is known to be mathematically impossible in principle to solve this equation exactly in terms of the usual functions of mathematical physics, or in any simple way. To get even an approximate solution, it is necessary to use special approximate methods known in physics as "semi-classical" or "quasi-classical" methods. Worse, a mathematical error was made in the original application of these methods to field emission, and even the corrected theory that was put in place in the 1950s has been formally incomplete until very recently. A consequence of these (and other) difficulties has been a heritage of misunderstanding and disinformation that still persists in some current field emission research literature. This article tries to present a basic account of field emission "for the 21st century and beyond" that is free from these confusions.
Terminology and conventions
Field electron emission, field-induced electron emission, field emission and electron field emission are general names for this experimental phenomenon and its theory. The first name is used here.Fowler–Nordheim tunneling is the wave-mechanical tunneling of electrons through a rounded triangular barrier created at the surface of an electron conductor by applying a very high electric field. Individual electrons can escape by Fowler-Nordheim tunneling from many materials in various different circumstances.
Cold field electron emission (CFE) is the name given to a particular statistical emission regime, in which the electrons in the emitter are initially in internal thermodynamic equilibrium
Thermodynamic equilibrium
In thermodynamics, a thermodynamic system is said to be in thermodynamic equilibrium when it is in thermal equilibrium, mechanical equilibrium, radiative equilibrium, and chemical equilibrium. The word equilibrium means a state of balance...
, and in which most emitted electrons escape by Fowler-Nordheim tunneling from electron states close to the emitter Fermi level. [By contrast, in the Schottky emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
regime, most electrons escape over the top of a field-reduced barrier, from states well above the Fermi level.] Many solid and liquid materials can emit electrons in a CFE regime if an electric field of an appropriate size is applied.
Fowler–Nordheim-type equations are a family of approximate equations derived to describe CFE from the internal electron states in bulk metals. The different members of the family represent different degrees of approximation to reality. Approximate equations are necessary because, for physically realistic models of the tunneling barrier, it is mathematically impossible in principle to solve the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
exactly in any simple way. There is no theoretical reason to believe that Fowler-Nordheim-type equations validly describe field emission from materials other than bulk crystalline solids.
For metals, the CFE regime extends to well above room temperature. There are other electron emission regimes (such as "thermal electron emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
" and "Schottky emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
") that require significant external heating of the emitter. There are also emission regimes where the internal electrons are not in thermodynamic equilibrium and the emission current is, partly or completely, determined by the supply of electrons to the emitting region. A non-equilibrium emission process of this kind may be called field (electron) emission if most of the electrons escape by tunneling, but strictly it is not CFE, and is not accurately described by a Fowler-Nordheim-type equation.
Care is necessary because in some contexts (e.g. spacecraft engineering), the name "field emission" is applied to the field-induced emission of ions (field ion emission), rather than electrons, and because in some theoretical contexts "field emission" is used as a general name covering both field electron emission and field ion emission.
Historically, the phenomenon of field electron emission has been known by a variety of names, including "the aeona effect", "autoelectronic emission", "cold emission", "cold cathode emission", "field emission", "field electron emission" and "electron field emission".
Equations in this article are written using the International System of Quantities (ISQ). This is the modern (post-1970s) international system, based around the rationalized-meter-kilogram-second (rmks) system of equations, which is used to define SI units. Older field emission literature (and papers that directly copy equations from old literature) often write some equations using an older equation system that does not use the quantity ε0. In this article, all such equations have been converted to modern international form. For clarity, this should always be done.
Since work function is normally given in electronvolts (eV), and it is often convenient to measure fields in volts per nanometer (V/nm), values of most universal constants are given here in units involving the eV, V and nm. Increasingly, this is normal practice in field emission research. However, all equations here are ISQ-compatible equations and remain dimensionally consistent, as is required by the modern international system. To indicate their status, numerical values of universal constants are given to seven significant figures. Values are derived using the 2006 values of the fundamental constants.
Early history of field electron emission
Field electron emission has a long, complicated and messy history. This section covers the early history, up to the derivation of the original Fowler–Nordheim-type equation in 1928.In retrospect, it seems likely that the electrical discharges reported by Winkler in 1744 were started by CFE from his wire electrode. However, meaningful investigations had to wait until after J.J. Thomson's identification of the electron in 1897, and until after it was understood – from thermal emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
and photo-emission work – that electrons could be emitted from inside metals (rather than from surface-adsorbed gas molecules
Adsorption
Adsorption is the adhesion of atoms, ions, biomolecules or molecules of gas, liquid, or dissolved solids to a surface. This process creates a film of the adsorbate on the surface of the adsorbent. It differs from absorption, in which a fluid permeates or is dissolved by a liquid or solid...
), and that – in the absence of applied fields – electrons escaping from metals had to overcome a work function barrier.
It was suspected at least as early as 1913 that field-induced emission was a separate physical effect. However, only after vacuum and specimen cleaning techniques had significantly improved, did this become well established. Lilienfeld
Julius Edgar Lilienfeld
Julius Edgar Lilienfeld was an Austro-Hungarian physicist. He was born in Lemberg in Austria-Hungary , moved to the United States in the early 1920s, and became American citizen in 1934...
(who was primarily interested in electron sources for medical X-ray
X-ray
X-radiation is a form of electromagnetic radiation. X-rays have a wavelength in the range of 0.01 to 10 nanometers, corresponding to frequencies in the range 30 petahertz to 30 exahertz and energies in the range 120 eV to 120 keV. They are shorter in wavelength than UV rays and longer than gamma...
applications) published in 1922 the first clear account in English of the experimental phenomenology of the effect he had called "autoelectronic emission". He had worked on this topic, in Leipzig, since about 1910. Kleint describes this and other early work.
After 1922, experimental interest increased, particularly in the groups led by Millikan at the California Institute in Pasadena, and by Gossling at the General Electric Company in London. Attempts to understand autoelectronic emission included plotting experimental current-voltage (i - V) data in different ways, to look for a straight-line relationship. Current increased with voltage more rapidly than linearly, but plots of type (log(i) vs. V) were not straight. Schottky
Walter H. Schottky
Walter Hermann Schottky was a German physicist who played a major early role in developing the theory of electron and ion emission phenomena, invented the screen-grid vacuum tube in 1915 and the pentode in 1919 while working at Siemens, and later made many significant contributions in the areas of...
suggested in 1923 that the effect might be due to thermally-induced emission over a field-reduced barrier. If so, then plots of type (log(i) vs. V1/2) should be straight; but they were not. Nor is Schottky's explanation compatible with the experimental observation of only very weak temperature dependence in CFE – a point initially overlooked.
A breakthrough came when Lauritsen
(and Oppenheimer independently) found that plots of type (log(i) vs. 1/V) yielded good straight lines. This result, published by Millikan and Lauritsen in early 1928, was known to Fowler
Ralph H. Fowler
Sir Ralph Howard Fowler OBE FRS was a British physicist and astronomer.-Education:Fowler was initially educated at home but then attended Evans' preparatory school at Horris Hill and Winchester College...
and Nordheim
Lothar Wolfgang Nordheim
Lothar Wolfgang Nordheim was a German-born Jewish theoretical physicist...
.
Oppenheimer had predicted that the field-induced tunneling of electrons from atoms (the effect now called field ionization) would have this i(V) dependence, had found this dependence in the published experimental field emission results of Millikan and Eyring, and proposed that CFE was due to field-induced tunneling
Quantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
of electrons from atomic-like orbitals
Atomic orbital
An atomic orbital is a mathematical function that describes the wave-like behavior of either one electron or a pair of electrons in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus...
in surface metal atoms. An alternative Fowler-Nordheim theory explained both the Millikan-Lauritsen finding and the very weak dependence of current on temperature. Fowler-Nordheim theory predicted both to be consequences if CFE were due to field-induced tunneling from free-electron-type states
Free electron model
In solid-state physics, the free electron model is a simple model for the behaviour of valence electrons in a crystal structure of a metallic solid. It was developed principally by Arnold Sommerfeld who combined the classical Drude model with quantum mechanical Fermi-Dirac statistics and hence it...
in what we would now call a metal conduction band
Electronic band structure
In solid-state physics, the electronic band structure of a solid describes those ranges of energy an electron is "forbidden" or "allowed" to have. Band structure derives from the diffraction of the quantum mechanical electron waves in a periodic crystal lattice with a specific crystal system and...
, with the electron states occupied in accordance with Fermi–Dirac statistics.
In fact, Oppenheimer (although right in principle about the theory of field ionization) had mathematical details of his theory seriously incorrect. There was also a small numerical error in the final equation given by Fowler-Nordheim theory for CFE current density
Current density
Current density is a measure of the density of flow of a conserved charge. Usually the charge is the electric charge, in which case the associated current density is the electric current per unit area of cross section, but the term current density can also be applied to other conserved...
: this was corrected in the 1929 paper of .
Strictly, if the barrier field in Fowler-Nordheim 1928 theory is exactly proportional to the applied voltage, and if the emission area is independent of voltage, then the Fowler-Nordheim 1928 theory predicts that plots of the form (log(i/V2) vs. 1/V) should be exact straight lines. However, contemporary experimental techniques were not good enough to distinguish between the Fowler-Nordheim theoretical result and the Millikan-Lauritsen experimental result.
Thus, by 1928 basic physical understanding of the origin of CFE from bulk metals had been achieved, and the original Fowler-Nordheim-type equation had been derived.
The literature often presents Fowler-Nordheim work as a proof of the existence of electron tunneling
Quantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
, as predicted by wave-mechanics. Whilst this is correct, the validity of wave-mechanics was largely accepted by 1928. The more important role of the Fowler-Nordheim paper was that it was a convincing argument from experiment that Fermi–Dirac statistics applied to the behavior of electrons in metals, as suggested by Sommerfeld
Arnold Sommerfeld
Arnold Johannes Wilhelm Sommerfeld was a German theoretical physicist who pioneered developments in atomic and quantum physics, and also educated and groomed a large number of students for the new era of theoretical physics...
in 1927. The success of Fowler-Nordheim theory did much to support the correctness of Sommerfeld's ideas, and greatly helped to establish modern electron band theory
Electronic band structure
In solid-state physics, the electronic band structure of a solid describes those ranges of energy an electron is "forbidden" or "allowed" to have. Band structure derives from the diffraction of the quantum mechanical electron waves in a periodic crystal lattice with a specific crystal system and...
. In particular, the original Fowler-Nordheim-type equation was one of the first to incorporate the statistical-mechanical
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
consequences of the existence of electron spin into the theory of an experimental condensed-matter effect. The Fowler-Nordheim paper also established the physical basis for a unified treatment of field-induced and thermally-induced electron emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
. Prior to 1928 it had been hypothesized that two types of electrons, "thermions" and "conduction electrons", existed in metals, and that thermally emitted electron currents were due to the emission of thermions, but that field-emitted currents were due to the emission of conduction electrons. The Fowler-Nordheim 1928 work suggested that thermions did not need to exist as a separate class of internal electrons: electrons could come from a single band
Electronic band structure
In solid-state physics, the electronic band structure of a solid describes those ranges of energy an electron is "forbidden" or "allowed" to have. Band structure derives from the diffraction of the quantum mechanical electron waves in a periodic crystal lattice with a specific crystal system and...
occupied in accordance with Fermi–Dirac statistics, but would be emitted in statistically different ways under different conditions of temperature and applied field.
The ideas of Oppenheimer, Fowler
Ralph H. Fowler
Sir Ralph Howard Fowler OBE FRS was a British physicist and astronomer.-Education:Fowler was initially educated at home but then attended Evans' preparatory school at Horris Hill and Winchester College...
and Nordheim
Lothar Wolfgang Nordheim
Lothar Wolfgang Nordheim was a German-born Jewish theoretical physicist...
were also an important stimulus to the development, by Gurney
Ronald W. Gurney
Ronald Wilfrid Gurney was an physicist who worked in the early years of Quantum Mechanics together with Edward Condon on the theory of radioactive decay of nuclei. He also gave a theoretical description for the electrolytic evolution of hydrogen gas from an acidified solution.Gurney was a writer...
and Condon
Edward Condon
Edward Uhler Condon was a distinguished American nuclear physicist, a pioneer in quantum mechanics, and a participant in the development of radar and nuclear weapons during World War II.-Early life and career:...
, later in 1928, of the theory of the radioactive decay
Radioactive decay
Radioactive decay is the process by which an atomic nucleus of an unstable atom loses energy by emitting ionizing particles . The emission is spontaneous, in that the atom decays without any physical interaction with another particle from outside the atom...
of nuclei (by alpha particle
Alpha particle
Alpha particles consist of two protons and two neutrons bound together into a particle identical to a helium nucleus, which is classically produced in the process of alpha decay, but may be produced also in other ways and given the same name...
tunneling).
Field electron microscopy and related basics
As already indicated, the early experimental work on field electron emission (1910–1920) was driven by Lilienfeld'sJulius Edgar Lilienfeld
Julius Edgar Lilienfeld was an Austro-Hungarian physicist. He was born in Lemberg in Austria-Hungary , moved to the United States in the early 1920s, and became American citizen in 1934...
desire to develop miniaturized X-ray
X-ray
X-radiation is a form of electromagnetic radiation. X-rays have a wavelength in the range of 0.01 to 10 nanometers, corresponding to frequencies in the range 30 petahertz to 30 exahertz and energies in the range 120 eV to 120 keV. They are shorter in wavelength than UV rays and longer than gamma...
tubes for medical applications. However, it was too early for this technology to succeed.
After Fowler-Nordheim theoretical work in 1928, a major advance came with the development in 1937 by Erwin W. Mueller of the spherical-geometry field electron microscope (FEM) (also called the "field emission microscope"). In this instrument, the electron emitter is a sharply pointed wire, of apex radius r. This is placed, in a vacuum enclosure, opposite an image detector (originally a phosphor screen), at a distance R from it. The microscope screen shows a projection image of the distribution of current-density J across the emitter apex, with magnification approximately (R/r), typically 105 to 106. In FEM studies the apex radius is typically 100 nm to 1 μm. The tip of the pointed wire, when referred to as a physical object, has been called a "field emitter", a "tip", or (recently) a "Mueller emitter".
When the emitter surface is clean, this FEM image is characteristic of: (a) the material from which the emitter is made: (b) the orientation of the material relative to the needle/wire axis; and (c) to some extent, the shape of the emitter endform. In the FEM image, dark areas correspond to regions where the local work function φ is relatively high and/or the local barrier field F is relatively low, so J is relatively low; the light areas correspond to regions where φ is relatively low and/or F is relatively high, so J is relatively high. This is as predicted by the exponent of Fowler-Nordheim-type equations [see eq. (30) below].
The adsorption
Adsorption
Adsorption is the adhesion of atoms, ions, biomolecules or molecules of gas, liquid, or dissolved solids to a surface. This process creates a film of the adsorbate on the surface of the adsorbent. It differs from absorption, in which a fluid permeates or is dissolved by a liquid or solid...
of layers of gas atoms (such as oxygen) onto the emitter surface, or part of it, can create surface electric dipoles that change the local work function of this part of the surface. This affects the FEM image; also, the change of work-function can be measured using a Fowler-Nordheim plot (see below). Thus, the FEM became an early observational tool of surface science
Surface science
Surface science is the study of physical and chemical phenomena that occur at the interface of two phases, including solid–liquid interfaces, solid–gas interfaces, solid–vacuum interfaces, and liquid-gas interfaces. It includes the fields of surface chemistry and surface physics. Some related...
. For example, in the 1960s, FEM results contributed significantly to discussions on heterogeneous catalysis
Heterogeneous catalysis
In chemistry, heterogeneous catalysis refers to the form of catalysis where the phase of the catalyst differs from that of the reactants. Phase here refers not only to solid, liquid, vs gas, but also immiscible liquids, e.g. oil and water. The great majority of practical heterogeneous catalysts...
. FEM has also been used for studies of surface-atom diffusion
Surface diffusion
Surface diffusion is a general process involving the motion of adatoms, molecules, and atomic clusters at solid material surfaces. The process can generally be thought of in terms of particles jumping between adjacent adsorption sites on a surface, as in figure 1...
. However, FEM has now been almost completely superseded by newer surface-science techniques.
A consequence of FEM development, and subsequent experimentation, was that it became possible to identify (from FEM image inspection) when an emitter was "clean", and hence exhibiting its clean-surface work-function as established by other techniques. This was important in experiments designed to test the validity of the standard Fowler-Nordheim-type equation.
These experiments deduced a value of voltage-to-barrier-field conversion factor β from a Fowler-Nordheim plot (see below), assuming the clean-surface φ–value for tungsten, and compared this with values derived from electron-microscope observations of emitter shape and electrostatic modeling. Agreement to within about 10% was achieved. Only very recently has it been possible to do the comparison the other way round, by bringing a well-prepared probe so close to a well-prepared surface that approximate parallel-plate geometry can be assumed and the conversion factor can be taken as 1/W, where W is the measured probe-to emitter separation. Analysis of the resulting Fowler-Nordheim plot yields a work-function value close to the independently known work-function of the emitter.
Field electron spectroscopy (electron energy analysis)
Energy distribution measurements of field-emitted electrons were first reported in 1939. In 1959 it was realized theoretically by Young, and confirmed experimentally by Young and Mueller that the quantity measured in spherical geometry was the distribution of the total energy of the emitted electron (its "total energy distribution"). This is because, in spherical geometry, the electrons move in such a fashion that angular momentumAngular momentum
In physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
about a point in the emitter is very nearly conserved. Hence any kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
that, at emission, is in a direction parallel to the emitter surface gets converted into energy associated with the radial direction of motion. So what gets measured in an energy analyzer is the total energy at emission.
With the development of sensitive electron energy analyzers in the 1960s, it became possible to measure fine details of the total energy distribution. These reflect fine details of the surface physics, and the technique of Field Electron Spectroscopy flourished for a while, before being superseded by newer surface-science techniques.
Field electron emitters as electron-gun sources
To achieve high-resolution in electron microscopes and other electron beam instruments (such as those used for electron beam lithographyElectron beam lithography
Electron beam lithography is the practice of emitting a beam of electrons in a patterned fashion across a surface covered with a film , and of selectively removing either exposed or non-exposed regions of the resist...
), it is helpful to start with an electron source that is small, optically bright and stable. Sources based on the geometry of a Mueller emitter qualify well on the first two criteria. The first electron microscope
Electron microscope
An electron microscope is a type of microscope that uses a beam of electrons to illuminate the specimen and produce a magnified image. Electron microscopes have a greater resolving power than a light-powered optical microscope, because electrons have wavelengths about 100,000 times shorter than...
(EM) observation of an individual atom was made by Crewe, Wall and Langmore in 1970, using a scanning electron microscope
Scanning electron microscope
A scanning electron microscope is a type of electron microscope that images a sample by scanning it with a high-energy beam of electrons in a raster scan pattern...
equipped with an early field emission gun.
From the 1950s onwards, extensive effort has been devoted to the development of field emission sources for use in electron gun
Electron gun
An electron gun is an electrical component that produces an electron beam that has a precise kinetic energy and is most often used in television sets and computer displays which use cathode ray tube technology, as well as in other instruments, such as electron microscopes and particle...
s. [e.g., DD53] Methods have been developed for generating on-axis beams, either by field-induced emitter build-up, or by selective deposition of a low-work-function adsorbate
Adsorption
Adsorption is the adhesion of atoms, ions, biomolecules or molecules of gas, liquid, or dissolved solids to a surface. This process creates a film of the adsorbate on the surface of the adsorbent. It differs from absorption, in which a fluid permeates or is dissolved by a liquid or solid...
(usually Zirconium oxide - ZrO) into the flat apex of a (100) oriented
Miller index
Miller indices form a notation system in crystallography for planes and directions in crystal lattices.In particular, a family of lattice planes is determined by three integers h, k, and ℓ, the Miller indices. They are written , and each index denotes a plane orthogonal to a direction in the...
Tungsten
Tungsten
Tungsten , also known as wolfram , is a chemical element with the chemical symbol W and atomic number 74.A hard, rare metal under standard conditions when uncombined, tungsten is found naturally on Earth only in chemical compounds. It was identified as a new element in 1781, and first isolated as...
emitter.
Sources that operate at room temperature have the disadvantage that they rapidly become covered with adsorbate molecule
Molecule
A molecule is an electrically neutral group of at least two atoms held together by covalent chemical bonds. Molecules are distinguished from ions by their electrical charge...
s that arrive from the vacuum
Vacuum
In everyday usage, vacuum is a volume of space that is essentially empty of matter, such that its gaseous pressure is much less than atmospheric pressure. The word comes from the Latin term for "empty". A perfect vacuum would be one with no particles in it at all, which is impossible to achieve in...
system walls, and the emitter has to be cleaned from time to time by "flashing" to high temperature. Nowadays, it is more common to use Mueller-emitter-based sources that are operated at elevated temperatures, either in the Schottky emission
Thermionic emission
Thermionic emission is the heat-induced flow of charge carriers from a surface or over a potential-energy barrier. This occurs because the thermal energy given to the carrier overcomes the binding potential, also known as work function of the metal. The charge carriers can be electrons or ions, and...
regime or in the so-called temperature-field intermediate regime. Many modern high-resolution electron microscopes and electron beam instruments use some form of Mueller-emitter-based electron source. Currently, attempts are being made to develop carbon nanotubes (CNTs) as electron-gun field emission sources.
The use of field emission sources in electron optical instruments has involved the development of appropriate theories of charged particle optics, and the development of related modeling. Various shape models have been tried for Mueller emitters; the best seems to be the "Sphere on Orthogonal Cone" (SOC) model introduced by Dyke, Trolan. Dolan and Barnes in 1953. Important simulations, involving trajectory tracing using the SOC emitter model, were made by Wiesener and Everhart. Nowadays, the facility to simulate field emission from Mueller emitters is often incorporated into the commercial electron-optics programmes used to design electron beam instruments. The design of efficient modern field-emission electron guns requires highly specialized expertise.
Atomically sharp emitters
Nowadays it is possible to prepare very sharp emitters, including emitters that end in a single atom. In this case, electron emission comes from an area about twice the crystallographic size of a single atom. This was demonstrated by comparing FEM and field ion microscopeField ion microscope
Field ion microscopy is an analytical technique used in materials science. The field ion microscope is a type of microscope that can be used to image the arrangement of atoms at the surface of a sharp metal tip....
(FIM) images of the emitter. Single-atom-apex Mueller emitters also have relevance to the scanning probe microscopy
Scanning probe microscopy
Scanning Probe Microscopy is a branch of microscopy that forms images of surfaces using a physical probe that scans the specimen. An image of the surface is obtained by mechanically moving the probe in a raster scan of the specimen, line by line, and recording the probe-surface interaction as a...
and helium scanning ion microscopy
Scanning Helium Ion Microscope
A Scanning Helium Ion Microscope is a new imaging technology based on a scanning helium ion beam. This technology has several advantages over the traditional scanning electron microscope...
(He SIM). Techniques for preparing them have been under investigation for many years. A related important recent advance has been the development (for use in the He SIM) of an automated technique for restoring a three-atom ("trimer") apex to its original state, if the trimer breaks up.
Materials aspects
Large-area field emission sources have been of interest since the 1970s. In these devices, a high density of individual field emission sites is created on a substrate (originally silicon). This research area became known, first as "vacuum microelectronics", now as "vacuum nanoelectronics".One of the original two device types, the "Spindt array", used silicon-integrated-circuit (IC)
Integrated circuit
An integrated circuit or monolithic integrated circuit is an electronic circuit manufactured by the patterned diffusion of trace elements into the surface of a thin substrate of semiconductor material...
fabrication techniques to make regular arrays in which molybdenum
Molybdenum
Molybdenum , is a Group 6 chemical element with the symbol Mo and atomic number 42. The name is from Neo-Latin Molybdaenum, from Ancient Greek , meaning lead, itself proposed as a loanword from Anatolian Luvian and Lydian languages, since its ores were confused with lead ores...
cones were deposited in small cylindrical voids in an oxide film, with the void covered by a counterelectrode with a central circular aperture. This overall geometry has also been used with carbon nanotubes grown in the void.
The other original device type was the "Latham emitter". These were MIMIV (metal-insulator-metal-insulator-vacuum) – or, more generally, CDCDV (conductor-dielectric-conductor-dielectric-vacuum) – devices that contained conducting particulates in a dielectric film. The device field-emits because its microstructure/nanostructure has field-enhancing properties. This material had a potential production advantage, in that it could be deposited as an "ink", so IC fabrication techniques were not needed. However in practice, uniformly reliable devices proved difficult to fabricate.
Research advanced to look for other materials that could be deposited/grown as thin films with suitable field-enhancing properties. In a parallel-plate arrangement, the "macroscopic" field FM between the plates is given by FM = V/W, where W is the plate separation and V is the applied voltage. If a sharp object is created on one plate, then the local field F at its apex is greater than FM and can be related to FM by
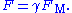
The parameter γ is called the "field enhancement factor" and is basically determined by the object's shape. Since field emission characteristics are determined by the local field F, then the higher the γ-value of the object, then the lower the value of FM at which significant emission occurs. Hence, for a given value of W, the lower the applied voltage V at which significant emission occurs.
For a roughly ten year-period from the mid-1990s, there was great interest in field emission from plasma-deposited films of amorphous and "diamond-like" carbon. However, interest subsequently lessened, partly due to the arrival of CNT
Carbon nanotube
Carbon nanotubes are allotropes of carbon with a cylindrical nanostructure. Nanotubes have been constructed with length-to-diameter ratio of up to 132,000,000:1, significantly larger than for any other material...
emitters, and partly because evidence emerged that the emission sites might be associated with particulate carbon objects created in an unknown way during the deposition process
Chemical vapor deposition
Chemical vapor deposition is a chemical process used to produce high-purity, high-performance solid materials. The process is often used in the semiconductor industry to produce thin films. In a typical CVD process, the wafer is exposed to one or more volatile precursors, which react and/or...
: this suggested that quality control
Quality control
Quality control, or QC for short, is a process by which entities review the quality of all factors involved in production. This approach places an emphasis on three aspects:...
of an industrial-scale production process might be problematic.
The introduction of CNT field emitters, both in "mat" form and in "grown array" forms, was a significant step forwards. Extensive research has been undertaken into both their physical characteristics and possible technological applications. For field emission, an advantage of CNTs is that, due to their shape, with its high aspect ratio
Aspect ratio
The aspect ratio of a shape is the ratio of its longer dimension to its shorter dimension. It may be applied to two characteristic dimensions of a three-dimensional shape, such as the ratio of the longest and shortest axis, or for symmetrical objects that are described by just two measurements,...
, they are "natural field-enhancing objects".
In recent years there has also been massive growth in interest in the development of other forms of thin-film emitter, both those based on other carbon forms (such as "carbon nanowalls") and on various forms of wide-band-gap semiconductor. A particular aim is to develop "high-γ" nanostructures with a sufficiently high density of individual emission sites.
Common problems with all field emission devices, particularly those that operate in "industrial vacuum conditions" is that the emission performance can be degraded by the adsorption of gas atoms arriving from elsewhere in the system, and the emitter shape can be in principle be modified deleteriously by a variety of unwanted subsidiary processes, such as bombardment by ions created by the impact of emitted electrons onto gas-phase atoms and/or onto the surface of counter-electrodes. Thus, an important industrial requirement is "robustness in poor vacuum conditions"; this needs to be taken into account in research on new emitter materials.
At the time of writing, the most promising forms of large-area field emission source (certainly in terms of achieved average emission current density) seem to be Spindt arrays and the various forms of source based on CNTs.
Applications
The development of large-area field emission sources was originally driven by the wish to create new, more efficient, forms of electronic information displayFlat panel display
Flat panel displays encompass a growing number of electronic visual display technologies. They are far lighter and thinner than traditional television sets and video displays that use cathode ray tubes , and are usually less than thick...
. These are known as "field emission display
Field emission display
A field emission display is a display technology that incorporates flat panel display technology that uses large-area field electron emission sources to provide electrons that strike colored phosphor to produce a color image as a electronic visual display...
s" or "nano-emissive displays". Although several prototypes have been demonstrated, the development of such displays into reliable commercial products has been hindered by a variety of industrial production problems not directly related to the source characteristics [En08].
Other proposed applications of large-area field emission sources include microwave
Microwave
Microwaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
generation, space-vehicle neutralization, X-ray generation, and (for array sources) multiple e-beam lithography
Electron beam lithography
Electron beam lithography is the practice of emitting a beam of electrons in a patterned fashion across a surface covered with a film , and of selectively removing either exposed or non-exposed regions of the resist...
. There are also recent attempts to develop large-area emitters on flexible substrates, in line with wider trends towards "plastic electronics".
The development of such applications is the mission of vacuum nanoelectronics. However, field emitters work best in conditions of good ultrahigh vacuum. Their most successful applications to date (FEM, FES and EM guns) have occurred in these conditions. The sad fact remains that field emitters and industrial vacuum conditions do not go well together, and the related problems of reliably ensuring good "vacuum robustness" of field emission sources used in such conditions still await better solutions (probably cleverer materials solutions) than we currently have.
Vacuum breakdown and electrical discharge phenomena
As already indicated, it is now thought that the earliest manifestations of field electron emission were the electrical discharges it caused. After Fowler-Nordheim work, it was understood that CFE was one of the possible primary underlying causes of vacuum breakdown and electrical discharge phenomena. (The detailed mechanisms and pathways involved can be very complicated, and there is no single universal cause) Where vacuum breakdown is known to be caused by electron emission from a cathode, then the original thinking was that the mechanism was CFE from small conducting needle-like surface protrusions. Procedures were (and are) used to round and smooth the surfaces of electrodes that might generate unwanted field electron emission currents. However the work of Latham and others showed that emission could also be associated with the presence of semiconducting inclusions in smooth surfaces. The physics of how the emission is generated is still not fully understood, but suspicion exists that so-called "triple-junction effects" may be involved. Further information may be found in Latham's book and in the on-line bibliography.Internal electron transfer in electronic devices
In some electronic devices, electron transfer from one material to another, or (in the case of sloping bands) from one band to another ("Zener tunnelingZener diode
A Zener diode is a special kind of diode which allows current to flow in the forward direction in the same manner as an ideal diode, but will also permit it to flow in the reverse direction when the voltage is above a certain value known as the breakdown voltage, "Zener knee voltage" or "Zener...
"), takes place by a field-induced tunneling process that can be regarded as a form of Fowler-Nordheim tunneling. For example, Rhoderick's
Emlyn Rhoderick
Emlyn Huw Rhoderick was a Welsh physicist and academic, who spent 33 years as professor of solid-state electronics at the Manchester College of Science and Technology .-Life:...
book discusses the theory relevant to metal-semiconductor contacts
Metal-semiconductor junction
In solid-state physics, a metal–semiconductor junction is a type of junction in which a metal comes in close contact with a semiconductor material...
.
Introduction
The next part of this article deals with the basic theory of cold field electron emission from bulk metals. This is best treated in four main stages, involving theory associated with: (1) derivation of a formula for "escape probabilityTransmission coefficient
The transmission coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered...
", by considering electron tunneling
Quantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
through a rounded triangular barrier; (2) an integration over internal electron states to obtain the "total energy distribution"; (3) a second integration, to obtain the emission current density as a function of local barrier field and local work function; (4) conversion of this to a formula for current as a function of applied voltage. The modified equations needed for large-area emitters, and issues of experimental data analysis, are dealt with separately.
Fowler–Nordheim tunneling is the wave-mechanical tunneling
Quantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
of an electron through an exact or rounded triangular barrier. Two basic situations are recognized: (1) when the electron is initially in a localized state
Surface states
Surface states are electronic states found at the surface of materials. They are formed due to the sharp transition from solid material that ends with a surface and are found only at the atom layers closest to the surface. The termination of a material with a surface leads to a change of the...
; (2) when the electron is initially not strongly localized, and is best represented by a travelling wave. Emission from a bulk metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
conduction band
Conduction band
In the solid-state physics field of semiconductors and insulators, the conduction band is the range of electron energies, higher than that of the valence band, sufficient to free an electron from binding with its individual atom and allow it to move freely within the atomic lattice of the material...
is a situation of the second type, and discussion here relates to this case. It is also assumed that the barrier is one-dimensional (i.e., has no lateral structure), and has no fine-scale structure that causes "scattering
Scattering
Scattering is a general physical process where some forms of radiation, such as light, sound, or moving particles, are forced to deviate from a straight trajectory by one or more localized non-uniformities in the medium through which they pass. In conventional use, this also includes deviation of...
" or "resonance" effects. To keep this explanation of Fowler-Nordheim tunneling relatively simple, these assumptions are needed; but the atomic structure of matter is in effect being disregarded.
Motive energy
For an electron, the one-dimensional Schrödinger equationSchrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
can be written in the form
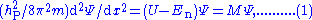
where Ψ(x) is the electron wave-function, expressed as a function of distance x measured from the emitter's electrical surface, hP is Planck's constant, m is the electron mass, U(x) is the electron potential energy
Potential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
, En is the total electron energy associated with motion in the x-direction, and M(x) [=U(x)–En] is called the electron motive energy. M(x) can be interpreted as the negative of the electron kinetic energy associated with the motion of a hypothetical classical point electron in the x-direction, and is positive in the barrier.
The shape of a tunneling barrier is determined by how M(x) varies with position, in the region where M(x)>0. Two models have special status in field emission theory. (1) The exact triangular (ET) barrier is described by
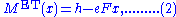
where h is the zero-field height (or unreduced height) of the barrier, e is the elementary positive charge
Elementary charge
The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called...
, and F is the barrier field. By convention, F is taken as positive, even though the classical electrostatic field
Electric field
In physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
would be negative. (2) The Schottky-Nordheim (SN) barrier is described by
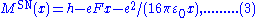
where ε0 is the electric constant (formerly called the "permittivity of free space"). This uses the classical image potential energy to represent the physical effect "correlation and exchange".
Escape probability
For an electron approaching a given barrier from the inside, the probability of escape (or "transmission coefficientTransmission coefficient
The transmission coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered...
" or "penetration coefficient") is a function of h and F, and is denoted by D(h,F). The primary aim of tunneling theory is to calculate D(h,F). For physically realistic barrier models, such as the Schottky-Nordheim barrier, the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
cannot be solved exactly in any simple way. The following so-called "semi-classical" approach can be used. A parameter G(h,F) can be defined by the JWKB (Jeffreys-Wentzel-Kramers-Brillouin)
WKB
WKB may mean:*WKB approximation, a method of finding approximate solutions of a differential equation in physics and mathematics*Well-known binary, a markup language in Geographical Information Systems for representing vector geometry objects on a map...
integral:
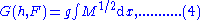
where the integral is taken across the barrier (i.e., across the region where M>0), and the parameter g is a universal constant given by
Forbes has re-arranged a result proved by Fröman and Fröman, to show that – formally – in a one-dimensional treatment – the exact solution for D can be written
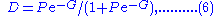
where the tunneling pre-factor P can in principle be evaluated by complicated iterative integrations along a path in complex space
Complex space
In mathematics, n-dimensional complex space is a multi-dimensional generalisation of the complex numbers, which have both real and imaginary parts or dimensions...
. In the CFE regime we have (by definition) G>>1. Also, for simple models P~1. So eq. (6) reduces to the so-called simple JWKB
WKB
WKB may mean:*WKB approximation, a method of finding approximate solutions of a differential equation in physics and mathematics*Well-known binary, a markup language in Geographical Information Systems for representing vector geometry objects on a map...
formula:
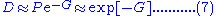
For the exact triangular barrier, putting eq. (2) into eq. (4) yields
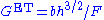, where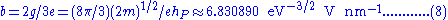This parameter b is a universal constant sometimes called the Second Fowler–Nordheim Constant. For barriers of other shapes, we write:
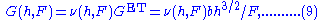
where
("nu") is a correction factor that in general has to be determined by numerical integrationNumerical integration
In numerical analysis, numerical integration constitutes a broad family of algorithms for calculating the numerical value of a definite integral, and by extension, the term is also sometimes used to describe the numerical solution of differential equations. This article focuses on calculation of...
, using eq. (4).
Correction factor for the Schottky-Nordheim barrier
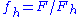The Schottky-Nordheim barrier, which is the barrier model used in deriving the standard Fowler-Nordheim-type equation, is a special case. In this case, it is known that the correction factor is a function of a single variable fh, defined by , where 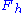is the field necessary to reduce the height of a Schottky-Nordheim barrier from h to 0. This field is given by: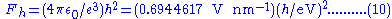.The parameter fh runs from 0 to 1, and may be called the scaled barrier field, for a Schottky-Nordheim barrier of zero-field height h.
For the Schottky-Nordheim barrier,
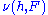is given by the particular value v(fh) of a function v(). The latter is a function of mathematical physics in its own right and has been called the Principal Schottky-Nordheim Barrier Function. An explicit series expansion for v() is derived in. The following good simple approximation for v(fh) has been found: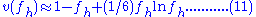Decay width
The decay width (in energy), dh, measures how fast the escape probabilityTransmission coefficient
The transmission coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered...
D decreases as the barrier height h increases; dh is defined by:
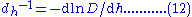
When h increases by dh then the escape probability D decreases by a factor close to e [≈2.718282], where e is the base of natural logarithms. For an elementary model, based on the exact triangular barrier, where we put
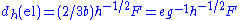and P ≈ 1, we get . The decay width dh derived from the more general expression (12) differs from this by a "decay-width correction factor" λd, so:Usually, the correction factor can be approximated as unity.
The decay-width
for a barrier with h equal to the local work-function φ is of special interest. Numerically this is given by: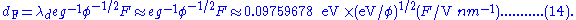For metals, the value of
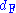is typically of order 0.2 eV, but varies with barrier-field F.Comments
A historical note is necessary. The idea that the Schottky-Nordheim barrier needed a correction factor, as in eq. (9), was introduced by Nordheim in 1928, but his mathematical analysis of the factor was incorrect. A new (correct) function was introduced by Burgess, KroemerHerbert Kroemer
Herbert Kroemer , a professor of electrical and computer engineering at the University of California, Santa Barbara, received his Ph.D. in theoretical physics in 1952 from the University of Göttingen, Germany, with a dissertation on hot electron effects in the then-new transistor, setting the stage...
and Houston in 1953, and its mathematics was developed further by Murphy and Good in 1956. This corrected function, sometimes known as a "special field emission elliptic function", was expressed as a function of a mathematical variable y known as the "Nordheim parameter". Only recently (2006 to 2008) has it been realized that, mathematically, it is much better to use the variable
[]. And only recently has it been possible to complete the definition of v() by developing and proving the validity of an exact series expansion for this function (by starting from known special-case solutions of the Gauss hypergeometric differential equationHypergeometric differential equation
In mathematics, the Gaussian or ordinary hypergeometric function 2F1 is a special function represented by the hypergeometric series, that includes many other special functions as specific or limiting cases. It is a solution of a second-order linear ordinary differential equation...
). Also, approximation (11) has been found only recently. Approximation (11) outperforms, and will presumably eventually displace, all older approximations of equivalent complexity. These recent developments, and their implications, will probably have a significant impact on field emission research in due course.
The following summary brings these results together. For tunneling well below the top of a well-behaved barrier of reasonable height, the escape probability D(h,F) is given formally by:
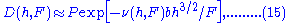
where
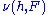is a correction factor that in general has to be found by numerical integration. For the special case of a Schottky-Nordheim barrier, an analytical result exists and 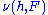is given by v(fh), as discussed above; approximation (11) for v(fh) is more than sufficient for all technological purposes. The pre-factor P is also in principle a function of h and (maybe) F, but for the simple physical models discussed here it is usually satisfactory to make the approximation P=1. The exact triangular barrier is a special case where the Schrödinger equationSchrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
can be solved exactly, as was done by Fowler and Nordheim; for this physically unrealistic case,
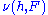=1, and an analytical approximation for P exists.The approach described here was originally developed to describe Fowler-Nordheim tunneling from smooth, classically flat, planar emitting surfaces. It is adequate for smooth, classical curved surfaces of radii down to about 10 to 20 nm. It can be adapted to surfaces of sharper radius, but quantities such as
and D then become significant functions of the parameter(s) used to describe the surface curvature. When the emitter is so sharp that atomic-level detail cannot be neglected, and/or the tunneling barrier is thicker than the emitter-apex dimensions, then a more sophisticated approach is desirable.As noted at the beginning, the effects of the atomic structure of materials are disregarded in the relatively simple treatments of field electron emission discussed here. Taking atomic structure properly into account is a very difficult problem, and only limited progress has been made. However, it seems probable that the main influences on the theory of Fowler-Nordheim tunneling will (in effect) be to change the values of P and
in eq. (15), by amounts that cannot easily be estimated at present.All these remarks apply in principle to Fowler-Nordheim tunneling from any conductor where (before tunneling) the electrons may be treated as in travelling-wave states. The approach may be adapted to apply (approximately) to situations where the electrons are initially in localized states
Surface states
Surface states are electronic states found at the surface of materials. They are formed due to the sharp transition from solid material that ends with a surface and are found only at the atom layers closest to the surface. The termination of a material with a surface leads to a change of the...
at or very close inside the emitting surface, but this is beyond the scope of this article.
Total-energy distribution
The energy distribution of the emitted electrons is important both for scientific experiments that use the emitted electron energy distribution to probe aspects of the emitter surface physics and for the field emission sources used in electron beam instruments such as electron microscopes. In the latter case, the "width" (in energy) of the distribution influences how finely the beam can be focused.The theoretical explanation here follows the approach of Forbes. If ε denotes the total electron energy relative to the emitter Fermi level, and Kp denotes the kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
of the electron parallel to the emitter surface, then the electron's normal energy εn (sometimes called its "forwards energy") is defined by
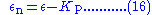.Two types of theoretical energy distribution are recognized: the normal-energy distribution (NED), which shows how the energy εn is distributed immediately after emission (i.e., immediately outside the tunneling barrier); and the total-energy distribution, which shows how the total energy ε is distributed. When the emitter Fermi level is used as the reference zero level, both ε and εn can be either positive or negative.
Energy analysis experiments have been made on field emitters since the 1930s. However, only in the late 1950s was it realized (by Young and Mueller[,YM58]) that these experiments always measured the total energy distribution, which is now usually denoted by j(ε). This is also true (or nearly true) when the emission comes from a small field enhancing protrusion on an otherwise flat surface.
To see how the total energy distribution can be calculated within the framework of a Sommerfeld free-electron-type model
Free electron model
In solid-state physics, the free electron model is a simple model for the behaviour of valence electrons in a crystal structure of a metallic solid. It was developed principally by Arnold Sommerfeld who combined the classical Drude model with quantum mechanical Fermi-Dirac statistics and hence it...
, look at the P-T energy-space diagram (P-T="parallel-total").
This shows the "parallel kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
" Kp on the horizontal axis and the total energy ε on the vertical axis. An electron inside the bulk metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
usually has values of Kp and ε that lie within the lightly shaded area. It can be shown that each element dεdKp of this energy space makes a contribution
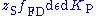to the electron current density incident on the inside of the emitter boundary. Here, zS is the universal constant (called here the SommerfeldArnold Sommerfeld
Arnold Johannes Wilhelm Sommerfeld was a German theoretical physicist who pioneered developments in atomic and quantum physics, and also educated and groomed a large number of students for the new era of theoretical physics...
supply density):
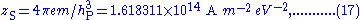
and
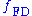is the Fermi-Dirac distribution functionFermi-Dirac statistics
Fermi–Dirac statistics is a part of the science of physics that describes the energies of single particles in a system comprising many identical particles that obey the Pauli Exclusion Principle...
:
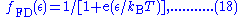
where T is thermodynamic temperature
Thermodynamic temperature
Thermodynamic temperature is the absolute measure of temperature and is one of the principal parameters of thermodynamics. Thermodynamic temperature is an "absolute" scale because it is the measure of the fundamental property underlying temperature: its null or zero point, absolute zero, is the...
and kB is Boltzmann's constant.
This element of incident current density sees a barrier of height h given by:
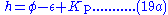
The corresponding escape probability
Transmission coefficient
The transmission coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered...
is D(h,F): this may be expanded (approximately) in the form
where DF is the escape probability for a barrier of unreduced height equal to the local work-function φ
Work function
In solid-state physics, the work function is the minimum energy needed to remove an electron from a solid to a point immediately outside the solid surface...
. Hence, the element dεdKp makes a contribution
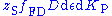to the emission current density, and the total contribution made by incident electrons with energies in the elementary range dε is thus,where the integral is in principle taken along the strip shown in the diagram, but can in practice be extended to ∞ when the decay-width dF is very much less than the Fermi energy
Fermi energy
The Fermi energy is a concept in quantum mechanics usually referring to the energy of the highest occupied quantum state in a system of fermions at absolute zero temperature....
KF (which is always the case for a metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
). The outcome of the integration can be written:
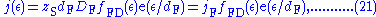
where
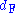and are values appropriate to a barrier of unreduced height h equal to the local work function φ, and 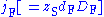is defined by this equation.For a given emitter, with a given field applied to it,
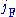is independent of F, so eq. (21) shows that the shape of the distribution (as ε increases from a negative value well below the Fermi level) is a rising exponential, multiplied by the FD distribution functionFermi-Dirac statistics
Fermi–Dirac statistics is a part of the science of physics that describes the energies of single particles in a system comprising many identical particles that obey the Pauli Exclusion Principle...
. This generates the familiar distribution shape first predicted by Young. At low temperatures,
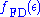goes sharply from 1 to 0 in the vicinity of the Fermi level, and the FWHMFull width at half maximum
Full width at half maximum is an expression of the extent of a function, given by the difference between the two extreme values of the independent variable at which the dependent variable is equal to half of its maximum value....
of the distribution is given by:
The fact that experimental CFE total energy distributions have this basic shape is a good experimental confirmation that electrons in metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
s obey Fermi-Dirac statistics
Fermi-Dirac statistics
Fermi–Dirac statistics is a part of the science of physics that describes the energies of single particles in a system comprising many identical particles that obey the Pauli Exclusion Principle...
.
Introduction
Fowler–Nordheim-type equations, in the J-F form, are (approximate) theoretical equations derived to describe the local current density J emitted from the internal electron states in the conduction bandConduction band
In the solid-state physics field of semiconductors and insulators, the conduction band is the range of electron energies, higher than that of the valence band, sufficient to free an electron from binding with its individual atom and allow it to move freely within the atomic lattice of the material...
of a bulk metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
. The emission current density (ECD) J for some small uniform region of an emitting surface is usually expressed as a function J(φ,F) of the local work-function φ
Work function
In solid-state physics, the work function is the minimum energy needed to remove an electron from a solid to a point immediately outside the solid surface...
and the local barrier field F that characterize the small region. For sharply curved surfaces, J may also depend on the parameter(s) used to describe the surface curvature.
Owing to the physical assumptions made in the original derivation, the term Fowler-Nordheim-type equation has long been used only for equations that describe the ECD at zero temperature. However, it is better to allow this name to include the slightly modified equations (discussed below) that are valid for finite temperatures within the CFE emission regime.
Zero-temperature form
Current density is best measured in A/m2. The total current density emitted from a small uniform region can be obtained by integrating the total energy distribution j(ε) with respect to total electron energy ε. At zero temperature, the Fermi-Dirac distribution functionFermi-Dirac statistics
Fermi–Dirac statistics is a part of the science of physics that describes the energies of single particles in a system comprising many identical particles that obey the Pauli Exclusion Principle...
fFD = 1 for ε<0, and fFD = 0 for ε>0. So the ECD at 0 K, J0, is given from eq. (18) by
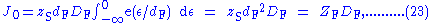
where
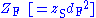is the effective supply for state F, and is defined by this equation. Strictly, the lower limit of the integral should be –KF, where KF is the Fermi EnergyFermi energy
The Fermi energy is a concept in quantum mechanics usually referring to the energy of the highest occupied quantum state in a system of fermions at absolute zero temperature....
; but if dF is very much less than KF (which is always the case for a metal) then no significant contribution to the integral comes from energies below KF, and it can formally be extended to –∞.
Result (23) can be given a simple and useful physical interpretation by referring to Fig. 1. The electron state at point "F" on the diagram ("state F") is the "forwards moving state at the Fermi level" (i.e., it describes a Fermi-level electron moving normal to and towards the emitter surface). At 0 K, an electron in this state sees a barrier of unreduced height φ, and has an escape probability DF that is higher than that for any other occupied electron state. So it is convenient to write J0 as ZFDF, where the "effective supply" ZF is the current density that would have to be carried by state F inside the metal if all of the emission came out of state F.
In practice, the current density mainly comes out of a group of states close in energy to state F, most of which lie within the heavily shaded area in the energy-space diagram. Since, for a free-electron model
Free electron model
In solid-state physics, the free electron model is a simple model for the behaviour of valence electrons in a crystal structure of a metallic solid. It was developed principally by Arnold Sommerfeld who combined the classical Drude model with quantum mechanical Fermi-Dirac statistics and hence it...
, the contribution to the current density is directly proportional to the area in energy space (with the Sommerfeld
Arnold Sommerfeld
Arnold Johannes Wilhelm Sommerfeld was a German theoretical physicist who pioneered developments in atomic and quantum physics, and also educated and groomed a large number of students for the new era of theoretical physics...
supply density zS as the constant of proportionality), it is useful to think of the ECD as drawn from electron states in an area of size dF2 (measured in eV2) in the energy-space diagram. That is, it is useful to think of the ECD as drawn from states in the heavily-shaded area in Fig. 1. (This approximation gets slowly worse as temperature increases.)
ZF can also be written in the form:
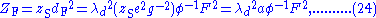
where the universal constant a, sometimes called the First Fowler–Nordheim Constant, is given by
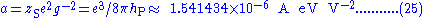
This shows clearly that the pre-exponential factor a φ−1F2, that appears in Fowler-Nordheim-type equations, relates to the effective supply of electrons to the emitter surface, in a free-electron model.
Non-zero temperatures
To obtain a result valid for non-zero temperature, we note from eq. (23) that zSdFDF = J0/dF. So when eq. (21) is integrated at non-zero temperature, then – on making this substitution, and inserting the explicit form of the Fermi-Dirac distribution functionFermi-Dirac statistics
Fermi–Dirac statistics is a part of the science of physics that describes the energies of single particles in a system comprising many identical particles that obey the Pauli Exclusion Principle...
– the ECD J can be written in the form:
where λT is a temperature correction factor given by the integral. The integral can be transformed, by writing
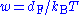and 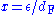, and then 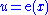, into the standard result::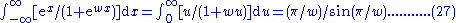This is valid for w>1 (i.e., dF/kBT > 1). Hence – for temperatures such that kBT<dF:
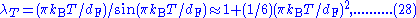
where the expansion is valid only if (πkBT /dF) << 1. An example value (for φ= 4.5 eV, F= 5 V/nm, T= 300 K) is λT= 1.024. Normal thinking has been that, in the CFE regime, λT is always small in comparison with other uncertainties, and that it is usually unnecessary to explicitly include it in formulae for the current density at room temperature.
The emission regimes for metals are, in practice, defined, by the ranges of barrier field F and temperature T for which a given family of emission equations is mathematically adequate. When the barrier field F is high enough for the CFE regime to be operating for metal emission at 0 K, then the condition kBT<dF provides a formal upper bound (in temperature) to the CFE emission regime. However, it has been argued that (due to approximations made elsewhere in the derivation) the condition kBT<0.7dF is a better working limit: this corresponds to a λT-value of around 1.09, and (for the example case) an upper temperature limit on the CFE regime of around 1770 K. This limit is a function of barrier field.
Note that result (28) here applies for a barrier of any shape (though dF will be different for different barriers).
Physically complete Fowler-Nordheim-type equation
Result (23) also leads to some understanding of what happens when atomic-level effects are taken into account, and the band-structure is no longer free-electron like. Due to the presence of the atomic ion-cores, the surface barrier, and also the electron wave-functions at the surface, will be different. This will affect the values of the correction factor, the prefactor P, and (to a limited extent) the correction factor λd. These changes will, in turn, affect the values of the parameter DF and (to a limited extent) the parameter dF. For a real metalMetal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
, the supply density will vary with position in energy space, and the value at point "F" may be different from the Sommerfeld supply density. We can take account of this effect by introducing an electronic-band-structure correction factor λB into eq. (23). Modinos has discussed how this factor might be calculated: he estimates that it is most likely to be between 0.1 and 1; it might lie outside these limits but is most unlikely to lie outside the range 0.01<λB<10.
By defining an overall supply correction factor λZ equal to λT λB λd2, and combining equations above, we reach the so-called physically complete Fowler-Nordheim-type equation:
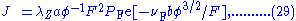
where
[=(φ,F)] is the exponent correction factor for a barrier of unreduced height φ. This is the most general equation of the Fowler–Nordheim type. Other equations in the family are obtained by substituting specific expressions for the three correction factors , PF and λZ it contains. The so-called elementary Fowler-Nordheim-type equation, that appears in undergraduate textbook discussions of field emission, is obtained by putting λZ→1, PF→1, →1; this does not yield good quantitative predictions because it makes the barrier stronger than it is in physical reality. The so-called standard Fowler-Nordheim-type equation, originally developed by Murphy and Good, and much used in past literature, is obtained by putting λZ→tF−2, PF→1, →vF, where vF is v(f), where f is the value of fh obtained by putting h=φ, and tF is a related parameter (of value close to unity).Within the more complete theory described here, the factor tF−2 is a component part of the correction factor λd2 [see, and note that λd2 is denoted by λD there]. There is no significant value in continuing the separate identification of tF−2. Probably, in the present state of knowledge, the best approximation for simple Fowler-Nordheim-type equation based modeling of CFE from metals is obtained by putting λZ→1, PF → 1,
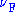→ v(f). This re-generates the Fowler-Nordheim-type equation used by Dyke and Dolan in 1956, and can be called the "simplified standard Fowler-Nordheim-type equation".Recommended form for simple Fowler-Nordheim-type calculations
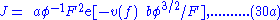
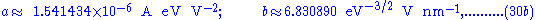
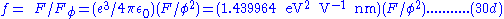
Explicitly, this recommended simplified standard Fowler-Nordheim-type equation, and associated formulae, are:where Fφ here is the field needed to reduce to zero a Schottky-Nordheim barrier of unreduced height equal to the local work-function φ
Work function
In solid-state physics, the work function is the minimum energy needed to remove an electron from a solid to a point immediately outside the solid surface...
, and f is the scaled barrier field for a Schottky-Nordheim barrier of unreduced height φ. [This quantity f could have been written more exactly as fφSN, but it makes this Fowler-Nordheim-type equation look less cluttered if the convention is adopted that simple f means the quantity denoted by fφSN in, eq. (2.16).] For the example case (φ= 4.5 eV, F= 5 V/nm), f≈ 0.36 and v(f) ≈ 0.58; practical ranges for these parameters are discussed further in.
Note that the variable f (the scaled barrier field) is not the same as the variable y (the Nordheim parameter) extensively used in past field emission literature, and that " v(f) " does NOT have the same mathematical meaning and values as the quantity " v(y) " that appears in field emission literature. In the context of the revised theory described here, formulae for v(y), and tables of values for v(y) should be disregarded, or treated as values of v(f1/2). If more exact values for v(f) are required, then provides formulae that give values for v(f) to an absolute mathematical accuracy of better than 8×10−10. However, approximation formula (30c) above, which yields values correct to within an absolute mathematical accuracy of better 0.0025, should gives values sufficiently accurate for all technological purposes.
Comments
A historical note on methods of deriving Fowler-Nordheim-type equations is necessary. There are several possible approaches to deriving these equations, using free-electron theoryFree electron model
In solid-state physics, the free electron model is a simple model for the behaviour of valence electrons in a crystal structure of a metallic solid. It was developed principally by Arnold Sommerfeld who combined the classical Drude model with quantum mechanical Fermi-Dirac statistics and hence it...
. The approach used here was introduced by Forbes in 2004 and may be described as "integrating via the total energy distribution, using the parallel kinetic energy Kp as the first variable of integration". Basically, it is a free-electron equivalent of the Modinos procedure (in a more advanced quantum-mechanical treatment) of "integrating over the surface Brillouin zone". By contrast, the free-electron treatments of CFE by Young in 1959, Gadzuk and Plummer in 1973 and Modinos in 1984, also integrate via the total energy distribution, but use the normal energy εn (or a related quantity) as the first variable of integration.
There is also an older approach, based on a seminal paper by Nordheim in 1928, that formulates the problem differently and then uses first Kp and then εn (or a related quantity) as the variables of integration: this is known as "integrating via the normal-energy distribution". This approach continues to be used by some authors. Although it has some advantages, particularly when discussing resonance phenomena, it requires integration of the Fermi-Dirac distribution function in the first stage of integration: for non-free-electron-like electronic band-structures this can lead to very complex and error-prone mathematics (as in the work of Stratton on semiconductors). Further, integrating via the normal-energy distribution does not generate experimentally measured electron energy distributions.
In general, the approach used here seems easier to understand, and leads to simpler mathematics.
It is also closer in principle to the more sophisticated approaches used when dealing with real bulk crystalline solids, where the first step is either to integrate contributions to the ECD over constant energy surfaces in a wave-vector
Wave vector
In physics, a wave vector is a vector which helps describe a wave. Like any vector, it has a magnitude and direction, both of which are important: Its magnitude is either the wavenumber or angular wavenumber of the wave , and its direction is ordinarily the direction of wave propagation In...
space ( k -space), or to integrate contributions over the relevant surface Brillouin zone. The Forbes approach is equivalent either to integrating over a spherical surface in k -space, using the variable Kp to define a ring-like integration element that has cylindrical symmetry about an axis in a direction normal to the emitting surface, or to integrating over an (extended) surface Brillouin zone using circular-ring elements.
CFE theoretical equations (i-V forms)
The preceding section explains how to derive Fowler-Nordheim-type equations. Strictly, these equations apply only to CFE from bulk metals. The ideas in the following sections apply to CFE more generally, but eq. (30) will be used to illustrate them.For CFE, basic theoretical treatments provide a relationship between the local emission current density J and the local barrier field F, at a local position on the emitting surface. Experiments measure the emission current i from some defined part of the emission surface, as a function of the voltage V applied to some counter-electrode. To relate these variables to J and F, auxiliary equations are used.
The voltage-to-barrier-field conversion factor β is defined by:
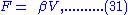
The value of F varies from position to position on an emitter surface, and the value of β varies correspondingly.
For a metal emitter, the β−value for a given position will be constant (independent of voltage) under the following conditions: (1) the apparatus is a "diode" arrangement, where the only electrodes present are the emitter and a set of "surroundings", all parts of which are at the same voltage; (2) no significant field-emitted vacuum space-charge
Space charge
Space charge is a concept in which excess electric charge is treated as a continuum of charge distributed over a region of space rather than distinct point-like charges...
(FEVSC) is present (this will be true except at very high emission current densities, around 109 A/m2 or higher ); (3) no significant "patch fields" exist, as a result of non-uniformities in local work-function
Work function
In solid-state physics, the work function is the minimum energy needed to remove an electron from a solid to a point immediately outside the solid surface...
(this is normally assumed to be true, but may not be in some circumstances). For non-metals, the physical effects called "field penetration" and "band bending
Band bending
Band bending refers to the local change in energy of electrons at a semiconductor junction due to space charge effects. Because the common way to visualize the electron energy states and Fermi level in a material is to draw bands on an energy vs...
" [M084] can make β a function of applied voltage, although – surprisingly – there are few studies of this effect.
The emission current density J varies from position to position across the emitter surface. The total emission current i from a defined part of the emitter is obtained by integrating J across this part. To obtain a simple equation for i(V), the following procedure is used. A reference point "r" is selected within this part of the emitter surface (often the point at which the current density is highest), and the current density at this reference point is denoted by Jr. A parameter Ar, called the notional emission area (with respect to point "r"), is then defined by:
where the integral is taken across the part of the emitter of interest.
This parameter Ar was introduced into CFE theory by Stern, Gossling and Fowler in 1929 (who called it a "weighted mean area"). For practical emitters, the emission current density used in Fowler-Nordheim-type equations is always the current density at some reference point (though this is usually not stated). Long-established convention denotes this reference current density by the simple symbol J, and the corresponding local field and conversion factor by the simple symbols F and β, without the subscript "r" used above; in what follows, this convention is used.
The notional emission area Ar will often be a function of the reference local field (and hence voltage), and in some circumstances might be a significant function of temperature.
Because Ar has a mathematical definition, it does not necessarily correspond to the area from which emission is observed to occur from a single-point emitter in a field electron (emission) microscope. With a large-area emitter, which contains many individual emission sites, Ar will nearly always be very very much less than the "macroscopic" geometrical area (AM) of the emitter as observed visually (see below).
Incorporating these auxiliary equations into eq. (30a) yields
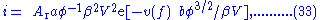
This is the simplified standard Fowler-Nordheim-type equation, in i-V form. The corresponding "physically complete" equation is obtained by multiplying by λZPF.
Modified equations for large-area emitters
The equations in the preceding section apply to all field emitters operating in the CFE regime. However, further developments are useful for large-area emitters that contain many individual emission sites.For such emitters, the notional emission area will nearly always be very very much less than the apparent "macroscopic" geometrical area (AM) of the physical emitter as observed visually. A dimensionless parameter αr, the area efficiency of emission, can be defined by
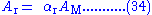
Also, a "macroscopic" (or "mean") emission current density JM (averaged over the geometrical area AM of the emitter) can be defined, and related to the reference current density Jr used above, by
This leads to the following "large-area versions" of the simplified standard Fowler-Nordheim-type equation:
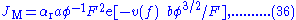
Both these equations contain the area efficiency of emission αr. For any given emitter this parameter has a value that is usually not well known. In general, αr varies greatly as between different emitter materials, and as between different specimens of the same material prepared and processed in different ways. Values in the range 10−10 to 10−6 appear to be likely, and values outside this range may be possible.
The presence of αr in eq. (36) accounts for the difference between the macroscopic current densities often cited in the literature (typically 10 A/m2 for many forms of large-area emitter other than Spindt arrays) and the local current densities at the actual emission sites, which can vary widely but which are thought to be generally of the order of 109 A/m2, or possibly slightly less.
A significant part of the technological literature on large-area emitters fails to make clear distinctions between local and macroscopic current densities, or between notional emission area Ar and macroscopic area AM, and/or omits the parameter αr from cited equations. Care is necessary in order to avoid errors of interpretation.
It is also sometimes convenient to split the conversion factor βr into a "macroscopic part" that relates to the overall geometry of the emitter and its surroundings, and a "local part" that relates to the ability of the very-local structure of the emitter surface to enhance the electric field. This is usually done by defining a "macroscopic field" FM that is the field that would be present at the emitting site in the absence of the local structure that causes enhancement. This field FM is related to the applied voltage by a "voltage-to-macroscopic-field conversion factor" βM defined by:
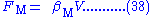
In the common case of a system comprising two parallel plates, separated by a distance W, with emitting nanostructures created on one of them, βM = 1/W.
A "field enhancement factor" γ is then defined and related to the values of βr and βM by
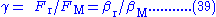
With eq. (31), this generates the following formulae:
where, in accordance with the usual convention, the suffix "r" has now been dropped from parameters relating to the reference point. Formulae exist for the estimation of γ, using classical electrostatics
Electrostatics
Electrostatics is the branch of physics that deals with the phenomena and properties of stationary or slow-moving electric charges....
, for a variety of emitter shapes, in particular the "hemisphere on a post".
Equation (40) implies that versions of Fowler-Nordheim-type equations can be written where either F or βV is everywhere replaced by
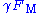. This is often done in technological applications where the primary interest is in the field enhancing properties of the local emitter nanostructure. However in some past work, failure to make a clear distinction between barrier field F and macroscopic field FM has caused confusion or error.More generally, the aims in technological development of large-area field emitters are to enhance the uniformity of emission by increasing the value of the area efficiency of emission αr, and to reduce the "onset" voltage at which significant emission occurs, by increasing the value of β. Eq. (41) shows that this can be done in two ways: either by trying to develop "high-γ" nanostructures, or by changing the overall geometry of the system so that βM is increased. Various trade-offs and constraints exist.
In practice, although the definition of macroscopic field used above is the commonest one, other (differently defined) types of macroscopic field and field enhancement factor are used in the literature, particularly in connection with the use of probes to investigate the i-V characteristics of individual emitters.
In technological contexts field emission data are often plotted using (a particular definition of) FM or 1/FM as the x-coordinate. However, for scientific analysis it usually better not to pre-manipulate the experimental data, but to plot the raw measured i-V data directly. Values of technological parameters such as (the various forms of) γ can then be obtained from the fitted parameters of the i-V data plot (see below), using the relevant definitions.
Empirical CFE i-V equation
At the present stage of CFE theory development, it is important to make a distinction between theoretical CFE equations and an empirical CFE equation. The former are derived from condensed matter physics (albeit in contexts where their detailed development is difficult). An empirical CFE equation, on the other hand, simply attempts to represent the actual experimental form of the dependence of current i on voltage V.In the 1920s, empirical equations were used to find the power of V that appeared in the exponent of a semi-logarithmic equation assumed to describe experimental CFE results. In 1928, theory and experiment were brought together to show that (except, possibly, for very sharp emitters) this power is V−1. It has recently been suggested that CFE experiments should now be carried out to try to find the power (κ) of V in the pre-exponential of the following empirical CFE equation:
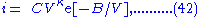
where B, C and κ are treated as constants.
From eq. (42) it is readily shown that
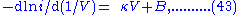
In the 1920s, experimental techniques could not distinguish between the results κ =0 (assumed by Millikan and Laurtisen) and κ=2 (predicted by the original Fowler-Nordheim-type equation). However, it should now be possible to make reasonably accurate measurements of dlni/d(1/V) (if necessary by using lock-in amplifier
Lock-in amplifier
A lock-in amplifier is a type of amplifier that can extract a signal with a known carrier wave from an extremely noisy environment . It is essentially a homodyne with an extremely low pass filter...
/phase-sensitive detection techniques and computer-controlled equipment), and to derive κ from the slope of an appropriate data plot.
Following the discovery of approximation (30b), it is now very clear that – even for CFE from bulk metals – the value κ=2 is not expected. This can be shown as follows. Using eq. (30c) above, a dimensionless parameter η may be defined by
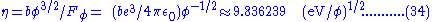
For φ = 4.50 eV, this parameter has the value η = 4.64. Since f = F/Fφ and v(f) is given by eq (30b), the exponent in the simplified standard Fowler-Nordheim-type equation (30) can be written in an alternative form and then expanded as follows:
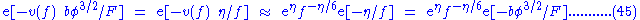
Provided that the conversion factor β is independent of voltage, the parameter f has the alternative definition f = V/Vφ, where Vφ is the voltage needed, in a particular experimental system, to reduce the height of a Schottky-Nordheim barrier from φ to zero. Thus, it is clear that the factor v(f) in the exponent of the theoretical equation (30) gives rise to additional V-dependence in the pre-exponential of the empirical equation. Thus, (for effects due to the Schottky-Nordheim barrier, and for an emitter with φ=4.5 eV) we obtain the prediction:
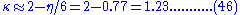
Since there may also be voltage dependence in other factors in a Fowler-Nordheim-type equation, in particular in the notional emission area Ar and in the local work-function, it is not necessarily expected that κ for CFE from a metal of local work-function 4.5 eV should have the value κ = 1.23, but there is certainly no reason to expect that it will have the original Fowler-Nordheim value κ = 2.
A first experimental test of this proposal has been carried out by Kirk, who used a slightly more complex form of data analysis to find a value 1.36 for his parameter κ. His parameter κ is very similar to, but not quite the same as, the parameter κ used here, but nevertheless his results do appear to confirm the potential usefulness of this form of analysis.
Use of the empirical CFE equation (42), and the measurement of κ, may be of particular use for non-metals. Strictly, Fowler-Nordheim-type equations apply only to emission from the conduction band
Conduction band
In the solid-state physics field of semiconductors and insulators, the conduction band is the range of electron energies, higher than that of the valence band, sufficient to free an electron from binding with its individual atom and allow it to move freely within the atomic lattice of the material...
of bulk crystalline solids. However, empirical equations of form (42) should apply to all materials (though, conceivably, modification might be needed for very sharp emitters). It seems very likely that one way in which CFE equations for newer materials may differ from Fowler-Nordheim-type equations is that these CFE equations may have a different power of F (or V) in their pre-exponentials. Measurements of κ might provide some experimental indication of this.
Fowler-Nordheim plots and Millikan-Lauritsen plots
The original theoretical equation derived by Fowler and Nordheim has, for the last 80 years, influenced the way that experimental CFE data has been plotted and analyzed. In the very widely used Fowler-Nordheim plot, as introduced by Stern et al. in 1929, the quantity ln{i/V2} is plotted against 1/V. The original thinking was that (as predicted by the original or the elementary Fowler-Nordheim-type equation) this would generate an exact straight line of slope SFN. SFN would be related to the parameters that appear in the exponent of a Fowler-Nordheim-type equation of i-V form by:Hence, knowledge of φ would allow β to be determined, or vice-versa.
[In principle, in system geometries where there is local field-enhancing nanostructure present, and the macroscopic conversion factor βM can be determined, knowledge of β then allows the value of the emitter's effective field enhancement factor γ to be determined from the formula γ = β/βM. In the common case of a film emitter generated on one plate of a two-plate arrangement with plate-separation W (so βM = 1/W) then
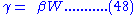
Nowadays, this is one of the most likely applications of Fowler-Nordheim plots.]
It subsequently became clear that the original thinking above is strictly correct only for the physically unrealistic situation of a flat emitter and an exact triangular barrier. For real emitters and real barriers a "slope correction factor" σFN has to be introduced, yielding the revised formula
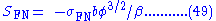
The value of σFN will, in principle, be influenced by any parameter in the physically complete Fowler-Nordheim-type equation for i(V) that has a voltage dependence.
At present, the only parameter that is considered important is the correction factor
relating to the barrier shape, and the only barrier for which there is any well-established detailed theory is the Schottky-Nordheim barrier. In this case, σFN is given by a mathematical function called s. This function s was first tabulated correctly (as a function of the Nordheim parameter y) by Burgess, KroemerHerbert Kroemer
Herbert Kroemer , a professor of electrical and computer engineering at the University of California, Santa Barbara, received his Ph.D. in theoretical physics in 1952 from the University of Göttingen, Germany, with a dissertation on hot electron effects in the then-new transistor, setting the stage...
and Houston in 1953; and a modern treatment that gives s as function of the scaled barrier field f for a Schottky-Nordheim barrier is given in. However, it has long been clear that, for practical emitter operation, the value of s lies in the range 0.9 to 1.
In practice, due to the extra complexity involved in taking the slope correction factor into detailed account, many authors (in effect) put σFN = 1 in eq. (49), thereby generating a systematic error in their estimated values of β and/or γ, thought usually to be around 5%.
However, empirical equation (42) – which in principle is more general than Fowler-Nordheim-type equations - brings with it possible new ways of analyzing field emission i-V data. In general, it may be assumed that the parameter B in the empirical equation is related to the unreduced height H of some characteristic barrier seen by tunneling electrons by
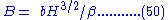
(In most cases, but not necessarily all, H would be equal to the local work-function; certainly this is true for metals.) The issue is how to determine the value of B by experiment. There are two obvious ways. (1) Suppose that eq. (43) can be used to determine a reasonably accurate experimental value of κ, from the slope of a plot of form [–dln{i}/d(1/V) vs. V]. In this case, a second plot, of ln(i)/Vκ vs. 1/V, should be an exact straight line of slope –B. This approach should be the most accurate way of determining B.
(2) Alternatively, if the value of κ is not exactly known, and cannot be accurately measured, but can be estimated or guessed, then a value for B can be derived from a plot of the form [ln{i} vs. 1/V]. This is the form of plot used by Millikan and Lauritsen in 1928. Re-arranging eq. (43) gives
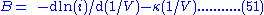
Thus, B can be determined, to a good degree of approximation, by determining the mean slope of a Millikan-Lauritsen plot over some range of values of 1/V, and by applying a correction, using the value of 1/V at the mid-point of the range and an assumed value of κ.
The main advantages of using a Millikan-Lauritsen plot, and this form of correction procedure, rather than a Fowler-Nordheim plot and a slope correction factor, are seen to be the following. (1) The plotting procedure is marginally more straightforward. (2) The correction involves a physical parameter (V) that is a measured quantity, rather than a physical parameter (f) that has to be calculated [in order to then calculate a value of s(f) or, more generally σFN(f)]. (3) Both the parameter κ itself, and the correction procedure, are more transparent (and more readily understood) than the Fowler-Nordheim-plot equivalents. (4) This procedure takes into account all physical effects that influence the value of κ, whereas the Fowler-Nordheim-plot correction procedure (in the form in which it has been carried out for the last 50 years) takes into account only those effects associated with barrier shape – assuming, furthermore, that this shape is that of a Schottky-Nordheim barrier. (5) There is a cleaner separation of theoretical and technological concerns: theoreticians will be interested in establishing what information any measured values of κ provide about CFE theory; but experimentalists can simply use measured values of κ to make more accurate estimates (if needed) of field enhancement factors.
This correction procedure for Millikan-Lauritsen plots will become easier to apply when a sufficient number of measurements of κ have been made, and a better idea is available of what typical values actually are. At present, it seems probable that for most materials κ will lie in the range -1<κ<3.
Further theoretical information
Developing the approximate theory of CFE from metals above is comparatively easy, for the following reasons. (1) Sommerfeld's free-electron theoryFree electron model
In solid-state physics, the free electron model is a simple model for the behaviour of valence electrons in a crystal structure of a metallic solid. It was developed principally by Arnold Sommerfeld who combined the classical Drude model with quantum mechanical Fermi-Dirac statistics and hence it...
, with its particular assumptions about the distribution of internal electron states in energy, applies adequately to many metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
s as a first approximation. (2) Most of the time, metals have no surface states
Surface states
Surface states are electronic states found at the surface of materials. They are formed due to the sharp transition from solid material that ends with a surface and are found only at the atom layers closest to the surface. The termination of a material with a surface leads to a change of the...
and (in many cases) metal wave-functions
Wavefunction
Not to be confused with the related concept of the Wave equationA wave function or wavefunction is a probability amplitude in quantum mechanics describing the quantum state of a particle and how it behaves. Typically, its values are complex numbers and, for a single particle, it is a function of...
have no significant "surface resonances
Surface states
Surface states are electronic states found at the surface of materials. They are formed due to the sharp transition from solid material that ends with a surface and are found only at the atom layers closest to the surface. The termination of a material with a surface leads to a change of the...
". (3) Metals have a high density of states
Density of states
In solid-state and condensed matter physics, the density of states of a system describes the number of states per interval of energy at each energy level that are available to be occupied by electrons. Unlike isolated systems, like atoms or molecules in gas phase, the density distributions are not...
at the Fermi level, so the charge that generates/screens external electric fields lies mainly on the outside of the top atomic layer, and no meaningful "field penetration" occurs. (4) Metals have high electrical conductivity: no significant voltage drops occur inside metal
Metal
A metal , is an element, compound, or alloy that is a good conductor of both electricity and heat. Metals are usually malleable and shiny, that is they reflect most of incident light...
emitters: this means that there are no factors obstructing the supply of electrons to the emitting surface, and that the electrons in this region can be both in effective local thermodynamic equilibrium
Thermodynamic equilibrium
In thermodynamics, a thermodynamic system is said to be in thermodynamic equilibrium when it is in thermal equilibrium, mechanical equilibrium, radiative equilibrium, and chemical equilibrium. The word equilibrium means a state of balance...
and in effective thermodynamic equilibrium with the electrons in the metal support structure on which the emitter is mounted. (5) Atomic-level effects are disregarded.
The development of "simple" theories of field electron emission, and in particular the development of Fowler-Nordheim-type equations, relies on all five of the above factors being true. For materials other than metals (and for atomically sharp metal emitters) one or more of the above factors will be untrue. For example, crystalline semiconductors do not have a free-electron-like band-structure, do have surface states, are subject to field penetration and band bending
Band bending
Band bending refers to the local change in energy of electrons at a semiconductor junction due to space charge effects. Because the common way to visualize the electron energy states and Fermi level in a material is to draw bands on an energy vs...
, and may exhibit both internal voltage drops and statistical decoupling of the surface-state electron distribution from the electron distribution in the surface region of the bulk band-structure (this decoupling is known as "the Modinos effect").
This, basically, is why this article is confined to the theory of CFE from bulk metals. The complications involved in presenting CFE theory for non-metals are too great to be dealt with satisfactorily via Wikipedia. In any case, the basic theory of CFE from bulk metals needs to be understood first.
In practice, the theory of the actual Fowler-Nordheim tunneling process is much the same for all materials (though details of barrier shape may vary, and modified theory has to be developed for initial states that are localized rather than are travelling-wave-like). However, notwithstanding such differences, one expects (for thermodynamic equilibrium
Thermodynamic equilibrium
In thermodynamics, a thermodynamic system is said to be in thermodynamic equilibrium when it is in thermal equilibrium, mechanical equilibrium, radiative equilibrium, and chemical equilibrium. The word equilibrium means a state of balance...
situations) that all CFE equations will have exponents that behave in a generally similar manner. This is why applying Fowler-Nordheim-type equations to materials outside the scope of the derivations given here often works. If interest is only in parameters (such as field enhancement factor) that relate to the slope of Fowler-Nordheim or Millikan-Lauritsen plots and to the exponent of the CFE equation, then Fowler-Nordheim-type theory will often give sensible estimates. However, attempts to derive meaningful current density values will usually or always fail.
Note that a straight line in a Fowler-Nordheim or Millikan-Lauritsen plot does not indicate that emission from the corresponding material obeys a Fowler-Nordheim-type equation: it indicates only that the emission mechanism for individual electrons is probably Fowler-Nordheim tunneling.
Different materials may have radically different distributions in energy of their internal electron states, so the process of integrating current-density contributions over the internal electron states may give rise to significantly different expressions for the current-density pre-exponentials, for different classes of material. In particular, the power of barrier field appearing in the pre-exponential may be different from the original Fowler-Nordheim value "2". Investigation of effects of this kind is an active research topic. Atomic-level "resonance" and "scattering
Scattering
Scattering is a general physical process where some forms of radiation, such as light, sound, or moving particles, are forced to deviate from a straight trajectory by one or more localized non-uniformities in the medium through which they pass. In conventional use, this also includes deviation of...
" effects, if they occur, will also modify the theory.
Where materials are subject to field penetration and band bending, a necessary preliminary is to have good theories of such effects (for each different class of material) before detailed theories of CFE can be developed. Where voltage-drop effects occur, then the theory of the emission current may, to a greater or lesser extent, become theory that involves internal transport effects, and may become very complex.
See also
- Field emission microscope
- Field emitter arrayField emitter arrayA field emitter array is a particular form of large-area field electron source. FEAs are prepared on a silicon substrate by lithographic techniques similar to those used in the fabrication of integrated circuits. Their structure consists of a very large number of individual, similar, small field...
- Field emission displayField emission displayA field emission display is a display technology that incorporates flat panel display technology that uses large-area field electron emission sources to provide electrons that strike colored phosphor to produce a color image as a electronic visual display...
- Franz–Keldysh effect
Further reading
General informationField penetration and band bending (semiconductors)
- A. Many, Y. Goldstein, and N.B. Grover, Semiconductor Surfaces (North Holland, Amsterdam, 1965).
- W. Mönsch, Semiconductor Suraces and Interfaces (Springer, Berlin, 1995).
- J. Peng, Z.B. Li, et al. J. Appl. Phys. 104 (2008) 014310.
Field emitted vacuum space-charge
Space charge
Space charge is a concept in which excess electric charge is treated as a continuum of charge distributed over a region of space rather than distinct point-like charges...
Field emission at high temperatures, and photo-field emission
- K.L. Jensen, Electron Emission Physics, Adv. Imaging Electron Phys., Vol. 149 (Academic, New York, 2007).
Field-induced explosive electron emission
Ectons
Ectons are explosive electron emissions observed as individual packets or avalanches of electrons, occurring as microexplosions at the cathode. The electron current in an ecton starts flowing as a result of overheating of the metal cathode because of the high energy density , and stops when the...
- G.A. Mesyats, Explosive Electron Emission (URO Press, Ekaterinburg, 1998),