Henri Poincaré
Encyclopedia
Jules Henri Poincaré (ˈʒyl ɑ̃ˈʁi pwɛ̃nkaˈʁe) was a French
mathematician
, theoretical physicist, engineer
, and a philosopher of science
. He is often described as a polymath
, and in mathematics as The Last Universalist, since he excelled in all fields of the discipline as it existed during his lifetime.
As a mathematician and physicist, he made many original fundamental contributions to pure
and applied mathematics
, mathematical physics
, and celestial mechanics
. He was responsible for formulating the Poincaré conjecture
, one of the most famous unsolved problems in mathematics, until it was solved in 2002–3. In his research on the three-body problem
, Poincaré became the first person to discover a chaotic deterministic system which laid the foundations of modern chaos theory
. He is also considered to be one of the founders of the field of topology
.
Poincaré introduced the modern principle of relativity
and was the first to present the Lorentz transformations in their modern symmetrical form. Poincaré discovered the remaining relativistic velocity transformations and recorded them in a letter to Dutch physicist Hendrik Lorentz
(1853–1928) in 1905. Thus he obtained perfect invariance of all of Maxwell's equations
, an important step in the formulation of the theory of special relativity
.
The Poincaré group
used in physics and mathematics was named after him.
. Another notable member of Jules' family was his cousin, Raymond Poincaré
, who would become the President of France, 1913 to 1920, and a fellow member of the Académie française
.
and received special instruction from his mother, Eugénie Launois (1830–1897).
In 1862, Henri entered the Lycée in Nancy (now renamed the Lycée Henri Poincaré in his honour, along with the University of Nancy). He spent eleven years at the Lycée and during this time he proved to be one of the top students in every topic he studied. He excelled in written composition. His mathematics teacher described him as a "monster of mathematics" and he won first prizes in the concours général
, a competition between the top pupils from all the Lycées across France. His poorest subjects were music and physical education, where he was described as "average at best" (O'Connor et al., 2002). However, poor eyesight and a tendency towards absentmindedness may explain these difficulties (Carl, 1968). He graduated from the Lycée in 1871 with a Bachelor's degree in letters and sciences.
During the Franco-Prussian War
of 1870 he served alongside his father in the Ambulance Corps.
Poincaré entered the École Polytechnique
in 1873. There he studied mathematics as a student of Charles Hermite
, continuing to excel and publishing his first paper (Démonstration nouvelle des propriétés de l'indicatrice d'une surface) in 1874. He graduated in 1875 or 1876. He went on to study at the École des Mines, continuing to study mathematics in addition to the mining engineering syllabus and received the degree of ordinary engineer in March 1879.
As a graduate of the École des Mines he joined the Corps des Mines as an inspector for the Vesoul
region in northeast France. He was on the scene of a mining disaster at Magny
in August 1879 in which 18 miners died. He carried out the official investigation into the accident in a characteristically thorough and humane way.
At the same time, Poincaré was preparing for his doctorate in sciences in mathematics under the supervision of Charles Hermite. His doctoral thesis was in the field of differential equations. It was named Sur les propriétés des fonctions définies par les équations différences. Poincaré devised a new way of studying the properties of these equations. He not only faced the question of determining the integral of such equations, but also was the first person to study their general geometric properties. He realised that they could be used to model the behaviour of multiple bodies in free motion within the solar system
. Poincaré graduated from the University of Paris
in 1879.
, but he never fully abandoned his mining career to mathematics. He worked at the Ministry of Public Services as an engineer in charge of northern railway development from 1881 to 1885. He eventually became chief engineer of the Corps de Mines in 1893 and inspector general in 1910.
Beginning in 1881 and for the rest of his career, he taught at the University of Paris (the Sorbonne
). He was initially appointed as the maître de conférences d'analyse (associate professor of analysis) (Sageret, 1911). Eventually, he held the chairs of Physical and Experimental Mechanics, Mathematical Physics and Theory of Probability, and Celestial Mechanics and Astronomy.
Also in that same year, Poincaré married Miss Poulain d'Andecy. Together they had four children: Jeanne (born 1887), Yvonne (born 1889), Henriette (born 1891), and Léon (born 1893).
In 1887, at the young age of 32, Poincaré was elected to the French Academy of Sciences
. He became its president in 1906, and was elected to the Académie française
in 1909.
In 1887 he won Oscar II, King of Sweden
's mathematical competition for a resolution of the three-body problem
concerning the free motion of multiple orbiting bodies. (See #The three-body problem section below)
In 1893, Poincaré joined the French Bureau des Longitudes
, which engaged him in the synchronisation of time around the world. In 1897 Poincaré backed an unsuccessful proposal for the decimalisation of circular measure
, and hence time and longitude
(see Galison 2003). It was this post which led him to consider the question of establishing international time zones and the synchronisation of time between bodies in relative motion. (See #Work on relativity section below)
In 1899, and again more successfully in 1904, he intervened in the trials of Alfred Dreyfus
. He attacked the spurious scientific claims of some of the evidence brought against Dreyfus, who was a Jewish officer in the French army charged with treason by fellow colleagues.
In 1912, Poincaré underwent surgery for a prostate
problem and subsequently died from an embolism
on 17 July 1912, in Paris. He was 58 years of age. He is buried in the Poincaré family vault in the Cemetery of Montparnasse, Paris.
A former French Minister of Education, Claude Allègre
, has recently (2004) proposed that Poincaré be reburied in the Panthéon
in Paris, which is reserved for French citizens only of the highest honour.
(1900) and Dimitrie Pompeiu
(1905).
, fluid mechanics
, optics
, electricity
, telegraphy
, capillarity, elasticity
, thermodynamics
, potential theory
, quantum theory
, theory of relativity
and physical cosmology
.
He was also a populariser of mathematics and physics and wrote several books for the lay public.
Among the specific topics he contributed to are the following:
time. This was known originally as the three-body problem and later the n-body problem
, where n is any number of more than two orbiting bodies. The n-body solution was considered very important and challenging at the close of the nineteenth century. Indeed in 1887, in honour of his 60th birthday, Oscar II, King of Sweden
, advised by Gösta Mittag-Leffler, established a prize for anyone who could find the solution to the problem. The announcement was quite specific:
In case the problem could not be solved, any other important contribution to classical mechanics would then be considered to be prizeworthy. The prize was finally awarded to Poincaré, even though he did not solve the original problem.
One of the judges, the distinguished Karl Weierstrass
, said, "This work cannot indeed be considered as furnishing the complete solution of the question proposed, but that it is nevertheless of such importance that its publication will inaugurate a new era in the history of celestial mechanics."
(The first version of his contribution even contained a serious error; for details see the article by Diacu). The version finally printed contained many important ideas which led to the theory of chaos
. The problem as stated originally was finally solved by Karl F. Sundman for n = 3 in 1912 and was generalised to the case of n > 3 bodies by Qiudong Wang
in the 1990s.
"), could be synchronised. At the same time Dutch
theorist Hendrik Lorentz
was developing Maxwell's theory into a theory of the motion of charged particles ("electrons" or "ions"), and their interaction with radiation. In 1895 Lorentz had introduced an auxiliary quantity (without physical interpretation) called "local time"
and introduced the hypothesis of length contraction
to explain the failure of optical and electrical experiments to detect motion relative to the aether (see Michelson–Morley experiment).
Poincaré was a constant interpreter (and sometimes friendly critic) of Lorentz's theory. Poincaré as a philosopher was interested in the "deeper meaning". Thus he interpreted Lorentz's theory and in so doing he came up with many insights that are now associated with special relativity. In The Measure of Time (1898), Poincaré said, "
A little reflection is sufficient to understand that all these affirmations have by themselves no meaning. They can have one only as the result of a convention." He also argued that scientists have to set the constancy of the speed of light as a postulate to give physical theories the simplest form.
Based on these assumptions he discussed in 1900 Lorentz's "wonderful invention" of local time and remarked that it arose when moving clocks are synchronised by exchanging light signals assumed to travel with the same speed in both directions in a moving frame.
and named it the principle of relativity
in 1904, according to which no physical experiment can discriminate between a state of uniform motion and a state of rest.
In 1905 Poincaré wrote to Lorentz about Lorentz's paper of 1904, which Poincaré described as a "paper of supreme importance." In this letter he pointed out an error Lorentz had made when he had applied his transformation to one of Maxwell's equations, that for charge-occupied space, and also questioned the time dilation factor given by Lorentz.
In a second letter to Lorentz, Poincaré gave his own reason why Lorentz's time dilation factor was indeed correct after all: it was necessary to make the Lorentz transformation form a group and gave what is now known as the relativistic velocity-addition law.
Poincaré later delivered a paper at the meeting of the Academy of Sciences in Paris on 5 June 1905 in which these issues were addressed. In the published version of that he wrote:
and showed that the arbitrary function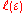
must be unity for all 
(Lorentz had set 
by a different argument) to make the transformations form a group. In an enlarged version of the paper that appeared in 1906 Poincaré pointed out that the combination 
is invariant
. He noted that a Lorentz transformation is merely a rotation in four-dimensional space about the origin by introducing
as a fourth imaginary coordinate, and he used an early form of four-vector
s. Poincaré expressed a disinterest in a four-dimensional reformulation of his new mechanics in 1907, because in his opinion the translation of physics into the language of four-dimensional geometry would entail too much effort for limited profit. So it was Hermann Minkowski
who worked out the consequences of this notion in 1907.
, he tried to determine whether the center of gravity
still moves with a uniform velocity when electromagnetic fields are included. He noticed that the action/reaction principle does not hold for matter alone, but that the electromagnetic field has its own momentum. Poincaré concluded that the electromagnetic field energy of an electromagnetic wave behaves like a fictitious fluid
("fluide fictif") with a mass density of E/c2. If the center of mass frame is defined by both the mass of matter and the mass of the fictitious fluid, and if the fictitious fluid is indestructible—it's neither created or destroyed—then the motion of the center of mass frame remains uniform. But electromagnetic energy can be converted into other forms of energy. So Poincaré assumed that there exists a non-electric energy fluid at each point of space, into which electromagnetic energy can be transformed and which also carries a mass proportional to the energy. In this way, the motion of the center of mass remains uniform. Poincaré said that one should not be too surprised by these assumptions, since they are only mathematical fictions.
However, Poincaré's resolution led to a paradox when changing frames: if a Hertzian oscillator radiates in a certain direction, it will suffer a recoil
from the inertia of the fictitious fluid. Poincaré performed a Lorentz boost (to order v/c) to the frame of the moving source. He noted that energy conservation holds in both frames, but that the law of conservation of momentum is violated. This would allow perpetual motion
, a notion which he abhorred. The laws of nature would have to be different in the frames of reference, and the relativity principle would not hold. Therefore he argued that also in this case there has to be another compensating mechanism in the ether.
Poincaré himself came back to this topic in his St. Louis lecture (1904). This time (and later also in 1908) he rejected the possibility that energy carries mass and criticized the ether solution to compensate the above mentioned problems:
He also discussed two other unexplained effects: (1) non-conservation of mass implied by Lorentz's variable mass
, Abraham's theory of variable mass and Kaufmann
's experiments on the mass of fast moving electrons and (2) the non-conservation of energy in the radium experiments of Madame Curie.
It was Albert Einstein
's concept of mass–energy equivalence (1905) that a body losing energy as radiation or heat was losing mass of amount m = E/c2 that resolved Poincaré's paradox, without using any compensating mechanism within the ether. The Hertzian oscillator loses mass in the emission process, and momentum is conserved in any frame. However, concerning Poincaré's solution of the Center of Gravity problem, Einstein noted that Poincaré's formulation and his own from 1906 were mathematically equivalent.
) that Poincaré (1900) had described, but was remarkable in that it contained no references at all. Poincaré never acknowledged Einstein's work on special relativity
. Einstein acknowledged Poincaré in the text of a lecture
in 1921 called Geometrie und Erfahrung in connection with non-Euclidean geometry
, but not in connection with special relativity. A few years before his death Einstein commented on Poincaré as being one of the pioneers of relativity, saying "Lorentz had already recognised that the transformation named after him is essential for the analysis of Maxwell's equations, and Poincaré deepened this insight still further ...."
The mathematician Darboux claimed he was un intuitif (intuitive), arguing that this is demonstrated by the fact that he worked so often by visual representation. He did not care about being rigorous and disliked logic. He believed that logic was not a way to invent but a way to structure ideas and that logic limits ideas.
These abilities were offset to some extent by his shortcomings:
In addition, Toulouse stated that most mathematicians worked from principles already established while Poincaré started from basic principles each time (O'Connor et al., 2002).
His method of thinking is well summarised as:
's theory of transfinite number
s, and referred to it as a "disease" from which mathematics would eventually be cured.
Poincaré said, "There is no actual infinite; the Cantorians have forgotten this, and that is why they have fallen into contradiction."
and finance as peripheral. In 1900 Poincaré commented on Louis Bachelier
's thesis "The Theory of Speculation", saying: "M. Bachelier has evidenced an original and precise mind [but] the subject is somewhat remote from those our other candidates are in the habit of treating." (Bernstein, 1996, pp. 199–200) Bachelier's work explained what was then the French government's pricing options on French Bonds and anticipated many of the pricing theories in financial markets used today.
Named after him
and Gottlob Frege
, who believed that mathematics was a branch of logic
. Poincaré strongly disagreed, claiming that intuition
was the life of mathematics. Poincaré gives an interesting point of view in his book Science and Hypothesis:
Poincaré believed that arithmetic
is a synthetic
science. He argued that Peano's axioms cannot be proven non-circularly with the principle of induction (Murzi, 1998), therefore concluding that arithmetic is a priori
synthetic and not analytic. Poincaré then went on to say that mathematics cannot be deduced from logic since it is not analytic. His views were similar to those of Immanuel Kant
(Kolak, 2001, Folina 1992). He strongly opposed Cantorian set theory
, objecting to its use of impredicative definitions.
However, Poincaré did not share Kantian views in all branches of philosophy and mathematics. For example, in geometry, Poincaré believed that the structure of non-Euclidean space
can be known analytically. Poincaré held that convention plays an important role in physics. His view (and some later, more extreme versions of it) came to be known as "conventionalism
". Poincaré believed that Newton's first law was not empirical but is a conventional framework assumption for mechanics. He also believed that the geometry of physical space is conventional. He considered examples in which either the geometry of the physical fields or gradients of temperature can be changed, either describing a space as non-Euclidean measured by rigid rulers, or as a Euclidean space where the rulers are expanded or shrunk by a variable heat distribution. However, Poincaré thought that we were so accustomed to Euclidean geometry
that we would prefer to change the physical laws to save Euclidean geometry rather than shift to a non-Euclidean physical geometry.
as the source for the idea that creativity
and invention
consist of two mental stages, first random combinations of possible solutions to a problem, followed by a critical evaluation.
Although he most often spoke of a deterministic universe, Poincaré said that the subconscious generation of new possibilities involves chance
.
Poincaré's two stages—random combinations followed by selection—became the basis for Daniel Dennett
's two-stage model of free will
.
:; This book includes the English translations of Science and Hypothesis (1902), The Value of Science (1905), Science and Method (1908).
On algebraic topology
:
On celestial mechanics
:
On the philosophy of mathematics
:
French people
The French are a nation that share a common French culture and speak the French language as a mother tongue. Historically, the French population are descended from peoples of Celtic, Latin and Germanic origin, and are today a mixture of several ethnic groups...
mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
, theoretical physicist, engineer
Engineer
An engineer is a professional practitioner of engineering, concerned with applying scientific knowledge, mathematics and ingenuity to develop solutions for technical problems. Engineers design materials, structures, machines and systems while considering the limitations imposed by practicality,...
, and a philosopher of science
Philosophy of science
The philosophy of science is concerned with the assumptions, foundations, methods and implications of science. It is also concerned with the use and merit of science and sometimes overlaps metaphysics and epistemology by exploring whether scientific results are actually a study of truth...
. He is often described as a polymath
Polymath
A polymath is a person whose expertise spans a significant number of different subject areas. In less formal terms, a polymath may simply be someone who is very knowledgeable...
, and in mathematics as The Last Universalist, since he excelled in all fields of the discipline as it existed during his lifetime.
As a mathematician and physicist, he made many original fundamental contributions to pure
Pure mathematics
Broadly speaking, pure mathematics is mathematics which studies entirely abstract concepts. From the eighteenth century onwards, this was a recognized category of mathematical activity, sometimes characterized as speculative mathematics, and at variance with the trend towards meeting the needs of...
and applied mathematics
Applied mathematics
Applied mathematics is a branch of mathematics that concerns itself with mathematical methods that are typically used in science, engineering, business, and industry. Thus, "applied mathematics" is a mathematical science with specialized knowledge...
, mathematical physics
Mathematical physics
Mathematical physics refers to development of mathematical methods for application to problems in physics. The Journal of Mathematical Physics defines this area as: "the application of mathematics to problems in physics and the development of mathematical methods suitable for such applications and...
, and celestial mechanics
Celestial mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of celestial objects. The field applies principles of physics, historically classical mechanics, to astronomical objects such as stars and planets to produce ephemeris data. Orbital mechanics is a subfield which focuses on...
. He was responsible for formulating the Poincaré conjecture
Poincaré conjecture
In mathematics, the Poincaré conjecture is a theorem about the characterization of the three-dimensional sphere , which is the hypersphere that bounds the unit ball in four-dimensional space...
, one of the most famous unsolved problems in mathematics, until it was solved in 2002–3. In his research on the three-body problem
Three-body problem
Three-body problem has two distinguishable meanings in physics and classical mechanics:# In its traditional sense the three-body problem is the problem of taking an initial set of data that specifies the positions, masses and velocities of three bodies for some particular point in time and then...
, Poincaré became the first person to discover a chaotic deterministic system which laid the foundations of modern chaos theory
Chaos theory
Chaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
. He is also considered to be one of the founders of the field of topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
.
Poincaré introduced the modern principle of relativity
Principle of relativity
In physics, the principle of relativity is the requirement that the equations describing the laws of physics have the same form in all admissible frames of reference....
and was the first to present the Lorentz transformations in their modern symmetrical form. Poincaré discovered the remaining relativistic velocity transformations and recorded them in a letter to Dutch physicist Hendrik Lorentz
Hendrik Lorentz
Hendrik Antoon Lorentz was a Dutch physicist who shared the 1902 Nobel Prize in Physics with Pieter Zeeman for the discovery and theoretical explanation of the Zeeman effect...
(1853–1928) in 1905. Thus he obtained perfect invariance of all of Maxwell's equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
, an important step in the formulation of the theory of special relativity
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
.
The Poincaré group
Poincaré group
In physics and mathematics, the Poincaré group, named after Henri Poincaré, is the group of isometries of Minkowski spacetime.-Simple explanation:...
used in physics and mathematics was named after him.
Life
Poincaré was born on 29 April 1854 in Cité Ducale neighborhood, Nancy, Meurthe-et-Moselle into an influential family (Belliver, 1956). His father Leon Poincaré (1828–1892) was a professor of medicine at the University of Nancy (Sagaret, 1911). His adored younger sister Aline married the spiritual philosopher Emile BoutrouxEmile Boutroux
Étienne Émile Marie Boutroux was an eminent 19th century French philosopher of science and religion, and an historian of philosophy. He was a firm opponent of materialism in science. He was a spiritual philosopher who defended the idea that religion and science are compatible at a time when the...
. Another notable member of Jules' family was his cousin, Raymond Poincaré
Raymond Poincaré
Raymond Poincaré was a French statesman who served as Prime Minister of France on five separate occasions and as President of France from 1913 to 1920. Poincaré was a conservative leader primarily committed to political and social stability...
, who would become the President of France, 1913 to 1920, and a fellow member of the Académie française
Académie française
L'Académie française , also called the French Academy, is the pre-eminent French learned body on matters pertaining to the French language. The Académie was officially established in 1635 by Cardinal Richelieu, the chief minister to King Louis XIII. Suppressed in 1793 during the French Revolution,...
.
Education
During his childhood he was seriously ill for a time with diphtheriaDiphtheria
Diphtheria is an upper respiratory tract illness caused by Corynebacterium diphtheriae, a facultative anaerobic, Gram-positive bacterium. It is characterized by sore throat, low fever, and an adherent membrane on the tonsils, pharynx, and/or nasal cavity...
and received special instruction from his mother, Eugénie Launois (1830–1897).
In 1862, Henri entered the Lycée in Nancy (now renamed the Lycée Henri Poincaré in his honour, along with the University of Nancy). He spent eleven years at the Lycée and during this time he proved to be one of the top students in every topic he studied. He excelled in written composition. His mathematics teacher described him as a "monster of mathematics" and he won first prizes in the concours général
Concours général
In France, the concours général is a national competition held every year between students of Première and Terminale in almost all subjects taught in both general, technological and professional high schools...
, a competition between the top pupils from all the Lycées across France. His poorest subjects were music and physical education, where he was described as "average at best" (O'Connor et al., 2002). However, poor eyesight and a tendency towards absentmindedness may explain these difficulties (Carl, 1968). He graduated from the Lycée in 1871 with a Bachelor's degree in letters and sciences.
During the Franco-Prussian War
Franco-Prussian War
The Franco-Prussian War or Franco-German War, often referred to in France as the 1870 War was a conflict between the Second French Empire and the Kingdom of Prussia. Prussia was aided by the North German Confederation, of which it was a member, and the South German states of Baden, Württemberg and...
of 1870 he served alongside his father in the Ambulance Corps.
Poincaré entered the École Polytechnique
École Polytechnique
The École Polytechnique is a state-run institution of higher education and research in Palaiseau, Essonne, France, near Paris. Polytechnique is renowned for its four year undergraduate/graduate Master's program...
in 1873. There he studied mathematics as a student of Charles Hermite
Charles Hermite
Charles Hermite was a French mathematician who did research on number theory, quadratic forms, invariant theory, orthogonal polynomials, elliptic functions, and algebra....
, continuing to excel and publishing his first paper (Démonstration nouvelle des propriétés de l'indicatrice d'une surface) in 1874. He graduated in 1875 or 1876. He went on to study at the École des Mines, continuing to study mathematics in addition to the mining engineering syllabus and received the degree of ordinary engineer in March 1879.
As a graduate of the École des Mines he joined the Corps des Mines as an inspector for the Vesoul
Vesoul
Vesoul is a commune in the Haute-Saône department in the region of Franche-Comté in eastern France.The town is the capital of the department, its inhabitants are known in French as Vésuliens.-Notable people:...
region in northeast France. He was on the scene of a mining disaster at Magny
Magny-lès-Jussey
Magny-lès-Jussey is a commune in the Haute-Saône department in the region of Franche-Comté in eastern France.-References:*...
in August 1879 in which 18 miners died. He carried out the official investigation into the accident in a characteristically thorough and humane way.
At the same time, Poincaré was preparing for his doctorate in sciences in mathematics under the supervision of Charles Hermite. His doctoral thesis was in the field of differential equations. It was named Sur les propriétés des fonctions définies par les équations différences. Poincaré devised a new way of studying the properties of these equations. He not only faced the question of determining the integral of such equations, but also was the first person to study their general geometric properties. He realised that they could be used to model the behaviour of multiple bodies in free motion within the solar system
Solar System
The Solar System consists of the Sun and the astronomical objects gravitationally bound in orbit around it, all of which formed from the collapse of a giant molecular cloud approximately 4.6 billion years ago. The vast majority of the system's mass is in the Sun...
. Poincaré graduated from the University of Paris
University of Paris
The University of Paris was a university located in Paris, France and one of the earliest to be established in Europe. It was founded in the mid 12th century, and officially recognized as a university probably between 1160 and 1250...
in 1879.
Career
Soon after, he was offered a post as junior lecturer in mathematics at Caen UniversityCaen University
The Université de Caen Basse-Normandie is a university in Caen, in France.-History:The institution was founded in 1432 by John of Lancaster, 1st Duke of Bedford, the first rector being a Cornishman, Michael Tregury, afterwards Archbishop of Dublin. It originally consisted of a faculty of Canon Law...
, but he never fully abandoned his mining career to mathematics. He worked at the Ministry of Public Services as an engineer in charge of northern railway development from 1881 to 1885. He eventually became chief engineer of the Corps de Mines in 1893 and inspector general in 1910.
Beginning in 1881 and for the rest of his career, he taught at the University of Paris (the Sorbonne
Sorbonne
The Sorbonne is an edifice of the Latin Quarter, in Paris, France, which has been the historical house of the former University of Paris...
). He was initially appointed as the maître de conférences d'analyse (associate professor of analysis) (Sageret, 1911). Eventually, he held the chairs of Physical and Experimental Mechanics, Mathematical Physics and Theory of Probability, and Celestial Mechanics and Astronomy.
Also in that same year, Poincaré married Miss Poulain d'Andecy. Together they had four children: Jeanne (born 1887), Yvonne (born 1889), Henriette (born 1891), and Léon (born 1893).
In 1887, at the young age of 32, Poincaré was elected to the French Academy of Sciences
French Academy of Sciences
The French Academy of Sciences is a learned society, founded in 1666 by Louis XIV at the suggestion of Jean-Baptiste Colbert, to encourage and protect the spirit of French scientific research...
. He became its president in 1906, and was elected to the Académie française
Académie française
L'Académie française , also called the French Academy, is the pre-eminent French learned body on matters pertaining to the French language. The Académie was officially established in 1635 by Cardinal Richelieu, the chief minister to King Louis XIII. Suppressed in 1793 during the French Revolution,...
in 1909.
In 1887 he won Oscar II, King of Sweden
Oscar II of Sweden
Oscar II , baptised Oscar Fredrik was King of Sweden from 1872 until his death and King of Norway from 1872 until 1905. The third son of King Oscar I of Sweden and Josephine of Leuchtenberg, he was a descendant of Gustav I of Sweden through his mother.-Early life:At his birth in Stockholm, Oscar...
's mathematical competition for a resolution of the three-body problem
Three-body problem
Three-body problem has two distinguishable meanings in physics and classical mechanics:# In its traditional sense the three-body problem is the problem of taking an initial set of data that specifies the positions, masses and velocities of three bodies for some particular point in time and then...
concerning the free motion of multiple orbiting bodies. (See #The three-body problem section below)
In 1893, Poincaré joined the French Bureau des Longitudes
Bureau des Longitudes
The Bureau des Longitudes is a French scientific institution, founded by decree of 25 June 1795 and charged with the improvement of nautical navigation, standardisation of time-keeping, geodesy and astronomical observation. During the 19th century, it was responsible for synchronizing clocks...
, which engaged him in the synchronisation of time around the world. In 1897 Poincaré backed an unsuccessful proposal for the decimalisation of circular measure
Decimal degrees
Decimal degrees express latitude and longitude geographic coordinates as decimal fractions and are used in many Geographic Information Systems , web mapping applications such as Google Maps, and GPS devices. Decimal degrees are an alternative to using degrees, minutes, and seconds...
, and hence time and longitude
Longitude
Longitude is a geographic coordinate that specifies the east-west position of a point on the Earth's surface. It is an angular measurement, usually expressed in degrees, minutes and seconds, and denoted by the Greek letter lambda ....
(see Galison 2003). It was this post which led him to consider the question of establishing international time zones and the synchronisation of time between bodies in relative motion. (See #Work on relativity section below)
In 1899, and again more successfully in 1904, he intervened in the trials of Alfred Dreyfus
Alfred Dreyfus
Alfred Dreyfus was a French artillery officer of Jewish background whose trial and conviction in 1894 on charges of treason became one of the most tense political dramas in modern French and European history...
. He attacked the spurious scientific claims of some of the evidence brought against Dreyfus, who was a Jewish officer in the French army charged with treason by fellow colleagues.
In 1912, Poincaré underwent surgery for a prostate
Prostate
The prostate is a compound tubuloalveolar exocrine gland of the male reproductive system in most mammals....
problem and subsequently died from an embolism
Embolism
In medicine, an embolism is the event of lodging of an embolus into a narrow capillary vessel of an arterial bed which causes a blockage in a distant part of the body.Embolization is...
on 17 July 1912, in Paris. He was 58 years of age. He is buried in the Poincaré family vault in the Cemetery of Montparnasse, Paris.
A former French Minister of Education, Claude Allègre
Claude Allègre
Claude Allègre is a French politician and scientist.- Scientific work :The main scientific area of Claude Allègre is geochemistry....
, has recently (2004) proposed that Poincaré be reburied in the Panthéon
Panthéon, Paris
The Panthéon is a building in the Latin Quarter in Paris. It was originally built as a church dedicated to St. Genevieve and to house the reliquary châsse containing her relics but, after many changes, now functions as a secular mausoleum containing the remains of distinguished French citizens...
in Paris, which is reserved for French citizens only of the highest honour.
Students
Poincaré had two notable doctoral students at the University of Paris, Louis BachelierLouis Bachelier
-External links:** Louis Bachelier webpage at the Université de Franche-Comté, Besançon / France. Text in French.** also from Index Funds Advisors, this discussion of...
(1900) and Dimitrie Pompeiu
Dimitrie Pompeiu
-Biography:After studying in Dorohoi and Bucharest, he went to France, where he studied mathematics at the University of Paris . He obtained a Ph.D. degree in mathematics in 1905 with a thesis, On the continuity of complex variable functions, written under the direction of Henri Poincaré...
(1905).
Summary
Poincaré made many contributions to different fields of pure and applied mathematics such as: celestial mechanicsCelestial mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of celestial objects. The field applies principles of physics, historically classical mechanics, to astronomical objects such as stars and planets to produce ephemeris data. Orbital mechanics is a subfield which focuses on...
, fluid mechanics
Fluid mechanics
Fluid mechanics is the study of fluids and the forces on them. Fluid mechanics can be divided into fluid statics, the study of fluids at rest; fluid kinematics, the study of fluids in motion; and fluid dynamics, the study of the effect of forces on fluid motion...
, optics
Optics
Optics is the branch of physics which involves the behavior and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behavior of visible, ultraviolet, and infrared light...
, electricity
Electricity
Electricity is a general term encompassing a variety of phenomena resulting from the presence and flow of electric charge. These include many easily recognizable phenomena, such as lightning, static electricity, and the flow of electrical current in an electrical wire...
, telegraphy
Telegraphy
Telegraphy is the long-distance transmission of messages via some form of signalling technology. Telegraphy requires messages to be converted to a code which is known to both sender and receiver...
, capillarity, elasticity
Elasticity (physics)
In physics, elasticity is the physical property of a material that returns to its original shape after the stress that made it deform or distort is removed. The relative amount of deformation is called the strain....
, thermodynamics
Thermodynamics
Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...
, potential theory
Potential theory
In mathematics and mathematical physics, potential theory may be defined as the study of harmonic functions.- Definition and comments :The term "potential theory" was coined in 19th-century physics, when it was realized that the fundamental forces of nature could be modeled using potentials which...
, quantum theory
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
, theory of relativity
Theory of relativity
The theory of relativity, or simply relativity, encompasses two theories of Albert Einstein: special relativity and general relativity. However, the word relativity is sometimes used in reference to Galilean invariance....
and physical cosmology
Physical cosmology
Physical cosmology, as a branch of astronomy, is the study of the largest-scale structures and dynamics of the universe and is concerned with fundamental questions about its formation and evolution. For most of human history, it was a branch of metaphysics and religion...
.
He was also a populariser of mathematics and physics and wrote several books for the lay public.
Among the specific topics he contributed to are the following:
- algebraic topologyAlgebraic topologyAlgebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
- the theory of analytic functions of several complex variablesSeveral complex variablesThe theory of functions of several complex variables is the branch of mathematics dealing with functionson the space Cn of n-tuples of complex numbers...
- the theory of abelian functionsAbelian varietyIn mathematics, particularly in algebraic geometry, complex analysis and number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functions...
- algebraic geometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
- Poincaré was responsible for formulating one of the most famous problems in mathematics, the Poincaré conjecturePoincaré conjectureIn mathematics, the Poincaré conjecture is a theorem about the characterization of the three-dimensional sphere , which is the hypersphere that bounds the unit ball in four-dimensional space...
, proven in 2003 by Grigori PerelmanGrigori PerelmanGrigori Yakovlevich Perelman is a Russian mathematician who has made landmark contributions to Riemannian geometry and geometric topology.In 1992, Perelman proved the soul conjecture. In 2002, he proved Thurston's geometrization conjecture...
. - Poincaré recurrence theoremPoincaré recurrence theoremIn mathematics, the Poincaré recurrence theorem states that certain systems will, after a sufficiently long time, return to a state very close to the initial state. The Poincaré recurrence time is the length of time elapsed until the recurrence. The result applies to physical systems in which...
- hyperbolic geometryHyperbolic geometryIn mathematics, hyperbolic geometry is a non-Euclidean geometry, meaning that the parallel postulate of Euclidean geometry is replaced...
- number theoryNumber theoryNumber theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
- the three-body problemN-body problemThe n-body problem is the problem of predicting the motion of a group of celestial objects that interact with each other gravitationally. Solving this problem has been motivated by the need to understand the motion of the Sun, planets and the visible stars...
- the theory of diophantine equationsDiophantine equationIn mathematics, a Diophantine equation is an indeterminate polynomial equation that allows the variables to be integers only. Diophantine problems have fewer equations than unknown variables and involve finding integers that work correctly for all equations...
- the theory of electromagnetismElectromagnetismElectromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
- the special theory of relativitySpecial relativitySpecial relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
- In an 1894 paper, he introduced the concept of the fundamental groupFundamental groupIn mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
. - In the field of differential equations Poincaré has given many results that are critical for the qualitative theory of differential equations, for example the Poincaré sphere and the Poincaré mapPoincaré mapIn mathematics, particularly in dynamical systems, a first recurrence map or Poincaré map, named after Henri Poincaré, is the intersection of a periodic orbit in the state space of a continuous dynamical system with a certain lower dimensional subspace, called the Poincaré section, transversal to...
. - Poincaré on "everybody's belief" in the Normal Law of Errors (see normal distribution for an account of that "law")
- Published an influential paper providing a novel mathematical argument in support of quantum mechanicsQuantum mechanicsQuantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
.
The three-body problem
The problem of finding the general solution to the motion of more than two orbiting bodies in the solar system had eluded mathematicians since Newton'sIsaac Newton
Sir Isaac Newton PRS was an English physicist, mathematician, astronomer, natural philosopher, alchemist, and theologian, who has been "considered by many to be the greatest and most influential scientist who ever lived."...
time. This was known originally as the three-body problem and later the n-body problem
N-body problem
The n-body problem is the problem of predicting the motion of a group of celestial objects that interact with each other gravitationally. Solving this problem has been motivated by the need to understand the motion of the Sun, planets and the visible stars...
, where n is any number of more than two orbiting bodies. The n-body solution was considered very important and challenging at the close of the nineteenth century. Indeed in 1887, in honour of his 60th birthday, Oscar II, King of Sweden
Oscar II of Sweden
Oscar II , baptised Oscar Fredrik was King of Sweden from 1872 until his death and King of Norway from 1872 until 1905. The third son of King Oscar I of Sweden and Josephine of Leuchtenberg, he was a descendant of Gustav I of Sweden through his mother.-Early life:At his birth in Stockholm, Oscar...
, advised by Gösta Mittag-Leffler, established a prize for anyone who could find the solution to the problem. The announcement was quite specific:
In case the problem could not be solved, any other important contribution to classical mechanics would then be considered to be prizeworthy. The prize was finally awarded to Poincaré, even though he did not solve the original problem.
One of the judges, the distinguished Karl Weierstrass
Karl Weierstrass
Karl Theodor Wilhelm Weierstrass was a German mathematician who is often cited as the "father of modern analysis".- Biography :Weierstrass was born in Ostenfelde, part of Ennigerloh, Province of Westphalia....
, said, "This work cannot indeed be considered as furnishing the complete solution of the question proposed, but that it is nevertheless of such importance that its publication will inaugurate a new era in the history of celestial mechanics."
(The first version of his contribution even contained a serious error; for details see the article by Diacu). The version finally printed contained many important ideas which led to the theory of chaos
Chaos theory
Chaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
. The problem as stated originally was finally solved by Karl F. Sundman for n = 3 in 1912 and was generalised to the case of n > 3 bodies by Qiudong Wang
Qiudong Wang
Qiudong Wang is an Associate Professor at the Department of Mathematics, the University of Arizona. In 1982 he received a B.S. at Nanjing University and in 1994 a Ph.D. at the University of Cincinnati....
in the 1990s.
Work on relativity
Local time
Poincaré's work at the Bureau des Longitudes on establishing international time zones led him to consider how clocks at rest on the Earth, which would be moving at different speeds relative to absolute space (or the "luminiferous aetherLuminiferous aether
In the late 19th century, luminiferous aether or ether, meaning light-bearing aether, was the term used to describe a medium for the propagation of light....
"), could be synchronised. At the same time Dutch
Netherlands
The Netherlands is a constituent country of the Kingdom of the Netherlands, located mainly in North-West Europe and with several islands in the Caribbean. Mainland Netherlands borders the North Sea to the north and west, Belgium to the south, and Germany to the east, and shares maritime borders...
theorist Hendrik Lorentz
Hendrik Lorentz
Hendrik Antoon Lorentz was a Dutch physicist who shared the 1902 Nobel Prize in Physics with Pieter Zeeman for the discovery and theoretical explanation of the Zeeman effect...
was developing Maxwell's theory into a theory of the motion of charged particles ("electrons" or "ions"), and their interaction with radiation. In 1895 Lorentz had introduced an auxiliary quantity (without physical interpretation) called "local time"
and introduced the hypothesis of length contraction
Length contraction
In physics, length contraction – according to Hendrik Lorentz – is the physical phenomenon of a decrease in length detected by an observer of objects that travel at any non-zero velocity relative to that observer...
to explain the failure of optical and electrical experiments to detect motion relative to the aether (see Michelson–Morley experiment).
Poincaré was a constant interpreter (and sometimes friendly critic) of Lorentz's theory. Poincaré as a philosopher was interested in the "deeper meaning". Thus he interpreted Lorentz's theory and in so doing he came up with many insights that are now associated with special relativity. In The Measure of Time (1898), Poincaré said, "
A little reflection is sufficient to understand that all these affirmations have by themselves no meaning. They can have one only as the result of a convention." He also argued that scientists have to set the constancy of the speed of light as a postulate to give physical theories the simplest form.
Based on these assumptions he discussed in 1900 Lorentz's "wonderful invention" of local time and remarked that it arose when moving clocks are synchronised by exchanging light signals assumed to travel with the same speed in both directions in a moving frame.
Principle of relativity and Lorentz transformations
He discussed the "principle of relative motion" in two papers in 1900and named it the principle of relativity
Principle of relativity
In physics, the principle of relativity is the requirement that the equations describing the laws of physics have the same form in all admissible frames of reference....
in 1904, according to which no physical experiment can discriminate between a state of uniform motion and a state of rest.
In 1905 Poincaré wrote to Lorentz about Lorentz's paper of 1904, which Poincaré described as a "paper of supreme importance." In this letter he pointed out an error Lorentz had made when he had applied his transformation to one of Maxwell's equations, that for charge-occupied space, and also questioned the time dilation factor given by Lorentz.
In a second letter to Lorentz, Poincaré gave his own reason why Lorentz's time dilation factor was indeed correct after all: it was necessary to make the Lorentz transformation form a group and gave what is now known as the relativistic velocity-addition law.
Poincaré later delivered a paper at the meeting of the Academy of Sciences in Paris on 5 June 1905 in which these issues were addressed. In the published version of that he wrote:
and showed that the arbitrary function
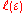must be unity for all (Lorentz had set by a different argument) to make the transformations form a group. In an enlarged version of the paper that appeared in 1906 Poincaré pointed out that the combination is invariantInvariant (mathematics)
In mathematics, an invariant is a property of a class of mathematical objects that remains unchanged when transformations of a certain type are applied to the objects. The particular class of objects and type of transformations are usually indicated by the context in which the term is used...
. He noted that a Lorentz transformation is merely a rotation in four-dimensional space about the origin by introducing
as a fourth imaginary coordinate, and he used an early form of four-vectorFour-vector
In the theory of relativity, a four-vector is a vector in a four-dimensional real vector space, called Minkowski space. It differs from a vector in that it can be transformed by Lorentz transformations. The usage of the four-vector name tacitly assumes that its components refer to a standard basis...
s. Poincaré expressed a disinterest in a four-dimensional reformulation of his new mechanics in 1907, because in his opinion the translation of physics into the language of four-dimensional geometry would entail too much effort for limited profit. So it was Hermann Minkowski
Hermann Minkowski
Hermann Minkowski was a German mathematician of Ashkenazi Jewish descent, who created and developed the geometry of numbers and who used geometrical methods to solve difficult problems in number theory, mathematical physics, and the theory of relativity.- Life and work :Hermann Minkowski was born...
who worked out the consequences of this notion in 1907.
Mass–energy relation
Like others before, Poincaré (1900) discovered a relation between mass and electromagnetic energy. While studying the conflict between the action/reaction principle and Lorentz ether theoryLorentz ether theory
What is now often called Lorentz Ether theory has its roots in Hendrik Lorentz's "Theory of electrons", which was the final point in the development of the classical aether theories at the end of the 19th and at the beginning of the 20th century....
, he tried to determine whether the center of gravity
Center of gravity
In physics, a center of gravity of a material body is a point that may be used for a summary description of gravitational interactions. In a uniform gravitational field, the center of mass serves as the center of gravity...
still moves with a uniform velocity when electromagnetic fields are included. He noticed that the action/reaction principle does not hold for matter alone, but that the electromagnetic field has its own momentum. Poincaré concluded that the electromagnetic field energy of an electromagnetic wave behaves like a fictitious fluid
Fluid
In physics, a fluid is a substance that continually deforms under an applied shear stress. Fluids are a subset of the phases of matter and include liquids, gases, plasmas and, to some extent, plastic solids....
("fluide fictif") with a mass density of E/c2. If the center of mass frame is defined by both the mass of matter and the mass of the fictitious fluid, and if the fictitious fluid is indestructible—it's neither created or destroyed—then the motion of the center of mass frame remains uniform. But electromagnetic energy can be converted into other forms of energy. So Poincaré assumed that there exists a non-electric energy fluid at each point of space, into which electromagnetic energy can be transformed and which also carries a mass proportional to the energy. In this way, the motion of the center of mass remains uniform. Poincaré said that one should not be too surprised by these assumptions, since they are only mathematical fictions.
However, Poincaré's resolution led to a paradox when changing frames: if a Hertzian oscillator radiates in a certain direction, it will suffer a recoil
Recoil
Recoil is the backward momentum of a gun when it is discharged. In technical terms, the recoil caused by the gun exactly balances the forward momentum of the projectile and exhaust gasses, according to Newton's third law...
from the inertia of the fictitious fluid. Poincaré performed a Lorentz boost (to order v/c) to the frame of the moving source. He noted that energy conservation holds in both frames, but that the law of conservation of momentum is violated. This would allow perpetual motion
Perpetual motion
Perpetual motion describes hypothetical machines that operate or produce useful work indefinitely and, more generally, hypothetical machines that produce more work or energy than they consume, whether they might operate indefinitely or not....
, a notion which he abhorred. The laws of nature would have to be different in the frames of reference, and the relativity principle would not hold. Therefore he argued that also in this case there has to be another compensating mechanism in the ether.
Poincaré himself came back to this topic in his St. Louis lecture (1904). This time (and later also in 1908) he rejected the possibility that energy carries mass and criticized the ether solution to compensate the above mentioned problems:
He also discussed two other unexplained effects: (1) non-conservation of mass implied by Lorentz's variable mass
, Abraham's theory of variable mass and KaufmannWalter Kaufmann (physicist)
Walter Kaufmann was a German physicist. He is most well known for his first experimental proof of the velocity dependence of mass, which was an important contribution to the development of modern physics, including special relativity.-Life:In 1890/91 he studied mechanical engineering at the...
's experiments on the mass of fast moving electrons and (2) the non-conservation of energy in the radium experiments of Madame Curie.
It was Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
's concept of mass–energy equivalence (1905) that a body losing energy as radiation or heat was losing mass of amount m = E/c2 that resolved Poincaré's paradox, without using any compensating mechanism within the ether. The Hertzian oscillator loses mass in the emission process, and momentum is conserved in any frame. However, concerning Poincaré's solution of the Center of Gravity problem, Einstein noted that Poincaré's formulation and his own from 1906 were mathematically equivalent.
Poincaré and Einstein
Einstein's first paper on relativity was published three months after Poincaré's short paper, but before Poincaré's longer version. It relied on the principle of relativity to derive the Lorentz transformations and used a similar clock synchronisation procedure (Einstein synchronisationEinstein synchronisation
Einstein synchronisation is a convention for synchronising clocks at different places by means of signal exchanges. This synchronisation method was used already by telegraphers in the middle 19th century, but was popularized by H. Poincaré and A...
) that Poincaré (1900) had described, but was remarkable in that it contained no references at all. Poincaré never acknowledged Einstein's work on special relativity
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
. Einstein acknowledged Poincaré in the text of a lecture
Lecture
thumb|A lecture on [[linear algebra]] at the [[Helsinki University of Technology]]A lecture is an oral presentation intended to present information or teach people about a particular subject, for example by a university or college teacher. Lectures are used to convey critical information, history,...
in 1921 called Geometrie und Erfahrung in connection with non-Euclidean geometry
Non-Euclidean geometry
Non-Euclidean geometry is the term used to refer to two specific geometries which are, loosely speaking, obtained by negating the Euclidean parallel postulate, namely hyperbolic and elliptic geometry. This is one term which, for historical reasons, has a meaning in mathematics which is much...
, but not in connection with special relativity. A few years before his death Einstein commented on Poincaré as being one of the pioneers of relativity, saying "Lorentz had already recognised that the transformation named after him is essential for the analysis of Maxwell's equations, and Poincaré deepened this insight still further ...."
Assessments
Poincaré's work in the development of special relativity is well recognised, though most historians stress that despite many similarities with Einstein's work, the two had very different research agendas and interpretations of the work. Poincaré developed a similar physical interpretation of local time and noticed the connection to signal velocity, but contrary to Einstein he continued to use the ether-concept in his papers and argued that clocks in the ether show the "true" time, and moving clocks show the local time. So Poincaré tried to keep the relativity principle in accordance with classical concepts, while Einstein developed a mathematically equivalent kinematics based on the new physical concepts of the relativity of space and time. While this is the view of most historians, a minority go much further, such as E.T. Whittaker, who held that Poincaré and Lorentz were the true discoverers of Relativity.Character
Poincaré's work habits have been compared to a bee flying from flower to flower. Poincaré was interested in the way his mind worked; he studied his habits and gave a talk about his observations in 1908 at the Institute of General Psychology in Paris. He linked his way of thinking to how he made several discoveries.The mathematician Darboux claimed he was un intuitif (intuitive), arguing that this is demonstrated by the fact that he worked so often by visual representation. He did not care about being rigorous and disliked logic. He believed that logic was not a way to invent but a way to structure ideas and that logic limits ideas.
Toulouse's characterisation
Poincaré's mental organisation was not only interesting to Poincaré himself but also to Toulouse, a psychologist of the Psychology Laboratory of the School of Higher Studies in Paris. Toulouse wrote a book entitled Henri Poincaré (1910). In it, he discussed Poincaré's regular schedule:- He worked during the same times each day in short periods of time. He undertook mathematical research for four hours a day, between 10 a.m. and noon then again from 5 p.m. to 7 p.m.. He would read articles in journals later in the evening.
- His normal work habit was to solve a problem completely in his head, then commit the completed problem to paper.
- He was ambidextrous and nearsighted.
- His ability to visualise what he heard proved particularly useful when he attended lectures, since his eyesight was so poor that he could not see properly what the lecturer wrote on the blackboard.
These abilities were offset to some extent by his shortcomings:
- He was physically clumsy and artistically inept.
- He was always in a rush and disliked going back for changes or corrections.
- He never spent a long time on a problem since he believed that the subconscious would continue working on the problem while he consciously worked on another problem.
In addition, Toulouse stated that most mathematicians worked from principles already established while Poincaré started from basic principles each time (O'Connor et al., 2002).
His method of thinking is well summarised as:
Attitude towards transfinite numbers
Poincaré was dismayed by Georg CantorGeorg Cantor
Georg Ferdinand Ludwig Philipp Cantor was a German mathematician, best known as the inventor of set theory, which has become a fundamental theory in mathematics. Cantor established the importance of one-to-one correspondence between the members of two sets, defined infinite and well-ordered sets,...
's theory of transfinite number
Transfinite number
Transfinite numbers are numbers that are "infinite" in the sense that they are larger than all finite numbers, yet not necessarily absolutely infinite. The term transfinite was coined by Georg Cantor, who wished to avoid some of the implications of the word infinite in connection with these...
s, and referred to it as a "disease" from which mathematics would eventually be cured.
Poincaré said, "There is no actual infinite; the Cantorians have forgotten this, and that is why they have fallen into contradiction."
View on economics
Poincaré saw mathematical work in economicsEconomics
Economics is the social science that analyzes the production, distribution, and consumption of goods and services. The term economics comes from the Ancient Greek from + , hence "rules of the house"...
and finance as peripheral. In 1900 Poincaré commented on Louis Bachelier
Louis Bachelier
-External links:** Louis Bachelier webpage at the Université de Franche-Comté, Besançon / France. Text in French.** also from Index Funds Advisors, this discussion of...
's thesis "The Theory of Speculation", saying: "M. Bachelier has evidenced an original and precise mind [but] the subject is somewhat remote from those our other candidates are in the habit of treating." (Bernstein, 1996, pp. 199–200) Bachelier's work explained what was then the French government's pricing options on French Bonds and anticipated many of the pricing theories in financial markets used today.
Honours
Awards- Oscar II, King of Sweden's mathematical competition (1887)
- American Philosophical SocietyAmerican Philosophical SocietyThe American Philosophical Society, founded in 1743, and located in Philadelphia, Pa., is an eminent scholarly organization of international reputation, that promotes useful knowledge in the sciences and humanities through excellence in scholarly research, professional meetings, publications,...
1899 - Gold Medal of the Royal Astronomical SocietyGold Medal of the Royal Astronomical Society-History:In the early years, more than one medal was often awarded in a year, but by 1833 only one medal was being awarded per year. This caused a problem when Neptune was discovered in 1846, because many felt an award should jointly be made to John Couch Adams and Urbain Le Verrier...
of London (1900) - Bolyai PrizeBolyai PrizeThe International Bolyai János Prize of Mathematics is an international prize for mathematicians founded by the Hungarian Academy of Sciences. The prize is awarded in every five years to mathematicians having published their monograph describing their own highly important new results in the past 10...
in 1905 - Matteucci MedalMatteucci MedalThe Matteucci Medal was established to award physicists for their fundamental contributions. Under an Italian Royal Decree dated July 10, 1870, the Italian Society of Sciences was authorized to receive a donation from Carlo Matteucci for the establishment of the Prize.Matteucci Medalists* 1868...
1905 - French Academy of SciencesFrench Academy of SciencesThe French Academy of Sciences is a learned society, founded in 1666 by Louis XIV at the suggestion of Jean-Baptiste Colbert, to encourage and protect the spirit of French scientific research...
1906 - Académie FrançaiseAcadémie françaiseL'Académie française , also called the French Academy, is the pre-eminent French learned body on matters pertaining to the French language. The Académie was officially established in 1635 by Cardinal Richelieu, the chief minister to King Louis XIII. Suppressed in 1793 during the French Revolution,...
1909 - Bruce MedalBruce MedalThe Catherine Wolfe Bruce Gold Medal is awarded every year by the Astronomical Society of the Pacific for outstanding lifetime contributions to astronomy. It is named after Catherine Wolfe Bruce, an American patroness of astronomy, and was first awarded in 1898...
(1911)
Named after him
- Poincaré PrizePoincaré PrizeThe Henri Poincaré Prize sponsored by the Daniel Iagolnitzer Foundation was created in 1997 to recognize outstanding contributions in mathematical physics, and contributions which lay the groundwork for novel developments in this broad field...
(Mathematical Physics International Prize) - Annales Henri PoincaréAnnales Henri PoincaréAnnales Henri Poincaré is a scientific journal which collects and publishes original research papers in the field of theoretical and mathematical physics. The emphasis is on "analytical theoretical and mathematical physics" in a broad sense...
(Scientific Journal) - Poincaré Seminar (nicknamed "BourbaphyBourbaphyThe Poincaré Seminars, named for the mathematician and theoretical physicist Henri Poincaré, were founded in 2001. They are nicknamed Bourbaphy for their inspiration by the Bourbaki Seminars....
") - The crater PoincaréPoincaré (crater)Poincaré is a large lunar impact basin that lies in the southern hemisphere on the far side of the Moon. Most of the formation has been heavily eroded by subsequent impacts, leaving a battered formation with only rugged remnants of the original outer rim to the west.The eastern half has been...
on the Moon - AsteroidAsteroidAsteroids are a class of small Solar System bodies in orbit around the Sun. They have also been called planetoids, especially the larger ones...
2021 Poincaré2021 Poincaré2021 Poincaré is a main belt asteroid that was discovered by the French astronomer Louis Boyer on June 26, 1936 at Algiers. The asteroid is named after the French mathematician Henri Poincaré.-External links:...
Philosophy
Poincaré had philosophical views opposite to those of Bertrand RussellBertrand Russell
Bertrand Arthur William Russell, 3rd Earl Russell, OM, FRS was a British philosopher, logician, mathematician, historian, and social critic. At various points in his life he considered himself a liberal, a socialist, and a pacifist, but he also admitted that he had never been any of these things...
and Gottlob Frege
Gottlob Frege
Friedrich Ludwig Gottlob Frege was a German mathematician, logician and philosopher. He is considered to be one of the founders of modern logic, and made major contributions to the foundations of mathematics. He is generally considered to be the father of analytic philosophy, for his writings on...
, who believed that mathematics was a branch of logic
Logic
In philosophy, Logic is the formal systematic study of the principles of valid inference and correct reasoning. Logic is used in most intellectual activities, but is studied primarily in the disciplines of philosophy, mathematics, semantics, and computer science...
. Poincaré strongly disagreed, claiming that intuition
Intuition (knowledge)
Intuition is the ability to acquire knowledge without inference or the use of reason. "The word 'intuition' comes from the Latin word 'intueri', which is often roughly translated as meaning 'to look inside'’ or 'to contemplate'." Intuition provides us with beliefs that we cannot necessarily justify...
was the life of mathematics. Poincaré gives an interesting point of view in his book Science and Hypothesis:
Poincaré believed that arithmetic
Arithmetic
Arithmetic or arithmetics is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple day-to-day counting to advanced science and business calculations. It involves the study of quantity, especially as the result of combining numbers...
is a synthetic
Analytic/synthetic distinction
The analytic–synthetic distinction is a conceptual distinction, used primarily in philosophy to distinguish propositions into two types: analytic propositions and synthetic propositions. Analytic propositions are true simply by virtue of their meaning, while synthetic propositions are not...
science. He argued that Peano's axioms cannot be proven non-circularly with the principle of induction (Murzi, 1998), therefore concluding that arithmetic is a priori
A priori and a posteriori (philosophy)
The terms a priori and a posteriori are used in philosophy to distinguish two types of knowledge, justifications or arguments...
synthetic and not analytic. Poincaré then went on to say that mathematics cannot be deduced from logic since it is not analytic. His views were similar to those of Immanuel Kant
Immanuel Kant
Immanuel Kant was a German philosopher from Königsberg , researching, lecturing and writing on philosophy and anthropology at the end of the 18th Century Enlightenment....
(Kolak, 2001, Folina 1992). He strongly opposed Cantorian set theory
Set theory
Set theory is the branch of mathematics that studies sets, which are collections of objects. Although any type of object can be collected into a set, set theory is applied most often to objects that are relevant to mathematics...
, objecting to its use of impredicative definitions.
However, Poincaré did not share Kantian views in all branches of philosophy and mathematics. For example, in geometry, Poincaré believed that the structure of non-Euclidean space
Non-Euclidean geometry
Non-Euclidean geometry is the term used to refer to two specific geometries which are, loosely speaking, obtained by negating the Euclidean parallel postulate, namely hyperbolic and elliptic geometry. This is one term which, for historical reasons, has a meaning in mathematics which is much...
can be known analytically. Poincaré held that convention plays an important role in physics. His view (and some later, more extreme versions of it) came to be known as "conventionalism
Conventionalism
Conventionalism is the philosophical attitude that fundamental principles of a certain kind are grounded on agreements in society, rather than on external reality...
". Poincaré believed that Newton's first law was not empirical but is a conventional framework assumption for mechanics. He also believed that the geometry of physical space is conventional. He considered examples in which either the geometry of the physical fields or gradients of temperature can be changed, either describing a space as non-Euclidean measured by rigid rulers, or as a Euclidean space where the rulers are expanded or shrunk by a variable heat distribution. However, Poincaré thought that we were so accustomed to Euclidean geometry
Euclidean geometry
Euclidean geometry is a mathematical system attributed to the Alexandrian Greek mathematician Euclid, which he described in his textbook on geometry: the Elements. Euclid's method consists in assuming a small set of intuitively appealing axioms, and deducing many other propositions from these...
that we would prefer to change the physical laws to save Euclidean geometry rather than shift to a non-Euclidean physical geometry.
Free will
Poincaré's famous lectures before the Société de Psychologie in Paris (published as Science and Hypothesis, The Value of Science, and Science and Method) were cited by Jacques HadamardJacques Hadamard
Jacques Salomon Hadamard FRS was a French mathematician who made major contributions in number theory, complex function theory, differential geometry and partial differential equations.-Biography:...
as the source for the idea that creativity
Creativity
Creativity refers to the phenomenon whereby a person creates something new that has some kind of value. What counts as "new" may be in reference to the individual creator, or to the society or domain within which the novelty occurs...
and invention
Invention
An invention is a novel composition, device, or process. An invention may be derived from a pre-existing model or idea, or it could be independently conceived, in which case it may be a radical breakthrough. In addition, there is cultural invention, which is an innovative set of useful social...
consist of two mental stages, first random combinations of possible solutions to a problem, followed by a critical evaluation.
Although he most often spoke of a deterministic universe, Poincaré said that the subconscious generation of new possibilities involves chance
Randomness
Randomness has somewhat differing meanings as used in various fields. It also has common meanings which are connected to the notion of predictability of events....
.
It is certain that the combinations which present themselves to the mind in a kind of sudden illumination after a somewhat prolonged period of unconscious work are generally useful and fruitful combinations... all the combinations are formed as a result of the automatic action of the subliminal ego, but those only which are interesting find their way into the field of consciousness... A few only are harmonious, and consequently at once useful and beautiful, and they will be capable of affecting the geometrician's special sensibility I have been speaking of; which, once aroused, will direct our attention upon them, and will thus give them the opportunity of becoming conscious... In the subliminal ego, on the contrary, there reigns what I would call liberty, if one could give this name to the mere absence of discipline and to disorder born of chance.
Poincaré's two stages—random combinations followed by selection—became the basis for Daniel Dennett
Daniel Dennett
Daniel Clement Dennett is an American philosopher, writer and cognitive scientist whose research centers on the philosophy of mind, philosophy of science and philosophy of biology, particularly as those fields relate to evolutionary biology and cognitive science. He is currently the Co-director of...
's two-stage model of free will
Two-stage model of free will
A two-stage model of free will separates the free stage from the will stage.In the first stage, alternative possibilities for thought and action are generated, in part indeterministically....
.
See also
Poincaré's writings in English translation
Popular writings on the philosophy of sciencePhilosophy of science
The philosophy of science is concerned with the assumptions, foundations, methods and implications of science. It is also concerned with the use and merit of science and sometimes overlaps metaphysics and epistemology by exploring whether scientific results are actually a study of truth...
:; This book includes the English translations of Science and Hypothesis (1902), The Value of Science (1905), Science and Method (1908).
- 1913.
On algebraic topology
Algebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
:
- 1895. Analysis situs. The first systematic study of topologyTopologyTopology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
.
On celestial mechanics
Celestial mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of celestial objects. The field applies principles of physics, historically classical mechanics, to astronomical objects such as stars and planets to produce ephemeris data. Orbital mechanics is a subfield which focuses on...
:
- 1892–99. New Methods of Celestial Mechanics, 3 vols. English trans., 1967. ISBN 1-56396-117-2.
- 1905–10. Lessons of Celestial Mechanics.
On the philosophy of mathematics
Philosophy of mathematics
The philosophy of mathematics is the branch of philosophy that studies the philosophical assumptions, foundations, and implications of mathematics. The aim of the philosophy of mathematics is to provide an account of the nature and methodology of mathematics and to understand the place of...
:
-
- Ewald, William B., ed., 1996. From Kant to Hilbert: A Source Book in the Foundations of Mathematics, 2 vols. Oxford Univ. Press. Contains the following works by Poincaré:
- 1894, "On the nature of mathematical reasoning," 972–81.
- 1898, "On the foundations of geometry," 982–1011.
- 1900, "Intuition and Logic in mathematics," 1012–20.
- 1905–06, "Mathematics and Logic, I–III," 1021–70.
- 1910, "On transfinite numbers," 1071–74.
General references
- Bell, Eric TempleEric Temple BellEric Temple Bell , was a mathematician and science fiction author born in Scotland who lived in the U.S. for most of his life...
, 1986. Men of Mathematics (reissue edition). Touchstone Books. ISBN 0-671-62818-6. - Belliver, André, 1956. Henri Poincaré ou la vocation souveraine. Paris: Gallimard.
- Bernstein, Peter L, 1996. "Against the Gods: A Remarkable Story of Risk". (p. 199–200). John Wiley & Sons.
- Boyer, B. Carl, 1968. A History of Mathematics: Henri Poincaré, John Wiley & Sons.
- Grattan-Guinness, IvorIvor Grattan-GuinnessIvor Grattan-Guinness, born 23 June 1941, in Bakewell, in England, is a historian of mathematics and logic.He gained his Bachelor degree as a Mathematics Scholar at Wadham College, Oxford, got an M.Sc in Mathematical Logic and the Philosophy of Science at the London School of Economics in 1966...
, 2000. The Search for Mathematical Roots 1870–1940. Princeton Uni. Press.. Internet version published in Journal of the ACMS 2004. - Folina, Janet, 1992. Poincare and the Philosophy of Mathematics. Macmillan, New York.
- Gray, Jeremy, 1986. Linear differential equations and group theory from Riemann to Poincaré, Birkhauser
- Kolak, Daniel, 2001. Lovers of Wisdom, 2nd ed. Wadsworth.
- Murzi, 1998. "Henri Poincaré".
- O'Connor, J. John, and Robertson, F. Edmund, 2002, "Jules Henri Poincaré". University of St. Andrews, Scotland.
- Peterson, IvarsIvars PetersonIvars Peterson is an award-winning mathematics writer.He is currently Director of Publications for Journals and Communications at the Mathematical Association of America....
, 1995. Newton's Clock: Chaos in the Solar System (reissue edition). W H Freeman & Co. ISBN 0-7167-2724-2. - Sageret, Jules, 1911. Henri Poincaré. Paris: Mercure de France.
- Toulouse, E.,1910. Henri Poincaré.—(Source biography in French) at University of Michigan Historic Math Collection.
External links
- Free audio download of Poincaré's Science and Hypothesis, from LibriVoxLibriVoxLibriVox is an online digital library of free public domain audiobooks, read by volunteers and is probably, since 2007, the world's most prolific audiobook publisher...
. - Internet Encyclopedia of PhilosophyInternet Encyclopedia of PhilosophyThe Internet Encyclopedia of Philosophy is a free online encyclopedia on philosophical topics and philosophers founded by James Fieser in 1995. The current general editors are James Fieser and Bradley Dowden...
: "Henri Poincare"—by Mauro Murzi. - Henri Poincaré on Information Philosopher
- A timeline of Poincaré's life University of Nancy (in French).
- Bruce Medal page
- Collins, Graham P., "Henri Poincaré, His Conjecture, Copacabana and Higher Dimensions," Scientific AmericanScientific AmericanScientific American is a popular science magazine. It is notable for its long history of presenting science monthly to an educated but not necessarily scientific public, through its careful attention to the clarity of its text as well as the quality of its specially commissioned color graphics...
, 9 June 2004. - BBC In Our Time, "Discussion of the Poincaré conjecture," 2 November 2006, hosted by Melvynn Bragg. See Internet Archive
- Poincare Contemplates Copernicus at MathPages
- High Anxieties - The Mathematics of Chaos (2008) BBC documentary directed by David MaloneDavid Malone (independent filmmaker)David Malone, author of The Debt Generation, is also director of acclaimed documentaries on philosophy, science and religion originally broadcast in the UK by the BBC and Channel 4.-Work:Malone's work includes...
looking at the influence of Poincaré's discoveries on 20th Century mathematics.